Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-28T13:26:56.086Z Has data issue: false hasContentIssue false
  • English
  • Français

Montel's theorem for subharmonic functions and solutions of partial differential equations

Published online by Cambridge University Press:  24 October 2008

A. F. Beardon
A. F. Beardon
Affiliation:
St Catharine's College, Cambridge
Get access Rights & Permissions [Opens in a new window]

Extract

1. A theorem of Montel (14), states that if f(z) is analytic and bounded in the half-strip

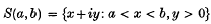
and if there exists an x0 in (a, b) such that

as y → ∞, then

as y → ∞ l. u. on (a, b)(locally uniformly on (a, b), i.e. uniformly on every compact subset of (a, b)). Bohr (3), has proved a version of this result applicable to functions analytic, but not necessarily bounded, in S(a, b) and Hardy, Ingham and Pólya (10), have considered whether or not f(z) can be replaced by |f(z)| in (1·1) and (1·2). They show that this is not so but prove that if f(z) is analytic and bounded in S(a, b) and if

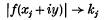
as y → ∞ for three distinct values x1, x2 and z3 in (a, b) then there exist constants A and B such that

as y → ∞, l.u. on (a, b). Cartwright (Theorem 5, (6)) has proved that if f(z) is analytic and satisfies |f(z)| < 1 in, S(a, b) and if for some x0 in (a, b)

asy → ∞, then

as y → ∞ co, l.u. on (a, b) and Bowen (5), has shown that if (1·4) is replaced by the weaker condition

as n → ∞ for some suitable sequence of points Zn in S(a, b) then (1·5) is still valid. In sections 2–5 of this paper we shall consider whether or not these results are valid |f(z)| is replaced by a subharmonic function.

Type
Research Article
Information
Mathematical Proceedings of the Cambridge Philosophical Society , Volume 69 , Issue 1 , January 1971 , pp. 123 - 150
Copyright
Copyright © Cambridge Philosophical Society 1971

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

REFERENCES

(1)Bers, L., John, F. and Screchter, M.Partial differential equations (Wiley and Sons, 1964).Google Scholar
(2)Boas, R. P.A primer of real functions (Carus monograph 13, Wiley and Sons, 1960).Google Scholar
(3)Bohr, H.On the limit values of analytic functions. J. London Math. Soc. 2 (1927), 180–181.CrossRefGoogle Scholar
(4)Bonsall, F. F.Note on a theorem of Hardy and Rogosinski. Quart. J. Math. Oxford Ser. 2 20 (1949), 254–256.CrossRefGoogle Scholar
(5)Bowen, N. A.On the limit of the modulus of a bounded regular function. Proc. Edinburgh Math. Soc. 14 (1964), 21–24.CrossRefGoogle Scholar
(6)Cartwright, M. L.A generalization of Montel's theorem. J. London Math. Soc. 37 (1962), 179–184.CrossRefGoogle Scholar
(7)Courant, R. and Hilbert, D.Methods of mathematical physics, Vol. 2 (Wiley and Sons, 1962).Google Scholar
(8)Friedman, A.Partial differential equations of parabolic type (Prentice-Hall, 1964).Google Scholar
(9)Hardy, G. H.A theorem concerning harmonic functions. J. London Math. Soc. 1 (1926), 130–131.CrossRefGoogle Scholar
(10)Hardy, G. H., Ingram, A. E. and Pólya, G.Notes on moduli and mean values. Proc. London Math. Soc. (2), 27 (1928), 401–409.CrossRefGoogle Scholar
(11)Hausdorff, F.Dimension und äusseres Mass. Math. Ann. 79 (1919), 157–179.CrossRefGoogle Scholar
(12)Hayman, W. K.The minimum modulus of large integral functions. Proc. London Math. Soc. (3), 2 (1952), 469–512.CrossRefGoogle Scholar
(13)Heins, M.Selected topics in the classical theory of functions of a complex variable (Holt, Rinehart and Winston, 1962).Google Scholar
(14)Montel, P.Sur les familles de fonctions analytiques qui admettent des valeurs exception-nelles dans un domaine. Ann. Sci. École Norm. Sup. (3), 23 (1912), 487–535.CrossRefGoogle Scholar
(15)Montel, P.Sur quelques families de fonctions harmoniques. Fund. Math. 25 (1935), 388–407.CrossRefGoogle Scholar
(16)Rado, T.Subharmonic functions (Chelsea, 1949).Google Scholar
(17)Serrin, J.On the Harnack inequality for linear elliptic equations. J. Analyse Math. 4 (1954–1956), 292–308.CrossRefGoogle Scholar