Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Griffeath, David
1975.
Uniform coupling of non-homogeneous Markov chains.
Journal of Applied Probability,
Vol. 12,
Issue. 4,
p.
753.
Griffeath, David
1975.
Uniform coupling of non-homogeneous Markov chains.
Journal of Applied Probability,
Vol. 12,
Issue. 4,
p.
753.
Cohn, Harry
1976.
Finite non-homogeneous Markov chains: Asymptotic behaviour.
Advances in Applied Probability,
Vol. 8,
Issue. 3,
p.
502.
Cohn, Harry
1976.
Finite non-homogeneous Markov chains: Asymptotic behaviour.
Advances in Applied Probability,
Vol. 8,
Issue. 3,
p.
502.
Kingman, J. F. C.
1976.
Ecole d'Eté de Probabilités de Saint-Flour V-1975.
Vol. 539,
Issue. ,
p.
167.
Seneta, E.
1979.
Coefficients of ergodicity: structure and applications.
Advances in Applied Probability,
Vol. 11,
Issue. 2,
p.
270.
Seneta, E.
1979.
Coefficients of ergodicity: structure and applications.
Advances in Applied Probability,
Vol. 11,
Issue. 2,
p.
270.
Seneta, E.
1979.
Coefficients of ergodicity: structure and applications.
Advances in Applied Probability,
Vol. 11,
Issue. 3,
p.
576.
Frydman, Halina
and
Singer, Burton
1979.
Total positivity and the embedding problem for Markov chains.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 86,
Issue. 2,
p.
339.
Seneta, E.
1979.
Coefficients of ergodicity: structure and applications.
Advances in Applied Probability,
Vol. 11,
Issue. 3,
p.
576.
Cohn, Harry
1981.
On a paper by Doeblin on non-homogeneous Markov chains.
Advances in Applied Probability,
Vol. 13,
Issue. 2,
p.
388.
Winkler, Gerhard
1981.
The Number of Phases of Inhomogeneous Markov Fields with Finite State Spaces on N and Z and their Behaviour at Infinity.
Mathematische Nachrichten,
Vol. 104,
Issue. 1,
p.
101.
Cohn, Harry
1981.
On a paper by Doeblin on non-homogeneous Markov chains.
Advances in Applied Probability,
Vol. 13,
Issue. 2,
p.
388.
Cohn, Harry
1982.
On a class of non-homogeneous Markov chains.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 92,
Issue. 3,
p.
527.
Mukherjea, Arunava
1983.
Non-homogeneous markov chains: Tail idempotents, tail sigma-fields and basis.
Mathematische Zeitschrift,
Vol. 183,
Issue. 3,
p.
293.
Gibert, S.
and
Mukherjea, A.
1984.
Probability Measures on Groups VII.
Vol. 1064,
Issue. ,
p.
147.
Gray, R.
Dunham, M.
and
Gobbi, R.
1987.
Ergodicity of Markov channels.
IEEE Transactions on Information Theory,
Vol. 33,
Issue. 5,
p.
656.
Ipsen, Ilse C. F.
and
Selee, Teresa M.
2011.
Ergodicity Coefficients Defined by Vector Norms.
SIAM Journal on Matrix Analysis and Applications,
Vol. 32,
Issue. 1,
p.
153.
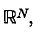 , is used to describe aspects of the chain. For example, an important theorem of Cohn on the structure of the tail σ-field is a simple corollary. The embedding problem is shown to be entirely geometrical in character. The representation extends to Markov processes on quite general state spaces, and the tail is then represented by the projective limit of these convex sets.
, is used to describe aspects of the chain. For example, an important theorem of Cohn on the structure of the tail σ-field is a simple corollary. The embedding problem is shown to be entirely geometrical in character. The representation extends to Markov processes on quite general state spaces, and the tail is then represented by the projective limit of these convex sets.

