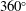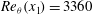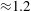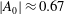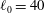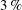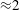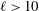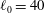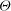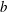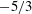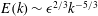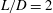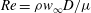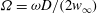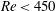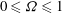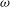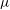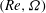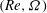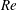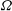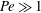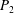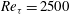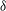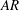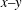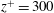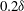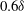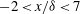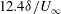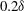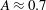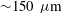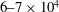In their recent publication Crouch et al. (J. Fluid Mech., this issue, vol. 748, 2014, pp. 5–35) use wind tunnel experiments to quantify the large-scale vortical structures that develop as a cyclist progresses through a full rotation of the pedals. The authors identify asymmetries in the trailing vortex wake, which intensify as one leg straightens, as the primary source of drag variation over one pedal cycle. These new data suggest that targeted approaches to mitigate asymmetries in the trailing wake present an intriguing opportunity to reduce drag in cycling strategies and technologies.

Three-dimensional flows around a full-scale cyclist mannequin were investigated experimentally to explain the large variations in aerodynamic drag that are measured as the legs are positioned around the $360^\circ $ crank cycle. It is found that the dominant mechanism affecting drag is not the small variation in frontal surface area over the pedal stroke but rather due to large changes in the flow structure over the crank cycle. This is clearly shown by a series of detailed velocity field wake surveys and skin friction flow visualizations. Two characteristic flow regimes are identified, corresponding to symmetrical low-drag and asymmetrical high-drag regimes, in which the primary feature of the wake is shown to be a large trailing streamwise vortex pair, orientated asymmetrically in the centre plane of the mannequin. These primary flow structures in the wake are the dominant mechanism driving the variation in drag throughout the pedal stroke. Topological critical points have been identified on the suction surfaces of the mannequin’s back and are discussed with velocity field measurements to elucidate the time-average flow topologies, showing the primary flow structures of the low- and high-drag flow regimes. The proposed flow topologies are then related to the measured surface pressures acting on the suction surface of the mannequin’s back. These measurements show that most of the variation in drag is due to changes in the pressure distribution acting on the lower back, where the large-scale flow structures having the greatest impact on drag develop.

This study focuses on the effects of mean (favourable) and large-scale fluctuating pressure gradients on boundary layer turbulence. Two-dimensional (2D) particle image velocimetry (PIV) measurements, some of which are time-resolved, have been performed upstream of and within a sink flow for two inlet Reynolds numbers, ${Re}_{\theta }(x_{1})=3360$ and 5285. The corresponding acceleration parameters, $K$, are ${1.3\times 10^{-6}}$ and ${0.6\times 10^{-6}}$. The time-resolved data at ${Re}_{\theta }(x_{1})=3360$ enables us to calculate the instantaneous pressure distributions by integrating the planar projection of the fluid material acceleration. As expected, all the locally normalized Reynolds stresses in the favourable pressure gradient (FPG) boundary layer are lower than those in the zero pressure gradient (ZPG) domain. However, the un-scaled stresses in the FPG region increase close to the wall and decay in the outer layer, indicating slow diffusion of near-wall turbulence into the outer region. Indeed, newly generated vortical structures remain confined to the near-wall region. An approximate analysis shows that this trend is caused by higher values of the streamwise and wall-normal gradients of mean streamwise velocity, combined with a slightly weaker strength of vortices in the FPG region. In both boundary layers, adverse pressure gradient fluctuations are mostly associated with sweeps, as the fluid approaching the wall decelerates. Conversely, FPG fluctuations are more likely to accompany ejections. In the ZPG boundary layer, loss of momentum near the wall during periods of strong large-scale adverse pressure gradient fluctuations and sweeps causes a phenomenon resembling local 3D flow separation. It is followed by a growing region of ejection. The flow deceleration before separation causes elevated near-wall small-scale turbulence, while high wall-normal momentum transfer occurs in the ejection region underneath the sweeps. In the FPG boundary layer, the instantaneous near-wall large-scale pressure gradient rarely becomes positive, as the pressure gradient fluctuations are weaker than the mean FPG. As a result, the separation-like phenomenon is markedly less pronounced and the sweeps do not show elevated small-scale turbulence and momentum transfer underneath them. In both boundary layers, periods of acceleration accompanying large-scale ejections involve near-wall spanwise contraction, and a high wall-normal momentum flux at all elevations. In the ZPG boundary layer, although some of the ejections are preceded, and presumably initiated, by regions of adverse pressure gradients and sweeps upstream, others are not. Conversely, in the FPG boundary layer, there is no evidence of sweeps or adverse pressure gradients immediately upstream of ejections. Apparently, the mechanisms initiating these ejections are either different from those involving large-scale sweeps or occur far upstream of the peak in FPG fluctuations.

We present large-eddy simulations (LES) of turbulent mixing at a perturbed, spherical interface separating two fluids of differing densities and subsequently impacted by a spherically imploding shock wave. This paper focuses on the differences between two fundamental configurations, keeping fixed the initial shock Mach number ${\approx }1.2$, the density ratio (precisely $|A_0|\approx 0.67$) and the perturbation shape (dominant spherical wavenumber $\ell _0=40$ and amplitude-to-initial radius of $3\, \%$): the incident shock travels from the lighter fluid to the heavy fluid or, inversely, from the heavy to the light fluid. After describing the computational problem we present results on the radially symmetric flow, the mean flow, and the growth of the mixing layer. Turbulent statistics are developed in Part 2 (Lombardini, M., Pullin, D. I. & Meiron, D. I. J. Fluid Mech., vol. 748, 2014, pp. 113–142). A wave-diagram analysis of the radially symmetric flow highlights that the light–heavy mixing layer is processed by consecutive reshocks, and not by reverberating rarefaction waves as is usually observed in planar geometry. Less surprisingly, reshocks process the heavy–light mixing layer as in the planar case. In both configurations, the incident imploding shock and the reshocks induce Richtmyer–Meshkov (RM) instabilities at the density layer. However, we observe differences in the mixing-layer growth because the RM instability occurrences, Rayleigh–Taylor (RT) unstable scenarios (due to the radially accelerated motion of the layer) and phase inversion events are different. A small-amplitude stability analysis along the lines of Bell (Los Alamos Scientific Laboratory Report, LA-1321, 1951) and Plesset (J. Appl. Phys., vol. 25, 1954, pp. 96–98) helps quantify the effects of the mean flow on the mixing-layer growth by decoupling the effects of RT/RM instabilities from Bell–Plesset effects associated with geometric convergence and compressibility for arbitrary convergence ratios. The analysis indicates that baroclinic instabilities are the dominant effect, considering the low convergence ratio (${\approx } 2$) and rather high ($\ell >10$) mode numbers considered.

We present large-eddy simulations (LES) of turbulent mixing at a perturbed, spherical interface separating two fluids of differing densities and subsequently impacted by a spherically imploding shock wave. This paper focuses on the differences between two fundamental configurations, keeping fixed the initial shock Mach number $\approx $1.2, the density ratio (precisely $|A_0|\approx 0.67$) and the perturbation shape (dominant spherical wavenumber 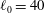$\ell _0=40$ and amplitude-to-initial radius of 3 %): the incident shock travels from the lighter fluid to the heavy one, or inversely, from the heavy to the light fluid. In Part 1 (Lombardini, M., Pullin, D. I. & Meiron, D. I., J. Fluid Mech., vol. 748, 2014, pp. 85–112), we described the computational problem and presented results on the radially symmetric flow, the mean flow, and the growth of the mixing layer. In particular, it was shown that both configurations reach similar convergence ratios $\approx $2. Here, turbulent mixing is studied through various turbulence statistics. The mixing activity is first measured through two mixing parameters, the mixing fraction parameter 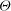$\varTheta $ and the effective Atwood ratio $A_e$, which reach similar late time values in both light–heavy and heavy–light configurations. The Taylor-scale Reynolds numbers attained at late times are estimated at approximately 2000 in the light–heavy case and 1000 in the heavy–light case. An analysis of the density self-correlation 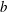$b$, a fundamental quantity in the study of variable-density turbulence, shows asymmetries in the mixing layer and non-Boussinesq effects generally observed in high-Reynolds-number Rayleigh–Taylor (RT) turbulence. These traits are more pronounced in the light–heavy mixing layer, as a result of its flow history, in particular because of RT-unstable phases (see Part 1). Another measure distinguishing light–heavy from heavy–light mixing is the velocity-to-scalar Taylor microscales ratio. In particular, at late times, larger values of this ratio are reported in the heavy–light case. The late-time mixing displays the traits some of the traits of the decaying turbulence observed in planar Richtmyer–Meshkov (RM) flows. Only partial isotropization of the flow (in the sense of turbulent kinetic energy (TKE) and dissipation) is observed at late times, the Reynolds normal stresses (and, thus, the directional Taylor microscales) being anisotropic while the directional Kolmogorov microscales approach isotropy. A spectral analysis is developed for the general study of statistically isotropic turbulent fields on a spherical surface, and applied to the present flow. The resulting angular power spectra show the development of an inertial subrange approaching a Kolmogorov-like 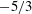$-5/3$ power law at high wavenumbers, similarly to the scaling obtained in planar geometry. It confirms the findings of Thomas & Kares (Phys. Rev. Lett., vol. 109, 2012, 075004) at higher convergence ratios and indicates that the turbulent scales do not seem to feel the effect of the spherical mixing-layer curvature.

We investigate the bulldozing motion of a granular sandpile driven forwards by a vertical plate. The problem is set up in the laboratory by emplacing the pile on a table rotating underneath a stationary plate; the continual circulation of the bulldozed material allows the dynamics to be explored over relatively long times, and the variation of the velocity with radius permits one to explore the dependence on bulldozing speed within a single experiment. We measure the time-dependent surface shape of the dune for a range of rotation rates, initial volumes and radial positions, for four granular materials, ranging from glass spheres to irregularly shaped sand. The evolution of the dune can be separated into two phases: a rapid initial adjustment to a state of quasi-steady avalanching perpendicular to the blade, followed by a much slower phase of lateral spreading and radial migration. The quasi-steady avalanching sets up a well-defined perpendicular profile with a nearly constant slope. This profile can be scaled by the depth against the bulldozer to collapse data from different times, radial positions and experiments onto common ‘master curves’ that are characteristic of the granular material and depend on the local Froude number. The lateral profile of the dune along the face of the bulldozer varies more gradually with radial position, and evolves by slow lateral spreading. The spreading is asymmetrical, with the inward progress of the dune eventually arrested and its bulk migrating to larger radii. A one-dimensional depth-averaged model recovers the nearly linear perpendicular profile of the dune, but does not capture the finer nonlinear details of the master curves. A two-dimensional version of the model leads to an advection–diffusion equation that reproduces the lateral spreading and radial migration. Simulations using the discrete element method reproduce in more quantitative detail many of the experimental findings and furnish further insight into the flow dynamics.

Spectral analysis of the Navier–Stokes equations requires treatment of the convolution of pairs of Fourier transforms $\hat{f}$ and $\hat{g}$. An exact, tractable representation of the nonlinear terms in spectral space is introduced, and relies on the definition and manipulation of a combination matrix. A spectral energy equation is derived where the nonlinear triad interactions are expressed using the combination matrix. The formulation is applied to the problem of homogeneous, isotropic turbulence. By finding the solution in an appropriate canonical basis, the energy spectrum in the inertial range $E(k)\sim \epsilon ^{2/3}k^{-5/3}$ is derived from the Navier–Stokes equations without invoking dimensional scaling arguments.

Three-dimensional instabilities arising in open cavity flows are responsible for complex broad-banded dynamics. Existing studies either focus on theoretical properties of ideal simplified flows or observe the final state of experimental flows. This paper aims to establish a connection between the onset of the centrifugal instabilities and their final expression within the fully saturated flow. To that end, a linear three-dimensional modal instability analysis of steady two-dimensional states developing in an open cavity of aspect ratio $L/D=2$ (length over depth) is conducted. This analysis is performed together with an experimental study in the same geometry adding spanwise endwalls. Two different Reynolds numbers are investigated through spectral analyses and modal decomposition. The physics of the flow is thoroughly described exploiting the strengths of each methodology. The main flow structures are identified and salient space and time scales are characterised. Results indicate that the structures obtained from linear analysis are mainly consistent with the fully saturated experimental flow. The analysis also brings to light the selection and alteration of certain wave properties, which could be caused by nonlinearities or the change of spanwise boundary conditions.

We consider the change in fluid density in a depth-integrated long-wave model. By allowing horizontal and vertical variation of fluid density, a depth-integrated model for long gravity waves over a variable-density fluid has been developed, where density change effects are included as correction terms. In particular, a two-layer fluid system is chosen to represent vertical density variations, where interfacial wave effects on the free surface are accounted for through direct inclusion of the velocity component of the interfacial wave. For the numerical implementation of the model, a finite-volume scheme coupled with an approximate Riemann solver is adopted for leading-order terms while cell-centred finite-volume methods are utilized for others. Numerical tests in which the density field is configured to vary either horizontally or vertically have been performed to verify the model. For horizontal variation of fluid density, a pneumatic breakwater system is simulated and fair agreement is observed between computed and measured data, indicating that the current induced by the upward bubble flux is responsible for wave attenuation to some degree. To investigate the effects of internal motion on the free surface, a two-layer fluid system with monochromatic internal wave motion is tested numerically. Simulated results agree well with the measured and analytical data. Lastly, nonlinear interactions between external- and internal-mode surface waves are studied numerically and analytically, and the model is shown to have nonlinear accuracy limitations similar to existing Boussinesq-type models.

We consider the nonlinear optimisation of the mixing of a passive scalar, initially arranged in two layers, in a two-dimensional plane Poiseuille flow at finite Reynolds and Péclet numbers, below the linear instability threshold. We use a nonlinear-adjoint-looping approach to identify optimal perturbations leading to maximum time-averaged energy as well as maximum mixing in a freely evolving flow, measured through the minimisation of either the passive scalar variance or the so-called mix-norm, as defined by Mathew, Mezić & Petzold (Physica D, vol. 211, 2005, pp. 23–46). We show that energy optimisation appears to lead to very weak mixing of the scalar field whereas the optimal mixing initial perturbations, despite being less energetic, are able to homogenise the scalar field very effectively. For sufficiently long time horizons, minimising the mix-norm identifies optimal initial perturbations which are very similar to those which minimise scalar variance, demonstrating that minimisation of the mix-norm is an excellent proxy for effective mixing in this finite-Péclet-number bounded flow. By analysing the time evolution from initial perturbations of several optimal mixing solutions, we demonstrate that our optimisation method can identify the dominant underlying mixing mechanism, which appears to be classical Taylor dispersion, i.e. shear-augmented diffusion. The optimal mixing proceeds in three stages. First, the optimal mixing perturbation, energised through transient amplitude growth, transports the scalar field across the channel width. In a second stage, the mean flow shear acts to disperse the scalar distribution leading to enhanced diffusion. In a final third stage, linear relaxation diffusion is observed. We also demonstrate the usefulness of the developed variational framework in a more realistic control case: mixing optimisation by prescribed streamwise velocity boundary conditions.

Direct numerical simulations (DNS) of controlled H- and K-type transitions to turbulence in an $M = 0.2$ (where $M$ is the Mach number) nominally zero-pressure-gradient and spatially developing flat-plate boundary layer are considered. Sayadi, Hamman & Moin (J. Fluid Mech., vol. 724, 2013, pp. 480–509) showed that with the start of the transition process, the skin-friction profiles of these controlled transitions diverge abruptly from the laminar value and overshoot the turbulent estimation. The objective of this work is to identify the structures of dynamical importance throughout the transitional region. Dynamic mode decomposition (DMD) (Schmid, J. Fluid Mech., vol. 656, 2010, pp. 5–28) as an optimal phase-averaging process, together with triple decomposition (Reynolds & Hussain, J. Fluid Mech., vol. 54 (02), 1972, pp. 263–288), is employed to assess the contribution of each coherent structure to the total Reynolds shear stress. This analysis shows that low-frequency modes, corresponding to the legs of hairpin vortices, contribute most to the total Reynolds shear stress. The use of composite DMD of the vortical structures together with the skin-friction coefficient allows the assessment of the coupling between near-wall structures captured by the low-frequency modes and their contribution to the total skin-friction coefficient. We are able to show that the low-frequency modes provide an accurate estimate of the skin-friction coefficient through the transition process. This is of interest since large-eddy simulation (LES) of the same configuration fails to provide a good prediction of the rise to this overshoot. The reduced-order representation of the flow is used to compare the LES and the DNS results within this region. Application of this methodology to the LES of the H-type transition illustrates the effect of the grid resolution and the subgrid-scale model on the estimated shear stress of these low-frequency modes. The analysis shows that although the shapes and frequencies of the low-frequency modes are independent of the resolution, the amplitudes are underpredicted in the LES, resulting in underprediction of the Reynolds shear stress.

We analyze the global linear stability of the axisymmetric flow around a spinning bullet-shaped body of length-to-diameter ratio $L/D=2$, as a function of the Reynolds number, $Re=\rho w_{\infty } D /\mu $, and of the rotation parameter $\varOmega =\omega D/(2 w_{\infty })$, in the ranges $Re<450$ and $0\leq \varOmega \leq 1$. Here, $w_{\infty }$ and $\omega $ are the free-stream and the body rotation velocities respectively, and $\rho $ and $\mu $ are the fluid density and viscosity. The two-dimensional eigenvalue problem (EVP) is solved numerically to find the spectrum of complex eigenvalues and their associated eigenfunctions, allowing us to explain the different bifurcations from the axisymmetric state observed in previous numerical studies. Our results reveal that, for the parameter ranges investigated herein, three global eigenmodes, denoted low-frequency (LF), medium-frequency (MF) and high-frequency (HF) modes, become unstable in different regions of the $(Re,\varOmega )$-parameter plane. We provide precise computations of the corresponding neutral curves, that divide the $(Re,\varOmega )$-plane into four different regions: the stable axisymmetric flow prevails for small enough values of $Re$ and $\varOmega $, while three different frozen states, where the wake structures co-rotate with the body at different angular velocities, take place as a consequence of the destabilization of the LF, MF and HF modes. Several direct numerical simulations (DNS) of the nonlinear state associated with the MF mode, identified here for the first time, are also reported to complement the linear stability results. Finally, we point out the important fact that, since the axisymmetric base flow is $SO(2)$-symmetric, the theory of equivariant bifurcations implies that the weakly nonlinear regimes that emerge close to criticality must necessarily take the form of rotating-wave states. These states, previously referred to as frozen wakes in the literature, are thus shown to result from the base-flow symmetry.

The problem of Brownian flocculation of spherical particles in strong shearing flow without hydrodynamic interactions is studied in detail using the singular perturbation method. All other types of interparticle interactions, such as van der Waals or Lennard-Jones forces, are also ignored. In the limit of strong external flow, the strength of which is measured by the Péclet number ($Pe\gg 1$), a complicated boundary layer structure for the pair probability density function ($P_{2}$) is identified and the complete stationary spatial distribution of $P_{2}(\boldsymbol {x})$ in the domain is found. The results, in particular the total mass flux in the accumulation process, are compared qualitatively and quantitatively with the case where the spheres interact hydrodynamically and it is demonstrated that the hydrodynamic interactions tend to decrease the rate of flocculation. An explicit simple formula for the flocculation rate for a general form of hydrodynamic interactions is provided. The limit of small Péclet number is also discussed to confirm the conclusion on the detrimental influence of hydrodynamic interactions on the rate of Brownian flocculation in shearing flow.

Two-dimensional oscillatory lid-driven cavity flow of a rarefied gas at arbitrary oscillation frequency is investigated using the linearized Boltzmann equation. An analytical solution at high oscillation frequencies is obtained, and detailed numerical results for a wide range of gas rarefaction are presented. The influence of both the aspect ratio of the cavity and the oscillating frequency on the damping force exerted on the moving lid is studied. Surprisingly, it is found that, over a certain frequency range, the damping is smaller than that in an oscillatory Couette flow. This reduction in damping is due to the anti-resonance of the rarefied gas. A scaling law between the anti-resonant frequency and the aspect ratio is established, which would enable the control of the damping through choosing an appropriate cavity geometry.

A zero pressure gradient turbulent boundary layer of $\textit {Re}_{\tau }=2500$ was perturbed by a single spanwise array of finite cylinders mounted on the bounding surface and extending through the logarithmic region. The cylinder height was $H/\delta =0.2$ ($H^{+}=500$), where $\delta $ is the boundary layer thickness, with an aspect ratio ($AR$) (height/diameter) of four. Streamwise–spanwise ($x\text {--}y$) planes of the flow were examined by particle image velocimetry (PIV) up to $7\delta $ downstream at a wall-normal location of $z^{+}=300$ for cylinder array spacings ranging from $0.2\delta $ to $0.8\delta $. Average streamwise velocity fields showed a splitting, then merging pattern of cylinder wakes which occurred further downstream as the cylinder spacing increased. Based on measurements at the furthest downstream location, both the spanwise variation of average streamwise velocity and the Fourier content in the instantaneous fields suggested that the case with $0.6\delta $ cylinder spacing, which matched the dominant spanwise scale in the unperturbed flow, yielded the most persistent downstream flow organization. A flying PIV method was implemented to track specific packet structures over a range $-2<x/\delta <7$ with respect to the cylinder array, corresponding to a time scale of $12.4\delta /U_{\infty }$. Packets approaching the $0.2\delta $ spacing array first lost their organization but then regained it a distance $2\delta $ downstream, suggesting that a persistent outer layer organization propagated inwards into the log region. For arrays with larger spanwise spacing, approaching packets were generally redirected into the spanwise location midway between cylinders and sometimes enhanced.

Wavepackets obtained by a linear stability analysis of the turbulent mean flow were shown in recent works to agree closely with some relevant statistics of turbulent jets, such as power spectral densities and averaged phases of flow fluctuations. However, when such wavepacket models were used to calculate the far-field sound, satisfactory agreement was only obtained for flows that were supersonic relative to the ambient speed of sound; attempts with subsonic flows led to errors of more than an order of magnitude. We investigate here the reasons for such discrepancies by developing the integral solution of the Helmholtz equation in terms of the cross-spectral densities of turbulent quantities. It is shown that agreement of a statistical source, such as would be obtained by the above-mentioned wavepacket models, in averaged amplitudes and phases in the near field is not a sufficient condition for exact agreement of the far-field sound. The sufficient condition is that, in addition to the amplitudes and phases, the statistical source should also match the coherence function of the flow fluctuations. This is exemplified in a model problem, where we show that the effect of coherence decay on sound radiation is more prominent for subsonic convection velocities, and its neglect leads to discrepancies of more than an order of magnitude in the far-field sound. For supersonic flows errors are reduced for the peak noise direction, but for other angles the coherence decay is also seen to have a significant effect. Coherence decay in the model source is seen to lead to similar decays in the coherence of two points in the far acoustic field, these decays being significantly faster for higher Mach numbers. The limitations of linear wavepacket models are illustrated with another simplified problem, showing that superposition of time-periodic solutions can lead to a correlation decay between two points. However, the coherence between any pair of points in such models remains unity, and cannot thus represent the behaviour observed in turbulent flows.

We present an exact analytical solution of the nonlinear shallow water theory for wave run-up in inclined channels of arbitrary cross-section, which generalizes previous studies on wave run-up for a plane beach and channels of parabolic cross-section. The solution is found using a hodograph-type transform, which extends the well-known Carrier–Greenspan transform for wave run-up on a plane beach. As a result, the nonlinear shallow water equations are reduced to a single one-dimensional linear wave equation for an auxiliary function and all physical variables can be expressed in terms of this function by purely algebraic formulas. In the special case of a U-shaped channel this equation coincides with a spherically symmetric wave equation in space, whose dimension is defined by the channel cross-section and can be fractional. As an example, the run-up of a sinusoidal wave on a beach is considered for channels of several different cross-sections and the influence of the cross-section on wave run-up characteristics is studied.

The wavelet-based eddy capturing approach is extended to three-dimensional bluff body flows, where the flow geometry is enforced through Brinkman volume penalization. The wavelet-collocation/volume-penalization combined method is applied to the simulation of vortex shedding flow behind an isolated stationary prism with square cross-section. Wavelet-based direct numerical simulation is conducted at low supercritical Reynolds number, where the wake develops fundamental three-dimensional flow structures, while wavelet-based adaptive large-eddy simulation supplied with the one-equation localized dynamic kinetic-energy-based model is performed at moderately high Reynolds number. The present results are in general agreement with experimental findings and numerical solutions provided by classical non-adaptive methods. This study demonstrates that the proposed hybrid methodology for modelling bluff body flows is feasible, accurate and efficient.

The Richtmyer–Meshkov instability (RMI) is experimentally investigated in a vertical shock tube using a broadband initial condition imposed on an interface between a helium–acetone mixture and argon ($A\approx 0.7$). The interface is created without the use of a membrane by first setting up a flat, gravitationally stable stagnation plane, where the gases are injected from the ends of the shock tube and exit through horizontal slots at the interface location. Following this, the interface is perturbed by injecting gas within the plane of the interface. Perturbations form in the lower portion of this layer due to the shear between this injected stream and the surrounding gas. This shear layer serves as a statistically repeatable broadband initial condition to the RMI. The interface is accelerated by either a $M= 1.6 $ or $M= 2.2 $ planar shock wave, and the development of the ensuing mixing layer is investigated using planar laser-induced fluorescence (PLIF). The PLIF images are processed to reveal the light-gas mole fraction by accounting for laser absorption and laser-steering effects. The images suggest a transition to turbulent mixing occurring during the experiment. An analysis of the mole-fraction distribution confirms this transition, showing the gases begin to homogenize at later times. The scalar variance energy spectra exhibits a near $k^{-5/3}$ inertial range, providing further evidence for turbulent mixing. Measurements of the Batchelor and Taylor microscales are made from the mole-fraction images, giving ${\sim }150\ \mu \mathrm{m}$ and 4 mm, respectively, by the latest times. The ratio of these scales implies an outer-scale Reynolds number of $6\text {--}7\times 10^4$.

The behaviour of a non-spherical osmotic motor – an axisymmetric catalytic particle self-propelling in a dilute dispersion of reactant particles – is considered. In contrast to a conventional osmotic motor that creates differences in concentration, and hence in osmotic pressure, due to asymmetry in reaction rate along its surface (e.g. a Janus particle with reactive and non-reactive patches), a non-spherical particle is able to move even with uniform chemical activity on its surface. For small departures from a sphere the velocity of self-propulsion is proportional to the square of the non-sphericity or distortion of the particle shape. It is shown that the inclusion of hydrodynamic interactions (HI) may drastically change the self-propulsion. Except for very slow chemical reactions, even the direction of self-propulsion changes with and without HI. Numerical calculations at finite non-sphericity suggest that the maximum velocity of self-propulsion is obtained by a sail-like motor shape, leading to the name ‘chemical sailing’. Moreover, no saturation in the speed of propulsion is found; the motor velocity increases as the area of this ‘sail’ grows and its thickness decreases. The self-propulsion of a non-spherical particle releasing products of a chemical reaction – a constant flux motor – is also considered.