Hostname: page-component-78c5997874-ndw9j Total loading time: 0 Render date: 2024-11-04T22:00:55.565Z Has data issue: false hasContentIssue false
  • English
  • Français

An experimental investigation of the turbulent mixing transition in the Richtmyer–Meshkov instability

Published online by Cambridge University Press:  01 May 2014

Christopher R. Weber ,
Nicholas S. Haehn ,
Jason G. Oakley ,
David A. Rothamer  and
Riccardo Bonazza
Christopher R. Weber*
Affiliation:
University of Wisconsin, Madison, WI 53706, USA Lawrence Livermore National Laboratory, Livermore, CA 94550, USA
Nicholas S. Haehn*
Affiliation:
University of Wisconsin, Madison, WI 53706, USA
Jason G. Oakley
Affiliation:
University of Wisconsin, Madison, WI 53706, USA
David A. Rothamer
Affiliation:
University of Wisconsin, Madison, WI 53706, USA
Riccardo Bonazza
Affiliation:
University of Wisconsin, Madison, WI 53706, USA
*
†Email address for correspondence: [email protected]
‡Present address: Intel Corporation, Chandler, AZ 85226, USA.
Get access Rights & Permissions [Opens in a new window]

Abstract

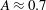The Richtmyer–Meshkov instability (RMI) is experimentally investigated in a vertical shock tube using a broadband initial condition imposed on an interface between a helium–acetone mixture and argon ($A\approx 0.7$). The interface is created without the use of a membrane by first setting up a flat, gravitationally stable stagnation plane, where the gases are injected from the ends of the shock tube and exit through horizontal slots at the interface location. Following this, the interface is perturbed by injecting gas within the plane of the interface. Perturbations form in the lower portion of this layer due to the shear between this injected stream and the surrounding gas. This shear layer serves as a statistically repeatable broadband initial condition to the RMI. The interface is accelerated by either a $M= 1.6 $ or $M= 2.2 $ planar shock wave, and the development of the ensuing mixing layer is investigated using planar laser-induced fluorescence (PLIF). The PLIF images are processed to reveal the light-gas mole fraction by accounting for laser absorption and laser-steering effects. The images suggest a transition to turbulent mixing occurring during the experiment. An analysis of the mole-fraction distribution confirms this transition, showing the gases begin to homogenize at later times. The scalar variance energy spectra exhibits a near $k^{-5/3}$ inertial range, providing further evidence for turbulent mixing. Measurements of the Batchelor and Taylor microscales are made from the mole-fraction images, giving 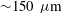${\sim }150\ \mu \mathrm{m}$ and 4 mm, respectively, by the latest times. The ratio of these scales implies an outer-scale Reynolds number of 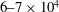$6\text {--}7\times 10^4$.

JFM classification

Instability: Instability Mixing: Turbulent mixing Turbulent Flows: Turbulent transition
Type
Papers
Information
Journal of Fluid Mechanics , Volume 748 , 10 June 2014 , pp. 457 - 487
Copyright
© 2014 Cambridge University Press 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Balakumar, B. J., Orlicz, G. C., Ristorcelli, J. R., Balasubramanian, S., Prestridge, K. P. & Tomkins, C. D. 2012 Turbulent mixing in a Richtmyer–Meshkov fluid layer after reshock: velocity and density statistics. J. Fluid Mech. 696 (410), 67–93.Google Scholar
Balasubramanian, S., Orlicz, G. C. & Prestridge, K. P. 2013 Experimental study of initial condition dependence on turbulent mixing in shock-accelerated Richtmyer–Meshkov fluid layers. J. Turbul. 14 (3), 170–196.Google Scholar
Banerjee, A., Kraft, W. N. & Andrews, M. J. 2010 Detailed measurements of a statistically steady Rayleigh–Taylor mixing layer from small to high Atwood numbers. J. Fluid Mech. 659, 127–190.CrossRefGoogle Scholar
Besnard, D., Harlow, F. H., Rauenzahn, R. M. & Zemach, C.1992 Turbulent transport equations for variable-density turbulence and their relationship to two-field models. Tech. Rep. LA-12303-MA. Los Alamos National Laboratory.Google Scholar
Buch, K. A. & Dahm, W. J. A. 1996 Experimental study of the fine-scale structure of conserved scalar mixing in turbulent shear flows. Part 1. $Sc\gg 1$ . J. Fluid Mech. 317, 21–71.Google Scholar
Buch, K. A. & Dahm, W. J. A. 1998 Experimental study of the fine-scale structure of conserved scalar mixing in turbulent shear flows. Part 2. $\mathit{Sc}\sim 1$ . J. Fluid Mech. 364, 1–29.Google Scholar
Cabot, W. H. & Cook, A. W. 2006 Reynolds number effects on Rayleigh–Taylor instability with possible implications for type Ia supernovae. Nat. Phys. 2 (8), 562–568.Google Scholar
Canny, J. 1986 A computational approach to edge detection. IEEE Trans. Pattern Anal. Mach. Intell. 6, 679–698.Google Scholar
Champagne, F. H., Harris, V. G. & Corrsin, S. 1970 Experiments on nearly homogeneous turbulent shear flow. J. Fluid Mech. 41 (01), 81–139.Google Scholar
Chisnell, R. F. 1955 The normal motion of a shock wave through a non-uniform one-dimensional medium. Proc. R. Soc. Lond. A 232 (1190), 350–370.Google Scholar
Clemens, N. T. 2002 Flow imaging. In Encyclopedia of Imaging Science and Technology (ed. Hornak, J. P.), pp. 390–420. John Wiley and Sons.Google Scholar
Collins, B. D. & Jacobs, J. W. 2002 PLIF flow visualization and measurements of the Richtmyer–Meshkov instability of an air/SF6 interface. J. Fluid Mech. 464, 113–136.Google Scholar
Cook, A. W., Cabot, W. & Miller, P. L. 2004 The mixing transition in Rayleigh–Taylor instability. J. Fluid Mech. 511, 333–362.Google Scholar
Cook, A. W. & Dimotakis, P. E. 2001 Transition stages of Rayleigh–Taylor instability between miscible fluids. J. Fluid Mech. 443, 69–99.Google Scholar
Dimonte, G. & Schneider, M. 1997 Turbulent Richtmyer–Meshkov instability experiments with strong radiatively driven shocks. Phys. Plasmas 4 (12), 4347–4357.Google Scholar
Dimonte, G. & Schneider, M. 2000 Density ratio dependence of Rayleigh–Taylor mixing for sustained and impulsive acceleration histories. Phys. Fluids 12, 304–321.CrossRefGoogle Scholar
Dimotakis, P. E. 2000 The mixing transition in turbulent flows. J. Fluid Mech. 409, 69–98.Google Scholar
Dimotakis, P. E., Catrakis, H. J. & Fourguette, D. C. 2001 Flow structure and optical beam propagation in high-Reynolds-number gas-phase shear layers and jets. J. Fluid Mech. 433, 105–134.Google Scholar
Ghandhi, J. B. 2006 Spatial resolution and noise considerations in determining scalar dissipation rate from passive scalar image data. Exp. Fluids 40, 577–588.Google Scholar
Glezer, A. 1988 The formation of vortex rings. Phys. Fluids 31, 3532–3542.Google Scholar
Grégoire, O., Souffland, D. & Gauthier, S. 2005 A second-order turbulence model for gaseous mixtures induced by Richtmyer–Meshkov instability. J. Turbul. 6, N29.Google Scholar
Jacobs, J. W., Krivets, V. V., Tsiklashvili, V. & Likhachev, O. A. 2013 Experiments on the Richtmyer–Meshkov instability with an imposed, random initial perturbation. Shock Waves 23 (4), 407–413.Google Scholar
Jacobs, J. W. & Sheeley, J. M. 1996 Experimental study of incompressible Richtmyer–Meshkov instability. Phys. Fluids 8 (2), 405–415.CrossRefGoogle Scholar
Jones, M. A. & Jacobs, J. W. 1997 A membraneless experiment for the study of Richtmyer–Meshkov instability of a shock-accelerated gas interface. Phys. Fluids 9 (10), 3078–3085.Google Scholar
Kaiser, S. A. & Frank, J. H. 2007 Imaging of dissipative structures in the near field of a turbulent non-premixed jet flame. Proc. Combust. Inst. 31 (1), 1515–1523.Google Scholar
Kaiser, S. A. & Frank, J. H. 2011 The effects of laser-sheet thickness on dissipation measurements in turbulent non-reacting jets and jet flames. Meas. Sci. Technol. 22 (4), 045403.Google Scholar
Kane, J., Drake, R. P. & Remington, B. A. 1999 An evaluation of the Richtmyer–Meshkov instability in supernova remnant formation. Astrophys. J. 511 (1), 335–340.Google Scholar
Leinov, E., Malamud, G., Elbaz, Y., Levin, L. A., Ben-Dor, G., Shvarts, D. & Sadot, O. 2009 Experimental and numerical investigation of the Richtmyer–Meshkov instability under re-shock conditions. J. Fluid Mech. 626, 449–475.Google Scholar
Lindl, J. D., Amendt, P., Berger, R. L., Glendinning, S. G., Glenzer, S. H., Haan, S. W., Kauffman, R. L., Landen, O. L. & Suter, L. J. 2004 The physics basis for ignition using indirect-drive targets on the national ignition facility. Phys. Plasmas 11 (2), 339–491.Google Scholar
Livescu, D. & Ristorcelli, J. R. 2008 Variable-density mixing in buoyancy-driven turbulence. J. Fluid Mech. 605, 145–180.CrossRefGoogle Scholar
Livescu, D., Ristorcelli, J. R., Gore, R. A., Dean, S. H., Cabot, W. H. & Cook, A. W. 2009 High-Reynolds number Rayleigh–Taylor turbulence. J. Turbul. 10 (13), 1–32.Google Scholar
Lombardini, M., Pullin, D. I. & Meiron, D. I. 2012 Transition to turbulence in shock-driven mixing: a Mach number study. J. Fluid Mech. 690, 203–226.Google Scholar
Marble, F., Hendricks, G. & Zukoski, E.1987 Progress toward shock enhancement of supersonic combustion processes. In 23rd AIAA, SAE, ASME, and ASEE, Joint Propulsion Conference, San Diego, CA, June 29–July 2, American Institute of Aeronautics and Astronautics, New York, pp. 1–8.Google Scholar
Meshkov, E. E. 1970 Instability of a shock wave accelerated interface between two gases. NASA Tech. Transl. 13, 1–14.Google Scholar
Mikaelian, K. 1989 Turbulent mixing generated by Rayleigh–Taylor and Richtmyer–Meshkov instabilities. Physica D 36, 343–357.Google Scholar
Motl, B., Oakley, J., Ranjan, D., Weber, C., Anderson, M. & Bonazza, R. 2009 Experimental validation of a Richtmyer–Meshkov scaling law over large density ratio and shock strength ranges. Phys. Fluids 21 (12), 126102.Google Scholar
Orlicz, G. C., Balakumar, B. J., Tomkins, C. D. & Prestridge, K. P. 2009 A Mach number study of the Richtmyer–Meshkov instability in a varicose, heavy-gas curtain. Phys. Fluids 21 (6), 064102.Google Scholar
Orlicz, G. C., Balasubramanian, S. & Prestridge, K. P. 2013 Incident shock Mach number effects on Richtmyer–Meshkov mixing in a heavy gas layer. Phys. Fluids 25 (11), 114101.Google Scholar
Petersen, B. & Ghandhi, J. 2011 High-resolution turbulent scalar field measurements in an optically accessible internal combustion engine. Exp. Fluids 51, 1695–1708.Google Scholar
Pope, S. 2000 Turbulent Flows. Cambridge University Press.Google Scholar
Prasad, J. K., Rasheed, A., Kumar, S. & Sturtevant, B. 2000 The late-time development of the Richtmyer–Meshkov instability. Phys. Fluids 12 (8), 2108–2115.Google Scholar
Ramaprabhu, P. & Andrews, M. J. 2004 Experimental investigation of Rayleigh–Taylor mixing at small Atwood numbers. J. Fluid Mech. 502, 233–271.CrossRefGoogle Scholar
Ranjan, D., Oakley, J. & Bonazza, R. 2011 Shock-bubble interactions. Annu. Rev. Fluid Mech. 43, 117–140.Google Scholar
Rayleigh, L. 1883 Investigation of the character of the equilibrium of an incompressible heavy fluid of variable density. Proc. Lond. Math. Soc. 14 (1), 170–177.Google Scholar
Read, K. I. 1984 Experimental investigation of turbulent mixing by Rayleigh–Taylor instability. Physica D 12 (1–3), 45–58.CrossRefGoogle Scholar
Reid, R. C., Prausnitz, J. M. & Poling, B. E. 1987 The Properties of Gases and Liquids. McGraw-Hill.Google Scholar
Richtmyer, R. D. 1960 Taylor instability in shock acceleration of compressible fluids. Commun. Pure Appl. Maths 13 (2), 297–319.Google Scholar
Rightley, P. M., Vorobieff, P., Martin, R. & Benjamin, R. F. 1999 Experimental observations of the mixing transition in a shock-accelerated gas curtain. Phys. Fluids 11 (1), 186–200.Google Scholar
Ristorcelli, J. R. & Clark, T. T. 2004 Rayleigh–Taylor turbulence: self-similar analysis and direct numerical simulations. J. Fluid Mech. 507, 213–253.Google Scholar
Ristorcelli, J. R., Gowardhan, A. A. & Grinstein, F. F. 2013 Two classes of Richtmyer–Meshkov instabilities: a detailed statistical look. Phys. Fluids 25 (4), 044106.Google Scholar
Robey, H. F., Zhou, Y., Buckingham, A. C., Keiter, P., Remington, B. A. & Drake, R. P. 2003 The time scale for the transition to turbulence in a high Reynolds number, accelerated flow. Phys. Plasmas 10 (3), 614–622.Google Scholar
Saffman, P. G. & Meiron, D. I. 1989 Kinetic energy generated by the incompressible Richtmyer–Meshkov instability in a continuously stratified fluid. Phys. Fluids A 1 (11), 1767–1771.Google Scholar
Su, L. K. & Clemens, N. T. 2003 The structure of fine-scale scalar mixing in gas-phase planar turbulent jets. J. Fluid Mech. 488, 1–29.Google Scholar
Taylor, G. 1950 The instability of liquid surfaces when accelerated in a direction perpendicular to their planes. I. Proc. R. Soc. Lond. 201 (1065), 192–196.Google Scholar
Tennekes, H. & Lumley, J. L. 1972 A First Course in Turbulence. The MIT Press.Google Scholar
Tomkins, C. D., Balakumar, B. J., Orlicz, G., Prestridge, K. P. & Ristorcelli, J. R. 2013 Evolution of the density self-correlation in developing Richtmyer–Meshkov turbulence. J. Fluid Mech. 735, 288–306.Google Scholar
Tomkins, C., Kumar, S., Orlicz, G. & Prestridge, K. 2008 An experimental investigation of mixing mechanisms in shock-accelerated flow. J. Fluid Mech. 611, 131–150.Google Scholar
Vetter, M. & Sturtevant, B. 1995 Experiments on the Richtmyer–Meshkov instability of an air/SF $_6$ interface. Shock Waves 4, 247–252.Google Scholar
Vorobieff, P., Mohamed, N. G., Tomkins, C., Goodenough, C., Marr-Lyon, M. & Benjamin, R. F. 2003 Scaling evolution in shock-induced transition to turbulence. Phys. Rev. E 68 (6), 065301.Google Scholar
Vorobieff, P., Rightley, P. M. & Benjamin, R. F. 1998 Power-law spectra of incipient gas-curtain turbulence. Phys. Rev. Lett. 81, 2240–2243.Google Scholar
Wang, G. H. & Clemens, N. T. 2004 Effects of imaging system blur on measurements of flow scalars and scalar gradients. Exp. Fluids 37 (2), 194–205.Google Scholar
Wang, G. H., Clemens, N. T., Barlow, R. S. & Varghese, P. L. 2007 A system model for assessing scalar dissipation measurement accuracy in turbulent flows. Meas. Sci. Technol. 18, 1287–1303.Google Scholar
Weber, C. R.2012 Turbulent mixing measurements in the Richtmyer–Meshkov instability. PhD thesis, University of Wisconsin–Madison.Google Scholar
Weber, C. R., Cook, A. W. & Bonazza, R. 2013 Growth rate of a shocked mixing layer with known initial perturbations. J. Fluid Mech. 725, 372–401.Google Scholar
Weber, C. R., Haehn, N., Oakley, J., Rothamer, D. & Bonazza, R. 2012 Turbulent mixing measurements in the Richtmyer–Meshkov instability. Phys. Fluids 24 (7), 074105.CrossRefGoogle Scholar
Youngs, D. L. 1984 Numerical simulation of turbulent mixing by Rayleigh–Taylor instability. Physica D 12 (1–3), 32–44.Google Scholar
Zhou, Y., Robey, H. & Buckingham, A. 2003 Onset of turbulence in accelerated high-Reynolds-number flow. Phys. Rev. E 67 (5), 056305.Google Scholar