1. Introduction
Ocean waves, typically spanning tens to hundreds of metres in wavelengths, are powerful and pose a significant threat to marine structures. Therefore, marine structures should be designed to survive these waves (Goda Reference Goda2010). If a calm free surface can be created on an open sea, ocean space utilisation will be more convenient and expanded.
To reduce the wave action to structures, coastlines and harbours, floating breakwaters have been developed (see a review by Dai et al. Reference Dai, Wang, Utsunomiya and Duan2018). Most of the concepts are for inshore, but some are developed for open sea utilisation (e.g. Xiao et al. Reference Xiao, Kou, Tao and Yang2016; Cominelli et al. Reference Cominelli, Sinigaglia, Quadrelli and Braghin2023). Breakwaters manipulate waves by controlling various physical mechanics, such as phase interaction, reflection (or scattering), refraction, breaking and friction (Sawaragi Reference Sawaragi1995). Among them, we focus on the wave energy conservation process (i.e. breaking and friction are not scoped).
Over the past decade, transformation-based wave manipulation (Leonhardt Reference Leonhardt2006; Pendry et al. Reference Pendry, Schurig and Smith2006) has been studied in the water wave communities. In particular, the realisation of water wave cloaking has attracted much attention. Since the governing equation of shallow water waves is invariant under the coordinate transformation (Cummer & Schurig Reference Cummer and Schurig2007), the methodologies of shallow water cloaking are well established (Farhat et al. Reference Farhat, Enoch, Guenneau and Movchan2008; Zareei & Alam Reference Zareei and Alam2015; Dupont et al. Reference Dupont, Guenneau, Kimmoun, Molin and Enoch2016; Iida & Kashiwagi Reference Iida and Kashiwagi2018; Zhang et al. Reference Zhang, Iida, He, Mo, Liu, Luan and Jing2024). However, the transformation-based wave manipulation is not applicable to ocean waves because the governing equation of non-shallow water waves is not form-invariant (Porter Reference Porter2017). Alternately, the cloaking by bending wave rays from the surface into the internal surface using the nonlinear resonance of the bottom topography was proposed for the intermediate-depth and stratified sea waves (Alam Reference Alam2012). In the ocean engineering communities, however, the scattering cancellation-based cloaking has been studied (Porter & Newman Reference Porter and Newman2014; Newman Reference Newman2014; Iida et al. Reference Iida, Kashiwagi and He2014, Reference Iida, Kashiwagi and Miki2016, Reference Iida, Zareei and Alam2023). Sub-structures (such as columns or elastic plate) are arranged to surround an inner structure, and these are designed to cloak the inner structure from waves. The scattering cancellation-based cloaking realises almost zero outgoing scattering waves, and the existence of the inner structure cannot be detected even when waves are observed outside the cloak. As a result, the steady wave drift force does not act on the cloaked structure. Nevertheless, this cloaking does not create a calm free surface within the cloaked domain, and the cloaked structure still meets waves. Therefore, the wave exciting force is acting on the structure (Newman Reference Newman2014). In addition, the optimal design of the cloak changes when the shape of the inner structure changes.
In light of previous studies, the term ‘cloaking’ encompasses two different states. The first is preventing the detection of an object by suppressing wave scattering outside the cloak. The second is protecting the object from wave influence by shielding it from wave penetration within the cloak. How much the object scatters waves can be estimated by the scattered-wave energy (Newman Reference Newman2014; Iida et al. Reference Iida, Kashiwagi and He2014), and the first state is achieved by reducing the scattered-wave energy. Scattering cancellation-based cloaking satisfies this first state. However, the second state is more critical for protecting marine structures from ocean waves.
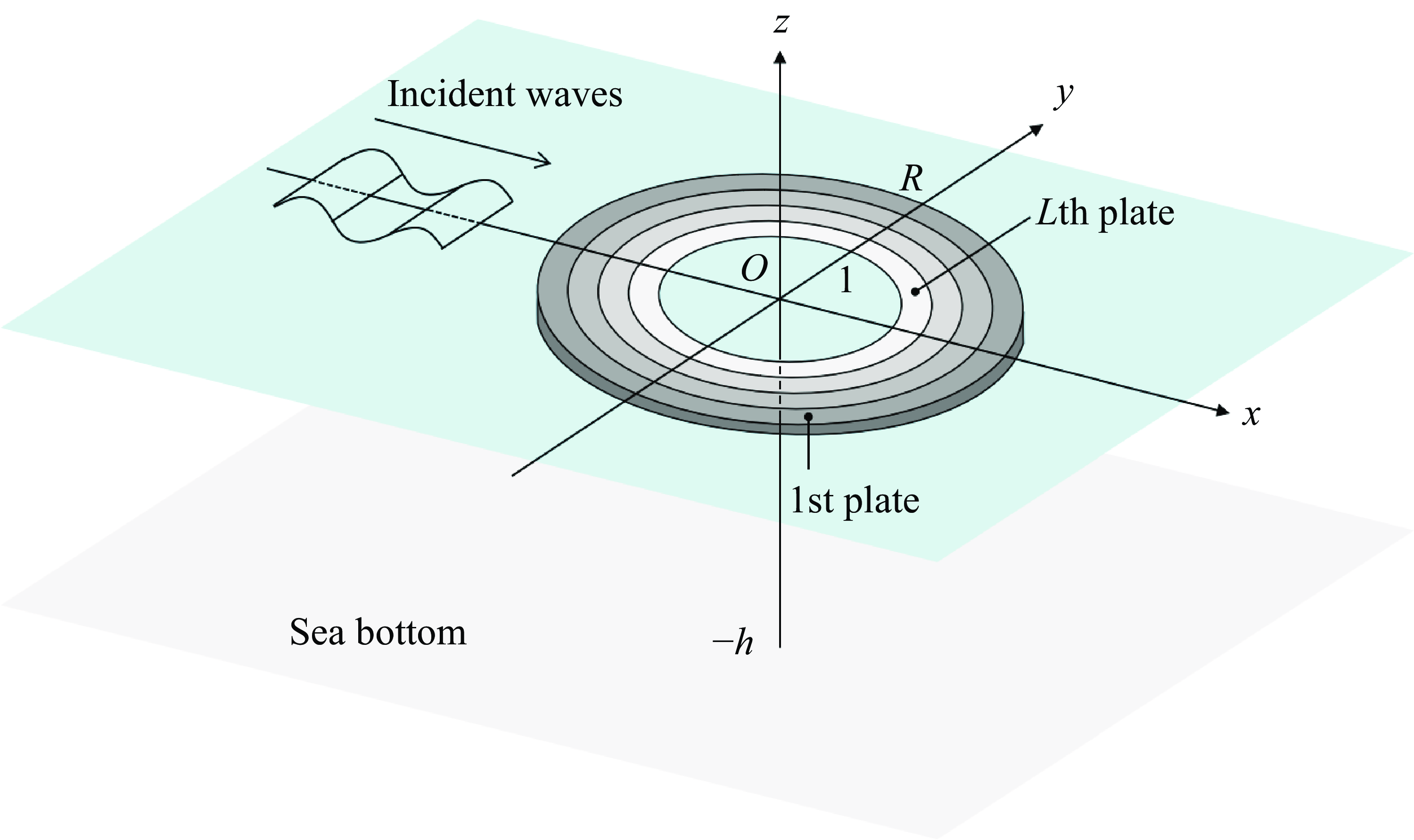
Figure 1. Schematic representation of floating annulus consisting of
![]() $L$
horizontal concentric annular plates. The annulus is designed to serve as a wave shied and a cloak.
$L$
horizontal concentric annular plates. The annulus is designed to serve as a wave shied and a cloak.
Here, we study the second state, protection from invading waves, to create a calm free surface within an inner domain of a floating elastic annulus. We newly derive a mean energy density of waves entering into the inner domain of the annulus (namely inlet wave energy density). The calmness of the free surface within the inner domain is estimated by a metric called the inlet wave energy factor, which is defined by the ratio of the inlet wave energy density to the mean density of the plane waves. The second state is achieved by reducing the inlet wave energy factor.
The concept of the floating elastic annulus is described in figure 1. The annulus consists of
![]() $L$
horizontal plates of which adjacent plates are rigidly connected. The elastic annulus is modelled as Kirchhoff’s thin plate (Meylan & Squire Reference Meylan and Squire1994; Peter et al. Reference Peter, Meylan and Chung2004). The response of multi-connected elastic plates is solved by the developed eigenvalue matching method (Iida et al. Reference Iida, Zareei and Alam2023) based on Peter et al. (Reference Peter, Meylan and Chung2004), where assumed thin-plate modelling was validated through the experiment (Meylan et al. Reference Meylan, Bennetts, Cavaliere, Alberello and Toffoli2015). It should be mentioned that the use of a floating elastic annulus to reduce waves has already been attempted (Loukogeorgaki & Kashiwagi Reference Loukogeorgaki and Kashiwagi2019; Malenica et al. Reference Malenica, Liang, Zheng and Korobkin2023). Nevertheless, performances were not dramatic because they assumed constant flexural rigidity throughout the annulus.
$L$
horizontal plates of which adjacent plates are rigidly connected. The elastic annulus is modelled as Kirchhoff’s thin plate (Meylan & Squire Reference Meylan and Squire1994; Peter et al. Reference Peter, Meylan and Chung2004). The response of multi-connected elastic plates is solved by the developed eigenvalue matching method (Iida et al. Reference Iida, Zareei and Alam2023) based on Peter et al. (Reference Peter, Meylan and Chung2004), where assumed thin-plate modelling was validated through the experiment (Meylan et al. Reference Meylan, Bennetts, Cavaliere, Alberello and Toffoli2015). It should be mentioned that the use of a floating elastic annulus to reduce waves has already been attempted (Loukogeorgaki & Kashiwagi Reference Loukogeorgaki and Kashiwagi2019; Malenica et al. Reference Malenica, Liang, Zheng and Korobkin2023). Nevertheless, performances were not dramatic because they assumed constant flexural rigidity throughout the annulus.
We design two types of annuli. The first annulus is designed as a ‘wave shield’ that creates a calm free surface within the inner domain of the annulus. This annulus is given by reducing the inlet wave energy factor (i.e. achieving the second state). The second annulus, however, is designed as a ‘cloak’ that blocks wave penetration and suppresses outgoing waves by achieving both first and second states. Flexural rigidities of the plates are optimised to maximise their performances at a target frequency. These parameters are designed within constraints based on a hypothetical scenario to demonstrate the feasibility of the concept, rather than as part of a practical application. We investigate the sensitivity of flexural rigidities to the inlet wave energy factor and scattered-wave energy and show that minimising them is typically in trade-off; achieving both states is challenging. Nevertheless, we demonstrate that this optimisation is successfully achieved by using the floating annulus with multi-plates. We numerically show that both the wave shield and the cloak can create a calm free surface within the inner domain of the annuli. In addition, the outgoing scattering waves can be also suppressed while maintaining a calm free surface using the cloak. The cloak also demonstrates that the wave drift force does not act on the cloak.
2. Theoretical descriptions
2.1. Formulation of the boundary-value problem and its solutions
We design both a floating wave shield and a floating cloak to illustrate the concepts for protecting marine structures from ocean waves. In that sense, a float must be applicable to deep water and performed for multi-directional sea waves. To meet these demands, we use a floating annulus, and the calm free surface is created within the inner radius of the annulus (inner domain). The concept and the problem definitions are shown in figure 1. A three-dimensional Cartesian coordinate system
![]() $O{\text -}xyz$
is defined where the origin
$O{\text -}xyz$
is defined where the origin
![]() $O$
is on the undisturbed (mean) free surface. The vertical axis
$O$
is on the undisturbed (mean) free surface. The vertical axis
![]() $z$
is positive in the upward direction. Although our target is deep sea, we formulate the problem so that numerical calculation can be performed at any water depth, with the flat bottom topography at
$z$
is positive in the upward direction. Although our target is deep sea, we formulate the problem so that numerical calculation can be performed at any water depth, with the flat bottom topography at
![]() $z=-h$
. We consider an elastic annulus floating on the free surface of the sea of which the centre coincides with the origin. The outer and inner radii of the annulus are
$z=-h$
. We consider an elastic annulus floating on the free surface of the sea of which the centre coincides with the origin. The outer and inner radii of the annulus are
![]() $R$
and 1, respectively. The annulus consists of
$R$
and 1, respectively. The annulus consists of
![]() $L$
horizontal concentric annular plates, and adjacent plates are rigidly connected. The annular plates are numbered from the outer to the inner (
$L$
horizontal concentric annular plates, and adjacent plates are rigidly connected. The annular plates are numbered from the outer to the inner (
![]() $\ell=1,2,\ldots ,L$
), and the outer radius of each annular plate is denoted by
$\ell=1,2,\ldots ,L$
), and the outer radius of each annular plate is denoted by
![]() $R^{(\ell)}$
(i.e.
$R^{(\ell)}$
(i.e.
![]() $R^{(1)}=R$
and
$R^{(1)}=R$
and
![]() $R^{(L+1)}=1$
). We do not use any active control force to create a calm space; the space will be made by the passive wave response of the annulus. We assume an elastic motion of the annulus can be described by Kirchhoff’s thin plate theory (Meylan & Squire Reference Meylan and Squire1994; Peter et al. Reference Peter, Meylan and Chung2004). In addition, plane waves with single circular frequency incident on the annulus are considered. Since the annulus is axisymmetric, the wave response does not depend on the incident wave angle (i.e. omnidirectional). Therefore, we simply assume waves are propagating from the negative
$R^{(L+1)}=1$
). We do not use any active control force to create a calm space; the space will be made by the passive wave response of the annulus. We assume an elastic motion of the annulus can be described by Kirchhoff’s thin plate theory (Meylan & Squire Reference Meylan and Squire1994; Peter et al. Reference Peter, Meylan and Chung2004). In addition, plane waves with single circular frequency incident on the annulus are considered. Since the annulus is axisymmetric, the wave response does not depend on the incident wave angle (i.e. omnidirectional). Therefore, we simply assume waves are propagating from the negative
![]() $x$
direction.
$x$
direction.
The problem is formulated under the linear potential flow theory; we assume incompressible and inviscid fluid with the irrotational motion of the flow, and wave amplitude is sufficiently smaller than the wavelength. In addition, a time-harmonic solution of the response is considered. Therefore, we omit the time variable; a frequency domain analysis is carried out. All values are normalised (non-dimensionalised) by the incident wave amplitude, the fluid density, the gravitational acceleration and the inner radius of the annulus. The normalised governing equation and boundary conditions in the frequency domain are given as
where
![]() $\phi$
is the complex amplitude of the velocity potential (i.e.
$\phi$
is the complex amplitude of the velocity potential (i.e.
![]() $\Phi ({\boldsymbol {x}},t)= \textrm{Re}[\phi ({\boldsymbol {x}})$
$\Phi ({\boldsymbol {x}},t)= \textrm{Re}[\phi ({\boldsymbol {x}})$
![]() $\exp (-i\omega t)]$
),
$\exp (-i\omega t)]$
),
![]() $\omega$
is the circular frequency, and
$\omega$
is the circular frequency, and
![]() $\nabla =(\partial /\partial x,\partial /\partial y,\partial /\partial z)$
and
$\nabla =(\partial /\partial x,\partial /\partial y,\partial /\partial z)$
and
![]() $\nabla _\perp =(\partial /\partial x,\partial /\partial y)$
are the three-dimensional and horizontal gradient operators, respectively. In addition,
$\nabla _\perp =(\partial /\partial x,\partial /\partial y)$
are the three-dimensional and horizontal gradient operators, respectively. In addition,
![]() $\beta ^{(\ell)}=D^{(\ell)}/(\rho _w g a^4)$
and
$\beta ^{(\ell)}=D^{(\ell)}/(\rho _w g a^4)$
and
![]() $\gamma =\rho _pt_p/(\rho _w a)$
are non-dimensional flexural rigidity and mass where
$\gamma =\rho _pt_p/(\rho _w a)$
are non-dimensional flexural rigidity and mass where
![]() $\rho _w$
and
$\rho _w$
and
![]() $\rho _p$
are the density of fluid and plate,
$\rho _p$
are the density of fluid and plate,
![]() $g$
is the gravitational acceleration,
$g$
is the gravitational acceleration,
![]() $a$
is the inner radius of the annulus,
$a$
is the inner radius of the annulus,
![]() $t_p$
is the thickness of the annulus,
$t_p$
is the thickness of the annulus,
![]() $D^{(\ell)}=E^{(\ell)}t_p^3/12(1-\nu ^2)$
is the dimensional flexural rigidity,
$D^{(\ell)}=E^{(\ell)}t_p^3/12(1-\nu ^2)$
is the dimensional flexural rigidity,
![]() $E^{(\ell)}$
is the Young’s modulus, and
$E^{(\ell)}$
is the Young’s modulus, and
![]() $\nu$
is Poisson’s ratio. Here, (2.1) is the Laplace equation, (2.2) is the linearised free surface condition of water, (2.3) is the linearised surface condition on the
$\nu$
is Poisson’s ratio. Here, (2.1) is the Laplace equation, (2.2) is the linearised free surface condition of water, (2.3) is the linearised surface condition on the
![]() $\ell$
th plate and (2.4) is the sea bottom condition. Our previous study (Iida et al. Reference Iida, Zareei and Alam2023) revealed that changing flexural rigidity is more effective than that of mass for controlling waves. Therefore, we use different values of flexural rigidity for each plate (i.e.
$\ell$
th plate and (2.4) is the sea bottom condition. Our previous study (Iida et al. Reference Iida, Zareei and Alam2023) revealed that changing flexural rigidity is more effective than that of mass for controlling waves. Therefore, we use different values of flexural rigidity for each plate (i.e.
![]() $\beta ^{(\ell)}$
), and the mass remains constant for all plates (i.e.
$\beta ^{(\ell)}$
), and the mass remains constant for all plates (i.e.
![]() $\gamma$
). Except for this point, other formulations are the same as in Iida et al. (Reference Iida, Zareei and Alam2023), and the detail can be seen in this reference (although notation is slightly changed).
$\gamma$
). Except for this point, other formulations are the same as in Iida et al. (Reference Iida, Zareei and Alam2023), and the detail can be seen in this reference (although notation is slightly changed).
To obtain the solution, the fluid domain is decomposed into an outer domain (
![]() $R\leqslant r$
), plate’s domains (
$R\leqslant r$
), plate’s domains (
![]() $R^{(\ell+1)}\leqslant r\lt R^{(\ell)}$
) and inner domain (
$R^{(\ell+1)}\leqslant r\lt R^{(\ell)}$
) and inner domain (
![]() $0\leqslant r\lt 1$
). Using the variable separation technique and spectral decomposition, the velocity potentials in these domains are given as
$0\leqslant r\lt 1$
). Using the variable separation technique and spectral decomposition, the velocity potentials in these domains are given as
 \begin{align} \phi _{{ outer}} & = \frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\textrm { i}^m\textrm { J}_m(k_0r)f_0(z)\textrm { e}^{\textrm { i}m\theta }\nonumber \\ &\quad +\frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\bigg \{a_{m0}\textrm { H}_m^{(1)}(k_0r)f_0(z)+\sum _{n=1}^\infty a_{mn}\textrm { K}_m(k_nr)f_n(z)\bigg \}\textrm { e}^{\textrm { i}m\theta }\quad R\leqslant r, \end{align}
\begin{align} \phi _{{ outer}} & = \frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\textrm { i}^m\textrm { J}_m(k_0r)f_0(z)\textrm { e}^{\textrm { i}m\theta }\nonumber \\ &\quad +\frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\bigg \{a_{m0}\textrm { H}_m^{(1)}(k_0r)f_0(z)+\sum _{n=1}^\infty a_{mn}\textrm { K}_m(k_nr)f_n(z)\bigg \}\textrm { e}^{\textrm { i}m\theta }\quad R\leqslant r, \end{align}
 \begin{align} \phi _{{ plate}}^{(\ell)} & =\frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\!\Bigg \{b^{(\ell)}_{m0}\textrm { J}_m\big(\mu ^{(k)}_0r\big)F^{(k)}_0(z)+\hspace {-4mm}\sum _{n=-2,n\ne 0}^\infty \hspace {-3mm}b^{(\ell)}_{mn}\textrm { I}_m\big(\mu ^{(\ell)}_nr\big)F^{(\ell)}_n(z)\nonumber \\ & \quad +c^{(\ell)}_{m0}\textrm { H}_m^{(1)}\big(\mu ^{(k)}_0r\big)F^{(k)}_0(z)+\hspace {-4mm}\sum _{n=-2,n\ne 0}^\infty \hspace {-3mm}c^{(\ell)}_{mn}\textrm { K}_m\big(\mu ^{(\ell)}_nr\big)F^{(\ell)}_n(z)\Bigg \}\!\textrm { e}^{\textrm { i}m\theta }\; R^{(\ell+1)}\leqslant r\lt R^{(\ell)},\; \end{align}
\begin{align} \phi _{{ plate}}^{(\ell)} & =\frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\!\Bigg \{b^{(\ell)}_{m0}\textrm { J}_m\big(\mu ^{(k)}_0r\big)F^{(k)}_0(z)+\hspace {-4mm}\sum _{n=-2,n\ne 0}^\infty \hspace {-3mm}b^{(\ell)}_{mn}\textrm { I}_m\big(\mu ^{(\ell)}_nr\big)F^{(\ell)}_n(z)\nonumber \\ & \quad +c^{(\ell)}_{m0}\textrm { H}_m^{(1)}\big(\mu ^{(k)}_0r\big)F^{(k)}_0(z)+\hspace {-4mm}\sum _{n=-2,n\ne 0}^\infty \hspace {-3mm}c^{(\ell)}_{mn}\textrm { K}_m\big(\mu ^{(\ell)}_nr\big)F^{(\ell)}_n(z)\Bigg \}\!\textrm { e}^{\textrm { i}m\theta }\; R^{(\ell+1)}\leqslant r\lt R^{(\ell)},\; \end{align}
 \begin{align} &\phi _{{ inner}}=\frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\bigg \{d_{m0}\textrm { J}_m(k_0r)f_0(z)+\sum _{n=1}^\infty d_{mn}\textrm { I}_m(k_nr)f_n(z)\bigg \}\textrm { e}^{\textrm { i}m\theta }\; 0\leqslant r\lt 1, \end{align}
\begin{align} &\phi _{{ inner}}=\frac {1}{\textrm { i}\omega }\sum _{m=-\infty }^{\infty }\bigg \{d_{m0}\textrm { J}_m(k_0r)f_0(z)+\sum _{n=1}^\infty d_{mn}\textrm { I}_m(k_nr)f_n(z)\bigg \}\textrm { e}^{\textrm { i}m\theta }\; 0\leqslant r\lt 1, \end{align}
where
 \begin{align} f_n(z)=\left \{\begin{array}{l@{\quad}l} \dfrac {\cosh {k_0(z+h)}}{\cosh k_0h}&n=0,\\[4pt] \dfrac {\cos {k_n(z+h)}}{\cos k_nh} &n\gt 0,\\ \end{array}\right .\; F^{(\ell)}_n(z)=\left \{\begin{array}{l@{\quad}l} \dfrac {\cosh {\mu ^{(k)}_0(z+h)}}{\cosh \mu ^{(k)}_0h}&n=0,\\[4pt] \dfrac {\cos {\mu ^{(\ell)}_n(z+h)}}{\cos \mu ^{(\ell)}_nh} &n=-1,-2,n\gt 0. \end{array}\right .\nonumber\\ \end{align}
\begin{align} f_n(z)=\left \{\begin{array}{l@{\quad}l} \dfrac {\cosh {k_0(z+h)}}{\cosh k_0h}&n=0,\\[4pt] \dfrac {\cos {k_n(z+h)}}{\cos k_nh} &n\gt 0,\\ \end{array}\right .\; F^{(\ell)}_n(z)=\left \{\begin{array}{l@{\quad}l} \dfrac {\cosh {\mu ^{(k)}_0(z+h)}}{\cosh \mu ^{(k)}_0h}&n=0,\\[4pt] \dfrac {\cos {\mu ^{(\ell)}_n(z+h)}}{\cos \mu ^{(\ell)}_nh} &n=-1,-2,n\gt 0. \end{array}\right .\nonumber\\ \end{align}
Here,
![]() $\textrm { J}_m(\cdot )$
,
$\textrm { J}_m(\cdot )$
,
![]() $\textrm { H}^{(1)}_m(\cdot )$
,
$\textrm { H}^{(1)}_m(\cdot )$
,
![]() $\textrm { I}_m(\cdot )$
and
$\textrm { I}_m(\cdot )$
and
![]() $\textrm { K}_m(\cdot )$
are the Bessel function of the first kind, the Hankel function of the first kind, and the modified Bessel functions of the first and second kinds, respectively. Functions
$\textrm { K}_m(\cdot )$
are the Bessel function of the first kind, the Hankel function of the first kind, and the modified Bessel functions of the first and second kinds, respectively. Functions
![]() $f_n(z)$
and
$f_n(z)$
and
![]() $F_n^{(\ell)}(z)$
are solutions of the velocity potentials for
$F_n^{(\ell)}(z)$
are solutions of the velocity potentials for
![]() $z$
. In addition,
$z$
. In addition,
![]() $k_n$
and
$k_n$
and
![]() $\mu _n^{(\ell)}$
are the wavenumbers of water waves and elastic waves of the
$\mu _n^{(\ell)}$
are the wavenumbers of water waves and elastic waves of the
![]() $\ell$
th plate which satisfy the following dispersion relations (Peter et al. Reference Peter, Meylan and Chung2004):
$\ell$
th plate which satisfy the following dispersion relations (Peter et al. Reference Peter, Meylan and Chung2004):
and
 \begin{eqnarray} \frac {\omega ^2}{\beta ^{(\ell)}\mu _n^{(\ell)4}-\gamma \omega ^2+1}=\left \{\begin{array}{l@{\quad}l} \mu ^{(k)}_0\tanh {\mu ^{(k)}_0h},&n=0,\\[4pt] -\mu ^{(\ell)}_n\tan {\mu ^{(\ell)}_nh}, &n=-1,-2,n\gt 0. \end{array}\right . \end{eqnarray}
\begin{eqnarray} \frac {\omega ^2}{\beta ^{(\ell)}\mu _n^{(\ell)4}-\gamma \omega ^2+1}=\left \{\begin{array}{l@{\quad}l} \mu ^{(k)}_0\tanh {\mu ^{(k)}_0h},&n=0,\\[4pt] -\mu ^{(\ell)}_n\tan {\mu ^{(\ell)}_nh}, &n=-1,-2,n\gt 0. \end{array}\right . \end{eqnarray}
Note that the wavenumber
![]() $k_n$
has an infinite number of positive and real solutions, and
$k_n$
has an infinite number of positive and real solutions, and
![]() $\mu _n^{(\ell)}$
also has an infinite number of positive and real solutions and two complex solutions
$\mu _n^{(\ell)}$
also has an infinite number of positive and real solutions and two complex solutions
![]() $\mu _{-1}^{(\ell)}=(\mu _{-2}^{(\ell)})^*$
where these real parts are positive (Fox & Squire Reference Fox and Squire1994). To obtain the complex amplitudes
$\mu _{-1}^{(\ell)}=(\mu _{-2}^{(\ell)})^*$
where these real parts are positive (Fox & Squire Reference Fox and Squire1994). To obtain the complex amplitudes
![]() $a_{mn}$
,
$a_{mn}$
,
![]() $b_{mn}^{(\ell)}$
,
$b_{mn}^{(\ell)}$
,
![]() $c_{mn}^{(\ell)}$
and
$c_{mn}^{(\ell)}$
and
![]() $d_{mn}$
, further boundary conditions between adjacent domains are considered (see Appendix A). In this paper, the eigenvalue matching method (Peter et al. Reference Peter, Meylan and Chung2004; Iida et al. Reference Iida, Zareei and Alam2023) is employed to solve them.
$d_{mn}$
, further boundary conditions between adjacent domains are considered (see Appendix A). In this paper, the eigenvalue matching method (Peter et al. Reference Peter, Meylan and Chung2004; Iida et al. Reference Iida, Zareei and Alam2023) is employed to solve them.
2.2. Inlet wave energy factor within the inner domain of the annulus
To measure the calmness of the free surface within the inner domain of the annulus, we derive a mean wave energy density in the inner domain (namely, inlet wave energy density). At first, the time average of the kinetic energy density is given as
Since the incident wave energy is not distributed to local waves (i.e. energy conservation principle at far-field), the local wave component of (2.7) is not necessary to consider the energy balance. Therefore, substituting the first term of (2.7) into (2.11), the kinetic energy density in the inner domain is calculated as
 \begin{align} \overline {E_{{ kinetic}}}& = \frac {1}{16}\sum _{m=-\infty }^\infty |d_{m0}|^2\bigg [\bigg (1+\frac {2k_0h}{\sinh {2k_0h}}\bigg )\bigg \{\textrm { J}^{\prime 2}_{m-1}(k_0)+\bigg (1-\frac {(m-1)^2}{k_0^2}\bigg )\textrm { J}_{m-1}^2(k_0)\bigg \}\nonumber \\ &+\bigg (1+\frac {2k_0h}{\sinh {2k_0h}}\bigg )\bigg \{\textrm { J}^{\prime 2}_{m+1} (k_0)+\bigg (1-\frac {(m+1)^2}{k_0^2}\bigg )\textrm { J}_{m+1}^2(k_0)\bigg \}\nonumber \\ &+2\bigg (1-\frac {2k_0h}{\sinh {2k_0h}}\bigg )\bigg \{\textrm { J}^{\prime 2}_{m} (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_{m}^2(k_0)\bigg \} \bigg ]. \end{align}
\begin{align} \overline {E_{{ kinetic}}}& = \frac {1}{16}\sum _{m=-\infty }^\infty |d_{m0}|^2\bigg [\bigg (1+\frac {2k_0h}{\sinh {2k_0h}}\bigg )\bigg \{\textrm { J}^{\prime 2}_{m-1}(k_0)+\bigg (1-\frac {(m-1)^2}{k_0^2}\bigg )\textrm { J}_{m-1}^2(k_0)\bigg \}\nonumber \\ &+\bigg (1+\frac {2k_0h}{\sinh {2k_0h}}\bigg )\bigg \{\textrm { J}^{\prime 2}_{m+1} (k_0)+\bigg (1-\frac {(m+1)^2}{k_0^2}\bigg )\textrm { J}_{m+1}^2(k_0)\bigg \}\nonumber \\ &+2\bigg (1-\frac {2k_0h}{\sinh {2k_0h}}\bigg )\bigg \{\textrm { J}^{\prime 2}_{m} (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_{m}^2(k_0)\bigg \} \bigg ]. \end{align}
Similarly, the time average of the potential energy density is obtained as
 \begin{eqnarray} \overline {E_{{ potential}}}&=&\frac {1}{\pi }\overline {\int _{0}^\zeta \int _0^{2\pi }\int _0^1zr{\rm d}r{\rm d}\theta {\rm d}z}\nonumber \\ &=&\frac {1}{4}\sum _{m=-\infty }^\infty |d_{m0}|^2\bigg \{\textrm { J}^{\prime 2}_m (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_m^2(k_0)\bigg \}. \end{eqnarray}
\begin{eqnarray} \overline {E_{{ potential}}}&=&\frac {1}{\pi }\overline {\int _{0}^\zeta \int _0^{2\pi }\int _0^1zr{\rm d}r{\rm d}\theta {\rm d}z}\nonumber \\ &=&\frac {1}{4}\sum _{m=-\infty }^\infty |d_{m0}|^2\bigg \{\textrm { J}^{\prime 2}_m (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_m^2(k_0)\bigg \}. \end{eqnarray}
The inlet wave energy density is given by the sum of these kinetic and potential energy densities, as
 \begin{align} \overline {E_{{ inlet}}}& =\overline {E_{{ kinetic}}} + \overline {E_{{ potential}}}\nonumber \\& =\sum _{m=-\infty }^\infty |d_{m0}|^2 \bigg [\frac {\omega ^2+\big(k_0^2-\omega ^4\big)h}{16\omega ^2}\bigg \{\textrm { J}^{\prime 2}_{m-1} (k_0)+\bigg (1-\frac {(m-1)^2}{k_0^2}\bigg )\textrm { J}_{m-1}^2(k_0)\bigg \}\nonumber \\ &\quad +\frac {\omega ^2+\big(k_0^2-\omega ^4\big)h}{16\omega ^2}\bigg \{\textrm { J}^{\prime 2}_{m+1} (k_0)+\bigg (1-\frac {(m+1)^2}{k_0^2}\bigg )\textrm { J}_{m+1}^2(k_0)\bigg \}\nonumber \\ &\quad +\frac {3\omega ^2-\big(k_0^2-\omega ^4\big)h}{8\omega ^2}\bigg \{\textrm { J}^{\prime 2}_{m} (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_{m}^2(k_0)\bigg \} \bigg ]. \end{align}
\begin{align} \overline {E_{{ inlet}}}& =\overline {E_{{ kinetic}}} + \overline {E_{{ potential}}}\nonumber \\& =\sum _{m=-\infty }^\infty |d_{m0}|^2 \bigg [\frac {\omega ^2+\big(k_0^2-\omega ^4\big)h}{16\omega ^2}\bigg \{\textrm { J}^{\prime 2}_{m-1} (k_0)+\bigg (1-\frac {(m-1)^2}{k_0^2}\bigg )\textrm { J}_{m-1}^2(k_0)\bigg \}\nonumber \\ &\quad +\frac {\omega ^2+\big(k_0^2-\omega ^4\big)h}{16\omega ^2}\bigg \{\textrm { J}^{\prime 2}_{m+1} (k_0)+\bigg (1-\frac {(m+1)^2}{k_0^2}\bigg )\textrm { J}_{m+1}^2(k_0)\bigg \}\nonumber \\ &\quad +\frac {3\omega ^2-\big(k_0^2-\omega ^4\big)h}{8\omega ^2}\bigg \{\textrm { J}^{\prime 2}_{m} (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_{m}^2(k_0)\bigg \} \bigg ]. \end{align}
When the floating annulus is absent, the velocity potential of plane waves is of course represented by the first term of (2.5). Then, the mean wave energy density for any free surface position should be calculated by replacing
![]() $d_{m0}$
in (2.14) with
$d_{m0}$
in (2.14) with
![]() $\textrm { i}^m$
. The result is obtained as
$\textrm { i}^m$
. The result is obtained as
Note that the relation
 \begin{eqnarray} \sum _{m=-\infty }^\infty \bigg \{\textrm { J}^{\prime 2}_{m} (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_{m}^2(k_0)\bigg \}=1 \end{eqnarray}
\begin{eqnarray} \sum _{m=-\infty }^\infty \bigg \{\textrm { J}^{\prime 2}_{m} (k_0)+\bigg (1-\frac {m^2}{k_0^2}\bigg )\textrm { J}_{m}^2(k_0)\bigg \}=1 \end{eqnarray}
is used. The mean energy density (2.15) is the same result as the well-known one (Newman Reference Newman2018a
) where the dimensional expression is
![]() $\rho _w g\zeta ^2/2$
.
$\rho _w g\zeta ^2/2$
.
The calmness of the inner domain is evaluated by the ratio of the inlet wave energy density to the mean density of the plane wave, namely the inlet wave energy factor, defined by
The calm free surface is realised by
![]() $\mathcal {F}_{{ inlet}}\to 0$
.
$\mathcal {F}_{{ inlet}}\to 0$
.
It should be emphasised that the calmness of the inner domain should be evaluated by the energy throughout the inner domain. If the energy at a single point, such as the origin, is considered, there is a possibility that such a point could become a node of standing waves, which does not ensure calmness throughout the inner domain. In practice, it is simpler to minimise the wave amplitude
![]() $\sum _{m=-\infty }^\infty |d_{m0}|^2$
instead of (2.16). However, by using (2.16), the ratio to the incident wave energy can be defined as (2.17), and this makes physical interpretation easier than minimisation of the wave amplitude.
$\sum _{m=-\infty }^\infty |d_{m0}|^2$
instead of (2.16). However, by using (2.16), the ratio to the incident wave energy can be defined as (2.17), and this makes physical interpretation easier than minimisation of the wave amplitude.
2.3. Scattered-wave energy and wave drift force
The amount of waves scattered by the annulus is evaluated by the scattered-wave energy (Iida et al. Reference Iida, Kashiwagi and He2014). The energy conservation principle at far-field is given as (Kashiwagi et al. Reference Kashiwagi, Endo and Yamaguchi2005)
 \begin{eqnarray} W=\frac {1}{C_0k_0\omega }\sum _{m=-\infty }^\infty \left \{\textrm { Re}\big[\textrm { i}^ma_{m0}^*\big]+|a_{m0}|^2\right \}=0, \end{eqnarray}
\begin{eqnarray} W=\frac {1}{C_0k_0\omega }\sum _{m=-\infty }^\infty \left \{\textrm { Re}\big[\textrm { i}^ma_{m0}^*\big]+|a_{m0}|^2\right \}=0, \end{eqnarray}
where
Note that (2.18) can be used for verification of the numerical code (this will be demonstrated in § 2.4). The scattered-wave energy is defined by the second term of (2.18), i.e.
 \begin{eqnarray} W_S=\frac {1}{C_0k_0\omega }\sum _{m=-\infty }^\infty |a_{m0}|^2. \end{eqnarray}
\begin{eqnarray} W_S=\frac {1}{C_0k_0\omega }\sum _{m=-\infty }^\infty |a_{m0}|^2. \end{eqnarray}
Here,
![]() $a_{m0}$
is the complex amplitude of the outgoing scattering waves, and thus (2.20) indicates the energy of scattering waves. A zero scattering wave is realised by
$a_{m0}$
is the complex amplitude of the outgoing scattering waves, and thus (2.20) indicates the energy of scattering waves. A zero scattering wave is realised by
![]() $W_S\to 0$
.
$W_S\to 0$
.
The wave drift force, the steady component of the second-order hydrodynamic forces, acting on the annulus is also given by the complex amplitude of scattering waves (Kashiwagi et al. Reference Kashiwagi, Endo and Yamaguchi2005), as
 \begin{eqnarray} \overline {F_x}=\frac {1}{2C_0\omega }\sum _{m=-\infty }^\infty \textrm { Im}\big[2a_{m0}a_{m+1,0}^*+\textrm { i}^ma_{m+1,0}^*+(-\textrm { i})^{m+1}a_{m0} \big]. \end{eqnarray}
\begin{eqnarray} \overline {F_x}=\frac {1}{2C_0\omega }\sum _{m=-\infty }^\infty \textrm { Im}\big[2a_{m0}a_{m+1,0}^*+\textrm { i}^ma_{m+1,0}^*+(-\textrm { i})^{m+1}a_{m0} \big]. \end{eqnarray}
As (2.21) indicates, the wave drift force becomes very small when no wave is scattered by the annulus.
2.4. Design of the annulus
We design the annulus as a wave shield to prevent waves from invading its inner domain. Additionally, we design another annulus as a cloak to block wave penetration and suppress outgoing waves. Therefore, the following single-objective optimisation problem is considered:
where
![]() $w_1$
and
$w_1$
and
![]() $w_2$
are the weights. For the wave shield, we consider
$w_2$
are the weights. For the wave shield, we consider
![]() $w_1=1$
and
$w_1=1$
and
![]() $w_2=0$
. For the cloak, however,
$w_2=0$
. For the cloak, however,
![]() $w_1=w_2=1$
is used as a simple allocation. Note that the original dimensions of
$w_1=w_2=1$
is used as a simple allocation. Note that the original dimensions of
![]() $\mathcal {F}_{{ inlet}}$
and
$\mathcal {F}_{{ inlet}}$
and
![]() $W_S$
are different (although these are normalised here). The mean energy density has the dimension
$W_S$
are different (although these are normalised here). The mean energy density has the dimension
![]() $\textrm { kg}\textrm { s}^-{^2}$
, the inlet wave energy factor is dimensionless and the scattered-wave energy has the dimension
$\textrm { kg}\textrm { s}^-{^2}$
, the inlet wave energy factor is dimensionless and the scattered-wave energy has the dimension
![]() $\textrm { kg}\, \textrm { m}^2\textrm { s}^-{^3}$
. We do not consider
$\textrm { kg}\, \textrm { m}^2\textrm { s}^-{^3}$
. We do not consider
![]() $f=W_s$
(i.e.
$f=W_s$
(i.e.
![]() $w_1=0$
and
$w_1=0$
and
![]() $w_2=1$
) because this optimal solution is given by
$w_2=1$
) because this optimal solution is given by
![]() $\beta ^{(\ell)}\to 0$
; the annulus is negligibly soft.
$\beta ^{(\ell)}\to 0$
; the annulus is negligibly soft.
We solve the single optimisation (2.22) at non-dimensional wavenumber
![]() $k_0=1.0$
subject to
$k_0=1.0$
subject to
![]() $\nu =0.25$
,
$\nu =0.25$
,
![]() $\gamma =0.05$
and the constraint
$\gamma =0.05$
and the constraint
![]() $0.001\leqslant \beta ^{(\ell)}\leqslant$
220. We also assume the outer radius of the annulus is
$0.001\leqslant \beta ^{(\ell)}\leqslant$
220. We also assume the outer radius of the annulus is
![]() $R=5.0$
and the radial widths of all plates are the same. This condition reflects the following scenario: a calm free surface with a radius of 16 m is created against typical ocean waves with a wavelength of 100 m (i.e. the period is 8 s in deep water). Assuming 2 m for the thickness of the annulus, the dimensional values of the constraint of Young’s modulus are
$R=5.0$
and the radial widths of all plates are the same. This condition reflects the following scenario: a calm free surface with a radius of 16 m is created against typical ocean waves with a wavelength of 100 m (i.e. the period is 8 s in deep water). Assuming 2 m for the thickness of the annulus, the dimensional values of the constraint of Young’s modulus are
![]() $9\times 10^{-4}\sim 200\rm \,GPa$
, where these minimum and maximum constraints correspond to the properties of rubber and steel. In addition,
$9\times 10^{-4}\sim 200\rm \,GPa$
, where these minimum and maximum constraints correspond to the properties of rubber and steel. In addition,
![]() $\gamma =0.05$
is equivalent to the density ratio
$\gamma =0.05$
is equivalent to the density ratio
![]() $\rho _p/\rho _w=0.4$
(
$\rho _p/\rho _w=0.4$
(
![]() $\rho _w=1025\,\rm kg\,m^-{^3}$
is considered). Note that this scenario is set up to demonstrate that the annulus can be designed within a feasible range, but it is not necessarily useful for practical applications (for instance, the outer radius is considerably larger than that of the cloaked domain).
$\rho _w=1025\,\rm kg\,m^-{^3}$
is considered). Note that this scenario is set up to demonstrate that the annulus can be designed within a feasible range, but it is not necessarily useful for practical applications (for instance, the outer radius is considerably larger than that of the cloaked domain).
To seek an optimum design, the covariance matrix adaptation evolution strategy (CMA-ES; Hansen Reference Hansen2016) is employed. The CMA-ES uses the covariance matrix of a multivariate normal distribution for updating a searching area, and efficient optimisations can be achieved even for non-separable and ill-conditioned problems. Since all the tuning parameters have default recommendations (Hansen Reference Hansen2016), the CMA-ES could be applied to various problems without devoting effort to tuning these parameters. To find the optimal cloak design, multi-objective optimisation would be more suitable. However, since we only have the in-house code for the single-objective optimisation, we solve (2.22) in this study.
For the numerical simulations, finite truncating numbers
![]() $M=20$
and
$M=20$
and
![]() $N=15$
are used for velocity potentials (see Appendix A). Then, the simulations demonstrate good energy conservation (2.18) with an accuracy of less than
$N=15$
are used for velocity potentials (see Appendix A). Then, the simulations demonstrate good energy conservation (2.18) with an accuracy of less than
![]() $O(10^{-14})$
. The water depth is assumed
$O(10^{-14})$
. The water depth is assumed
![]() $h=\lambda$
(wavelength) to ensure the deep water assumption throughout all the frequencies.
$h=\lambda$
(wavelength) to ensure the deep water assumption throughout all the frequencies.
3. Numerical results and discussion
3.1. Sensitivity study
Before conducting the optimisation, we first investigate the sensitivity of the combinations of
![]() $\beta ^{(\ell)}$
to the objective functions. We consider the two-plates case (
$\beta ^{(\ell)}$
to the objective functions. We consider the two-plates case (
![]() $L=2$
), and the contour maps of
$L=2$
), and the contour maps of
![]() $\log _{10}(\mathcal {F}_{{ inlet}})$
and
$\log _{10}(\mathcal {F}_{{ inlet}})$
and
![]() $\log _{10}(W_S)$
against
$\log _{10}(W_S)$
against
![]() $\beta ^{(1)}$
(outer plate) and
$\beta ^{(1)}$
(outer plate) and
![]() $\beta ^{(2)}$
(inner plate) are plotted in figure 2. Here, common logarithm is used to highlight the differences among small values. Looking at figure 2(a), there is a peak around
$\beta ^{(2)}$
(inner plate) are plotted in figure 2. Here, common logarithm is used to highlight the differences among small values. Looking at figure 2(a), there is a peak around
![]() $(\beta ^{(1)},\beta ^{(2)})=(10,15)$
. As
$(\beta ^{(1)},\beta ^{(2)})=(10,15)$
. As
![]() $\beta ^{(1)}$
and
$\beta ^{(1)}$
and
![]() $\beta ^{(2)}$
increase,
$\beta ^{(2)}$
increase,
![]() $\mathcal {F}_{{ inlet}}$
decreases. Influences of
$\mathcal {F}_{{ inlet}}$
decreases. Influences of
![]() $\beta ^{(1)}$
and
$\beta ^{(1)}$
and
![]() $\beta ^{(2)}$
are not symmetrical; increasing
$\beta ^{(2)}$
are not symmetrical; increasing
![]() $\beta ^{(2)}$
results in a faster decrease in
$\beta ^{(2)}$
results in a faster decrease in
![]() $\mathcal {F}_{{ inlet}}$
. The result of
$\mathcal {F}_{{ inlet}}$
. The result of
![]() $W_S$
in figure 2(b) has similar contour lines around
$W_S$
in figure 2(b) has similar contour lines around
![]() $(\beta ^{(1)},\beta ^{(2)})=(10,15)$
, but this area becomes a valley (smaller than others). In addition, this also has another valley line around
$(\beta ^{(1)},\beta ^{(2)})=(10,15)$
, but this area becomes a valley (smaller than others). In addition, this also has another valley line around
![]() $\beta ^{(2)}=0.18(\beta ^{(1)}-130)$
. In contrast to
$\beta ^{(2)}=0.18(\beta ^{(1)}-130)$
. In contrast to
![]() $\mathcal {F}_{{ inlet}}$
,
$\mathcal {F}_{{ inlet}}$
,
![]() $W_S$
increases as
$W_S$
increases as
![]() $\beta ^{(1)}$
and
$\beta ^{(1)}$
and
![]() $\beta ^{(2)}$
increase. These indicate that bigger flexural rigidity leads to more incident waves being scattered (or reflected) at the outer boundary of the annulus, and it results in the prevention of wave propagation into the inner domain. Therefore,
$\beta ^{(2)}$
increase. These indicate that bigger flexural rigidity leads to more incident waves being scattered (or reflected) at the outer boundary of the annulus, and it results in the prevention of wave propagation into the inner domain. Therefore,
![]() $\mathcal {F}_{{ inlet}}\to 0$
and
$\mathcal {F}_{{ inlet}}\to 0$
and
![]() $W_S\to 0$
are typically in trade-off. As the plate number
$W_S\to 0$
are typically in trade-off. As the plate number
![]() $L$
increases, the solution becomes more complex, and the objective function is likely to become a multi-modal function. Therefore, a metaheuristic optimisation approach (i.e. the CMA-ES) would be suitable to find an optimal solution.
$L$
increases, the solution becomes more complex, and the objective function is likely to become a multi-modal function. Therefore, a metaheuristic optimisation approach (i.e. the CMA-ES) would be suitable to find an optimal solution.

Figure 2. Sensitivity studies of the flexural rigidities
![]() $\beta ^{(1)}$
(outer plate) and
$\beta ^{(1)}$
(outer plate) and
![]() $\beta ^{(2)}$
(inner plate) to (a) inlet wave energy factor
$\beta ^{(2)}$
(inner plate) to (a) inlet wave energy factor
![]() $\mathcal {F}_{{ inlet}}$
and (b) scattered-wave energy
$\mathcal {F}_{{ inlet}}$
and (b) scattered-wave energy
![]() $W_S$
. Results are presented using their common logarithm. Two plates case (
$W_S$
. Results are presented using their common logarithm. Two plates case (
![]() $L=2$
) is considered where
$L=2$
) is considered where
![]() $\beta ^{(1)}$
is of the outer plate and
$\beta ^{(1)}$
is of the outer plate and
![]() $\beta ^{(2)}$
is of the inner plate.
$\beta ^{(2)}$
is of the inner plate.
3.2. Optimisation
The annuli are optimised to minimise
![]() $f=\mathcal {F}_{{ inlet}}$
(inlet waves) and
$f=\mathcal {F}_{{ inlet}}$
(inlet waves) and
![]() $f=\mathcal {F}_{{ inlet}}+W_S$
(inlet waves and scattering waves), respectively. Optimisation results for the cases of the plate number
$f=\mathcal {F}_{{ inlet}}+W_S$
(inlet waves and scattering waves), respectively. Optimisation results for the cases of the plate number
![]() $L=1$
, 2, 4, 8, and 16 are shown in table 1. Looking at the results for
$L=1$
, 2, 4, 8, and 16 are shown in table 1. Looking at the results for
![]() $f=\mathcal {F}_{{ inlet}}$
, the inlet wave energy factor is less than 10 % for all plate numbers and less than 1% when
$f=\mathcal {F}_{{ inlet}}$
, the inlet wave energy factor is less than 10 % for all plate numbers and less than 1% when
![]() $L\geqslant 4$
. However, the scattered-wave energy is maintained at
$L\geqslant 4$
. However, the scattered-wave energy is maintained at
![]() $W_S\approx 5$
, and thus incident waves are highly scattered by the annulus. As for the results for
$W_S\approx 5$
, and thus incident waves are highly scattered by the annulus. As for the results for
![]() $f=\mathcal {F}_{{ inlet}}+W_S$
, cases of
$f=\mathcal {F}_{{ inlet}}+W_S$
, cases of
![]() $L=1$
, 2 and 4 show a reduction only in the scattered-wave energy, while the inlet wave energy factor remains at 1. This indicates that the optimisation is performed to minimise
$L=1$
, 2 and 4 show a reduction only in the scattered-wave energy, while the inlet wave energy factor remains at 1. This indicates that the optimisation is performed to minimise
![]() $W_S$
, and this results in
$W_S$
, and this results in
![]() $\beta ^{(\ell)}\to 0$
(
$\beta ^{(\ell)}\to 0$
(
![]() $\mathcal {F}_{{ inlet}}$
is not exactly 1 because the mass
$\mathcal {F}_{{ inlet}}$
is not exactly 1 because the mass
![]() $\gamma$
is a finite value). However, the results of
$\gamma$
is a finite value). However, the results of
![]() $L=8$
and 16 demonstrate reductions of both inlet wave energy factor and scattered-wave energy. Especially, the result of
$L=8$
and 16 demonstrate reductions of both inlet wave energy factor and scattered-wave energy. Especially, the result of
![]() $L=16$
can dramatically suppress the scattered-wave energy while keeping a low inlet wave energy factor. In both objective functions, the inlet wave energy factor can be reduced to a sufficiently small level for practical purposes. Therefore, the structure of the wave shield is not unique.
$L=16$
can dramatically suppress the scattered-wave energy while keeping a low inlet wave energy factor. In both objective functions, the inlet wave energy factor can be reduced to a sufficiently small level for practical purposes. Therefore, the structure of the wave shield is not unique.
Table 1. Optimisation results of the annulus designed as the wave shield and the cloak.

Figure 3 shows a plot of
![]() $\beta ^{(\ell)}$
along the radial direction of the annulus (
$\beta ^{(\ell)}$
along the radial direction of the annulus (
![]() $1\leqslant r\leqslant 5$
). The result of the wave shield is shown in figure 3(a), while the result of the cloak is in figure 3(b). Cases of
$1\leqslant r\leqslant 5$
). The result of the wave shield is shown in figure 3(a), while the result of the cloak is in figure 3(b). Cases of
![]() $L=8$
and 16 are shown. In figure 3(a), there is a peak around
$L=8$
and 16 are shown. In figure 3(a), there is a peak around
![]() $2.5\leqslant r \leqslant 4$
, where
$2.5\leqslant r \leqslant 4$
, where
![]() $\beta ^{(\ell)}$
reaches the maximum constraint value of 220. Outside this area,
$\beta ^{(\ell)}$
reaches the maximum constraint value of 220. Outside this area,
![]() $\beta ^{(\ell)}$
becomes small. In figure 3(b), there are two peaks for
$\beta ^{(\ell)}$
becomes small. In figure 3(b), there are two peaks for
![]() $L=16$
. At the peaks,
$L=16$
. At the peaks,
![]() $\beta ^{(\ell)}$
reaches the maximum constraint; outside the peaks, it is nearly at the minimum constraint; and inside the peaks, it takes on intermediate values. Such a structure cannot be achieved by
$\beta ^{(\ell)}$
reaches the maximum constraint; outside the peaks, it is nearly at the minimum constraint; and inside the peaks, it takes on intermediate values. Such a structure cannot be achieved by
![]() $L=4$
.
$L=4$
.
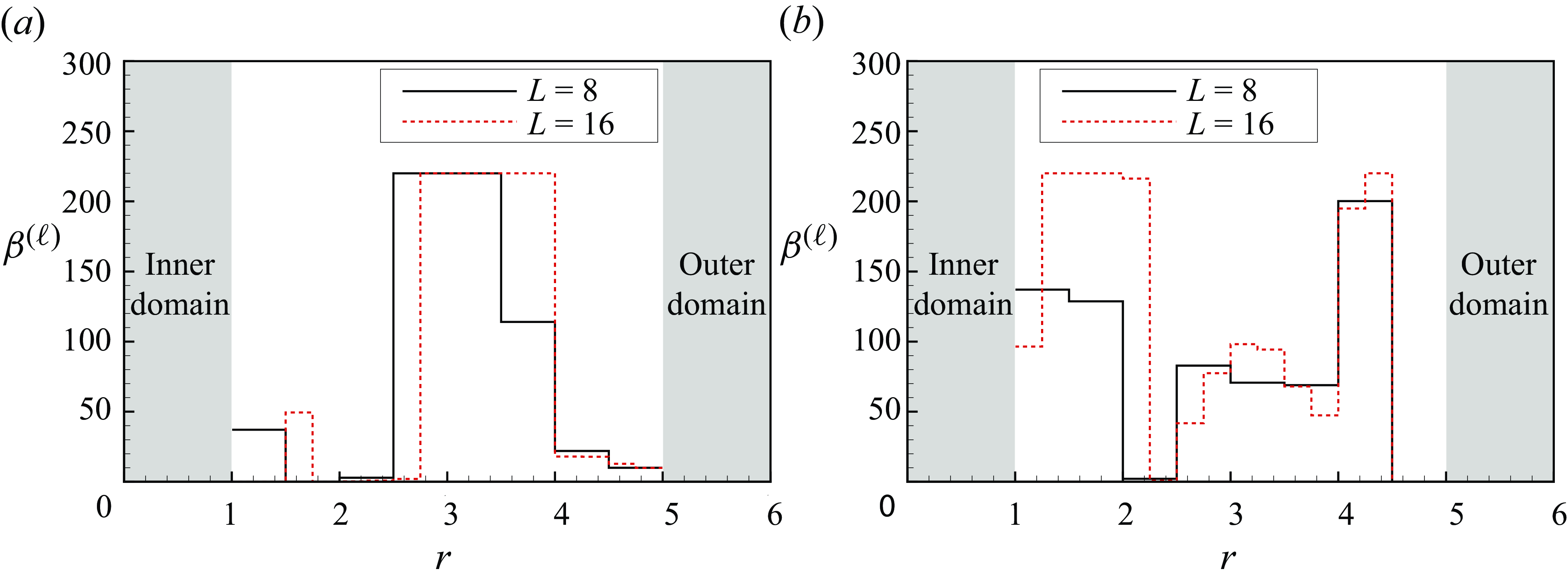
Figure 3. Spatial distributions of flexural rigidity
![]() $\beta ^{(\ell)}$
along the radial direction of the annulus (
$\beta ^{(\ell)}$
along the radial direction of the annulus (
![]() $1\leqslant r\leqslant 5$
). (a) Optimised result for wave shields (
$1\leqslant r\leqslant 5$
). (a) Optimised result for wave shields (
![]() $f=\mathcal {F}_{{ inlet}}$
). (b) Optimised result for cloaks (
$f=\mathcal {F}_{{ inlet}}$
). (b) Optimised result for cloaks (
![]() $f=\mathcal {F}_{{ inlet}}+W_S$
). Flexural rigidities are optimised within the constraint
$f=\mathcal {F}_{{ inlet}}+W_S$
). Flexural rigidities are optimised within the constraint
![]() $0.001\leqslant \beta ^{(\ell)}\leqslant 220$
.
$0.001\leqslant \beta ^{(\ell)}\leqslant 220$
.
Snapshots of wave fields are plotted in figures 4 and 5, where plane waves are incident from the left-hand side of the figures. Figure 4 is the result for the wave shield and figure 5 is for the cloak. Panels (a,c) are the snapshots of wave patterns (imaginary parts of complex wave amplitude) and panels (b,d) are the wave amplitudes (magnitude of complex wave amplitude). Case
![]() $L=8$
is shown in panel (a,b), and
$L=8$
is shown in panel (a,b), and
![]() $L=16$
is in panel (c,d). Figure 4 shows that almost no waves propagate into the inner domain of the annulus, and elastic waves on the annulus are also small. These indicate that incident waves are scattered by the outer boundary of the annulus. As a result, there is a shadow behind the wave shield. In figure 5, wave penetration is also prevented by the cloak. In addition, there are a few outgoing scattering waves outside the cloak (see panel b,d). Especially, case
$L=16$
is in panel (c,d). Figure 4 shows that almost no waves propagate into the inner domain of the annulus, and elastic waves on the annulus are also small. These indicate that incident waves are scattered by the outer boundary of the annulus. As a result, there is a shadow behind the wave shield. In figure 5, wave penetration is also prevented by the cloak. In addition, there are a few outgoing scattering waves outside the cloak (see panel b,d). Especially, case
![]() $L=16$
achieves seemingly perfect cloaking condition.
$L=16$
achieves seemingly perfect cloaking condition.
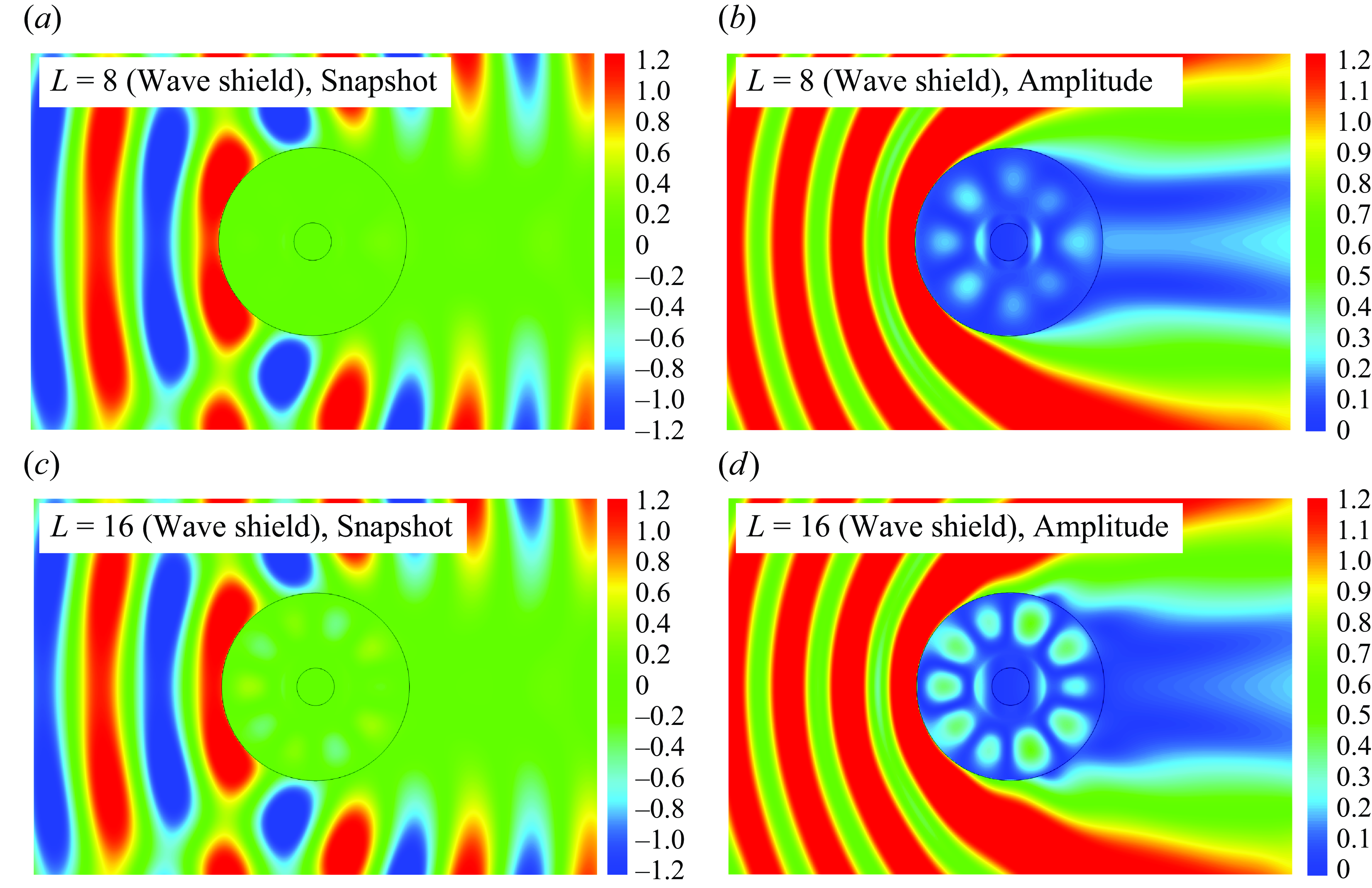
Figure 4. Wave fields for the ‘wave shield’ at wavenumber
![]() $k_0=1.0$
. (a,c) Snapshots of wave patterns. (b,d) Wave amplitude. The results of plate numbers
$k_0=1.0$
. (a,c) Snapshots of wave patterns. (b,d) Wave amplitude. The results of plate numbers
![]() $L=8$
and 16 are shown. Plane waves are incident from the left-hand side of the figures. The corresponding movie of panel (c) is available as supplementary movie 1 available at https://doi.org/10.1017/jfm.2025.106.
$L=8$
and 16 are shown. Plane waves are incident from the left-hand side of the figures. The corresponding movie of panel (c) is available as supplementary movie 1 available at https://doi.org/10.1017/jfm.2025.106.

Figure 5. Wave fields for the ‘cloak’ at wavenumber
![]() $k_0=1.0$
. (a,c) Snapshots of wave patterns. (b,d) Wave amplitude. The results of plate numbers
$k_0=1.0$
. (a,c) Snapshots of wave patterns. (b,d) Wave amplitude. The results of plate numbers
![]() $L=8$
and 16 are shown. Plane waves are incident from the left-hand side of the figures. The corresponding movie of panel (c) is available as supplementary movie 2.
$L=8$
and 16 are shown. Plane waves are incident from the left-hand side of the figures. The corresponding movie of panel (c) is available as supplementary movie 2.

Figure 6. (a) Inlet wave energy factor
![]() $\mathcal {F}_{{ inlet}}$
against wavenumber
$\mathcal {F}_{{ inlet}}$
against wavenumber
![]() $k_0$
. (b) Scattered-wave energy
$k_0$
. (b) Scattered-wave energy
![]() $W_S$
against wavenumber
$W_S$
against wavenumber
![]() $k_0$
. Both panels are plotted on a semi-log graph. The annuli are optimised at
$k_0$
. Both panels are plotted on a semi-log graph. The annuli are optimised at
![]() $k_0=1.0$
.
$k_0=1.0$
.
To investigate the frequency responses, inlet wave energy factor
![]() $\mathcal {F}_{{ inlet}}$
and scattered-wave energy
$\mathcal {F}_{{ inlet}}$
and scattered-wave energy
![]() $W_S$
against wavenumber
$W_S$
against wavenumber
![]() $k_0$
are plotted in figures 6(a) and 6(b), respectively. The results are plotted on a semi-log graph. In figure 6(a), the line on
$k_0$
are plotted in figures 6(a) and 6(b), respectively. The results are plotted on a semi-log graph. In figure 6(a), the line on
![]() $\mathcal {F}_{{ inlet}}=10^0=1$
indicates the same energy density as that of plane waves. In the long-wavelength region (
$\mathcal {F}_{{ inlet}}=10^0=1$
indicates the same energy density as that of plane waves. In the long-wavelength region (
![]() $k_0\lt 0.3$
), the results converge to
$k_0\lt 0.3$
), the results converge to
![]() $\mathcal {F}_{{ inlet}}=1$
because the annulus merely follows the motion of the waves without scattering them. There are some periodic peaks due to resonance in the inner domain, which is typically known as moonpool resonance (e.g. Newman Reference Newman2018b
). Similar results are found from Malenica et al. (Reference Malenica, Liang, Zheng and Korobkin2023). Since the simulation is based on the linear potential theory, the amplified wave energy is unrealistically high. In real situations, these waves are dissipated by nonlinear processes, such as viscosity and wave-breaking. Except for these regions, all the annuli can reduce the inlet wave energy factor not only at optimised wavenumber
$\mathcal {F}_{{ inlet}}=1$
because the annulus merely follows the motion of the waves without scattering them. There are some periodic peaks due to resonance in the inner domain, which is typically known as moonpool resonance (e.g. Newman Reference Newman2018b
). Similar results are found from Malenica et al. (Reference Malenica, Liang, Zheng and Korobkin2023). Since the simulation is based on the linear potential theory, the amplified wave energy is unrealistically high. In real situations, these waves are dissipated by nonlinear processes, such as viscosity and wave-breaking. Except for these regions, all the annuli can reduce the inlet wave energy factor not only at optimised wavenumber
![]() $k_0=1.0$
, but also at other frequencies. The optimisations for the wave shield achieve a greater reduction in the inlet wave energy factor compared with those for the cloak. In figure 6(b), the results of the cloak show dramatic reductions of the scattered-wave energy at
$k_0=1.0$
, but also at other frequencies. The optimisations for the wave shield achieve a greater reduction in the inlet wave energy factor compared with those for the cloak. In figure 6(b), the results of the cloak show dramatic reductions of the scattered-wave energy at
![]() $k_0=1.0$
. Apart from this wavenumber, the scattered-wave energy remains of a similar order in all cases. Although the scattered-wave energy for
$k_0=1.0$
. Apart from this wavenumber, the scattered-wave energy remains of a similar order in all cases. Although the scattered-wave energy for
![]() $L=16$
is lower than that for
$L=16$
is lower than that for
![]() $L=8$
at
$L=8$
at
![]() $k_0=1.0$
, the order of the magnitude relationships is not consistent at other frequencies.
$k_0=1.0$
, the order of the magnitude relationships is not consistent at other frequencies.
Finally, the wave drift force
![]() $\overline {F_x}$
acting on the annulus is shown in figure 7. The frequency responses of the wave drift force show similar behaviour to those of the scattered-wave energy as both are given by the scattering wave amplitude. As seen in figure 4(d), the wave shield scatters incident waves in front of the annulus at
$\overline {F_x}$
acting on the annulus is shown in figure 7. The frequency responses of the wave drift force show similar behaviour to those of the scattered-wave energy as both are given by the scattering wave amplitude. As seen in figure 4(d), the wave shield scatters incident waves in front of the annulus at
![]() $k_0=1.0$
. As a result, the large wave drift force acts on the annulus in the positive direction along the
$k_0=1.0$
. As a result, the large wave drift force acts on the annulus in the positive direction along the
![]() $x$
-axis due to the action and reaction law. However, the cloak does not scatter waves outside the annulus (see figure 5
d). Therefore, the wave drift force becomes almost zero at the target frequency as shown in figure 7.
$x$
-axis due to the action and reaction law. However, the cloak does not scatter waves outside the annulus (see figure 5
d). Therefore, the wave drift force becomes almost zero at the target frequency as shown in figure 7.
3.3. Further discussions
In this section, we further discuss the proposed annuli, including their performances, limitations and potentials for further development. Using the wave shield, the inlet wave energy can be reduced over a wide range of frequencies compared with that in an open sea. However, periodic wave amplifications were observed due to resonance. This may endanger marine structures in the annulus. Using dissipative plates (e.g. Xiao et al. Reference Xiao, Kou, Tao and Yang2016; Liu et al. Reference Liu, Chen, Zhang, Zhang and Liu2017) could be one of the solutions to reduce such a wave amplification.
When the annulus is designed to minimise the inlet wave energy factor, a high wave drift force acts on the annulus due to wave scattering. Therefore, a strong mooring system is necessary to maintain a position. Another annulus, designed to minimise both inlet wave energy factor and scattered-wave energy, achieved the cloaking and resultant low wave drift force at the optimised frequency.
Optimisations were carried out at the single frequency
![]() $k_0=1.0$
. For real applications, it is also demanded to perform over a range of frequencies. This could be achieved using a new objective function
$k_0=1.0$
. For real applications, it is also demanded to perform over a range of frequencies. This could be achieved using a new objective function
![]() $\int _{\Delta k}f(k){\rm d}k$
(e.g. Bobinski et al. Reference Bobinski, Maurel, Petitjeans and Pagneux2018). However, achieving the desired frequency band performance would require a larger outer radius and an increased number of plates. This may reduce practical applicability and increase optimisation time. Therefore, it is not addressed in this paper.
$\int _{\Delta k}f(k){\rm d}k$
(e.g. Bobinski et al. Reference Bobinski, Maurel, Petitjeans and Pagneux2018). However, achieving the desired frequency band performance would require a larger outer radius and an increased number of plates. This may reduce practical applicability and increase optimisation time. Therefore, it is not addressed in this paper.
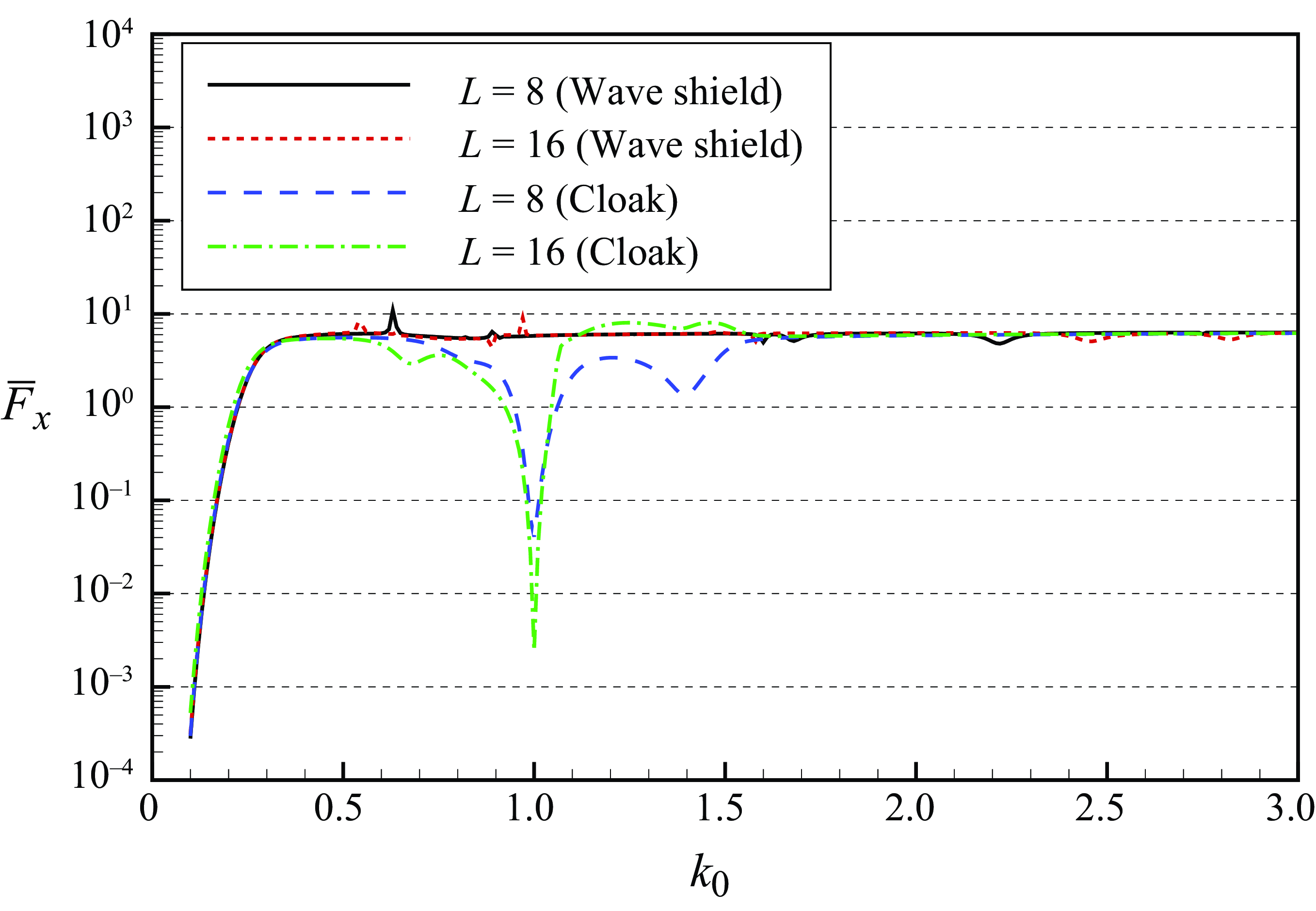
Figure 7. Wave drift force
![]() $\overline {F_x}$
acting on the annulus against wavenumber
$\overline {F_x}$
acting on the annulus against wavenumber
![]() $k_0$
(semi-log graph).
$k_0$
(semi-log graph).
We assumed a constant outer radius
![]() $(R=5.0)$
and mass (
$(R=5.0)$
and mass (
![]() $\gamma =0.05$
). When the outer radius is small, the performance is insufficient. However, increasing the outer radius does not necessarily improve the performance. At present,
$\gamma =0.05$
). When the outer radius is small, the performance is insufficient. However, increasing the outer radius does not necessarily improve the performance. At present,
![]() $R=5.0$
is the minimum size to achieve cloaking; however, for practical use, this annulus is too large compared with the inner cloaked domain. It is necessary to explore more practical sizes, such as by incorporating active control (e.g. Euvé et al. Reference Euvé, Pham, Petitjeans, Pagneux and Maurel2024). Heavy mass could give better performance. However, the density ratio to water is a limitation in design.
$R=5.0$
is the minimum size to achieve cloaking; however, for practical use, this annulus is too large compared with the inner cloaked domain. It is necessary to explore more practical sizes, such as by incorporating active control (e.g. Euvé et al. Reference Euvé, Pham, Petitjeans, Pagneux and Maurel2024). Heavy mass could give better performance. However, the density ratio to water is a limitation in design.
We developed the cloak based on the inlet-and-scattering-wave cancellation as the transformation-based cloak cannot be achieved for ocean waves. The coordinate transformation method is independent of wave frequency (Pendry et al. Reference Pendry, Schurig and Smith2006). In many cases, anisotropic medium properties are realised using homogenisation methods (e.g. Zareei & Alam Reference Zareei and Alam2015; Dupont et al. Reference Dupont, Guenneau, Kimmoun, Molin and Enoch2016), and the homogenised performance can be maintained over a frequency band as long as the microstructure is sufficiently smaller than the wavelength (Iida & Umazume Reference Iida and Umazume2020). Therefore, the transformation-based cloak is broadband. In addition, the physical interpretation is clear because this cloak is achieved by bending wave rays so that they bypass the inner cloaked domain. The present cloak, however, is currently limited to a single frequency as mentioned above. Moreover, the underlying mechanism of this cloak is not explicit because it involves a combination of various wave phenomena, such as reflection, refraction, trapping and superposition. Looking at supplementary movie 2, different behaviours are observed inside and outside
![]() $R=2.5$
: outside region (
$R=2.5$
: outside region (
![]() $2.5\leqslant R\leqslant 5.0$
), waves propagate as if they bypass the inner domain, which is similar to the transformation-based cloak; inside region (
$2.5\leqslant R\leqslant 5.0$
), waves propagate as if they bypass the inner domain, which is similar to the transformation-based cloak; inside region (
![]() $1.0\leqslant R\leqslant 2.5$
), there are nodes and anti-nodes as if standing waves, which is a similar result to the wave shield (see supplementary movie 1). We do not expect this structure to be the unique solution to achieve the cloak; however, it may be insightful to theoretically describe the ocean wave cloak in the future. In any case, similar to the cloaking factor (Porter & Newman Reference Porter and Newman2014), we expect the inlet wave energy factor to even contribute to studies of transformation-based cloaks and breakwaters as an important metric.
$1.0\leqslant R\leqslant 2.5$
), there are nodes and anti-nodes as if standing waves, which is a similar result to the wave shield (see supplementary movie 1). We do not expect this structure to be the unique solution to achieve the cloak; however, it may be insightful to theoretically describe the ocean wave cloak in the future. In any case, similar to the cloaking factor (Porter & Newman Reference Porter and Newman2014), we expect the inlet wave energy factor to even contribute to studies of transformation-based cloaks and breakwaters as an important metric.
We only demonstrated the potential of the wave shield and the cloak through numerical simulations based on the thin-plate assumption. Since this model assumes that the annulus only moves vertically in contact with the water surface, the annulus does not move in any other direction. However, in reality, motions in other modes (such as surge and sway) would be also induced. Therefore, a fully three-dimensional simulation is necessary for a detailed analysis. In addition, model experiments are also demanded to verify its feasibility. Although we assumed a closed annulus to simplify the problem, an opening is necessary for access to the inner domain. The influence of such an opening should also be investigated.
4. Conclusion
We presented the floating annuli that are used as a wave shield and a cloak. The annulus consists of
![]() $L$
horizontal concentric annular plates, and each plate is elastic and thin. Based on the linear potential flow theory, the inlet wave energy factor is defined to estimate the calmness of the free surface within the inner domain of the annulus. From the sensitivity studies of flexural rigidities to the inlet wave energy factor and scattered-wave energy in the case of two plates, we found that minimising them is in trade-off. The flexural rigidity of each plate was designed to achieve the wave shield and the cloak, respectively. The numerical simulations demonstrated that the calm free surfaces are created within the inner domains of the optimised annuli. In addition, the cloak successfully suppressed the outgoing scattering waves, and the cloaking condition was accomplished. It was also shown that the wave drift force acting on the cloak can be almost zero. Since the annuli are axisymmetric, the performances are omnidirectional. These annuli could protect marine structures from ocean waves. We believe this would expand the possibility of ocean space utilisation.
$L$
horizontal concentric annular plates, and each plate is elastic and thin. Based on the linear potential flow theory, the inlet wave energy factor is defined to estimate the calmness of the free surface within the inner domain of the annulus. From the sensitivity studies of flexural rigidities to the inlet wave energy factor and scattered-wave energy in the case of two plates, we found that minimising them is in trade-off. The flexural rigidity of each plate was designed to achieve the wave shield and the cloak, respectively. The numerical simulations demonstrated that the calm free surfaces are created within the inner domains of the optimised annuli. In addition, the cloak successfully suppressed the outgoing scattering waves, and the cloaking condition was accomplished. It was also shown that the wave drift force acting on the cloak can be almost zero. Since the annuli are axisymmetric, the performances are omnidirectional. These annuli could protect marine structures from ocean waves. We believe this would expand the possibility of ocean space utilisation.
Supplementary movies
Supplementary movies are available at https://doi.org/10.1017/jfm.2025.106.
Funding
This work was supported by JSPS KAKENHI Grant Number JP23K13504.
Declaration of interests
The author reports no conflict of interest.
Data availability statement
Data will be made available on request.
Appendix A. Boundary conditions
The coefficients
![]() $a_{mn}$
,
$a_{mn}$
,
![]() $b_{mn}^{(\ell)}$
,
$b_{mn}^{(\ell)}$
,
![]() $c_{mn}^{(\ell)}$
and
$c_{mn}^{(\ell)}$
and
![]() $d_{mn}$
in (2.5), (2.6) and (2.7) are unknown. By truncating the infinite series of azimuthal and radial modes to orders
$d_{mn}$
in (2.5), (2.6) and (2.7) are unknown. By truncating the infinite series of azimuthal and radial modes to orders
![]() $M$
and
$M$
and
![]() $N$
, the number of unknown coefficients becomes
$N$
, the number of unknown coefficients becomes
![]() $2(N+1)+2L(N+3)$
. To solve these unknown coefficients, the same number of boundary conditions are required. Here, we consider the following boundary conditions.
$2(N+1)+2L(N+3)$
. To solve these unknown coefficients, the same number of boundary conditions are required. Here, we consider the following boundary conditions.
-
(i) Matching conditions of the velocity potential between the outer free surface and the plate (
 $N+1$
equations).
$N+1$
equations). -
(ii) Matching conditions of the radial derivative of the velocity potential between the outer free surface and the plate (
 $N+1$
equations).
$N+1$
equations). -
(iii) Matching conditions of the velocity potential between adjacent plates (
 $(L-1)$
$(L-1)$
 $(N+1)$
equations).
$(N+1)$
equations). -
(iv) Matching conditions of the radial derivative of the velocity potential between adjacent plates (
 $(L-1)(N+1)$
equations).
$(L-1)(N+1)$
equations). -
(v) Matching conditions of the wave elevation between adjacent plates (
 $L-1$
equations).
$L-1$
equations). -
(vi) Matching conditions of the radial derivative of the wave elevation between adjacent plates (
 $L-1$
equations).
$L-1$
equations). -
(vii) Matching conditions of the bending moment between adjacent plates (
 $L-1$
equations).
$L-1$
equations). -
(viii) Matching conditions of the shear force between adjacent plates (
 $L-1$
equations).
$L-1$
equations). -
(ix) Free-free beam conditions; zero bending moment and shear force (four equations).
-
(x) Matching conditions of the velocity potential between the inner free surface and the plate (
 $N+1$
equations).
$N+1$
equations). -
(xi) Matching conditions of the radial derivative of the velocity potential between the inner free surface and the plate (
 $N+1$
equations).
$N+1$
equations).
The boundary conditions (i) to (ix) are the same as those used by Iida et al. (Reference Iida, Zareei and Alam2023), and details of equations are shown in the reference. Here, we only show the new boundary conditions (x) and (xi) as follows.
-
(i) Matching conditions of the velocity potential between the inner free surface and the plate (
 $N+1$
equations):(A1)
$N+1$
equations):(A1) \begin{align} d_{m0}\textrm { J}_m(k_0)A_{0q } & + \sum \limits _{n=1}^{N} d_{mn} \textrm { I}_m(k_n )A_{nq } = b_{m0}^{(\ell)}\textrm { J}_m\big(\mu ^{(\ell)}_0\big)B_{0q }^{(\ell)}+ \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm}b^{(\ell)}_{mn} \textrm { I}_m\big(\mu ^{(\ell)}_n \big) B_{nq }^{(\ell)} \nonumber\\[-4pt] & +c_{m0}^{(\ell)}\textrm { H}_m^{(\ell)}\big(\mu ^{(\ell)}_0 \big)B_{0q }^{(\ell)} + \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm} c^{(\ell)}_{mn} \textrm { K}_m\big(\mu ^{(\ell)}_n \big) B_{nq }^{(\ell)}. \end{align}
\begin{align} d_{m0}\textrm { J}_m(k_0)A_{0q } & + \sum \limits _{n=1}^{N} d_{mn} \textrm { I}_m(k_n )A_{nq } = b_{m0}^{(\ell)}\textrm { J}_m\big(\mu ^{(\ell)}_0\big)B_{0q }^{(\ell)}+ \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm}b^{(\ell)}_{mn} \textrm { I}_m\big(\mu ^{(\ell)}_n \big) B_{nq }^{(\ell)} \nonumber\\[-4pt] & +c_{m0}^{(\ell)}\textrm { H}_m^{(\ell)}\big(\mu ^{(\ell)}_0 \big)B_{0q }^{(\ell)} + \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm} c^{(\ell)}_{mn} \textrm { K}_m\big(\mu ^{(\ell)}_n \big) B_{nq }^{(\ell)}. \end{align}
-
(ii) Matching conditions of the radial derivative of the velocity potential between the inner free surface and the plate (
 $N+1$
equations):(A2)
$N+1$
equations):(A2) \begin{eqnarray} \begin{array}{l} d_{m0}k_0\textrm { J}^{\prime}_m (k_0)A_{0q }+ \sum \limits _{n=1}^{N} d_{mn}k_n \textrm { I}^{\prime}_m(k_n )A_{nq } \nonumber\\ \quad = b_{m0}^{(\ell)}\mu ^{(\ell)}_0\textrm { J}^{\prime}_m\big(\mu ^{(\ell)}_0\big)B_{0q }^{(\ell)}+ \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm} b^{(\ell)}_{mn}\mu ^{(\ell)}_n \textrm { I}^{\prime}_m(\mu ^{(\ell)}_n) B_{nq }^{(\ell)} \nonumber\\ \quad +{\kern1pt}c_{m0}^{(\ell)}\mu ^{(\ell)}_0\textrm { H}_m^{(\ell)^{\prime}}\big(\mu ^{(\ell)}_0\big)B_{0q }^{(\ell)} + \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm}c^{(\ell)}_{mn}\mu ^{(\ell)}_n \textrm { K}^{\prime}_m(\mu ^{(\ell)}_n) B_{nq }^{(\ell)}. \end{array} \nonumber\\[-21pt] \end{eqnarray}
\begin{eqnarray} \begin{array}{l} d_{m0}k_0\textrm { J}^{\prime}_m (k_0)A_{0q }+ \sum \limits _{n=1}^{N} d_{mn}k_n \textrm { I}^{\prime}_m(k_n )A_{nq } \nonumber\\ \quad = b_{m0}^{(\ell)}\mu ^{(\ell)}_0\textrm { J}^{\prime}_m\big(\mu ^{(\ell)}_0\big)B_{0q }^{(\ell)}+ \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm} b^{(\ell)}_{mn}\mu ^{(\ell)}_n \textrm { I}^{\prime}_m(\mu ^{(\ell)}_n) B_{nq }^{(\ell)} \nonumber\\ \quad +{\kern1pt}c_{m0}^{(\ell)}\mu ^{(\ell)}_0\textrm { H}_m^{(\ell)^{\prime}}\big(\mu ^{(\ell)}_0\big)B_{0q }^{(\ell)} + \hspace {-4mm} \sum \limits _{n=-2,n\ne 0}^{N}\hspace {-3mm}c^{(\ell)}_{mn}\mu ^{(\ell)}_n \textrm { K}^{\prime}_m(\mu ^{(\ell)}_n) B_{nq }^{(\ell)}. \end{array} \nonumber\\[-21pt] \end{eqnarray}
Note that
![]() $q =0,1,\ldots ,N$
and
$q =0,1,\ldots ,N$
and
![]() $A_{nq }$
and
$A_{nq }$
and
![]() $B_{nq }^{(\ell)}$
are given based on the eigenvalue matching method as
$B_{nq }^{(\ell)}$
are given based on the eigenvalue matching method as






































