Short Communications
The coupon-collector's problem revisited
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 513-518
-
- Article
- Export citation
Research Papers
On the entropy for semi-Markov processes
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1060-1068
-
- Article
- Export citation
Statistical properties of a system reliability estimator using the Littlewood software reliability model
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 766-778
-
- Article
- Export citation
Short Communications
The asymptotic joint normality of the numbers of upper records, lower records and inversions in a random sequence
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 257-263
-
- Article
- Export citation
SIR epidemics on a Bernoulli random graph
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 779-782
-
- Article
- Export citation
Research Papers
On moments and tail behaviors of storage processes
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1069-1086
-
- Article
- Export citation
Short Communications
Average cost under the PMλ, τ policy in a finite dam with compound Poisson inputs
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 519-526
-
- Article
- Export citation
A further extension of the generalized burn-in model
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 264-270
-
- Article
- Export citation
Research Papers
The classification of matrix GI/M/1-type Markov chains with a tree structure and its applications to queueing
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1087-1102
-
- Article
- Export citation
Short Communications
Perpetual American put options in a level-dependent volatility model
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 783-789
-
- Article
- Export citation
Front matter
JPR volume 40 issue 2 Cover and Front matter
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. f1-f4
-
- Article
-
- You have access
- Export citation
Letter to the Editor
An intuitive insight into a result of Cowan
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 271-272
-
- Article
-
- You have access
- Export citation
Back matter
JPR volume 40 issue 2 Cover and Back matter
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. b1-b2
-
- Article
-
- You have access
- Export citation
Front matter
JPR volume 40 issue 1 Cover and Front matter
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. f1-f6
-
- Article
-
- You have access
- Export citation
Research Papers
On the stochastic domination for batch-arrival, batch-service and assemble-transfer queueing networks
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1103-1120
-
- Article
- Export citation
Short Communications
Mixed distributions in Sattolo's algorithm for cyclic permutations via randomization and derandomization
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 790-796
-
- Article
- Export citation
On the moment problem for random sums
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 797-802
-
- Article
- Export citation
Research Papers
A lower bound using Hamilton-type circuits and its applications
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1121-1132
-
- Article
- Export citation
Back matter
JPR volume 40 issue 1 Cover and Back matter
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. b1-b5
-
- Article
-
- You have access
- Export citation
Research Papers
Long-memory continuous-time correlation models
- Part of:
-
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1133-1146
-
- Article
- Export citation

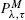 policy in a finite dam in which the input of water is formed by a compound Poisson process and the rate of water release is changed instantaneously from
policy in a finite dam in which the input of water is formed by a compound Poisson process and the rate of water release is changed instantaneously from