Let X be a separable infinite-dimensional Banach space, and T a bounded linear operator on X; T is hypercyclic if there is a vector x in X with dense orbit under the action of T. For a fixed ε∈(0,1), we say that T is ε-hypercyclic if there exists a vector x in X such that for every non-zero vector y∈X there exists an integer n with . The main result of this paper is a construction of a bounded linear operator T on the Banach space ℓ1 which is ε-hypercyclic without being hypercyclic. This answers a question from V. Müller [Three problems, Mini-Workshop: Hypercyclicity and linear chaos, organized by T. Bermudez, G. Godefroy, K.-G. Grosse-Erdmann and A. Peris. Oberwolfach Rep.3 (2006), 2227–2276].

Let (X,T) be a topologically transitive dynamical system. We show that if there is a subsystem (Y,T) of (X,T) such that (X×Y,T×T) is transitive, then (X,T) is strongly chaotic in the sense of Li and Yorke. We then show that many of the known sufficient conditions in the literature, as well as a few new results, are corollaries of this statement. In fact, the kind of chaotic behavior we deduce in these results is a much stronger variant of Li–Yorke chaos which we call uniform chaos. For minimal systems we show, among other results, that uniform chaos is preserved by extensions and that a minimal system which is not uniformly chaotic is PI.

We offer a proof of the following non-conventional ergodic theorem: If Ti:ℤr↷(X,Σ,μ) for i=1,2,…,d are commuting probability-preserving ℤr-actions, (IN)N≥1 is a Følner sequence of subsets of ℤr, (aN)N≥1 is a base-point sequence in ℤr and f1,f2,…,fd∈L∞(μ) then the non-conventional ergodic averages 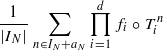converge to some limit in L2(μ) that does not depend on the choice of (aN)N≥1 or (IN)N≥1. The leading case of this result, with r=1 and the standard sequence of averaging sets, was first proved by Tao, following earlier analyses of various more special cases and related results by Conze and Lesigne, Furstenberg and Weiss, Zhang, Host and Kra, Frantzikinakis and Kra and Ziegler. While Tao’s proof rests on a conversion to a finitary problem, we invoke only techniques from classical ergodic theory, so giving a new proof of his result.

In Adler et al [Convex dynamics and applications. Ergod. Th. & Dynam. Sys.25 (2005), 321–352] certain piecewise linear maps were defined in terms of a convex polytope. When the convex polytope is a simplex, the resulting map has a dual nature. On one hand it is defined on ℝN and acts as a piecewise translation. On the other it can be viewed as a translation on the N-torus. What relates its two roles? A natural answer would be that there exists an invariant fundamental set into which all orbits under piecewise translation eventually enter. We prove this for N=1 and for acute and right triangles—i.e. non-obtuse triangles. We leave open the case of obtuse triangles and higher-dimensional simplices. Another question not treated is the connectivity of the invariant fundamental sets which arise.

We give two new proofs that the Sinai–Ruelle–Bowen (SRB) measure t↦μt of a C2 path ft of unimodal piecewise expanding C3 maps is differentiable at 0 if ft is tangent to the topological class of f0. The arguments are more conceptual than the original proof of Baladi and Smania [Linear response formula for piecewise expanding unimodal maps. Nonlinearity21 (2008), 677–711], but require proving Hölder continuity of the infinitesimal conjugacy α (a new result, of independent interest) and using spaces of bounded p-variation. The first new proof gives differentiability of higher order of ∫ ψ dμt if ft is smooth enough and stays in the topological class of f0 and if ψ is smooth enough (a new result). In addition, this proof does not require any information on the decomposition of the SRB measure into regular and singular terms, making it potentially amenable to extensions to higher dimensions. The second new proof allows us to recover the linear response formula (i.e. the formula for the derivative at 0) obtained by Baladi and Smania, by an argument more conceptual than the ‘brute force’ cancellation mechanism used by Baladi and Smania.

In this article we study conditions to be a continuous or a measurable eigenvalue of finite rank minimal Cantor systems, that is, systems given by an ordered Bratteli diagram with a bounded number of vertices per level. We prove that continuous eigenvalues always come from the stable subspace associated with the incidence matrices of the Bratteli diagram and we study rationally independent generators of the additive group of continuous eigenvalues. Given an ergodic probability measure, we provide a general necessary condition for there to be a measurable eigenvalue. Then, we consider two families of examples, a first one to illustrate that measurable eigenvalues do not need to come from the stable space. Finally, we study Toeplitz-type Cantor minimal systems of finite rank. We recover classical results in the continuous case and we prove that measurable eigenvalues are always rational but not necessarily continuous.

In this article we prove that given a self-similar interval exchange transformation T(λ,π), whose associated matrix verifies a quite general algebraic condition, there exists an affine interval exchange transformation with wandering intervals that is semi-conjugated to it. That is, in this context the existence of Denjoy counterexamples occurs very often, generalizing the result of Cobo [Piece-wise affine maps conjugate to interval exchanges. Ergod. Th. & Dynam. Sys.22 (2002), 375–407].

Anderson and Putnam showed that the cohomology of a substitution tiling space may be computed by collaring tiles to obtain a substitution which ‘forces its border’. One can then represent the tiling space as an inverse limit of an inflation and substitution map on a cellular complex formed from the collared tiles; the cohomology of the tiling space is computed as the direct limit of the homomorphism induced by inflation and substitution on the cohomology of the complex. In earlier work, Barge and Diamond described a modification of the Anderson–Putnam complex on collared tiles for one-dimensional substitution tiling spaces that allows for easier computation and provides a means of identifying certain special features of the tiling space with particular elements of the cohomology. In this paper, we extend this modified construction to higher dimensions. We also examine the action of the rotation group on cohomology and compute the cohomology of the pinwheel tiling space.

We introduce a class of two-dimensional piecewise isometries on the plane that we refer to as cone exchange transformations (CETs). These are generalizations of interval exchange transformations (IETs) to 2D unbounded domains. We show for a typical CET that boundedness of orbits is determined by ergodic properties of an associated IET and a quantity we refer to as the ‘flux at infinity’. In particular we show, under an assumption of unique ergodicity of the associated IET, that a positive flux at infinity implies unboundedness of almost all orbits outside some bounded region, while a negative flux at infinity implies boundedness of all orbits. We also discuss some examples of CETs for which the flux is zero and/or we do not have unique ergodicity of the associated IET; in these cases (which are of great interest from the point of view of applications such as dual billiards) it remains an outstanding problem to find computable necessary and sufficient conditions for boundedness of orbits.

The Anosov flows on compact manifolds M satisfy the following property: if p,q are points such that for all positive ϵ there is a trajectory from a point ϵ-close to p to a point ϵ-close to q, then there is a point whose α-limit set is that of p and whose ω-limit set is that of q. Here we give a version of this property for sectional-Anosov flows, namely, vector fields inwardly transverse to the boundary whose maximal invariant set is sectional-hyperbolic. Indeed, if in addition M is three-dimensional and p has non-singular α-limit set, then there is a point whose α-limit set is that of p and whose ω-limit set is either a singularity or that of q.

A set Λ is internally chain transitive if for any x,y∈Λ and ϵ>0 there is an ϵ-pseudo-orbit in Λ between x and y. In this paper we characterize all ω-limit sets in shifts of finite type by showing that, if Λ is a closed, strongly shift-invariant subset of a shift of finite type, X, then there is a point z∈X with ω(z)=Λ if and only if Λ is internally chain transitive. It follows immediately that any closed, strongly shift-invariant, internally chain transitive subset of a shift space over some alphabet ℬ is the ω-limit set of some point in the full shift space over ℬ. We use similar techniques to prove that, for a tent map f, a closed, strongly f-invariant, internally chain transitive subset of the interval is the ω-limit set of a point provided it does not contain the image of the critical point. We give an example of a sofic shift space Z𝒢 (a factor of a shift space of finite type) that is not of finite type that has an internally chain transitive subset that is not the ω-limit set of any point in Z𝒢.

We study dynamical systems acting on the path space of a stationary (non-simple) Bratteli diagram. For such systems we give an explicit description of all ergodic probability measures that are invariant with respect to the tail equivalence relation (or the Vershik map); these measures are completely described by the incidence matrix of the Bratteli diagram. Since such diagrams correspond to substitution dynamical systems, our description provides an algorithm for finding invariant probability measures for aperiodic non-minimal substitution systems. Several corollaries of these results are obtained. In particular, we show that the invariant measures are not mixing and give a criterion for a complex number to be an eigenvalue for the Vershik map.

We study two classes of dynamical systems with holes: expanding maps of the interval and Collet–Eckmann maps with singularities. In both cases, we prove that there is a natural absolutely continuous conditionally invariant measure μ (a.c.c.i.m.) with the physical property that strictly positive Hölder continuous functions converge to the density of μ under the renormalized dynamics of the system. In addition, we construct an invariant measure ν, supported on the Cantor set of points that never escape from the system, that is ergodic and enjoys exponential decay of correlations for Hölder observables. We show that ν satisfies an equilibrium principle which implies that the escape rate formula, familiar to the thermodynamic formalism, holds outside the usual setting. In particular, it holds for Collet–Eckmann maps with holes, which are not uniformly hyperbolic and do not admit a finite Markov partition. We use a general framework of Young towers with holes and first prove results about the a.c.c.i.m. and the invariant measure on the tower. Then we show how to transfer results to the original dynamical system. This approach can be expected to generalize to other dynamical systems than the two above classes.

In this paper we introduce a new functional invariant of discrete time dynamical systems—the so-called t-entropy. The main result is that this t-entropy is the Legendre dual functional to the logarithm of the spectral radius of the weighted shift operator on L1(X,m) generated by the dynamical system. This result is called the variational principle and is similar to the classical variational principle for the topological pressure.

This paper introduces Markov chains and processes over non-abelian free groups and semigroups. We prove a formula for the f-invariant of a Markov chain over a free group in terms of transition matrices that parallels the classical formula for the entropy a Markov chain. Applications include free group analogues of the Abramov–Rohlin formula for skew-product actions and Yuzvinskii’s addition formula for algebraic actions.

Krengel characterized weakly mixing actions (X,T) as those measure-preserving actions having a dense set of partitions of X with infinitely many jointly independent images under iterates of T. Using the tools developed in later papers—one by del Junco, Reinhold and Weiss, another by del Junco and Begun—we prove analogues of these results for weakly mixing random dynamical systems (in other words, relatively weakly mixing systems).

In this paper, we analyse the dynamics encoded in the spectral sequence (Er,dr) associated with certain Conley theory connection maps in the presence of an ‘action’ type filtration. More specifically, we present an algorithm for finding a chain complex C and its differential; the method uses a connection matrix Δ to provide a system that spans Er in terms of the original basis of C and to identify all of the differentials drp:Erp→Erp−r. In exploring the dynamical implications of a non-zero differential, we prove the existence of a path that joins the singularities generating E0p and E0p−r in the case where a direct connection by a flow line does not exist. This path is made up of juxtaposed orbits of the flow and of the reverse flow, and proves to be important in some applications.

The purpose of the present paper is to provide a link between skew-product systems and linear dynamics. In particular, we give a criterion for skew-products of linear operators to be topologically transitive. This is then applied to certain families of linear operators including scalar multiples of the backward shift, backward unilateral weighted shifts, composition, translation and differentiation operators. We also prove the existence of common hypercyclic vectors for certain families of skew-product systems.

We consider linear iterated function systems (IFS) with a constant contraction ratio in the plane for which the ‘overlap set’ 𝒪 is finite, and which are ‘invertible’ on the attractor A, in the sense that there is a continuous surjection q:A→A whose inverse branches are the contractions of the IFS. The overlap set is the critical set in the sense that q is not a local homeomorphism precisely at 𝒪. We suppose also that there is a rational function p with the Julia set J such that (A,q) and (J,p) are conjugate. We prove that if A has bounded turning and p has no parabolic cycles, then the conjugacy is quasisymmetric. This result is applied to some specific examples including an uncountable family. Our main focus is on the family of IFS {λz,λz+1} where λ is a complex parameter in the unit disk, such that its attractor Aλ is a dendrite, which happens whenever 𝒪 is a singleton. C. Bandt observed that a simple modification of such an IFS (without changing the attractor) is invertible and gives rise to a quadratic-like map qλ on Aλ. If the IFS is post-critically finite, then a result of A. Kameyama shows that there is a quadratic map pc (z)=z2 +c, with the Julia set Jc such that (Aλ,qλ) and (Jc,pc) are conjugate. We prove that this conjugacy is quasisymmetric and obtain partial results in the general (not post-critically finite) case.

The celebrated Livšic theorem [A. N. Livšic, Certain properties of the homology of Y-systems, Mat. Zametki 10 (1971), 555–564; A. N. Livšic, Cohomology of dynamical systems, Izv. Akad. Nauk SSSR Ser. Mat. 36 (1972), 1296–1320] states that given a manifold M, a Lie group G, a transitive Anosov diffeomorphism f on M and a Hölder function η:M↦G whose range is sufficiently close to the identity, it is sufficient for the existence of ϕ:M↦G satisfying η(x)=ϕ(f(x))ϕ(x)−1 that a condition—obviously necessary—on the cocycle generated by η restricted to periodic orbits is satisfied. In this paper we present a new proof of the main result. These methods allow us to treat cocycles taking values in the group of diffeomorphisms of a compact manifold. This has applications to rigidity theory. The localization procedure we develop can be applied to obtain some new results on the existence of conformal structures for Anosov systems.