1 results
The large-time asymptotic solution of the mKdV equation
-
- Journal:
- European Journal of Applied Mathematics / Volume 26 / Issue 6 / December 2015
- Published online by Cambridge University Press:
- 04 June 2015, pp. 931-943
-
- Article
- Export citation
-
In this paper, an initial-value problem for the modified Korteweg-de Vries (mKdV) equation is addressed. Previous numerical simulations of the solution of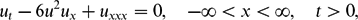
where x and t represent dimensionless distance and time respectively, have considered the evolution when the initial data is given by
\[
u_{t} - 6u^{2} u_{x}+u_{xxx}=0, \quad -\infty<x<\infty, \quad t>0,
\]
for C constant. These computations suggest that kink and soliton structures develop from this initial profile and here the method of matched asymptotic coordinate expansions is used to obtain the complete large-time structure of the solution in the particular case C = 1/3. The technique is able to confirm some of the numerical predictions, but also forms a basis that could be easily extended to account for other initial conditions and other physically significant equations. Not only can the details of the relevant long-time structure be determined but rates of convergence of the solution of the initial-value problem be predicted.
\[
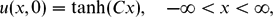u(x,0)=\tanh ( Cx ), \quad -\infty<x<\infty,
\]
