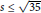1 results
A Note on Quaternionic Hyperbolic Ideal Triangle Groups
-
- Journal:
- Canadian Mathematical Bulletin / Volume 59 / Issue 2 / 01 June 2016
- Published online by Cambridge University Press:
- 20 November 2018, pp. 244-257
- Print publication:
- 01 June 2016
-
- Article
-
- You have access
- Export citation