1 results
CONVOLUTION OPERATORS AND HOMOMORPHISMS OF LOCALLY COMPACT GROUPS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 84 / Issue 3 / June 2008
- Published online by Cambridge University Press:
- 01 June 2008, pp. 329-344
- Print publication:
- June 2008
-
- Article
-
- You have access
- Export citation
-
Let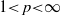
, let G and H be locally compact groups and let ω be a continuous homomorphism of G into H. We prove, if G is amenable, the existence of a linear contraction of the Banach algebra CVp(G) of the p-convolution operators on G into CVp(H) which extends the usual definition of the image of a bounded measure by ω. We also discuss the uniqueness of this linear contraction onto important subalgebras of CVp(G). Even if G and H are abelian, we obtain new results. Let Gd denote the group G provided with a discrete topology. As a corollary, we obtain, for every discrete measure, 
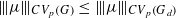, for Gd amenable. For arbitrary G, we also obtain 
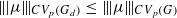. These inequalities were already known for p=2 . The proofs presented in this paper avoid the use of the Hilbertian techniques which are not applicable to 
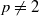. Finally, for Gd amenable, we construct a natural map of CVp (G) into CVp (Gd) .
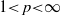, let 
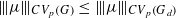, for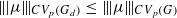 
. These inequalities were already known for 
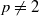. Finally, for 