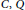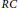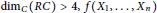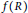Let R$R$ be a prime ring with extended centroid \text{C,Q}$\text{C,Q}$ maximal right ring of quotients of R$R$, RC$RC$ central closure of R$R$ such that {{\dim}_{C}}(RC)>4,f({{X}_{1}},...,{{X}_{n}})${{\dim}_{C}}(RC)>4,f({{X}_{1}},...,{{X}_{n}})$ a multilinear polynomial over C$C$ that is not central-valued on R$R$, and f(R)$f(R)$ the set of all evaluations of the multilinear polynomial 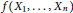f({{X}_{1}},...,{{X}_{n}})$f({{X}_{1}},...,{{X}_{n}})$ in R$R$. Suppose that 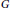G$G$ is a nonzero generalized derivation of R$R$ such that 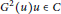{{G}^{2}}(u)u\in C${{G}^{2}}(u)u\in C$ for all 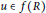u\in f(R)$u\in f(R)$. Then one of the following conditions holds:

• (i) there exists a\in \text{Q}$a\in \text{Q}$ such that {{a}^{2}}=0${{a}^{2}}=0$ and either G(x)=ax$G(x)=ax$ for all x\in R$x\in R$or G(x)=xa$G(x)=xa$ for all x\in R$x\in R$;

• (ii) there exists a\in \text{Q}$a\in \text{Q}$ such that 0\ne {{a}^{2}}\in C$0\ne {{a}^{2}}\in C$ and either G(x)=ax$G(x)=ax$ for all x\in R$x\in R$ or G(x)=xa$G(x)=xa$ for all x\in R$x\in R$ and f{{({{X}_{1}},...,{{X}_{n}})}^{2}}$f{{({{X}_{1}},...,{{X}_{n}})}^{2}}$is central-valued on R$R$;

• (iii) char (R)=2$(R)=2$ and one of the following holds:

• (a) there exist a,b,\in \text{Q}$a,b,\in \text{Q}$ such that 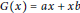G(x)=ax+xb$G(x)=ax+xb$ for all x\in R$x\in R$ and {{a}^{2}}={{b}^{2}}\in C${{a}^{2}}={{b}^{2}}\in C$;

• (b) there exist a,b,\in \text{Q}$a,b,\in \text{Q}$ such that 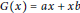G(x)=ax+xb$G(x)=ax+xb$ for all 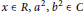x\in R,\,{{a}^{2}},{{b}^{2}}\in C$x\in R,\,{{a}^{2}},{{b}^{2}}\in C$ and 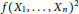f{{({{X}_{1}},...,{{X}_{n}})}^{2}}$f{{({{X}_{1}},...,{{X}_{n}})}^{2}}$ is central-valued on R$R$;

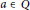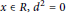• (c) there exist a\in \text{Q}$a\in \text{Q}$ and an X$X$-outer derivation d$d$ of R$R$ such that G(x)=ax+d(x)$G(x)=ax+d(x)$ for all x\in R,{{d}^{2}}=0$x\in R,{{d}^{2}}=0$ and 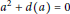{{a}^{2}}+d(a)=0${{a}^{2}}+d(a)=0$;

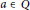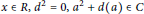• (d) there exist a\in \text{Q}$a\in \text{Q}$ and an X$X$-outer derivation d$d$ of R$R$ such that G(x)=ax+d(x)$G(x)=ax+d(x)$ for all x\in R,\,{{d}^{2}}=0,\,{{a}^{2}}+d(a)\in C$x\in R,\,{{d}^{2}}=0,\,{{a}^{2}}+d(a)\in C$ and 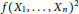f{{({{X}_{1}},...,{{X}_{n}})}^{2}}$f{{({{X}_{1}},...,{{X}_{n}})}^{2}}$ is central-valued on R$R$.

Moreover, we characterize the form of nonzero generalized derivations 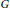G$G$ of R$R$ satisfying 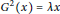{{G}^{2}}(x)=\lambda x${{G}^{2}}(x)=\lambda x$ for all x\in R$x\in R$, where 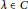\lambda \in C$\lambda \in C$.