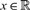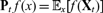Matryoshka dolls, the traditional Russian nesting figurines, are known worldwide for each doll’s encapsulation of a sequence of smaller dolls. In this paper, we exploit the structure of a new sequence of nested matrices we call matryoshkan matrices in order to compute the moments of the one-dimensional polynomial processes, a large class of Markov processes. We characterize the salient properties of matryoshkan matrices that allow us to compute these moments in closed form at a specific time without computing the entire path of the process. This simplifies the computation of the polynomial process moments significantly. Through our method, we derive explicit expressions for both transient and steady-state moments of this class of Markov processes. We demonstrate the applicability of this method through explicit examples such as shot noise processes, growth–collapse processes, ephemerally self-exciting processes, and affine stochastic differential equations from the finance literature. We also show that we can derive explicit expressions for the self-exciting Hawkes process, for which finding closed-form moment expressions has been an open problem since their introduction in 1971. In general, our techniques can be used for any Markov process for which the infinitesimal generator of an arbitrary polynomial is itself a polynomial of equal or lower order.

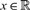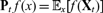Let X = (Xt)t≥0 be a stochastic process issued from $x \in \mathbb{R}$ that admits a marginal stationary measure v, i.e. vPtf = vf for all t ≥ 0, where $\textbf{P}_t\,f(x)= \mathbb{E}_x[f(\textbf{X}_t)]$ . In this paper, we introduce the (resp. biorthogonal) spectral projections correlation functions which are expressed in terms of projections.” Also, update first published online date, if available. into the eigenspaces of Pt (resp. and of its adjoint in the weighted Hilbert space L2 (v)). We obtain closed-form expressions involving eigenvalues, the condition number and/or the angle between the projections in the following different situations: when X = X with X = (Xt)t ≥ 0 being a Markov process, X is the subordination of X in the sense of Bochner, and X is a non-Markovian process which is obtained by time-changing X with an inverse of a subordinator. It turns out that these spectral projections correlation functions have different expressions with respect to these classes of processes which enables to identify substantial and deep properties about their dynamics. This interesting fact can be used to design original statistical tests to make inferences, for example, about the path properties of the process (presence of jumps), distance from symmetry (self-adjoint or non-self-adjoint) and short-to-long-range dependence. To reveal the usefulness of our results, we apply them to a class of non-self-adjoint Markov semigroups studied in Patie and Savov (to appear, Mem. Amer. Math. Soc., 179p), and then time-change by subordinators and their inverses.