We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
In this paper, the dimension function of a self-affine generalized scaling set associated with an 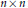n\,\times \,n integral expansive dilation 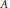A is studied. More specifically, we consider the dimension function of an 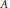A-dilation generalized scaling set 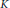K assuming that 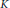K is a self-affine tile satisfying BK\,=\,\left( K\,+\,{{d}_{1}} \right)\,\cup \,\left( K\,+\,{{d}_{2}} \right), where 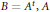B\,=\,{{A}^{t}},\,A is an 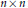n\,\times \,n integral expansive matrix with 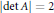\left| \det \,A \right|\,=\,2, and 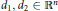{{d}_{1}},\,{{d}_{2}}\,\in \,{{\mathbb{R}}^{n}}. We show that the dimension function of 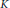K must be constant if either 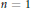n\,=1 or 2 or one of the digits is 0, and that it is bounded by 2\left| K \right| for any n.
Email your librarian or administrator to recommend adding this to your organisation's collection.
