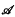The optimality of dualities on a quasivariety , generated by a finite algebra 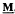, has been introduced by Davey and Priestley in the 1990s. Since every optimal duality is determined by a transversal of a certain family of subsets of Ω, where Ω is a given set of relations yielding a duality on , an understanding of the structures of these subsets—known as globally minimal failsets—was required. A complete description of globally minimal failsets which do not contain partial endomorphisms has recently been given by the author and H. A. Priestley. Here we are concerned with globally minimal failsets containing endomorphisms. We aim to explain what seems to be a pattern in the way endomorphisms belong to these failsets. This paper also gives a complete description of globally minimal failsets whose minimal elements are automorphisms, when is a subdirectly irreducible lattice-structured algebra.

Consider the quasi-variety generated by a finite algebra 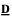and assume that 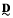yields a natural duality on 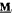based on which is optimal modulo endomorphisms. We shoe that, provided satisfies certain minimality conditions, we can transfer this duality to a natural duality on based on , which is also optimal modulo endormorphisms, for any finite algebra in that has a subalgebra isomorphic to .