2 results
STRUCTURE THEOREM FOR
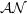 $\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathcal{AN}$-OPERATORS
$\def \xmlpi #1{}\def \mathsfbi #1{\boldsymbol {\mathsf {#1}}}\let \le =\leqslant \let \leq =\leqslant \let \ge =\geqslant \let \geq =\geqslant \def \Pr {\mathit {Pr}}\def \Fr {\mathit {Fr}}\def \Rey {\mathit {Re}}\mathcal{AN}$-OPERATORS
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 96 / Issue 3 / June 2014
- Published online by Cambridge University Press:
- 30 April 2014, pp. 386-395
- Print publication:
- June 2014
-
- Article
-
- You have access
- Export citation
ON A THEOREM OF A. A. GOL’DBERG
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society / Volume 47 / Issue 1 / February 2004
- Published online by Cambridge University Press:
- 27 May 2004, pp. 125-129
-
- Article
-
- You have access
- Export citation