1 results
Dimension Theory via Reduced Bisector Chains
-
- Journal:
- Canadian Mathematical Bulletin / Volume 21 / Issue 3 / 01 September 1978
- Published online by Cambridge University Press:
- 20 November 2018, pp. 305-311
- Print publication:
- 01 September 1978
-
- Article
-
- You have access
- Export citation
-
Let (X, d) be a metric space and Y and Z subsets of X. We say that Z is a bisector in Y and write Y⊳Z iff Y⊃Z and there are two distinct points y1, y2 ∈ Y such that Z = ={z:d(z, y1) = d(z, y2) and z∈Y}. By a reduced bisector chain in (X, d) of length n we understand a chain X =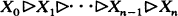
such that dim Xn≤0 and dimXn-1>0). By r(X, d) we denote the maximum length of reduced bisector chains in (X, d). For a metrizable topological space X we introduce the topological invariant r(X) as the minimum of r(X, d) taken over the set of all metrizations d of X. We prove that the function r(X) coincides with the dimension of X on the class of compact metric spaces.
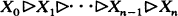such that dim X