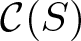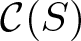1 results
Graph morphisms and exhaustion of curve graphs of low-genus surfaces
- Part of
-
- Journal:
- Proceedings of the Edinburgh Mathematical Society , First View
- Published online by Cambridge University Press:
- 08 April 2025, pp. 1-51
-
- Article
- Export citation
-
Let S be an orientable, connected surface of finite topological type, with genus
$g \leqslant 2$, empty boundary and complexity at least 2; we prove that any graph endomorphism of the curve graph of S is actually an automorphism. Also, as a complement of the author’s previous results, we prove that under mild conditions on the complexity of the underlying surfaces, any graph morphism between curve graphs is induced by a homeomorphism of the surfaces.To prove these results, we construct a finite subgraph whose union of iterated rigid expansions is the curve graph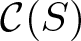
$\mathcal{C}(S)$. The sets constructed, and the method of rigid expansion, are closely related to Aramayona and Leininger’s finite rigid sets. We prove as a consequence that Aramayona and Leininger’s rigid set also exhausts 
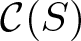$\mathcal{C}(S)$ via rigid expansions. The combinatorial rigidity results follow as an immediate consequence, based on the author’s previous results.
