1 results
COCHAIN SEQUENCES AND THE QUILLEN CATEGORY OF A COCLASS FAMILY
- Part of
-
- Journal:
- Journal of the Australian Mathematical Society / Volume 102 / Issue 2 / April 2017
- Published online by Cambridge University Press:
- 12 May 2016, pp. 185-204
- Print publication:
- April 2017
-
- Article
-
- You have access
- Export citation
-
We introduce the concept of infinite cochain sequences and initiate a theory of homological algebra for them. We show how these sequences simplify and improve the construction of infinite coclass families (as introduced by Eick and Leedham-Green) and also how they can be applied to prove that almost all groups in such a family have equivalent Quillen categories. We also include some examples of infinite families of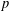
$p$-groups from different coclass families that have equivalent Quillen categories.
