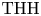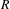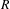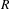John Rognes developed a notion of Galois extension of commutative ring spectra, and this includes a criterion for identifying an extension as unramified. Ramification for commutative ring spectra can be detected by relative topological Hochschild homology and by topological André–Quillen homology. In the classical algebraic context, it is important to distinguish between tame and wild ramification. Noether’s theorem characterizes tame ramification in terms of a normal basis, and tame ramification can also be detected via the surjectivity of the trace map. For commutative ring spectra, we suggest to study the Tate construction as a suitable analog. It tells us at which integral primes there is tame or wild ramification, and we determine its homotopy type in examples in the context of topological K-theory and topological modular forms.

In this paper we compute the topological Hochschild homology of quotients of discrete valuation rings (DVRs). Along the way we give a short argument for Bökstedt periodicity and generalizations over various other bases. Our strategy also gives a very efficient way to redo the computations of $\operatorname {THH}$ (respectively, logarithmic $\operatorname {THH}$) of complete DVRs originally due to Lindenstrauss and Madsen (respectively, Hesselholt and Madsen).

We develop a theory of $R$-module Thom spectra for a commutative symmetric ring spectrum $R$ and we analyze their multiplicative properties. As an interesting source of examples, we show that $R$-algebra Thom spectra associated to the special unitary groups can be described in terms of quotient constructions on $R$. We apply the general theory to obtain a description of the $R$-based topological Hochschild homology associated to an $R$-algebra Thom spectrum.