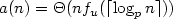Search

Skip to main content Accessibility help

Home
Search

Only show content I have access to (0)

Articles (1)

Over 3 years (1)

From year:

To year:

Apply

Computer Science (1)

toeplitz word

RAIRO - Theoretical Informatics and Applications (1)

View selected items
Save to my bookmarks
Export citations
Download PDF (zip)
Save to Kindle
Save to Dropbox
Save to Google Drive
Save content to
To save content items to your account, please confirm that you agree to abide by our usage policies. If this is the first time you use this feature, you will be asked to authorise Cambridge Core to connect with your account. Find out more about saving content to .

To save content items to your Kindle, first ensure [email protected] is added to your Approved Personal Document E-mail List under your Personal Document Settings on the Manage Your Content and Devices page of your Amazon account. Then enter the ‘name’ part of your Kindle email address below. Find out more about saving to your Kindle.

Note you can select to save to either the @free.kindle.com or @kindle.com variations. ‘@free.kindle.com’ emails are free but can only be saved to your device when it is connected to wi-fi. ‘@kindle.com’ emails can be delivered even when you are not connected to wi-fi, but note that service fees apply.

Find out more about the Kindle Personal Document Service.

Please be advised that item(s) you selected are not available.
You are about to save
Your Kindle email address

Please provide your Kindle email.

@free.kindle.com @kindle.com (service fees apply)

By using this service, you agree that you will only keep content for personal use, and will not openly distribute them via Dropbox, Google Drive or other file sharing services

1 results

On possible growths of arithmetical complexity
Anna E. Frid
Journal:

RAIRO - Theoretical Informatics and Applications / Volume 40 / Issue 3 / July 2006

Published online by Cambridge University Press:

18 October 2006, pp. 443-458

Print publication:

July 2006
- Article
- - Get access
    
    Check if you have access via personal or institutional login
    
    Log in Register
- Export citation
The arithmetical complexity of infinite words, defined by Avgustinovich, Fon-Der-Flaass and the author in 2000, is the number of words of length n which occur in the arithmetical subsequences of the infinite word. This is one of the modifications of the classical function of subword complexity, which is equal to the number of factors of the infinite word of length n. In this paper, we show that the orders of growth of the arithmetical complexity can behave as many sub-polynomial functions. More precisely, for each sequence u of subword complexity ƒu(n) and for each prime p ≥ 3 we build a Toeplitz word on the same alphabet whose arithmetical complexity is $a(n)=\Theta(n f_u(\lceil \log_p n \rceil))$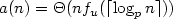.