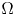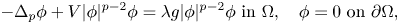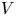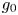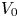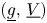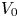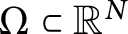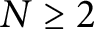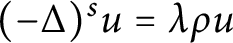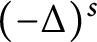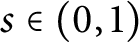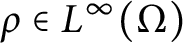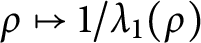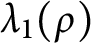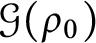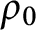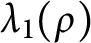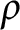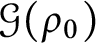For $N\geq 2$, a bounded smooth domain $\Omega$ in $\mathbb {R}^{N}$, and $g_0,\, V_0 \in L^{1}_{loc}(\Omega )$, we study the optimization of the first eigenvalue for the following weighted eigenvalue problem:

\[ -\Delta_p \phi + V |\phi|^{p-2}\phi = \lambda g |\phi|^{p-2}\phi \text{ in } \Omega, \quad \phi=0 \text{ on } \partial \Omega, \]

$g$

$V$

$g_0$

$V_0$

$(\underline {g},\,\underline {V})$

$(\overline {g},\,\overline {V})$

$g_0$

$V_0$

$p=2$

Let 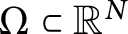$\Omega \subset \mathbb {R}^N$ , 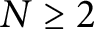$N\geq 2$ , be an open bounded connected set. We consider the fractional weighted eigenvalue problem 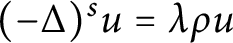$(-\Delta )^s u =\lambda \rho u$ in $\Omega $ with homogeneous Dirichlet boundary condition, where 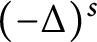$(-\Delta )^s$ , 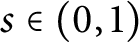$s\in (0,1)$ , is the fractional Laplacian operator, $\lambda \in \mathbb {R}$ and 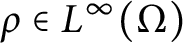$ \rho \in L^\infty (\Omega )$ .

We study weak* continuity, convexity and Gâteaux differentiability of the map 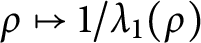$\rho \mapsto 1/\lambda _1(\rho )$ , where 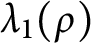$\lambda _1(\rho )$ is the first positive eigenvalue. Moreover, denoting by 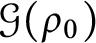$\mathcal {G}(\rho _0)$ the class of rearrangements of 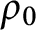$\rho _0$ , we prove the existence of a minimizer of 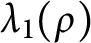$\lambda _1(\rho )$ when 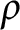$\rho $ varies on 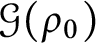$\mathcal {G}(\rho _0)$ . Finally, we show that, if $\Omega $ is Steiner symmetric, then every minimizer shares the same symmetry.