4 results
Online Selection of Alternating Subsequences from a Random Sample
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 48 / Issue 4 / December 2011
- Published online by Cambridge University Press:
- 14 July 2016, pp. 1114-1132
- Print publication:
- December 2011
-
- Article
-
- You have access
- Export citation
A prophet inequality for independent random variables with finite variances
- Part of
-
- Journal:
- Journal of Applied Probability / Volume 34 / Issue 4 / December 1997
- Published online by Cambridge University Press:
- 14 July 2016, pp. 945-958
- Print publication:
- December 1997
-
- Article
- Export citation
‘Wald's Lemma' for sums of order statistics of i.i.d. random variables
-
- Journal:
- Advances in Applied Probability / Volume 23 / Issue 3 / September 1991
- Published online by Cambridge University Press:
- 01 July 2016, pp. 612-623
- Print publication:
- September 1991
-
- Article
- Export citation
Optimal stopping problems with generalized objective functions
-
- Journal:
- Journal of Applied Probability / Volume 27 / Issue 4 / December 1990
- Published online by Cambridge University Press:
- 14 July 2016, pp. 828-838
- Print publication:
- December 1990
-
- Article
- Export citation

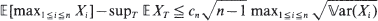 , where the supremum is over all stopping times
, where the supremum is over all stopping times 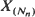 and
and 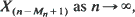 as
as 