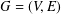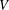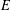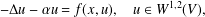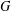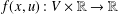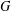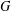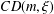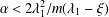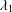1 results
ON A CLASS OF NONLINEAR SCHRÖDINGER EQUATIONS ON FINITE GRAPHS
- Part of
-
- Journal:
- Bulletin of the Australian Mathematical Society / Volume 101 / Issue 3 / June 2020
- Published online by Cambridge University Press:
- 20 February 2020, pp. 477-487
- Print publication:
- June 2020
-
- Article
- Export citation
-
Suppose that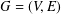
$G=(V,E)$ is a finite graph with the vertex set 
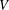$V$ and the edge set 
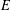$E$. Let 
$\unicode[STIX]{x1D6E5}$ be the usual graph Laplacian. Consider the nonlinear Schrödinger equation of the form on the graph
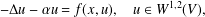$$\begin{eqnarray}-\unicode[STIX]{x1D6E5}u-\unicode[STIX]{x1D6FC}u=f(x,u),\quad u\in W^{1,2}(V),\end{eqnarray}$$
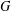$G$, where 
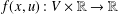$f(x,u):V\times \mathbb{R}\rightarrow \mathbb{R}$ is a nonlinear real-valued function and 
$\unicode[STIX]{x1D6FC}$ is a parameter. We prove an integral inequality on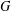 
$G$ under the assumption that 
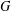$G$ satisfies the curvature-dimension type inequality 
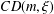$CD(m,\unicode[STIX]{x1D709})$. Then by using the Poincaré–Sobolev inequality, the Trudinger–Moser inequality and the integral inequality on 
$G$, we prove that there is a nontrivial solution to the nonlinear Schrödinger equation if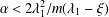 
$\unicode[STIX]{x1D6FC}<2\unicode[STIX]{x1D706}_{1}^{2}/m(\unicode[STIX]{x1D706}_{1}-\unicode[STIX]{x1D709})$, where 
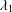$\unicode[STIX]{x1D706}_{1}$ is the first positive eigenvalue of the graph Laplacian.
