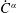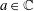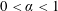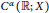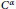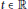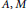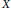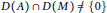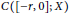In this paper, by using operator-valued ${\dot{C}}^{\unicode[STIX]{x1D6FC}}$-Fourier multiplier results on vector-valued Hölder continuous function spaces, we give a characterization of the $C^{\unicode[STIX]{x1D6FC}}$-well-posedness for the third order differential equations $au^{\prime \prime \prime }(t)+u^{\prime \prime }(t)=Au(t)+Bu^{\prime }(t)+f(t)$, ($t\in \mathbb{R}$), where $A,B$ are closed linear operators on a Banach space $X$ such that $D(A)\subset D(B)$, $a\in \mathbb{C}$ and $0<\unicode[STIX]{x1D6FC}<1$.

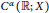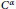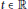Using known operator-valued Fourier multiplier results on vector-valued Hölder continuous function spaces ${{C}^{\alpha }}(\mathbb{R};\,X)$, we completely characterize the ${{C}^{\alpha }}$-well-posedness of the first order degenerate differential equations with finite delay $(Mu{)}'(t)\,=\,Au(t)\,+\,F{{u}_{t}}\,+\,f(t)$ for $t\,\in \,\mathbb{R}$ by the boundedness of the $(M,\,F)$-resolvent of A under suitable assumption on the delay operator $F$, where 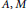$A,M$ are closed linear operators on a Banach space 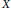$X$ satisfying 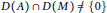$D(A)\,\cap \,D(M)\,\ne \,\{0\}$, the delay operator $F$ is a bounded linear operator from 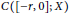$C([-r,0];X)$ to 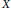$X$, and $r\,>\,0$ is fixed.