We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
We use cookies to distinguish you from other users and to provide you with a better experience on our websites. Close this message to accept cookies or find out how to manage your cookie settings.
The aim of this article is to classify two-dimensional split trianguline representations of p-adic fields. This is a generalization of a result of Colmez who classified two-dimensional split trianguline representations of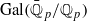 
for p≠2 by using (φ,Γ)-modules over a Robba ring. In this article, for any prime p and for any p-adic field K, we classify two-dimensional split trianguline representations of 
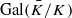using B-pairs as defined by Berger.
