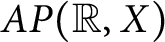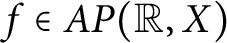In this paper, we consider an equivalence relation on the space
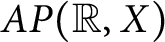 $AP(\mathbb {R},X)$
of almost periodic functions with values in a prefixed Banach space X. In this context, it is known that the normality or Bochner-type property, which characterizes these functions, is based on the relative compactness of the family of translates. Now, we prove that every equivalence class is sequentially compact and the family of translates of a function belonging to this subspace is dense in its own class, i.e., the condition of almost periodicity of a function
$AP(\mathbb {R},X)$
of almost periodic functions with values in a prefixed Banach space X. In this context, it is known that the normality or Bochner-type property, which characterizes these functions, is based on the relative compactness of the family of translates. Now, we prove that every equivalence class is sequentially compact and the family of translates of a function belonging to this subspace is dense in its own class, i.e., the condition of almost periodicity of a function
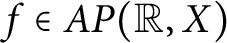 $f\in AP(\mathbb {R},X)$
yields that every sequence of translates of f has a subsequence that converges to a function equivalent to f. This extends previous work by the same authors on the case of numerical almost periodic functions.
$f\in AP(\mathbb {R},X)$
yields that every sequence of translates of f has a subsequence that converges to a function equivalent to f. This extends previous work by the same authors on the case of numerical almost periodic functions.