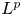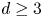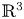1. Introduction
Let $\gamma$![]() be a smooth curve from $I=[-1,1]$
be a smooth curve from $I=[-1,1]$![]() to $\mathbb {R}^{d}$
to $\mathbb {R}^{d}$![]() . We consider
. We consider
where $\psi$![]() and $\chi$
and $\chi$![]() are smooth functions supported in the interiors of the intervals $I$
are smooth functions supported in the interiors of the intervals $I$![]() and $[1,2]$
and $[1,2]$![]() , respectively. The operator $\mathfrak Rf$
, respectively. The operator $\mathfrak Rf$![]() is referred to as the restriction of X-ray transform to the line complex generated by the directions $(\gamma (s),1)$
is referred to as the restriction of X-ray transform to the line complex generated by the directions $(\gamma (s),1)$![]() , $s \in \operatorname {supp} \psi$
, $s \in \operatorname {supp} \psi$![]() . We say $\gamma$
. We say $\gamma$![]() is nondegenerate if
is nondegenerate if
The operator $\mathfrak Rf$![]() is a model case of the general class of restricted X-ray transforms (see [Reference Gelfand and Graev12, Reference Greenleaf and Seeger16–Reference Greenleaf and Uhlmann19]). Especially in $\mathbb {R}^3$
is a model case of the general class of restricted X-ray transforms (see [Reference Gelfand and Graev12, Reference Greenleaf and Seeger16–Reference Greenleaf and Uhlmann19]). Especially in $\mathbb {R}^3$![]() , under the nondegeneracy assumption (1.1), $\mathfrak Rf$
, under the nondegeneracy assumption (1.1), $\mathfrak Rf$![]() is a typical example of Fourier integral operators with one-sided fold singularity [Reference Greenleaf and Seeger13]. Regularity properties of $\mathfrak Rf$
is a typical example of Fourier integral operators with one-sided fold singularity [Reference Greenleaf and Seeger13]. Regularity properties of $\mathfrak Rf$![]() have been studied in terms of $L^p$
have been studied in terms of $L^p$![]() improving and $L^p$
improving and $L^p$![]() Sobolev regularity estimates. The $L^p$
Sobolev regularity estimates. The $L^p$![]() improving property of $\mathfrak R$
improving property of $\mathfrak R$![]() is well understood by now [Reference Greenleaf and Seeger14, Reference Greenleaf, Seeger and Wainger15, Reference Laghi24, Reference Oberlin25]. The problem was, in fact, considered in a more general framework: $L^p-L^q(L^r)$
is well understood by now [Reference Greenleaf and Seeger14, Reference Greenleaf, Seeger and Wainger15, Reference Laghi24, Reference Oberlin25]. The problem was, in fact, considered in a more general framework: $L^p-L^q(L^r)$![]() estimates for $\mathfrak R$
estimates for $\mathfrak R$![]() were studied by some authors (see, e.g. [Reference Christ and Erdoğan7, Reference Erdoğan11, Reference Wolff34]) and the estimates on the optimal range of $p,q,r$
were studied by some authors (see, e.g. [Reference Christ and Erdoğan7, Reference Erdoğan11, Reference Wolff34]) and the estimates on the optimal range of $p,q,r$![]() were established except for some endpoint cases. (See also [Reference Christ and Erdoğan8–Reference Dendrinos and Stovall10, Reference Gressman20, Reference Tao and Wright33] for related results.)
were established except for some endpoint cases. (See also [Reference Christ and Erdoğan8–Reference Dendrinos and Stovall10, Reference Gressman20, Reference Tao and Wright33] for related results.)
$L^2-L_{1/(2d)}^2$![]() bound on $\mathfrak R$
bound on $\mathfrak R$![]() is easy to obtain via $TT^*$
is easy to obtain via $TT^*$![]() argument and van der Corput's lemma [Reference Greenleaf and Seeger16] (see also [Reference Greenleaf and Seeger13, Reference Greenleaf and Uhlmann19] for the sharp $L^2$
argument and van der Corput's lemma [Reference Greenleaf and Seeger16] (see also [Reference Greenleaf and Seeger13, Reference Greenleaf and Uhlmann19] for the sharp $L^2$![]() Sobolev estimates for general class of operators). Interpolation between this and the trivial $L^\infty$
Sobolev estimates for general class of operators). Interpolation between this and the trivial $L^\infty$![]() estimate shows that $\mathfrak R$
estimate shows that $\mathfrak R$![]() is bounded from $L^p$
is bounded from $L^p$![]() to $L_{1/(pd)}^p$
to $L_{1/(pd)}^p$![]() for $p\ge 2$
for $p\ge 2$![]() . This is optimal in that $L^p-L_{\alpha }^p$
. This is optimal in that $L^p-L_{\alpha }^p$![]() estimate fails if $\alpha > 1/(pd)$
estimate fails if $\alpha > 1/(pd)$![]() (see Proposition 5.1). However, when $p< 2$
(see Proposition 5.1). However, when $p< 2$![]() , the sharp $L^p$
, the sharp $L^p$![]() regularity estimate is less straightforward. Such an estimate has not known until recently. When $d=2$
regularity estimate is less straightforward. Such an estimate has not known until recently. When $d=2$![]() , the optimal $L^p-L_{1/p'}^p$
, the optimal $L^p-L_{1/p'}^p$![]() estimate was established for $1< p<4/3$
estimate was established for $1< p<4/3$![]() by Pramanik and Seeger's conditional result [Reference Pramanik and Seeger31] and the sharp decoupling inequality for the cone $\subset \mathbb {R}^3$
by Pramanik and Seeger's conditional result [Reference Pramanik and Seeger31] and the sharp decoupling inequality for the cone $\subset \mathbb {R}^3$![]() due to Bourgain and Demeter [Reference Bourgain and Demeter5]. Those estimates and interpolation give the sharp $L^p-L_{1/(2d+)}^p$
due to Bourgain and Demeter [Reference Bourgain and Demeter5]. Those estimates and interpolation give the sharp $L^p-L_{1/(2d+)}^p$![]() estimate for $4/3\le p<2$
estimate for $4/3\le p<2$![]() , but the endpoint $L^p-L_{1/(2d)}^p$
, but the endpoint $L^p-L_{1/(2d)}^p$![]() estimate remains open. (See Conjecture 1.1.) In $\mathbb {R}^3$
estimate remains open. (See Conjecture 1.1.) In $\mathbb {R}^3$![]() the result has been extended to more general operators. In fact, Pramanik and Seeger [Reference Pramanik and Seeger30] obtained the sharp $L^p$
the result has been extended to more general operators. In fact, Pramanik and Seeger [Reference Pramanik and Seeger30] obtained the sharp $L^p$![]() regularity estimates for the Fourier integral operator with folding canonical relation. Bentsen [Reference Bentsen4] (also see [Reference Bentsen3]) extended the result to a class of radon transforms with fold and blowdown singularities.
regularity estimates for the Fourier integral operator with folding canonical relation. Bentsen [Reference Bentsen4] (also see [Reference Bentsen3]) extended the result to a class of radon transforms with fold and blowdown singularities.
However, in higher dimensions ($d\ge 3$![]() ) the sharp $L^p$
) the sharp $L^p$![]() regularity estimates for $\mathfrak R$
regularity estimates for $\mathfrak R$![]() have remained open for $1< p<2$
have remained open for $1< p<2$![]() . Set $p_d=2d/(2d-1)$
. Set $p_d=2d/(2d-1)$![]() and
and

It is natural to conjecture the following.
Conjecture 1.1 Let $d\ge 3$![]() and $1< p < 2$
and $1< p < 2$![]() . Suppose $\gamma$
. Suppose $\gamma$![]() is a smooth nondegenerate curve. Then, $\mathfrak R$
is a smooth nondegenerate curve. Then, $\mathfrak R$![]() boundedly maps $L^p$
boundedly maps $L^p$![]() to $L_\alpha ^p$
to $L_\alpha ^p$![]() for $\alpha \le \alpha (p)$
for $\alpha \le \alpha (p)$![]() .
.
Failure of $L^p-L_\alpha ^p$![]() boundedness for $\alpha > \alpha (p)$
boundedness for $\alpha > \alpha (p)$![]() can be shown by a slight modification of the examples in [Reference Pramanik and Seeger31]. (See Proposition 5.1.) The following is our main result which verifies the conjecture except for some endpoint cases in every dimension $d\ge 3$
can be shown by a slight modification of the examples in [Reference Pramanik and Seeger31]. (See Proposition 5.1.) The following is our main result which verifies the conjecture except for some endpoint cases in every dimension $d\ge 3$![]() .
.
Theorem 1.2 Let $d\ge 3$![]() and $1\le p< p_d$
and $1\le p< p_d$![]() . Suppose $\gamma$
. Suppose $\gamma$![]() is nondegenerate. Then,
is nondegenerate. Then,
holds if and only if $\alpha \le 1-1/p$![]() .
.
When $p \in [p_d,2)$![]() , interpolation with the $L^2-L_{1/(2d)}^2$
, interpolation with the $L^2-L_{1/(2d)}^2$![]() estimate yields (1.2) for $\alpha <\alpha (p)$
estimate yields (1.2) for $\alpha <\alpha (p)$![]() but estimate (1.2) with the endpoint regularity $\alpha =\alpha (p)$
but estimate (1.2) with the endpoint regularity $\alpha =\alpha (p)$![]() , which looks to be a subtle problem, remains open. By a standard scaling argument [Reference Pramanik and Seeger29, Reference Pramanik and Seeger31] the result in Theorem 1.2 can be extended to the curves of finite type.
, which looks to be a subtle problem, remains open. By a standard scaling argument [Reference Pramanik and Seeger29, Reference Pramanik and Seeger31] the result in Theorem 1.2 can be extended to the curves of finite type.
A curve $\gamma : I \mapsto \mathbb {R}^{d}$![]() is said to be of finite type if there is an $L=L(s)$
is said to be of finite type if there is an $L=L(s)$![]() such that $\operatorname {span} \{ \gamma ^{(1)}(s), \dots, \gamma ^{(L)}(s)\}=\mathbb {R}^d$
such that $\operatorname {span} \{ \gamma ^{(1)}(s), \dots, \gamma ^{(L)}(s)\}=\mathbb {R}^d$![]() for each $s\in I$
for each $s\in I$![]() , and the smallest of such $L(s)$
, and the smallest of such $L(s)$![]() is called the type at $s$
is called the type at $s$![]() . The supremum of the type over $s \in I$
. The supremum of the type over $s \in I$![]() is called the maximal type of $\gamma$
is called the maximal type of $\gamma$![]() (see, e.g. [Reference Ham and Lee21, Reference Pramanik and Seeger29]).
(see, e.g. [Reference Ham and Lee21, Reference Pramanik and Seeger29]).
Corollary 1.3 Let $d\ge 3$![]() , $1\le p<2$
, $1\le p<2$![]() and $L>d$
and $L>d$![]() . Suppose $\gamma$
. Suppose $\gamma$![]() is a curve of maximal type $L$
is a curve of maximal type $L$![]() . Then, $\mathfrak Rf$
. Then, $\mathfrak Rf$![]() is bounded from $L^p$
is bounded from $L^p$![]() to $L_\alpha ^p$
to $L_\alpha ^p$![]() for $\alpha \le \min ( \alpha (p), 1/(Lp))$
for $\alpha \le \min ( \alpha (p), 1/(Lp))$![]() if $p\neq (L+1)/L$
if $p\neq (L+1)/L$![]() when $L\ge 2d-1$
when $L\ge 2d-1$![]() , and if $p \in (1,p_d) \cup (2d/L,2)$
, and if $p \in (1,p_d) \cup (2d/L,2)$![]() when $d< L <2d-1$
when $d< L <2d-1$![]() .
.
For $p\in [2,\infty ]$![]() , it is easy to show the sharp $L^p - L_{1/(Lp)}^p$
, it is easy to show the sharp $L^p - L_{1/(Lp)}^p$![]() estimate, which can be shown by using the $L^2-L_{1/(2L)}^2$
estimate, which can be shown by using the $L^2-L_{1/(2L)}^2$![]() estimate and interpolation in a similar manner as above. Corollary 1.3 and Proposition 5.1 give the optimal Sobolev regularity estimate for $\mathfrak R$
estimate and interpolation in a similar manner as above. Corollary 1.3 and Proposition 5.1 give the optimal Sobolev regularity estimate for $\mathfrak R$![]() if $L\ge 2d-1$
if $L\ge 2d-1$![]() when $p \neq (L+1)/L$
when $p \neq (L+1)/L$![]() . However, some endpoint cases remain open not to mention such estimates for the nondegenerate curves.
. However, some endpoint cases remain open not to mention such estimates for the nondegenerate curves.
In this paper, we make use of the inductive strategy in the recent work of the authors [Reference Ko, Lee and Oh23], where smoothing properties of the (convolution) averaging operator over curves were studied (see [Reference Beltran, Guo, Hickman and Seeger2, Reference Ko, Lee and Oh22, Reference Oberlin and Smith26, Reference Oberlin, Smith and Sogge27, Reference Tao and Vargas32] for previous works). Exploiting similarity between $\mathfrak R^\ast f$![]() and the averaging operator, we adapt our previous argument. The main new feature of the current paper is use of the decoupling inequality associated with the conical sets generated by curves (see Definition 2.5 and Theorem 3.1). Compared with our previous work where the averaging operator was decoupled by a class of symbols adjusted to short subcurves, our new decoupling inequality allows us to dispense with some technicalities related to the symbols. The decoupling inequality can also be used to simplify the argument in [Reference Ko, Lee and Oh23].
and the averaging operator, we adapt our previous argument. The main new feature of the current paper is use of the decoupling inequality associated with the conical sets generated by curves (see Definition 2.5 and Theorem 3.1). Compared with our previous work where the averaging operator was decoupled by a class of symbols adjusted to short subcurves, our new decoupling inequality allows us to dispense with some technicalities related to the symbols. The decoupling inequality can also be used to simplify the argument in [Reference Ko, Lee and Oh23].
Organization. In § 2, we reduce the proof of Theorem 1.2 to obtain Proposition 2.4. We prove a decoupling inequality associated with a nondegenerate curve (Theorem 3.1) in § 3 which is crucial for the proof of Proposition 2.4. The proofs of Proposition 2.4 and Theorem 1.2 are given in § 4 and § 5, respectively. We discuss the sharpness of the smoothing order $\alpha$![]() in § 5.
in § 5.
Notation. For positive constants $A, D$![]() , we denote $A\lesssim D$
, we denote $A\lesssim D$![]() if there exists a (independent) constant $C$
if there exists a (independent) constant $C$![]() such that $A\le CD$
such that $A\le CD$![]() , where the constant $C$
, where the constant $C$![]() may vary from line to line depending on the context.
may vary from line to line depending on the context.
2. Estimates with localized frequency
In this section, we reduce the proof of Theorem 1.2 to show an inductive statement (see Proposition 2.4). Afterwards, we obtain some preliminary results which are needed to prove Proposition 2.4.
Let us consider the operator
which is the dual operator of $\mathfrak R$![]() . By duality estimate (1.2) is equivalent to
. By duality estimate (1.2) is equivalent to
For the purpose, we closely follow the line of arguments in our previous paper [Reference Ko, Lee and Oh23]. So, there is a significant overlap between the current paper and [Reference Ko, Lee and Oh23]. This can be avoided by omitting some shared details. However, we decide to include them so that the paper is self-contained and more easily accessible.
2.1 Frequency localized estimate
We begin with defining a class of curves in order to prove (2.1) in an inductive manner. For an integer $1 \le L \le d$![]() , by ${\rm Vol} (v_1,\ldots,v_L)$
, by ${\rm Vol} (v_1,\ldots,v_L)$![]() we denote the $L$
we denote the $L$![]() -dimensional volume of the parallelepiped generated by vectors $v_1,\ldots, v_L \in \mathbb {R}^d$
-dimensional volume of the parallelepiped generated by vectors $v_1,\ldots, v_L \in \mathbb {R}^d$![]() .
.
Definition 2.1 Let $B\ge 1$![]() . We say $\gamma \in \mathfrak V^d(L,B)$
. We say $\gamma \in \mathfrak V^d(L,B)$![]() if $\gamma \in C^{3d+1}(I)$
if $\gamma \in C^{3d+1}(I)$![]() satisfies
satisfies
For a smooth function $a(s,t,\xi )$![]() on $I \times [1,2] \times \mathbb {R}^d$
on $I \times [1,2] \times \mathbb {R}^d$![]() , we define
, we define
Here, $\mathcal {F}_x$![]() denotes Fourier transform in $x$
denotes Fourier transform in $x$![]() . Note that $\mathcal {R}f =\mathcal {R}[a]f$
. Note that $\mathcal {R}f =\mathcal {R}[a]f$![]() if $a(s,t,\xi )=\psi (s)\chi (t)$
if $a(s,t,\xi )=\psi (s)\chi (t)$![]() . We prove estimate (2.1) by induction on $L$
. We prove estimate (2.1) by induction on $L$![]() for $\gamma \in \mathfrak V^d(L,B)$
for $\gamma \in \mathfrak V^d(L,B)$![]() under the localized nondegeneracy assumption:
under the localized nondegeneracy assumption:

which holds if $(s,t,\xi ) \in \operatorname {supp} a$![]() for some $t$
for some $t$![]() . When $L< d$
. When $L< d$![]() , (2.4) cannot be true in general even if $\gamma$
, (2.4) cannot be true in general even if $\gamma$![]() is nondegenerate. However, an appropriate decomposition in the frequency domain makes it possible that (2.4) holds. To do this, we consider a class of symbols $a$
is nondegenerate. However, an appropriate decomposition in the frequency domain makes it possible that (2.4) holds. To do this, we consider a class of symbols $a$![]() .
.
Definition 2.2 Let $\mathbb {A}_k=\{ \xi \in \mathbb {R}^d: 2^{k-1} \le |\xi | \le 2^{k+1}\}$![]() for $k\ge 0$
for $k\ge 0$![]() , and $\mathcal {I}_L=\{(j,\alpha ): 0 \le j \le 2L, |\alpha | \le d+L+2\}$
, and $\mathcal {I}_L=\{(j,\alpha ): 0 \le j \le 2L, |\alpha | \le d+L+2\}$![]() . We say a symbol $a\in C^{d+L+2}(\mathbb {R}^{d+2})$
. We say a symbol $a\in C^{d+L+2}(\mathbb {R}^{d+2})$![]() is of type $(2^k,L,B)$
is of type $(2^k,L,B)$![]() if $\operatorname {supp} a \subset I \times [2^{-1},2^2]\times \mathbb {A}_k$
if $\operatorname {supp} a \subset I \times [2^{-1},2^2]\times \mathbb {A}_k$![]()
and (2.4) holds on $\operatorname {supp}_{s,\xi } a$![]() . Here, as in [Reference Ko, Lee and Oh23], we denote $\operatorname {supp}_{s,\xi } a=\cup _{t} \operatorname {supp} a(\cdot,t,\cdot )$
. Here, as in [Reference Ko, Lee and Oh23], we denote $\operatorname {supp}_{s,\xi } a=\cup _{t} \operatorname {supp} a(\cdot,t,\cdot )$![]() . We simply say a statement $S(s,\xi )$
. We simply say a statement $S(s,\xi )$![]() , depending on $s,\xi$
, depending on $s,\xi$![]() , holds on $\operatorname {supp} a$
, holds on $\operatorname {supp} a$![]() if $S(s,\xi )$
if $S(s,\xi )$![]() holds for $s,\xi \in \operatorname {supp}_{a,\xi } a$
holds for $s,\xi \in \operatorname {supp}_{a,\xi } a$![]() . We also use the same convention with other variables.
. We also use the same convention with other variables.
Estimate (2.1) (and hence Theorem 1.2) follows from the next theorem via a standard argument using Fefferman–Stein $\#$![]() -function (e.g. see [Reference Pramanik, Rogers and Seeger28]). See § 5.1 for details.
-function (e.g. see [Reference Pramanik, Rogers and Seeger28]). See § 5.1 for details.
Theorem 2.3 Suppose that $\gamma \in \mathfrak V^d(L,B)$![]() and $a$
and $a$![]() is a symbol of type $(2^k,L,B)$
is a symbol of type $(2^k,L,B)$![]() . Then, for $p> 2L$
. Then, for $p> 2L$![]()
As mentioned above, we prove Theorem 2.3 by induction on $L$![]() . Theorem 2.3 with $L=1$
. Theorem 2.3 with $L=1$![]() is easy to prove. Indeed, setting $\tilde {\mathcal {R}}f=\mathcal {F}_x(\mathcal {R}[a] \mathcal {F}_x^{-1}\!f)$
is easy to prove. Indeed, setting $\tilde {\mathcal {R}}f=\mathcal {F}_x(\mathcal {R}[a] \mathcal {F}_x^{-1}\!f)$![]() , we note that
, we note that
where
Since (2.4) holds with $L=1$![]() on $\operatorname {supp} a$
on $\operatorname {supp} a$![]() , integration by parts gives $|\mathcal {K}(s,s',\xi )| \le C (1+2^k|s-s'|)^{-2}.$
, integration by parts gives $|\mathcal {K}(s,s',\xi )| \le C (1+2^k|s-s'|)^{-2}.$![]() By Young's convolution inequality it follows that $\|\tilde {\mathcal {R}}^* \tilde {\mathcal {R}}f \|_2 \lesssim 2^{-k}\|f\|_2$
By Young's convolution inequality it follows that $\|\tilde {\mathcal {R}}^* \tilde {\mathcal {R}}f \|_2 \lesssim 2^{-k}\|f\|_2$![]() . Thus, we get $\| \mathcal {R}[a]f\|_2 \lesssim 2^{-k/2}\|f\|_2$
. Thus, we get $\| \mathcal {R}[a]f\|_2 \lesssim 2^{-k/2}\|f\|_2$![]() by Plancherel's theorem. Interpolation with the trivial estimate $\| \mathcal {R}[a]f\|_\infty \lesssim \|f\|_\infty$
by Plancherel's theorem. Interpolation with the trivial estimate $\| \mathcal {R}[a]f\|_\infty \lesssim \|f\|_\infty$![]() gives (2.5) with $L=1$
gives (2.5) with $L=1$![]() .
.
Consequently, Theorem 2.3 for $L\ge 2$![]() follows from the next proposition (cf. [Reference Ko, Lee and Oh23, Proposition 2.3]).
follows from the next proposition (cf. [Reference Ko, Lee and Oh23, Proposition 2.3]).
Proposition 2.4 Let $2 \le N \le d$![]() . Suppose Theorem 2.3 holds with $L=N-1$
. Suppose Theorem 2.3 holds with $L=N-1$![]() . Then, Theorem 2.3 holds with $L=N$
. Then, Theorem 2.3 holds with $L=N$![]() .
.
We prove the proposition through the rest of this section, § 3 and § 4. Fixing $2\le N\le d$![]() , we assume that Theorem 2.3 holds with $L=N-1$
, we assume that Theorem 2.3 holds with $L=N-1$![]() . Additionally, assuming that $\gamma \in \mathfrak V^d(N,B)$
. Additionally, assuming that $\gamma \in \mathfrak V^d(N,B)$![]() and $a$
and $a$![]() is of type $(2^k,N,B)$
is of type $(2^k,N,B)$![]() , we prove (2.5) for $p>2N$
, we prove (2.5) for $p>2N$![]() . For the purpose, composing the symbol $a$
. For the purpose, composing the symbol $a$![]() , we may further assume that
, we may further assume that
holds on $\operatorname {supp} a$![]() . Otherwise, (2.4) holds with $L=N-1$
. Otherwise, (2.4) holds with $L=N-1$![]() , so the hypothesis (Theorem 2.3 with $L=N-1$
, so the hypothesis (Theorem 2.3 with $L=N-1$![]() ) yields (2.5) for $p> 2(N-1)$
) yields (2.5) for $p> 2(N-1)$![]() .
.
We prove Proposition 2.4 in § 4 using the associated decoupling inequality which is obtained in § 3. The rest of the section is devoted to proving two lemmas (2.6 and 2.8) which play crucial roles in proving Proposition 2.4.
2.2 Symbols adapted to $\gamma$
We define a class of symbols adapted to the curve $\gamma$![]() . From now on, we assume that $\delta$
. From now on, we assume that $\delta$![]() satisfies
satisfies
Let $\gamma$![]() satisfy (2.3) with $L=N-1$
satisfy (2.3) with $L=N-1$![]() . For $s\in I$
. For $s\in I$![]() , set $\mathrm V_s^{\gamma,\ell } = \operatorname {span} \{\gamma ^{(j)}(s) : j=1,\dots,\ell \}$
, set $\mathrm V_s^{\gamma,\ell } = \operatorname {span} \{\gamma ^{(j)}(s) : j=1,\dots,\ell \}$![]() . Consider a linear map $\tilde {\mathcal {L}}_s^\delta :\mathbb {R}^d \mapsto \mathbb {R}^d$
. Consider a linear map $\tilde {\mathcal {L}}_s^\delta :\mathbb {R}^d \mapsto \mathbb {R}^d$![]() given as follows:
given as follows:

We also consider a linear map $\mathcal {L}_s^\delta : \mathbb {R}^{d+1} \mapsto \mathbb {R}^{d+1}$![]() given by
given by
Denoting $G(s)=(1,\gamma (s))$![]() , we set
, we set
which roughly corresponds to the Fourier support of the operator $\mathcal {R}[a]f$![]() with $\operatorname {supp}_s a$
with $\operatorname {supp}_s a$![]() included in an interval centred at $s$
included in an interval centred at $s$![]() of length about $\delta$
of length about $\delta$![]() . We define a class of symbols associated with $\Lambda _k(\delta,s)$
. We define a class of symbols associated with $\Lambda _k(\delta,s)$![]() .
.
Definition 2.5 Let $s_{\circ } \in (-1,1)$![]() and $0<\delta \le 1$
and $0<\delta \le 1$![]() such that $I(s_{\circ },\delta ):=[s_{\circ }-\delta, s_{\circ }+\delta ]\subset I$
such that $I(s_{\circ },\delta ):=[s_{\circ }-\delta, s_{\circ }+\delta ]\subset I$![]() . We denote by $\mathfrak A_k(\delta,s_{\circ })=\mathfrak A_k(\delta, s_{\circ },d,N,B,\gamma )$
. We denote by $\mathfrak A_k(\delta,s_{\circ })=\mathfrak A_k(\delta, s_{\circ },d,N,B,\gamma )$![]() the set of smooth functions $\mathfrak a$
the set of smooth functions $\mathfrak a$![]() on $\mathbb {R}^{d+3}$
on $\mathbb {R}^{d+3}$![]() which satisfies the following $:$
which satisfies the following $:$![]()
It should be noted that there is no $s$![]() -differentiation in (2.9). Here, $\mathcal {I}_N$
-differentiation in (2.9). Here, $\mathcal {I}_N$![]() is given in Definition 2.2. We set
is given in Definition 2.2. We set
Clearly, $\mathcal {R}[a]f=\mathcal {T}[a]f$![]() if $\mathfrak a=a(s,t,\xi )$
if $\mathfrak a=a(s,t,\xi )$![]() . The following is an analogue of [Reference Ko, Lee and Oh23, Lemma 2.7].
. The following is an analogue of [Reference Ko, Lee and Oh23, Lemma 2.7].
Lemma 2.6 Let $\tilde \chi \in \mathrm C_0^\infty ((2^{-2},2^2))$![]() such that $\tilde \chi =1$
such that $\tilde \chi =1$![]() on $[3^{-1},3]$
on $[3^{-1},3]$![]() . Let $\mathfrak a$
. Let $\mathfrak a$![]() be a smooth function which satisfies (2.8) and (2.9) with $j\le 2$
be a smooth function which satisfies (2.8) and (2.9) with $j\le 2$![]() and $|\alpha | \le d+3$
and $|\alpha | \le d+3$![]() . Then, we have
. Then, we have
for $p\ge 2$![]() , and
, and
Proof. Note that $\mathcal {T}[\mathfrak a]f (x,t) = \int K[\mathfrak a](s,t, \cdot )\ast f(\cdot,s)(x)\,{\rm d}s$![]() where
where
It is easy to show that $|(\mathcal {L}_{s_{\circ }}^\delta )^{-1} \mathcal {L}_s^\delta (\tau,\xi )| \sim |(\tau,\xi )|$![]() provided $|s-s_{\circ }| \le \delta$
provided $|s-s_{\circ }| \le \delta$![]() (cf. [Reference Ko, Lee and Oh23, Lemma 2.6]). Since (2.8) and (2.9) hold with $j=0$
(cf. [Reference Ko, Lee and Oh23, Lemma 2.6]). Since (2.8) and (2.9) hold with $j=0$![]() and $|\alpha | \le d+3$
and $|\alpha | \le d+3$![]() , it follows that $\operatorname {supp} \mathfrak a(s,t,2^k\mathcal {L}_s^\delta \cdot ) \subset \{(\tau,\xi ):|(\tau,\xi )| \lesssim 1\}$
, it follows that $\operatorname {supp} \mathfrak a(s,t,2^k\mathcal {L}_s^\delta \cdot ) \subset \{(\tau,\xi ):|(\tau,\xi )| \lesssim 1\}$![]() and $|\partial _{\tau,\xi }^\alpha ( \mathfrak a(s,t,2^k\mathcal {L}_s^\delta (\tau,\xi )))| \lesssim 1$
and $|\partial _{\tau,\xi }^\alpha ( \mathfrak a(s,t,2^k\mathcal {L}_s^\delta (\tau,\xi )))| \lesssim 1$![]() , $|\alpha | \le d+3$
, $|\alpha | \le d+3$![]() . By changing variables $(\tau,\xi ) \rightarrow 2^k\mathcal {L}_{s}^\delta (\tau,\xi )$
. By changing variables $(\tau,\xi ) \rightarrow 2^k\mathcal {L}_{s}^\delta (\tau,\xi )$![]() followed by repeated integration by parts, we have
followed by repeated integration by parts, we have
This gives $\|K[\mathfrak a](s,t,\cdot )\|_{L^1_x}\lesssim 1$![]() . From (2.8), note $\mathcal {T}[\mathfrak a]f (x,t) = \int _{I(s_{\circ },\delta )} K[\mathfrak a](s,t, \cdot )\ast f(\cdot,s)(x)\,{\rm d}s$
. From (2.8), note $\mathcal {T}[\mathfrak a]f (x,t) = \int _{I(s_{\circ },\delta )} K[\mathfrak a](s,t, \cdot )\ast f(\cdot,s)(x)\,{\rm d}s$![]() . Thus, we get
. Thus, we get
Recall (2.10). By Plancherel's theorem, integration by parts in $t'$![]() , and Hölder's inequality, we get
, and Hölder's inequality, we get
Thus, interpolation gives (2.11). To show (2.12), we note from the above estimate for $K[\mathfrak a](s,t,x)$![]() that $\|(1-\tilde \chi (t))K[\mathfrak a](s,t,\cdot )\|_{L^1_x}\lesssim \mathfrak K(t)=: 2^{-k} \delta ^{-N} |t-1|^{-1}(1-\tilde \chi (t))$
that $\|(1-\tilde \chi (t))K[\mathfrak a](s,t,\cdot )\|_{L^1_x}\lesssim \mathfrak K(t)=: 2^{-k} \delta ^{-N} |t-1|^{-1}(1-\tilde \chi (t))$![]() . By (2.8), using Hölder's and Young's convolution inequalities, as before, we see that $\|(1-\tilde \chi )\mathcal {T}[\mathfrak a]f\|_p^p$
. By (2.8), using Hölder's and Young's convolution inequalities, as before, we see that $\|(1-\tilde \chi )\mathcal {T}[\mathfrak a]f\|_p^p$![]() is bounded above by constant times
is bounded above by constant times
This gives (2.12).
2.3 Rescaling
Let $I(s_{\circ },\delta )\subset I$![]() . For $\gamma \in \mathfrak V^d(N,B)$
. For $\gamma \in \mathfrak V^d(N,B)$![]() we consider a rescaled curve
we consider a rescaled curve
Lemma 2.7 Let $\gamma \in \mathfrak V^d(N,B)$![]() . If $0<\delta <\delta _\ast$
. If $0<\delta <\delta _\ast$![]() for a $\delta _\ast$
for a $\delta _\ast$![]() small enough, $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N,3 B)$
small enough, $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N,3 B)$![]() and $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N-1,B')$
and $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N-1,B')$![]() for some $B'$
for some $B'$![]() .
.
Proof. Taylor series expansion of $\gamma ^{(j)}(\delta s+s_{\circ })$![]() at $s=0$
at $s=0$![]() yields:
yields:

for $1 \le \ell \le N-1$![]() and $(\gamma _{s_{\circ }}^\delta )^{(N)}(s)=(\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } \gamma ^{(N)}(s_{\circ })+O(B\delta )$
and $(\gamma _{s_{\circ }}^\delta )^{(N)}(s)=(\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } \gamma ^{(N)}(s_{\circ })+O(B\delta )$![]() . Writing $\gamma ^{(N)}(s_{\circ })=v_1+v_2 \in \mathrm V_{s_{\circ }}^{\gamma,N-1} \oplus (\mathrm V_{s_{\circ }}^{\gamma,N-1})^\perp$
. Writing $\gamma ^{(N)}(s_{\circ })=v_1+v_2 \in \mathrm V_{s_{\circ }}^{\gamma,N-1} \oplus (\mathrm V_{s_{\circ }}^{\gamma,N-1})^\perp$![]() , we have $(\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } \gamma ^{(N)}(s_{\circ })=(\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } v_1+v_2=v_2+O(B\delta )$
, we have $(\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } \gamma ^{(N)}(s_{\circ })=(\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } v_1+v_2=v_2+O(B\delta )$![]() . Since $\gamma \in \mathfrak V^d(N,B)$
. Since $\gamma \in \mathfrak V^d(N,B)$![]() , we see $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N,3B)$
, we see $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N,3B)$![]() if $0<\delta <\delta _\ast$
if $0<\delta <\delta _\ast$![]() for a sufficiently small $\delta _\ast >0$
for a sufficiently small $\delta _\ast >0$![]() . Consequently, $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N-1,B')$
. Consequently, $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N-1,B')$![]() for some $B'$
for some $B'$![]() .
.
The following lemma, which is an analogue of [Reference Ko, Lee and Oh23, Lemma 2.8], is important for our inductive argument. Let us set
Lemma 2.8 Let $s_{\circ } \in (-1,1)$![]() , $\mathfrak a \in \mathfrak A_k(\delta,s_{\circ })$
, $\mathfrak a \in \mathfrak A_k(\delta,s_{\circ })$![]() , and $\gamma \in \mathfrak V^d(N,B)$
, and $\gamma \in \mathfrak V^d(N,B)$![]() . Suppose
. Suppose

for $(s,\xi )\in I(s_{\circ },\delta )\times \operatorname {supp}_\xi \mathfrak a$![]() . Then, there exist constants $C$
. Then, there exist constants $C$![]() , $\tilde B$
, $\tilde B$![]() , $\delta _{*}=\delta _{*}(B,N,d)$
, $\delta _{*}=\delta _{*}(B,N,d)$![]() , and $\tilde f$
, and $\tilde f$![]() and a symbol $\tilde a$
and a symbol $\tilde a$![]() such that
such that
for $0<\delta <\delta _{*}$![]() , $\|\tilde f\|_p=\|f\|_p$
, $\|\tilde f\|_p=\|f\|_p$![]() , $|\partial _t^j\partial _\xi ^\alpha \tilde a(s,t,\xi )| \le \tilde B|\xi |^{-|\alpha |}$
, $|\partial _t^j\partial _\xi ^\alpha \tilde a(s,t,\xi )| \le \tilde B|\xi |^{-|\alpha |}$![]() for $(j,\alpha )\in \mathcal {I}_{N-1}$
for $(j,\alpha )\in \mathcal {I}_{N-1}$![]() , and
, and
Proof. Let $\mathfrak a_\delta (s,t,\tau,\xi )=\mathfrak a(\delta s+s_{\circ },t,\tau,\xi )$![]() . By Fourier inversion and (2.10), changing variables $s\rightarrow \delta s+s_{\circ }$
. By Fourier inversion and (2.10), changing variables $s\rightarrow \delta s+s_{\circ }$![]() , $(\tau,\xi )\rightarrow (\tau -\gamma (s_{\circ })\cdot \xi, \xi )$
, $(\tau,\xi )\rightarrow (\tau -\gamma (s_{\circ })\cdot \xi, \xi )$![]() gives
gives
where
We observe that
where
It is clear that (2.15) holds for some $C\ge 1$![]() . Since $\mathfrak a \in \mathfrak A_k(\delta,s_{\circ })$
. Since $\mathfrak a \in \mathfrak A_k(\delta,s_{\circ })$![]() , it is not difficult to see $|\partial _t^j \partial _\xi ^\alpha \tilde a(s,t,\xi )| \le \tilde B |\xi |^{-|\alpha |}$
, it is not difficult to see $|\partial _t^j \partial _\xi ^\alpha \tilde a(s,t,\xi )| \le \tilde B |\xi |^{-|\alpha |}$![]() for $(j,\alpha ) \in \mathcal {I}_{N-1}$
for $(j,\alpha ) \in \mathcal {I}_{N-1}$![]() (see (2.25) in [Reference Ko, Lee and Oh23]).
(see (2.25) in [Reference Ko, Lee and Oh23]).
Set $\mathcal {C}_p=\mathcal {C}_p(\delta ):= \delta ^{1/p} |\det \delta ^{-N} \tilde {\mathcal {L}}_{s_{\circ }}^\delta |^{1-1/p}$![]() . Let $\tilde f$
. Let $\tilde f$![]() be given by $\mathcal {F}_x \tilde f (\xi,s)= \mathcal {C}_p \mathcal {F}_x f( \delta ^{-N} \tilde {\mathcal {L}}_{s_{\circ }}^\delta \xi, \delta s+s_{\circ })$
be given by $\mathcal {F}_x \tilde f (\xi,s)= \mathcal {C}_p \mathcal {F}_x f( \delta ^{-N} \tilde {\mathcal {L}}_{s_{\circ }}^\delta \xi, \delta s+s_{\circ })$![]() , thus $\|\tilde f\|_p=\|f\|_p$
, thus $\|\tilde f\|_p=\|f\|_p$![]() . Recalling (2.16) and changing variables $\xi \rightarrow \delta ^{-N} \tilde {\mathcal {L}}_{s_{\circ }}^\delta \xi$
. Recalling (2.16) and changing variables $\xi \rightarrow \delta ^{-N} \tilde {\mathcal {L}}_{s_{\circ }}^\delta \xi$![]() , we now have
, we now have
This gives $\tilde \chi (t)\mathcal {T} [\mathfrak a]f(x,t) =\mathcal {C}_{p'} \mathcal {R}[\gamma _{s_{\circ }}^\delta,\tilde a] \tilde f ( y,t)$![]() where $y=\delta ^{-N} ( \tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } (x-t\gamma (s_{\circ }))$
where $y=\delta ^{-N} ( \tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{\intercal } (x-t\gamma (s_{\circ }))$![]() . Therefore, changing variable $x \rightarrow \delta ^{N} (\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{-\intercal }x+t\gamma (s_{\circ })$
. Therefore, changing variable $x \rightarrow \delta ^{N} (\tilde {\mathcal {L}}_{s_{\circ }}^\delta )^{-\intercal }x+t\gamma (s_{\circ })$![]() , we obtain (2.14).
, we obtain (2.14).
Combining Lemma 2.8 and the hypothesis (Theorem 2.3 with $L=N-1$![]() ), we obtain the following.
), we obtain the following.
Corollary 2.9 Suppose that Theorem 2.3 holds with $L=N-1$![]() , and $\mathfrak a$
, and $\mathfrak a$![]() , $\gamma$
, $\gamma$![]() and $\delta _\ast$
and $\delta _\ast$![]() are the same as in Lemma 2.8. Then, if $p> 2(N-1)$
are the same as in Lemma 2.8. Then, if $p> 2(N-1)$![]() , for $0<\delta <\delta _*$
, for $0<\delta <\delta _*$![]() we have
we have
Proof. By (2.14) and dyadic decomposition (of $\tilde a$![]() in the Fourier side), we have
in the Fourier side), we have
for some constant $C$![]() where $\|f_\ell \|_p=\|f\|_p$
where $\|f_\ell \|_p=\|f\|_p$![]() , and $a_\ell$
, and $a_\ell$![]() are symbols of type $(2^j,N-1, \tilde B)$
are symbols of type $(2^j,N-1, \tilde B)$![]() with $C^{-1}2^k\delta ^N\le 2^j \le C2^k\delta ^N$
with $C^{-1}2^k\delta ^N\le 2^j \le C2^k\delta ^N$![]() . Once we have this, the proof is straightforward. By Lemma 2.7, $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N-1, B')$
. Once we have this, the proof is straightforward. By Lemma 2.7, $\gamma _{s_{\circ }}^\delta \in \mathfrak V^d(N-1, B')$![]() for some $B'>0$
for some $B'>0$![]() . Since $\|f_l\|_p =\|f\|_p$
. Since $\|f_l\|_p =\|f\|_p$![]() , applying Theorem 2.3 with $L=N-1$
, applying Theorem 2.3 with $L=N-1$![]() , we have
, we have
for $p> 2(N-1)$![]() . Recalling (2.7), we combine this and (2.12) to get the desired bound.
. Recalling (2.7), we combine this and (2.12) to get the desired bound.
It remains to show (2.18). In fact, after applying Lemma 2.8 we only need to adjust the support of the consequent symbol $\tilde a$![]() via by moderate decomposition and scaling. We omit details. (See the proof of [Reference Ko, Lee and Oh23, Lemma 2.8].)
via by moderate decomposition and scaling. We omit details. (See the proof of [Reference Ko, Lee and Oh23, Lemma 2.8].)
3. Decoupling inequalities for curves
In this section, we prove the decoupling inequality, which is to be used to decompose the operator $\mathcal {T}[\mathfrak a]f$![]() . In our earlier work [Reference Ko, Lee and Oh23], the averaging operator was decoupled by making use of decomposition based on a class of symbols that are adjusted to short subcurves. The same approach also works to prove Proposition 2.4. However, instead of following the previous strategy, we directly obtain a decoupling inequality associated with the conic sets:
. In our earlier work [Reference Ko, Lee and Oh23], the averaging operator was decoupled by making use of decomposition based on a class of symbols that are adjusted to short subcurves. The same approach also works to prove Proposition 2.4. However, instead of following the previous strategy, we directly obtain a decoupling inequality associated with the conic sets:
while $\{s_1,\ldots,s_L\}\subset I$![]() is a collection of $\delta$
is a collection of $\delta$![]() -separated points contained in $I$
-separated points contained in $I$![]() . More precisely, we have the following.
. More precisely, we have the following.
Theorem 3.1 Let $0<\delta \le 1$![]() and $S:=\{s_1,\dots,s_L\}\subset I$
and $S:=\{s_1,\dots,s_L\}\subset I$![]() be a collection of $\delta$
be a collection of $\delta$![]() -separated points. Then, if $2\le p\le N(N+1)$
-separated points. Then, if $2\le p\le N(N+1)$![]() , for any $\epsilon >0$
, for any $\epsilon >0$![]() there is a constant $C_\epsilon =C_\epsilon (B)$
there is a constant $C_\epsilon =C_\epsilon (B)$![]() , independent of $S$
, independent of $S$![]() , such that
, such that
holds whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l)$![]() .
.
Hölder's inequality gives $\big\| \sum _{1 \le l\le L} f_l \big\|_{p}\le C_\epsilon \delta ^{-\epsilon } \delta ^{1/p-1/2} (\sum _{1 \le l\le L} \|f_l\|_{p}^p)^{1/p}$![]() . Interpolation with the trivial $L^\infty -\ell ^\infty L^\infty$
. Interpolation with the trivial $L^\infty -\ell ^\infty L^\infty$![]() estimate yields the inequality:
estimate yields the inequality:
for $p>2N$![]() whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l)$
whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l)$![]() .
.
3.1 Decoupling inequalities for curves
Fixing $N\ge 2$![]() , we now consider the slabs given by an anisotropic neighbourhood of the moment curve
, we now consider the slabs given by an anisotropic neighbourhood of the moment curve
Definition 3.2 Let $0<\delta \le 1$![]() and $B\ge 1$
and $B\ge 1$![]() . For $s \in I$
. For $s \in I$![]() , let $\mathbf {S}(s,\delta, B)$
, let $\mathbf {S}(s,\delta, B)$![]() denote the set of $(\tau,\xi ) \in \mathbb {R} \times \mathbb {R}^N$
denote the set of $(\tau,\xi ) \in \mathbb {R} \times \mathbb {R}^N$![]() such that
such that
We now recall the decoupling inequality for such slabs as above which was shown in [Reference Beltran, Guo, Hickman and Seeger1, Reference Bourgain, Demeter and Guth6] (see also [Reference Ko, Lee and Oh23, Corollary 2.15]).
Theorem 3.3 Let $0<\delta \le 1$![]() and $\{s_1, \ldots, s_L\}\subset I$
and $\{s_1, \ldots, s_L\}\subset I$![]() be a collection of $\delta$
be a collection of $\delta$![]() -separated points contained in $I$
-separated points contained in $I$![]() . Denote $\mathbf {S}_l=\mathbf {S}(s_l,\delta, B)$
. Denote $\mathbf {S}_l=\mathbf {S}(s_l,\delta, B)$![]() . Then, if $2 \le p\le N(N+1)$
. Then, if $2 \le p\le N(N+1)$![]() , for any $\epsilon >0$
, for any $\epsilon >0$![]() there is a constant $C_\epsilon =C_\epsilon (B)$
there is a constant $C_\epsilon =C_\epsilon (B)$![]() such that
such that
holds whenever $\operatorname {supp} \widehat {F}_{l}\subset \mathbf {S}_l$![]() .
.
To show Theorem 3.1, we apply the decoupling inequality after projecting the sets $\Lambda _0(\delta,s_l)$![]() to the subspace $\mathrm V_\mu$
to the subspace $\mathrm V_\mu$![]() which is spanned by $\{G^{(0)}(\mu ) ,\dots, G^{(N)}(\mu )\}$
which is spanned by $\{G^{(0)}(\mu ) ,\dots, G^{(N)}(\mu )\}$![]() . To do so, for $\mu \in I$
. To do so, for $\mu \in I$![]() we consider a coordinate system $\mathbf {y}_\mu =\mathbf {y}_\mu (\tau,\xi )$
we consider a coordinate system $\mathbf {y}_\mu =\mathbf {y}_\mu (\tau,\xi )$![]() given by
given by
Recall that $\gamma \in \mathfrak V^d(N,B)$![]() , so ${\rm Vol}(\langle G^{(0)}(\mu ), \ldots, G^{(N)}(\mu ))\ge 1/B$
, so ${\rm Vol}(\langle G^{(0)}(\mu ), \ldots, G^{(N)}(\mu ))\ge 1/B$![]() . Let $\delta, \delta '$
. Let $\delta, \delta '$![]() be positive numbers satisfying
be positive numbers satisfying
Then, it is easy to see that
The following lemma shows that the projections of the sets $\Lambda _0(\delta,s_l)$![]() form a reverse $\delta /\delta '$
form a reverse $\delta /\delta '$![]() -adapted cover after a proper linear change of variables (cf. [Reference Ko, Lee and Oh23, Lemma 3.3]) if $s_l$
-adapted cover after a proper linear change of variables (cf. [Reference Ko, Lee and Oh23, Lemma 3.3]) if $s_l$![]() are contained in an interval of length $\delta '$
are contained in an interval of length $\delta '$![]() . Let $\mathrm D_\delta$
. Let $\mathrm D_\delta$![]() denote the $(N+1)\times (N+1)$
denote the $(N+1)\times (N+1)$![]() diagonal matrix given by
diagonal matrix given by
Lemma 3.4 Let $\delta, \delta '$![]() be positive numbers satisfying (3.4) and $s' \in [\mu -\delta ',\mu +\delta ']$
be positive numbers satisfying (3.4) and $s' \in [\mu -\delta ',\mu +\delta ']$![]() . Suppose $(\tau,\xi ) \in \Lambda _0(\delta,s')$
. Suppose $(\tau,\xi ) \in \Lambda _0(\delta,s')$![]() . Then, we have
. Then, we have
Proof. Note that (3.6) is clear from (2.6). To prove (3.7), we first note that $\langle \mathbf {y}_\mu,\gamma _{\vphantom {1}\circ }^{(j)}(s) \rangle =(\delta ')^{N+1-j} \langle \mathrm D_{\delta '} \mathbf {y}_\mu, \gamma _{\vphantom {1}\circ }^{(j)}(s/\delta ') \rangle$![]() . Thus, it is sufficient to show that
. Thus, it is sufficient to show that
for $1 \le j \le N$![]() . Recalling (3.3), we observe
. Recalling (3.3), we observe

Taylor's theorem gives

for $j=1,\dots,N$![]() . Since $|s'-\mu |\le \delta '$
. Since $|s'-\mu |\le \delta '$![]() and $(\tau,\xi ) \in \Lambda _0(\delta,s')$
and $(\tau,\xi ) \in \Lambda _0(\delta,s')$![]() , (3.8) follows by (3.5).
, (3.8) follows by (3.5).
By Lemma 3.4 and Theorem 3.3, we can show that (3.1) holds if a $\delta$![]() -separated set $\{s_1, \dots, s_L\}$
-separated set $\{s_1, \dots, s_L\}$![]() is contained in an interval of length $\lesssim \delta ^{N/(N+1)}$
is contained in an interval of length $\lesssim \delta ^{N/(N+1)}$![]() . More precisely, we have the following.
. More precisely, we have the following.
Lemma 3.5 Let $0<\delta \le 1$![]() and $\delta \le \delta '\le \delta ^{N/(N+1)}$
and $\delta \le \delta '\le \delta ^{N/(N+1)}$![]() . Let $\{s_1 ,\ldots,s_L \}\subset [\mu -\delta ' ,\mu +\delta ' ]$
. Let $\{s_1 ,\ldots,s_L \}\subset [\mu -\delta ' ,\mu +\delta ' ]$![]() be a collection of $\delta$
be a collection of $\delta$![]() -separated points. Then, if $2\le p\le N(N+1)$
-separated points. Then, if $2\le p\le N(N+1)$![]() , for any $\epsilon >0$
, for any $\epsilon >0$![]() there is a constant $C_\epsilon =C_\epsilon (B)$
there is a constant $C_\epsilon =C_\epsilon (B)$![]() such that (3.1) holds whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l )$
such that (3.1) holds whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l )$![]() .
.
Proof. Set $\mathrm V_\mu =\operatorname {span} \{ \gamma '(\mu ),\dots, \gamma ^{(N)}(\mu )\}$![]() and let $\{v_{N+1},\ldots,v_d\}$
and let $\{v_{N+1},\ldots,v_d\}$![]() be an orthonormal basis of $\mathrm V_\mu ^\perp$
be an orthonormal basis of $\mathrm V_\mu ^\perp$![]() . Recalling that (2.3) holds with $L=N$
. Recalling that (2.3) holds with $L=N$![]() , we write $\xi =\overline \xi +\sum _{j=N+1}^d y_j(\xi )v_j$
, we write $\xi =\overline \xi +\sum _{j=N+1}^d y_j(\xi )v_j$![]() for $\overline \xi \in \mathrm V_\mu$
for $\overline \xi \in \mathrm V_\mu$![]() . Changing variables
. Changing variables
(see (3.3)), we may work with the coordinate system given by $\{ \mathbf {y}_\mu,y_{N+1},\ldots,y_d\}$![]() instead of $(\tau,\xi )$
instead of $(\tau,\xi )$![]() . We consider the linear map
. We consider the linear map
Since $\{s_1 ,\dots,s_L \}\subset [\mu -\delta ',\mu +\delta ']$![]() and $\delta '\le \delta ^{N/(N+1)}$
and $\delta '\le \delta ^{N/(N+1)}$![]() , by Lemma 3.4 it follows that
, by Lemma 3.4 it follows that
for some $C>0$![]() depending only on $B$
depending only on $B$![]() . Applying Theorem 3.3 with $\delta$
. Applying Theorem 3.3 with $\delta$![]() replaced by $C\delta /\delta '$
replaced by $C\delta /\delta '$![]() and slabs $\mathbf {S}_l, 1\le l\le L$
and slabs $\mathbf {S}_l, 1\le l\le L$![]() , and then using a trivial extension via Minkowski's inequality, we have
, and then using a trivial extension via Minkowski's inequality, we have
for $2\le p \le N(N+1)$![]() whenever $\operatorname {supp} \widehat f_l\subset \mathbf {S}_l \times \mathbb {R}^{d-N}$
whenever $\operatorname {supp} \widehat f_l\subset \mathbf {S}_l \times \mathbb {R}^{d-N}$![]() . Since the decoupling inequality is invariant under affine changes of variables, by undoing the change of variables $(\tau,\xi ) \rightarrow \mathrm Y_\mu (\tau,\xi )$
. Since the decoupling inequality is invariant under affine changes of variables, by undoing the change of variables $(\tau,\xi ) \rightarrow \mathrm Y_\mu (\tau,\xi )$![]() and rescaling $(\tau,\xi ) \rightarrow 2^{-k}(\tau,\xi )$
and rescaling $(\tau,\xi ) \rightarrow 2^{-k}(\tau,\xi )$![]() , we obtain (3.1) whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l )$
, we obtain (3.1) whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l )$![]() .
.
3.2 Proof of Theorem 3.1
We now prove Theorem 3.1. Let $2 \le p\le N(N+1)$![]() . For the purpose, for some $\alpha >0$
. For the purpose, for some $\alpha >0$![]() we assume that
we assume that
holds for $0<\delta \le \delta _0:= (2^2B)^{-N-1}$![]() with a constant $C$
with a constant $C$![]() , independent of $S$
, independent of $S$![]() , whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l)$
, whenever $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l)$![]() , $1 \le l\le L$
, $1 \le l\le L$![]() . Of course, ($\mathfrak{D}(\alpha)$
. Of course, ($\mathfrak{D}(\alpha)$![]() ) holds true if $\alpha \ge 1/2$
) holds true if $\alpha \ge 1/2$![]() by Minkowski's and Hölder's inequalities. We set
by Minkowski's and Hölder's inequalities. We set
Let us denote $I _\nu$![]() , $1\le \nu \le M$
, $1\le \nu \le M$![]() , be disjoint intervals of length $\rho \in (2^{-3}\delta ', 2^{-2}\delta ']$
, be disjoint intervals of length $\rho \in (2^{-3}\delta ', 2^{-2}\delta ']$![]() which partition $I$
which partition $I$![]() . Let $s _\nu '$
. Let $s _\nu '$![]() be a point contained in $I _\nu$
be a point contained in $I _\nu$![]() such that $s_1', \dots, s_M'$
such that $s_1', \dots, s_M'$![]() are separated at least by $2^{-4}\delta '$
are separated at least by $2^{-4}\delta '$![]() . We now claim that
. We now claim that
if $s_l\in I _\nu$![]() . Indeed, by scaling it is sufficient to show $\Lambda _0(\delta,s_l )\subset \Lambda _0(\delta ',s' _\nu ).$
. Indeed, by scaling it is sufficient to show $\Lambda _0(\delta,s_l )\subset \Lambda _0(\delta ',s' _\nu ).$![]() Let $(\tau,\xi )\in \Lambda _0(\delta,s_l )$
Let $(\tau,\xi )\in \Lambda _0(\delta,s_l )$![]() . Then, it follows that $|\langle G^{(\ell )}(s_l),(\tau,\xi )\rangle |\le 2^5B \delta ^{1/(N+1)}(\delta ')^{N-\ell }$
. Then, it follows that $|\langle G^{(\ell )}(s_l),(\tau,\xi )\rangle |\le 2^5B \delta ^{1/(N+1)}(\delta ')^{N-\ell }$![]() . By Taylor's theorem we have
. By Taylor's theorem we have

where $|\mathcal {E}|\le 2B|s'_\nu -s_l|^{N-j}$![]() . Therefore, we see that $(\tau,\xi )\in \Lambda _0(\delta ',s'_\nu )$
. Therefore, we see that $(\tau,\xi )\in \Lambda _0(\delta ',s'_\nu )$![]() .
.
Let $\operatorname {supp} \widehat {f}_{l}\subset \Lambda _k(\delta,s_l)$![]() , $1 \le l\le L$
, $1 \le l\le L$![]() . We write $\sum _{1\le l\le L}f_l=\sum _{1\le \nu \le M}\sum _{s_l\in I_\nu } f_l$
. We write $\sum _{1\le l\le L}f_l=\sum _{1\le \nu \le M}\sum _{s_l\in I_\nu } f_l$![]() . By (3.10), the Fourier support of $\sum _{s_l\in I_\nu } f_l$
. By (3.10), the Fourier support of $\sum _{s_l\in I_\nu } f_l$![]() is included in $\Lambda _k(\delta ',s' _\nu )$
is included in $\Lambda _k(\delta ',s' _\nu )$![]() . Since $s'_\nu$
. Since $s'_\nu$![]() are separated by $2^{-4}\delta '$
are separated by $2^{-4}\delta '$![]() , ($\mathfrak{D}(\alpha)$
, ($\mathfrak{D}(\alpha)$![]() ) implies
) implies
for a constant $C$![]() . Since the length of interval $I_\nu$
. Since the length of interval $I_\nu$![]() is less than $\delta ^{N/(N+1)},$
is less than $\delta ^{N/(N+1)},$![]() by Lemma 3.5 we have $\|\sum _{s_l\in I_\nu } f_l\|_{p}\le C_\epsilon \delta ^{-\epsilon } (\sum _{s_l\in I_\nu }\| f_l\|_{p}^2 )^{1/2}$
by Lemma 3.5 we have $\|\sum _{s_l\in I_\nu } f_l\|_{p}\le C_\epsilon \delta ^{-\epsilon } (\sum _{s_l\in I_\nu }\| f_l\|_{p}^2 )^{1/2}$![]() . Therefore, combining this and the above inequality, we obtain
. Therefore, combining this and the above inequality, we obtain
for a constant $C_\epsilon$![]() . This establishes the implication $\mathfrak D(\alpha )\to \mathfrak D(\epsilon + {N\alpha }/(N+1))$
. This establishes the implication $\mathfrak D(\alpha )\to \mathfrak D(\epsilon + {N\alpha }/(N+1))$![]() . Iteration of this implication suppresses $\alpha$
. Iteration of this implication suppresses $\alpha$![]() arbitrarily small.
arbitrarily small.
4. Proof of Proposition 2.4
In this section, we prove Proposition 2.4 by making use of the decoupling inequality (3.2). As mentioned in § 2.1 (below Proposition 2.4), in order to prove Proposition 2.4, it suffices to show Theorem 2.3 with $L=N$![]() . We first reduce the matter to obtaining estimates for $\mathcal {T}[\mathfrak a_0]$
. We first reduce the matter to obtaining estimates for $\mathcal {T}[\mathfrak a_0]$![]() with a suitable $\mathfrak a_0$
with a suitable $\mathfrak a_0$![]() .
.
4.1 Reduction
We begin by recalling $\gamma \in \mathfrak V^d(N,B)$![]() and $a$
and $a$![]() is of type $(2^k,N,B)$
is of type $(2^k,N,B)$![]() . Let $\delta _*$
. Let $\delta _*$![]() be the small number given in Lemma 2.8 and set
be the small number given in Lemma 2.8 and set
Let $\beta _0\in \mathrm C_0^\infty ([-1,1])$![]() such that $\beta _0= 1$
such that $\beta _0= 1$![]() on $[-1/2,1/2]$
on $[-1/2,1/2]$![]() . We set
. We set
Clearly, (2.4) holds on $\operatorname {supp} (a-a_N)$![]() with $L=N-1$
with $L=N-1$![]() and $B$
and $B$![]() replaced by $100\,dB\delta _{\circ }^{-N}$
replaced by $100\,dB\delta _{\circ }^{-N}$![]() . Since $a$
. Since $a$![]() is of type $(2^k,N,B)$
is of type $(2^k,N,B)$![]() , it is easy to see $(a-a_N)$
, it is easy to see $(a-a_N)$![]() is a symbol of type $(2^k,N-1,B')$
is a symbol of type $(2^k,N-1,B')$![]() for some $B'$
for some $B'$![]() . Thus, the hypothesis (Theorem 2.3 with $L=N-1$
. Thus, the hypothesis (Theorem 2.3 with $L=N-1$![]() and $B=B'$
and $B=B'$![]() ) gives the estimate
) gives the estimate
for $p> 2(N-1)$![]() . So, we need only to consider $\mathcal {R}[a_N]$
. So, we need only to consider $\mathcal {R}[a_N]$![]() instead of $\mathcal {R}[a]$
instead of $\mathcal {R}[a]$![]() . Furthermore, by a moderate decomposition of $a_N$
. Furthermore, by a moderate decomposition of $a_N$![]() , we assume
, we assume
for some $s_{\circ } \in (-1,1)$![]() . We may assume that $s_{\circ }=\delta _{\circ }\nu$
. We may assume that $s_{\circ }=\delta _{\circ }\nu$![]() for $\nu \in \mathbb {Z}$
for $\nu \in \mathbb {Z}$![]() .
.
It is not difficult to see that the contribution of the frequency part $\{(\tau,\xi ): |\tau +\gamma (s)\cdot \xi | \gtrsim 2^{k+1} \delta _{\circ }^N, \, \forall s \in I\}$![]() is not significant. To see this, let us set
is not significant. To see this, let us set
and $\mathfrak a_1=\mathfrak a_0-a_N$![]() . Recalling (2.10), by Fourier inversion we have
. Recalling (2.10), by Fourier inversion we have
The operator $\mathcal {T}[\mathfrak a_1]$![]() is easy to handle. Let us set $\mathfrak a=-i2^{k}\delta _{\circ }^{N}(\tau +\langle \gamma (s), \xi \rangle )^{-1} \partial _{t}\mathfrak a_1$
is easy to handle. Let us set $\mathfrak a=-i2^{k}\delta _{\circ }^{N}(\tau +\langle \gamma (s), \xi \rangle )^{-1} \partial _{t}\mathfrak a_1$![]() . Then, by integration by parts in $t'$
. Then, by integration by parts in $t'$![]() and (2.10) we see $\mathcal {T} [\mathfrak a_1]=(2^{k}\delta _{\circ }^{N})^{-1}\mathcal {T} [\mathfrak a]$
and (2.10) we see $\mathcal {T} [\mathfrak a_1]=(2^{k}\delta _{\circ }^{N})^{-1}\mathcal {T} [\mathfrak a]$![]() . Note that $|\tau +\gamma (s)\cdot \xi | \gtrsim 2^{k} \delta _{\circ }^N$
. Note that $|\tau +\gamma (s)\cdot \xi | \gtrsim 2^{k} \delta _{\circ }^N$![]() on $\operatorname {supp} \mathfrak a_1$
on $\operatorname {supp} \mathfrak a_1$![]() and so on $\operatorname {supp} \mathfrak a$
and so on $\operatorname {supp} \mathfrak a$![]() . It is clear that $\mathfrak a$
. It is clear that $\mathfrak a$![]() satisfies (2.8) and (2.9) with $\delta =\delta _{\circ }$
satisfies (2.8) and (2.9) with $\delta =\delta _{\circ }$![]() and $B=C_1\delta _{\circ }^{-C}$
and $B=C_1\delta _{\circ }^{-C}$![]() for some large $C,C_1$
for some large $C,C_1$![]() . Thus, Lemma 2.6 gives $\|\mathcal {T} [ \mathfrak a_1]f\|_p\lesssim 2^{-k}\|f\|_p$
. Thus, Lemma 2.6 gives $\|\mathcal {T} [ \mathfrak a_1]f\|_p\lesssim 2^{-k}\|f\|_p$![]() for $p\ge 2.$
for $p\ge 2.$![]()
Therefore, the proof of Theorem 2.3 with $L=N$![]() is now reduced to show that
is now reduced to show that
4.2 Decomposition
For $n\ge 0$![]() , let us set $\delta _n=2^n2^{-k/N}$
, let us set $\delta _n=2^n2^{-k/N}$![]() and
and
We consider
by which we can decompose $\mathfrak a_0$![]() into the symbols contained in $\mathfrak A_k(\delta _n,s)$
into the symbols contained in $\mathfrak A_k(\delta _n,s)$![]() for $s\in \mathfrak J_n$
for $s\in \mathfrak J_n$![]() .
.
Set $\beta _\ast =\beta _0-\beta _0(2^{2N!}\cdot )$![]() . Note that $\beta _0+\sum _{n\ge 1} \beta _\ast (2^{-2N! n} \cdot )=1$
. Note that $\beta _0+\sum _{n\ge 1} \beta _\ast (2^{-2N! n} \cdot )=1$![]() . Let $\zeta \in \mathrm C_0^{\infty }([-1,1])$
. Let $\zeta \in \mathrm C_0^{\infty }([-1,1])$![]() such that $\sum _{\nu \in \mathbb {Z}} \zeta (\cdot -\nu )=1$
such that $\sum _{\nu \in \mathbb {Z}} \zeta (\cdot -\nu )=1$![]() . We set
. We set

Then, it follows that
Since $\delta _{\circ }$![]() is the fixed constant, it is clear that $C^{-1} \mathfrak a_0 \in \mathfrak A_k(\delta _{\circ },s_{\circ })$
is the fixed constant, it is clear that $C^{-1} \mathfrak a_0 \in \mathfrak A_k(\delta _{\circ },s_{\circ })$![]() for a large constant $C>0$
for a large constant $C>0$![]() . So, $\operatorname {supp} \mathfrak a_0 \subset \Lambda _k(\delta _{\circ },s_{\circ })$
. So, $\operatorname {supp} \mathfrak a_0 \subset \Lambda _k(\delta _{\circ },s_{\circ })$![]() and $\mathfrak G_N\lesssim 1$
and $\mathfrak G_N\lesssim 1$![]() for $(\tau,\xi )\in \operatorname {supp} \mathfrak a_\nu ^n$
for $(\tau,\xi )\in \operatorname {supp} \mathfrak a_\nu ^n$![]() . Obviously, we may assume $\delta _n\lesssim 1$
. Obviously, we may assume $\delta _n\lesssim 1$![]() since $\mathfrak a_\nu ^n=0$
since $\mathfrak a_\nu ^n=0$![]() otherwise.
otherwise.
The following tells that $\mathfrak a_{\nu }^{n}$![]() is contained in a proper symbol class.
is contained in a proper symbol class.
Lemma 4.1 (cf. [Reference Ko, Lee and Oh23, Lemma 3.2])
For $n\ge 0$![]() , there exists a constant $C$
, there exists a constant $C$![]() such that $C^{-1}\mathfrak a_{\nu }^{n}\in \mathfrak A_k(\delta _n,\delta _n\nu )$
such that $C^{-1}\mathfrak a_{\nu }^{n}\in \mathfrak A_k(\delta _n,\delta _n\nu )$![]() .
.
Proof. Condition (2.8) trivially holds for $\mathfrak a=\mathfrak a_\nu ^n$![]() . So, we only need to show (2.9) for $\delta =\delta _n$
. So, we only need to show (2.9) for $\delta =\delta _n$![]() and $s=\delta _n\nu$
and $s=\delta _n\nu$![]() .
.
It is not difficult to see that $\mathfrak a_0$![]() satisfies (2.9) (see [Reference Ko, Lee and Oh23, (3.35)]). So it suffices to show (2.9) for $\beta _N(\delta _n^{-2N!}\mathfrak G_N(s,\tau,\xi ))$
satisfies (2.9) (see [Reference Ko, Lee and Oh23, (3.35)]). So it suffices to show (2.9) for $\beta _N(\delta _n^{-2N!}\mathfrak G_N(s,\tau,\xi ))$![]() . By Leibniz's rule, it is enough to prove that
. By Leibniz's rule, it is enough to prove that
for $j=0,\ldots,d-1$![]() . Note that if $|\delta _n\nu -s|\le \delta _n$
. Note that if $|\delta _n\nu -s|\le \delta _n$![]() , then
, then
(see [Reference Ko, Lee and Oh23, Lemma 2.6]). Note that $\nabla _{\tau,\xi }\langle G^{(j)}(s), {\mathcal {L}}_{\delta _n\nu }^{\delta _n}(\tau, \xi )\rangle = ({\mathcal {L}}_{\delta _n\nu }^{\delta _n})^\intercal G^{(j)}(s)$![]() . Thus, by (4.6) we get (4.5).
. Thus, by (4.6) we get (4.5).
4.3 Proof of Proposition 2.4
By the reduction in § 4.1, it suffices to prove (4.2). Recalling (4.4) and applying the Minkowski inequality, we have
Using Lemma 4.1, one can easily see that $\operatorname {supp} \mathfrak a_{\nu }^{n}\subset \Lambda _k(\delta _n, \delta _n\nu )$![]() . Thus, we may use the decoupling inequality (3.2). Combining this and the above inequalities gives
. Thus, we may use the decoupling inequality (3.2). Combining this and the above inequalities gives
for $2N< p<\infty$![]() . Hence, for estimate (4.2) it suffice to show that
. Hence, for estimate (4.2) it suffice to show that
Indeed, let $f_\nu (x,s)=\tilde \zeta (\delta _n^{-1}s-\nu ) f(x,s)$![]() where $\tilde \zeta \in \mathrm C_0^\infty ([-2,2])$
where $\tilde \zeta \in \mathrm C_0^\infty ([-2,2])$![]() such that $\tilde \zeta =1$
such that $\tilde \zeta =1$![]() on $\operatorname {supp} \zeta$
on $\operatorname {supp} \zeta$![]() . From (2.10) we see $\mathcal {T} [\mathfrak a_{\nu }^{n}]f=\mathcal {T} [\mathfrak a_{\nu }^{n}]f_\nu$
. From (2.10) we see $\mathcal {T} [\mathfrak a_{\nu }^{n}]f=\mathcal {T} [\mathfrak a_{\nu }^{n}]f_\nu$![]() . Combining this and (4.7), we have
. Combining this and (4.7), we have

Therefore, taking the sum over $n$![]() , we get (4.2), which proves Proposition 2.4.
, we get (4.2), which proves Proposition 2.4.
It remains to prove (4.7). By Lemma 4.1, we have $C^{-1}\mathfrak a_{\nu }^{n} \in \mathfrak A_k(\delta _n,\delta _n\nu )$![]() for a constant $C>0$
for a constant $C>0$![]() . For $n=0$
. For $n=0$![]() , it is easy to show (4.7). Since $\delta _0=2^{-k/N}$
, it is easy to show (4.7). Since $\delta _0=2^{-k/N}$![]() , applying Lemma 2.6, we get
, applying Lemma 2.6, we get
For $n\ge 1$![]() , we need to decompose $\mathfrak a_{\nu }^{n}$
, we need to decompose $\mathfrak a_{\nu }^{n}$![]() further. Let us set
further. Let us set
and $\mathfrak a_{\nu,0}^{n} = \mathfrak a_{\nu }^{n}-\mathfrak a_{\nu,1}^{n}$![]() , so we have $\mathfrak a_{\nu }^{n} = \mathfrak a_{\nu,1}^{n}+\mathfrak a_{\nu,0}^{n}.$
, so we have $\mathfrak a_{\nu }^{n} = \mathfrak a_{\nu,1}^{n}+\mathfrak a_{\nu,0}^{n}.$![]() We note that $C^{-1} \mathfrak a_{\nu,i}^{n} \in \mathfrak A_k(\delta _n,\delta _n\nu )$
We note that $C^{-1} \mathfrak a_{\nu,i}^{n} \in \mathfrak A_k(\delta _n,\delta _n\nu )$![]() , $i=0,1$
, $i=0,1$![]() for some $C>0$
for some $C>0$![]() . This can be shown by following the proof of Lemma 4.1. So, we omit the detail.
. This can be shown by following the proof of Lemma 4.1. So, we omit the detail.
We now decompose $\mathcal {T} [\mathfrak a_{\nu }^{n}]f=\mathcal {T} [\mathfrak a_{\nu,1}^{n}]f+\mathcal {T} [\mathfrak a_{\nu,0}^{n}]f.$![]() For (4.7), it suffices to show
For (4.7), it suffices to show
for $p>2N-2$![]() . It is clear that (2.13) holds with $\delta =\delta _n$
. It is clear that (2.13) holds with $\delta =\delta _n$![]() , $s_{\circ }=\delta _n\nu$
, $s_{\circ }=\delta _n\nu$![]() , and some large $B$
, and some large $B$![]() on $\operatorname {supp} \mathfrak a_{\nu,1}^{n}$
on $\operatorname {supp} \mathfrak a_{\nu,1}^{n}$![]() . By Corollary 2.9, we have (4.8) for $i=1$
. By Corollary 2.9, we have (4.8) for $i=1$![]() if $p>2N-2$
if $p>2N-2$![]() . The operator $\mathcal {T} [\mathfrak a_{\nu,0}^{n}]$
. The operator $\mathcal {T} [\mathfrak a_{\nu,0}^{n}]$![]() can be handled in the same manner as $\mathcal {T}[\mathfrak a_1]$
can be handled in the same manner as $\mathcal {T}[\mathfrak a_1]$![]() since
since
holds on $\operatorname {supp} \mathfrak a_{\nu,0}^{n}$![]() . We set $\mathfrak a=-i2^{k}\delta _n^{N}(\tau +\langle \gamma (s), \xi \rangle )^{-1} \partial _{t}\mathfrak a_{\nu,0}^{n}$
. We set $\mathfrak a=-i2^{k}\delta _n^{N}(\tau +\langle \gamma (s), \xi \rangle )^{-1} \partial _{t}\mathfrak a_{\nu,0}^{n}$![]() . Integration by parts in $t'$
. Integration by parts in $t'$![]() and (2.10) yields $\mathcal {T} [\mathfrak a_{\nu,0}^{n}]=(2^{k}\delta _n^{N})^{-1}\mathcal {T} [\mathfrak a]$
and (2.10) yields $\mathcal {T} [\mathfrak a_{\nu,0}^{n}]=(2^{k}\delta _n^{N})^{-1}\mathcal {T} [\mathfrak a]$![]() . Using (4.9) and the fact that $C^{-1} \mathfrak a_{\nu,0}^{n} \in \mathfrak A_k(\delta _n,\delta _n\nu )$
. Using (4.9) and the fact that $C^{-1} \mathfrak a_{\nu,0}^{n} \in \mathfrak A_k(\delta _n,\delta _n\nu )$![]() for some $C>0$
for some $C>0$![]() , one can easily verify that (2.8) and (2.9) hold for $\mathfrak a$
, one can easily verify that (2.8) and (2.9) hold for $\mathfrak a$![]() with $\delta =\delta _n$
with $\delta =\delta _n$![]() , $s_{\circ }=\delta _n\nu$
, $s_{\circ }=\delta _n\nu$![]() . Thus, by Lemma 2.6, we have
. Thus, by Lemma 2.6, we have
for $p\ge 2$![]() , which gives (4.8) for $i=0$
, which gives (4.8) for $i=0$![]() . For the second inequality we use the fact that $\delta _n\ge 2^{-k/N}$
. For the second inequality we use the fact that $\delta _n\ge 2^{-k/N}$![]() .
.
5. Proof of Theorem 1.2
We first prove the sufficiency part, that is to say, estimate (1.2) with $\alpha =1-1/p$![]() for $1\le p< p_d$
for $1\le p< p_d$![]() by making use of Theorem 2.3.
by making use of Theorem 2.3.
5.1 Proof of estimate (1.2) with $\alpha =1-1/p$
We make use of the argument in [Reference Pramanik, Rogers and Seeger28, Reference Pramanik and Seeger31]. As mentioned before, it suffices to prove (2.1) by duality. Let $P_k$![]() denote the (Littlewood–Paley projection) operator defined by
denote the (Littlewood–Paley projection) operator defined by
for $\beta \in \mathrm C_0^\infty ([1/2,2])$![]() . Recall that $\beta _0\in \mathrm C_0^\infty ([-1,1])$
. Recall that $\beta _0\in \mathrm C_0^\infty ([-1,1])$![]() such that $\beta _0= 1$
such that $\beta _0= 1$![]() on $[-1/2,1/2]$
on $[-1/2,1/2]$![]() and set $\beta _\ast (t)=\beta _0(C_0^{-1}2^{-6}t)-\beta _0(C_0 2^{6}t)$
and set $\beta _\ast (t)=\beta _0(C_0^{-1}2^{-6}t)-\beta _0(C_0 2^{6}t)$![]() . Here, $C_0=1+2\sup \{|\gamma (s)|+|\gamma '(s)|: s \in \operatorname {supp} \psi \}$
. Here, $C_0=1+2\sup \{|\gamma (s)|+|\gamma '(s)|: s \in \operatorname {supp} \psi \}$![]() . Let $f_k$
. Let $f_k$![]() be given by
be given by
We claim that
for $p>2d$![]() and $M\gg 1$
and $M\gg 1$![]() . Then, (2.1) follows by the Littlewood–Paley inequality.
. Then, (2.1) follows by the Littlewood–Paley inequality.
Let $\tilde \beta =\beta _0(2^{-3}\,\cdot )-\beta _0(C_02^{3}\,\cdot )$![]() Considering an operator $\mathcal {R}_k$
Considering an operator $\mathcal {R}_k$![]() given by
given by
we decompose
In what follows, we show that the contributions from the second and third terms are negligible. In fact, for any $M\ge 1$![]() if $p\ge 1$
if $p\ge 1$![]() , we have
, we have
and (5.4).
To see (5.3), note $\mathcal {F}_x(\mathcal {R}_k g)(\xi,t')=\int m(\xi,t',u) \widehat g(\xi,u)\,{\rm d}u$![]() where
where
Set $g=f-f_k$![]() . Since $|(\xi,u)|\ge C_0 2^{k+5}$
. Since $|(\xi,u)|\ge C_0 2^{k+5}$![]() or $|(\xi,u)| \le C_0^{-1}2^{k-5}$
or $|(\xi,u)| \le C_0^{-1}2^{k-5}$![]() on $\operatorname {supp} \mathcal {F} (f-f_k)$
on $\operatorname {supp} \mathcal {F} (f-f_k)$![]() , we have $|u| \ge C_0 |\xi |$
, we have $|u| \ge C_0 |\xi |$![]() if $C_0^{-1}2^{k-4}\le |\xi | \le 2^{k+3}$
if $C_0^{-1}2^{k-4}\le |\xi | \le 2^{k+3}$![]() . Therefore, integration by parts gives
. Therefore, integration by parts gives
for any $\alpha$![]() and $N\ge 1$
and $N\ge 1$![]() . Note that $\textstyle P_k \mathcal {R}_kg(x,t)=\int K(x,y,t,s') g(y,s')\,{\rm d}y{\rm d}s'$
. Note that $\textstyle P_k \mathcal {R}_kg(x,t)=\int K(x,y,t,s') g(y,s')\,{\rm d}y{\rm d}s'$![]() where
where
Therefore, $|\partial _{\xi,u}^\alpha m(\xi,t',u)| \lesssim 2^{-kN} 2^{-jN}$![]() for $(\xi,u) \in \operatorname {supp} \widehat g$
for $(\xi,u) \in \operatorname {supp} \widehat g$![]() and integration by parts shows
and integration by parts shows
Decomposing $\mathcal {R}_k(f-f_k)=\sum _{j}\mathcal {R}_kP_j(f-f_k)$![]() , we get (5.3) for any $M\ge 1$
, we get (5.3) for any $M\ge 1$![]() and $p\ge 1$
and $p\ge 1$![]() .
.
We now show
for $p\ge 1$![]() and $M\ge 1$
and $M\ge 1$![]() . We write $\mathcal {F}(\mathcal {R}f-\mathcal {R}_kf)(\xi,\tau )=\int b(s,\xi,\tau )\mathcal {F}_xf(\xi,s)\,{\rm d}s$
. We write $\mathcal {F}(\mathcal {R}f-\mathcal {R}_kf)(\xi,\tau )=\int b(s,\xi,\tau )\mathcal {F}_xf(\xi,s)\,{\rm d}s$![]() where
where
Since $|\xi | \le C_0^{-1}2^{k-2}$![]() or $|\xi |\ge 2^{k+2}$
or $|\xi |\ge 2^{k+2}$![]() on $\operatorname {supp} \mathcal {F}_x(\mathcal {R}f-\mathcal {R}_kf)$
on $\operatorname {supp} \mathcal {F}_x(\mathcal {R}f-\mathcal {R}_kf)$![]() , we have $|\tau | \ge C_0 |\xi |$
, we have $|\tau | \ge C_0 |\xi |$![]() if $2^{k-1} \le |(\xi,\tau )| \le 2^{k+1}$
if $2^{k-1} \le |(\xi,\tau )| \le 2^{k+1}$![]() . Integration by parts gives $|\partial _\xi ^\alpha b(s,\xi,\tau )|\lesssim 2^{-kN}$
. Integration by parts gives $|\partial _\xi ^\alpha b(s,\xi,\tau )|\lesssim 2^{-kN}$![]() for any $\alpha$
for any $\alpha$![]() and $N$
and $N$![]() . Hence,
. Hence,
for all $N\ge 1$![]() . Since $|\xi | \le C_0^{-1}2^{k-2}$
. Since $|\xi | \le C_0^{-1}2^{k-2}$![]() on $\operatorname {supp}\mathcal {F}(P_k(\mathcal {R}-\mathcal {R}_k)f)$
on $\operatorname {supp}\mathcal {F}(P_k(\mathcal {R}-\mathcal {R}_k)f)$![]() , similarly as in the proof of (5.3), we have $\|P_k(\mathcal {R} -\mathcal {R}_k) P_jf\|_{p} \lesssim 2^{-jN} \|P_j f\|_p$
, similarly as in the proof of (5.3), we have $\|P_k(\mathcal {R} -\mathcal {R}_k) P_jf\|_{p} \lesssim 2^{-jN} \|P_j f\|_p$![]() for $j\ge k+C'$
for $j\ge k+C'$![]() for some $C'\ge 1$
for some $C'\ge 1$![]() . Estimate (5.5) gives $\|P_k(\mathcal {R} -\mathcal {R}_k) P_jf\|_{p} \lesssim 2^{-kN} \|P_j f\|_p$
. Estimate (5.5) gives $\|P_k(\mathcal {R} -\mathcal {R}_k) P_jf\|_{p} \lesssim 2^{-kN} \|P_j f\|_p$![]() for $j\le k+C'$
for $j\le k+C'$![]() . Combining those estimates, we get (5.4).
. Combining those estimates, we get (5.4).
Therefore, estimate (5.1) follows if we show
for $p>2d$![]() . This can be done by using [Reference Pramanik, Rogers and Seeger28, Theorem 1] and (2.5) (also see [Reference Beltran, Guo, Hickman and Seeger1, Reference Pramanik and Seeger29, Reference Pramanik and Seeger31]). Indeed, let $\tilde \beta \in \mathrm C_c^\infty ((1/4,4))$
. This can be done by using [Reference Pramanik, Rogers and Seeger28, Theorem 1] and (2.5) (also see [Reference Beltran, Guo, Hickman and Seeger1, Reference Pramanik and Seeger29, Reference Pramanik and Seeger31]). Indeed, let $\tilde \beta \in \mathrm C_c^\infty ((1/4,4))$![]() such that $\tilde \beta \beta =\beta$
such that $\tilde \beta \beta =\beta$![]() . Consider the operator $\tilde P_k$
. Consider the operator $\tilde P_k$![]() given by $\mathcal {F}(\tilde P_kg)(\xi,\tau )=\tilde \beta (2^{-k}|(\xi,\tau )|) \widehat g(\xi,\tau )$
given by $\mathcal {F}(\tilde P_kg)(\xi,\tau )=\tilde \beta (2^{-k}|(\xi,\tau )|) \widehat g(\xi,\tau )$![]() . Note that $P_k\mathcal {R}_k f_k=P_k \tilde P_k \mathcal {R}_k f_k$
. Note that $P_k\mathcal {R}_k f_k=P_k \tilde P_k \mathcal {R}_k f_k$![]() .
.
Let us denote the centre of a cube $Q$![]() by $(x_Q,t_Q)$
by $(x_Q,t_Q)$![]() and set
and set
Since $T_k=\tilde P_k\mathcal {R}_k$![]() and $\mathcal {E}_Q$
and $\mathcal {E}_Q$![]() satisfy the assumptions in [Reference Pramanik, Rogers and Seeger28, Theorem 1], by using (2.5) we obtain (5.6). We omit the details.
satisfy the assumptions in [Reference Pramanik, Rogers and Seeger28, Theorem 1], by using (2.5) we obtain (5.6). We omit the details.
5.2 Sharpness of smoothing order
In this section, we show upper bounds on the smoothing order $\alpha$![]() for which $L^p-L_\alpha ^p$
for which $L^p-L_\alpha ^p$![]() estimate for $\mathfrak Rf$
estimate for $\mathfrak Rf$![]() holds when $\gamma$
holds when $\gamma$![]() is of maximal type $L$
is of maximal type $L$![]() . In [Reference Pramanik and Seeger31], those bounds were obtained for $d=2$
. In [Reference Pramanik and Seeger31], those bounds were obtained for $d=2$![]() . Modifying the examples in [Reference Pramanik and Seeger31], we show the following.
. Modifying the examples in [Reference Pramanik and Seeger31], we show the following.
Proposition 5.1 Let $d\ge 3$![]() , $L\ge d$
, $L\ge d$![]() and $1 \le p \le \infty$
and $1 \le p \le \infty$![]() . Let $\psi$
. Let $\psi$![]() and $\chi$
and $\chi$![]() be nontrivial, nonnegative continuous functions supported in the interiors of $I$
be nontrivial, nonnegative continuous functions supported in the interiors of $I$![]() and $[1,2]$
and $[1,2]$![]() , respectively. Suppose there is an $s_{\circ }$
, respectively. Suppose there is an $s_{\circ }$![]() such that $\psi (s_{\circ })\neq 0$
such that $\psi (s_{\circ })\neq 0$![]() and $\gamma$
and $\gamma$![]() is of type $L$
is of type $L$![]() at $s_{\circ }$
at $s_{\circ }$![]() . Then, $\mathfrak Rf$
. Then, $\mathfrak Rf$![]() maps $L^p$
maps $L^p$![]() boundedly to $L_\alpha ^p$
boundedly to $L_\alpha ^p$![]() only if
only if
In particular, the upper bound $(i)$![]() provides the necessity part of Theorem 1.2, thus, the proof Theorem 1.2 is completed. We prove the upper bounds $(i)$
provides the necessity part of Theorem 1.2, thus, the proof Theorem 1.2 is completed. We prove the upper bounds $(i)$![]() , $(ii)$
, $(ii)$![]() and $(iii)$
and $(iii)$![]() , separately.
, separately.
Proof. Proof of (i)
Let $t_{0} \in (1,2)$![]() such that $\chi (t_{0})>0$
such that $\chi (t_{0})>0$![]() . We choose $\zeta \in \mathcal {S}(\mathbb {R}^{d})$
. We choose $\zeta \in \mathcal {S}(\mathbb {R}^{d})$![]() such that $\zeta \ge 1$
such that $\zeta \ge 1$![]() on $[-1,1]^{d}$
on $[-1,1]^{d}$![]() , $\operatorname {supp} \widehat \zeta \subset [1/2,4]^d$
, $\operatorname {supp} \widehat \zeta \subset [1/2,4]^d$![]() , and $\widehat \zeta =1$
, and $\widehat \zeta =1$![]() on $[1,2]^d$
on $[1,2]^d$![]() . Let $\psi _0\in \mathrm C_c^\infty ((-1,1))$
. Let $\psi _0\in \mathrm C_c^\infty ((-1,1))$![]() satisfy $\psi _0=1$
satisfy $\psi _0=1$![]() on $[-1/2,1/2]$
on $[-1/2,1/2]$![]() . We take
. We take
where $r_0=1+\sup _{s \in I}|\gamma (s)|$![]() . Note $\mathfrak Rf(x,s) \gtrsim \lambda ^{-1}$
. Note $\mathfrak Rf(x,s) \gtrsim \lambda ^{-1}$![]() if $|x+t_{0}\gamma (s)|\le c \lambda ^{-1}$
if $|x+t_{0}\gamma (s)|\le c \lambda ^{-1}$![]() and $|s-s_{\circ }|< c$
and $|s-s_{\circ }|< c$![]() for a small constant $c>0$
for a small constant $c>0$![]() . Thus, $\| \mathfrak Rf \|_{p} \gtrsim \lambda ^{-1-d/p}$
. Thus, $\| \mathfrak Rf \|_{p} \gtrsim \lambda ^{-1-d/p}$![]() . Since
. Since
it follows that $\operatorname {supp}_\xi \mathcal {F}_x(\mathfrak Rf)$![]() is included in $\{ \xi : |\xi | \sim \lambda \}$
is included in $\{ \xi : |\xi | \sim \lambda \}$![]() . Hence, $\| \mathfrak Rf(\cdot,s) \|_{L_\alpha ^p(\mathbb {R}^{d};{\rm d}x)} \gtrsim \lambda ^{\alpha -1-d/p}$
. Hence, $\| \mathfrak Rf(\cdot,s) \|_{L_\alpha ^p(\mathbb {R}^{d};{\rm d}x)} \gtrsim \lambda ^{\alpha -1-d/p}$![]() , so we have $\| \mathfrak Rf\|_{L_\alpha ^p(\mathbb {R}^{d+1})} \gtrsim \lambda ^{\alpha -1-d/p}$
, so we have $\| \mathfrak Rf\|_{L_\alpha ^p(\mathbb {R}^{d+1})} \gtrsim \lambda ^{\alpha -1-d/p}$![]() . Since $\|f\|_p \lesssim \lambda ^{-(d+1)/p}$
. Since $\|f\|_p \lesssim \lambda ^{-(d+1)/p}$![]() , we get $\alpha \le 1-1/p$
, we get $\alpha \le 1-1/p$![]() .
.
Proof. Proof of (ii)
Let $\tilde I \subset (-1,1)$![]() be a nonempty compact interval such that (1.1) holds for $s \in \tilde I$
be a nonempty compact interval such that (1.1) holds for $s \in \tilde I$![]() . Also, we fix a constant $\rho \gg 1$
. Also, we fix a constant $\rho \gg 1$![]() to be chosen later. Let $\{s_\ell \}\subset \tilde I$
to be chosen later. Let $\{s_\ell \}\subset \tilde I$![]() be a collection of $\rho \lambda ^{-1/d}$
be a collection of $\rho \lambda ^{-1/d}$![]() -separated points which are as many as $C\rho ^{-1}\lambda ^{1/d}$
-separated points which are as many as $C\rho ^{-1}\lambda ^{1/d}$![]() . Since $G(s_\ell ), G'(s_\ell ), \ldots, G^{(d-1)}(s_\ell )$
. Since $G(s_\ell ), G'(s_\ell ), \ldots, G^{(d-1)}(s_\ell )$![]() are linearly independent in $\mathbb {R}^{d+1}$
are linearly independent in $\mathbb {R}^{d+1}$![]() , there is a unit vector $\Xi _\ell \in ( \operatorname {span}\{ G^{(j)}(s_\ell ): j=0,1,\ldots,d-1\} )^\perp$
, there is a unit vector $\Xi _\ell \in ( \operatorname {span}\{ G^{(j)}(s_\ell ): j=0,1,\ldots,d-1\} )^\perp$![]() .
.
Let $\phi \in \mathcal {S}(\mathbb {R}^{d+1})$![]() such that $\phi \ge 1$
such that $\phi \ge 1$![]() on $[-3r_0,3r_0]^{d+1}$
on $[-3r_0,3r_0]^{d+1}$![]() and $\widehat \phi$
and $\widehat \phi$![]() is supported in $[-1,1]^{d+1}$
is supported in $[-1,1]^{d+1}$![]() where $r_0=1+\sup _{s \in I} |\gamma (s)|$
where $r_0=1+\sup _{s \in I} |\gamma (s)|$![]() . Let $\varepsilon _\ell \in \{\pm 1\}$
. Let $\varepsilon _\ell \in \{\pm 1\}$![]() be independent random variables. We consider
be independent random variables. We consider
Since $\langle \Xi _\ell, G^{(j)}(s_\ell ) \rangle =0$![]() for $j=0,\dots,d-1$
for $j=0,\dots,d-1$![]() , by Taylor's theorem we have
, by Taylor's theorem we have
Thus, $|t \langle \Xi _\ell, G(s)\rangle | \le 2^{-2}\lambda ^{-1}$![]() whenever $s \in I_\ell :=\{ s \in \tilde I : |s-s_\ell | \le c \lambda ^{-1/d}\}$
whenever $s \in I_\ell :=\{ s \in \tilde I : |s-s_\ell | \le c \lambda ^{-1/d}\}$![]() for a $c>0$
for a $c>0$![]() small enough. Noting that
small enough. Noting that
we see $|\mathfrak Rf_\ell (x,s)| \gtrsim 1$![]() if $(x,s) \in B_\ell :=[-c,c]^d\times I_\ell.$
if $(x,s) \in B_\ell :=[-c,c]^d\times I_\ell.$![]() Thus, $\sum _\ell \left \| \mathfrak Rf_\ell \right \|_{L^p(B_\ell )}^p \gtrsim \rho ^{-1}.$
Thus, $\sum _\ell \left \| \mathfrak Rf_\ell \right \|_{L^p(B_\ell )}^p \gtrsim \rho ^{-1}.$![]() Meanwhile, by (5.8), (5.7) and integration by parts in $t$
Meanwhile, by (5.8), (5.7) and integration by parts in $t$![]() we have $|\mathfrak Rf_m(x,s)| \lesssim (1+\lambda |s_\ell -s_m|^{d})^{-N}$
we have $|\mathfrak Rf_m(x,s)| \lesssim (1+\lambda |s_\ell -s_m|^{d})^{-N}$![]() for any $N\ge 1$
for any $N\ge 1$![]() if $m\neq \ell$
if $m\neq \ell$![]() and $s \in I_\ell$
and $s \in I_\ell$![]() . Since $\{s_\ell \}$
. Since $\{s_\ell \}$![]() are $\rho \lambda ^{-({1}/{d})}$
are $\rho \lambda ^{-({1}/{d})}$![]() -separated, it is easy to see
-separated, it is easy to see
Therefore, taking $\rho,N$![]() sufficiently large, we have $\|\mathfrak Rf \|_p^p \gtrsim \rho ^{-1}$
sufficiently large, we have $\|\mathfrak Rf \|_p^p \gtrsim \rho ^{-1}$![]() for any choice of $\varepsilon _\ell$
for any choice of $\varepsilon _\ell$![]() .
.
By our choice of $\phi$![]() it follows that $\mathcal {F}_x (\mathfrak Rf)$
it follows that $\mathcal {F}_x (\mathfrak Rf)$![]() is supported on $\{\xi : C_1\lambda \le |\xi | \le C_2 \lambda \}$
is supported on $\{\xi : C_1\lambda \le |\xi | \le C_2 \lambda \}$![]() for some positive constant $C_1, C_2$
for some positive constant $C_1, C_2$![]() . Thus, $\| \mathfrak Rf \|_{L_\alpha ^p} \gtrsim \lambda ^\alpha \|\mathfrak Rf\|_p$
. Thus, $\| \mathfrak Rf \|_{L_\alpha ^p} \gtrsim \lambda ^\alpha \|\mathfrak Rf\|_p$![]() . Combining this with the $L^p-L_\alpha ^p$
. Combining this with the $L^p-L_\alpha ^p$![]() estimate gives $\lambda ^\alpha \le C \|f\|_p$
estimate gives $\lambda ^\alpha \le C \|f\|_p$![]() for any choice of $\varepsilon _\ell$
for any choice of $\varepsilon _\ell$![]() . By Khintchine's inequality we have $\mathbb {E} (\| f \|_p^p) \sim \int (\sum _\ell | f_\ell |^2 )^{p/2}\,{\rm d}x\,{\rm d}t \sim C_\rho \lambda ^{{p}/{2d}}$
. By Khintchine's inequality we have $\mathbb {E} (\| f \|_p^p) \sim \int (\sum _\ell | f_\ell |^2 )^{p/2}\,{\rm d}x\,{\rm d}t \sim C_\rho \lambda ^{{p}/{2d}}$![]() . Therefore, we see $\lambda ^\alpha \lesssim \lambda ^{1/{2d}}$
. Therefore, we see $\lambda ^\alpha \lesssim \lambda ^{1/{2d}}$![]() and then $\alpha \le 1/(2d)$
and then $\alpha \le 1/(2d)$![]() taking $\lambda \to \infty$
taking $\lambda \to \infty$![]() .
.
Proof. Proof of (iii)
Since $\gamma$![]() is of type $L$
is of type $L$![]() at $s_{\circ }$
at $s_{\circ }$![]() , by an affine transformation and taking $\psi$
, by an affine transformation and taking $\psi$![]() supported near $s_{\circ }$
supported near $s_{\circ }$![]() , we may assume
, we may assume
for $1\le a_1 <\cdots < a_d=L$![]() and smooth functions $\varphi _j$
and smooth functions $\varphi _j$![]() , $j=1,\ldots,d$
, $j=1,\ldots,d$![]() , where $\|\varphi _j-1/a_j!\|_{\mathrm C^{a_d+1}(I)}\le c$
, where $\|\varphi _j-1/a_j!\|_{\mathrm C^{a_d+1}(I)}\le c$![]() for a small constant $c>0$
for a small constant $c>0$![]() . We may also assume $s_{\circ }=0$
. We may also assume $s_{\circ }=0$![]() and furthermore $\gamma (0)=0$
and furthermore $\gamma (0)=0$![]() by replacing $f(x,t)$
by replacing $f(x,t)$![]() by $f(x-t\gamma (0),t)$
by $f(x-t\gamma (0),t)$![]() .
.
Let $\phi _1\in \mathcal {S}(\mathbb {R})$![]() such that $\phi _1 \ge 1$
such that $\phi _1 \ge 1$![]() on $[-1,1]$
on $[-1,1]$![]() , and $\operatorname {supp} \widehat \phi _1\subset [1/2,4]$
, and $\operatorname {supp} \widehat \phi _1\subset [1/2,4]$![]() with $\widehat \phi _1=1$
with $\widehat \phi _1=1$![]() on $[1,2]$
on $[1,2]$![]() . Let $\psi _0\in \mathrm C_c^\infty ((-1,1))$
. Let $\psi _0\in \mathrm C_c^\infty ((-1,1))$![]() with $\psi _0=1$
with $\psi _0=1$![]() on $[-1/2,1/2]$
on $[-1/2,1/2]$![]() . We consider
. We consider

Denoting $\|a\|=\sum _{j=1}^d a_j$![]() , we have $\|f\|_p \lesssim \lambda ^{-\|a\|/(Lp)}$
, we have $\|f\|_p \lesssim \lambda ^{-\|a\|/(Lp)}$![]() . Set $E_\lambda =\{ (x,s) \in \mathbb {R}^d \times I: |x_j| \le c \lambda ^{-a_j/L}, j=1,\ldots,d, \quad |s| \le c \lambda ^{-1/L} \}$
. Set $E_\lambda =\{ (x,s) \in \mathbb {R}^d \times I: |x_j| \le c \lambda ^{-a_j/L}, j=1,\ldots,d, \quad |s| \le c \lambda ^{-1/L} \}$![]() for a sufficiently small $c>0$
for a sufficiently small $c>0$![]() . Since $\gamma (s)=(s^{a_1} \varphi _1(s),\dots, s^{a_d} \varphi _d(s))$
. Since $\gamma (s)=(s^{a_1} \varphi _1(s),\dots, s^{a_d} \varphi _d(s))$![]() , $| \langle x+t\gamma (s),e_j \rangle | \le 2^{-1}\lambda ^{-a_j/L},$
, $| \langle x+t\gamma (s),e_j \rangle | \le 2^{-1}\lambda ^{-a_j/L},$![]() $j=1,\dots,d$
$j=1,\dots,d$![]() , for $(x,s) \in E_\lambda$
, for $(x,s) \in E_\lambda$![]() and $t\in [1,2]$
and $t\in [1,2]$![]() . So, $\mathfrak Rf (x,s)\gtrsim 1$
. So, $\mathfrak Rf (x,s)\gtrsim 1$![]() for $(x,s) \in E_\lambda$
for $(x,s) \in E_\lambda$![]() . This gives $\| \mathfrak Rf\|_p\gtrsim \lambda ^{-(\|a\|+1)/(Lp)}$
. This gives $\| \mathfrak Rf\|_p\gtrsim \lambda ^{-(\|a\|+1)/(Lp)}$![]() . Since $\operatorname {supp} \mathcal {F}_{x_{d}} (\mathfrak Rf) \subset \{ \xi _{d} : |\xi _{d}| \sim \lambda \}$
. Since $\operatorname {supp} \mathcal {F}_{x_{d}} (\mathfrak Rf) \subset \{ \xi _{d} : |\xi _{d}| \sim \lambda \}$![]() , $\| \mathfrak Rf \|_{L_\alpha ^p} \gtrsim \lambda ^{\alpha -(\|a\|+1)/(Lp)}.$
, $\| \mathfrak Rf \|_{L_\alpha ^p} \gtrsim \lambda ^{\alpha -(\|a\|+1)/(Lp)}.$![]() Therefore, we obtain $\alpha \le 1/(Lp)$
Therefore, we obtain $\alpha \le 1/(Lp)$![]() .
.
Acknowledgements
The research was supported by NRF2022R1A4A1018904 (Ko, Lee and Oh) and NRF2022R1I1A1A01055527 (Ko), and a KIAS Individual Grant SP089101 (Oh).