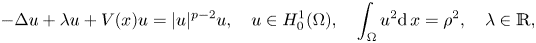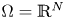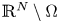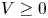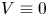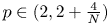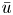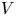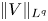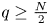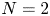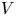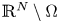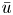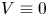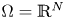1. Introduction and main results

In this paper, we study a class of problems of the form

(P)\[ \left\{\begin{array}{@{}ll@{}} -\Delta u+\lambda u+V(x)\, u=|u|^{p-2}u & \mbox{in }\Omega, \\ \lambda\in\mathbb{R}, \quad u\in H^1_0(\Omega) , \quad \displaystyle{\int_{\Omega} u^2\,{\rm d}\,x=\rho^2}, \end{array} \right.\]

where $\Omega =\mathbb {R}^N$ or $\Omega$ is an exterior domain, that is $\mathbb {R}^N\setminus \Omega$ is a compact set, $N\ge 1$, $V$ is a given potential and $2< p<2+4/N$, namely the nonlinearity is superlinear and mass-subcritical. Here $\lambda$ will arise as a Lagrange multiplier related to the mass constraint $\|u\|_{L^2}=\rho >0$. We will focus on potentials that verify

(1.1)\begin{equation} \begin{aligned} & V\in L^{q} (\mathbb{R}^N),\ q\in[\max(N/2, 1) ,\infty],\ \mbox{ with }q\neq 1\ \mbox{ if }N=2\\ & V(x)\ge 0\ \mbox{ a.e. in }\mathbb{R}^N. \end{aligned} \end{equation}

Problems of the form (P) arise from the nonlinear Schrödinger and Klein-Gordon equations

(S)\[ i \Phi_t+\Delta\Phi+f(\Phi)=0\]

(KG)\[ \Phi_{tt}-\Delta\Phi+m\Phi=f(\Phi),\]

where $\Phi :\mathbb {R}^N\times (0,+\infty )\to \mathbb {C}$. If $f(r e^{i\theta })=f(r)e^{i\theta }$, $r,\theta \in \mathbb {R}$, then one can look for standing wave solutions of (S) and (KG), namely solutions of the form

(1.2)\begin{equation} \Phi(x,t)=e^{i\lambda t}u(x),\quad x\in\mathbb{R}^N,\ t>0, \end{equation}

where $u$ is a real function. In the model case $f(\Phi )=|\Phi |^{p-2}\Phi$ we consider, $u$ has to solve the equation in (P). We refer the reader to [Reference Berestycki and Lions8–Reference Cazenave and Lions10] for more detailed physical motivations.

If the frequency $\lambda$ in ansatz (1.2) is fixed, and we consider the pure power model case with $p\in (2,2^*)$, where $2^*=\frac {2N}{(N-2)^+}$, then looking for solutions of (S) and (KG) corresponds to looking for critical points of the action functional

\[ E_\lambda (u)=\frac12\int_{\mathbb{R}^N}(|\nabla u|^2+[{\lambda}+V(x)]u^2){\rm d}\,x-\frac1p \int_{\mathbb{R}^N}|u|^{p}{\rm d}\,x,\qquad u\in H^1(\mathbb{R}^N). \]

A very large number of works are devoted to this unconstrained problem, we only refer the reader to [Reference Ambrosetti and Malchiodi2, Reference Cerami11, Reference Struwe39] for a survey on almost classical results, and to the recent papers [Reference Devillanova and Solimini16, Reference Molle and Passaseo33] and references therein for new contributions.

Another point of view is to fix a priori the $L^2$ norm of the solution. This point of view is particularly relevant because this quantity (the mass or the charge of the particle) is preserved along the time evolution. In this case, the solutions $u$ correspond to the critical points of the energy functional

\[ E(u)=\frac{1}{2}\int_{\mathbb{R}^N} \left[|\nabla u|^2+V(x) u^2\right]\,{\rm d}\,x -\frac{1}{p}\int_{\mathbb{R}^N} |u|^p\,{\rm d}\,x\qquad u\in H^1(\Omega), \]

constrained on

\[ S_{\rho}:=\left\{u\in H^1(\mathbb{R}^N):\quad |u|_2=\rho\right\}, \]

and the frequency $\lambda$ arises as a Lagrange multiplier. Even if this fixed mass problem is classic (see for example [Reference Cazenave and Lions10]), only in the last decade it has been studied extensively and, in particular, very little has been done in the non-autonomous case (see [Reference Alves and Ji1, Reference Bartsch and de Valeriola5, Reference Bartsch, Molle, Rizzi and Verzini6, Reference Ikoma and Miyamoto24–Reference Jeanjean and Lu26, Reference Molle, Riey and Verzini34, Reference Zhang and Zhang41] and references therein).

We refer the reader to [Reference Dovetta, Serra and Tilli17] for a recent analysis on the connections between the fixed mass and the fixed frequency points of view (see also [Reference Noris, Tavares and Verzini35] and references therein).

If $2+\frac 4N< p<2^*$, the so called mass-supercritical regime, then $E$ is not bounded from below on $S_\rho$, as follows by evaluating the functional over the fibres on $S_\rho$ of the type $u_t:=t^{N/2} u_1(t\cdot )$, for fixed $u_1\in S_\rho$ and $t>0$. So the problem cannot be addressed by minimization. Jeanjean in the pioneering paper [Reference Jeanjean25] analysed the autonomous problem, for more general mass-supercritical nonlinearities, and he recognized a mountain pass structure, related to the above-introduced fibres. The method developed in [Reference Jeanjean25] does not work in the non-autonomous case, even if the potential is radially symmetric. The non-autonomous case in the mass-supercritical regime has been studied in [Reference Bartsch, Molle, Rizzi and Verzini6, Reference Molle, Riey and Verzini34], for not necessarily symmetrical potentials. In [Reference Bartsch, Molle, Rizzi and Verzini6], the authors found a mountain-pass solution if $V\ge 0$ is suitably small, while [Reference Molle, Riey and Verzini34] concerns the case $V\le 0$ and the existence of two solutions is proved when $V$ is suitably small and the operator $-\Delta +V$ is not positive-definite.

In the mass-subcritical regime $2< p<2+\frac 4N$, the functional $E$ is bounded from below on $S_\rho$ by the Gagliardo-Nirenberg inequality (see (3.4), (3.5)). Ikoma e Miyamoto in [Reference Ikoma and Miyamoto24] considered more general non-autonomous problems of the type

(1.3)\begin{equation} \left\{\begin{array}{@{}lr@{}} -\Delta u+\lambda u+V(x)u=f(x,u) & \qquad (\mbox{eq})\\ u\in H^1_0(\Omega),\ u\in S_\rho,\ \lambda\in\mathbb{R}, & \end{array}\right. \end{equation}

where $\Omega =\mathbb {R}^N$, and the nonlinearity $f(x,u)=f(u)$ has sub-linear growth in 0 and mass-subcritical growth at infinity, that is

\[ \lim_{s\to 0}\frac{f(s)}{s}=\lim_{|s|\to \infty}\frac{f(s)}{|s|^{1+4/N}}=0 . \]

If the shape of the potential is $V\le 0$ and $V(x)\to 0$ as $|x|\to \infty$, and both $f$ and $V$ satisfy various technical assumptions, then the authors prove, by concentration-compactness arguments, that there exists $\rho _0>0$ such that the energy functional related to (1.3) has a global minimum in $S_\rho$ for $\rho >\rho _0$, and the global minimum is not attained if $0<\rho <\rho _0$. Moreover, some sufficient conditions on $f$ and $V$ are provided to get $\rho _0=0$.

In the more recent paper [Reference Alves and Ji1], Alves and Ji considered in problem (P) some classes of potentials $V$ where a global minimum for $E$ on $S_\rho$ exists, for suitable $\rho$. Namely, in [Reference Alves and Ji1] the authors work on potentials $V$, not required vanishing at infinity, that verify one of these assumptions: $(V_1)$ $\inf _{\mathbb {R}^N} V<\liminf _{|x|\to \infty }V(x)$, $(V_2)$ there exists $\mu _0>0$ such that $\mathop {\rm meas}\nolimits \{V>\mu _0\}<\infty$ and $\mathop {\rm int}\nolimits (V^{-1}(0))\neq \emptyset$, $(V_3)$ $V$ is 1-periodic in each variable, $(V_4)$ $V$ is asymptotically 1-periodic, that is there exists a 1-periodic function $V_p:\mathbb {R}^N\to \mathbb {R}$ such that

\[ V(x)\le V_p(x),\quad \forall x\in\mathbb{R}^N,\text{ and }\lim_{|x|\to\infty}|V_p(x)-V(x)|=0. \]

After submitting this paper, the interesting works [Reference Song37, Reference Yang, Qi and Zou40] have been brought to our attention. In those papers, non homogeneous nonlinearities as in (1.3) are studied.

Yang, Qi and Zou in [Reference Yang, Qi and Zou40] improve the result of [Reference Ikoma and Miyamoto24]. They consider problem (1.3) with $\Omega =\mathbb {R}^N$, $V(x)\le \lim _{|x|\to \infty }V(x)=:V_\infty \in (-\infty,+\infty ]$ and $f\in {\mathcal{C}}(\Omega \times \mathbb {R},\mathbb {R})$ satisfies Berestycki-Lions type conditions with mass-subcritical growth. Moreover, some technical assumptions used in [Reference Ikoma and Miyamoto24] are removed. In the coercive case $V_\infty =+\infty$, the existence of a ground state solution is proved for every $\rho >0$, while for $V_\infty <+\infty$ the authors show that still $\rho _0\ge 0$ exists such that there is a ground state solution for $\rho >\rho _0$ and it does not exist for $0<\rho <\rho _0$.

Some results in [Reference Song37] concern symmetric exterior domains in some mass-supercritical regimes. Therein, Song studies (1.3) when $\Omega$ is a bounded starshaped domain or the complementary of a ball in $\mathbb {R}^N$ with $N\ge 2$, $V\equiv 0$ and $f\in {\mathcal{C}}^1(\Omega \times \mathbb {R},\mathbb {R})$ is a superlinear and mass-supercritical nonlinearity. When $\Omega$ is the complementary of a ball and $f(\cdot,u)$ is radially symmetric, the author proves that there exists a sequence $((\lambda _k,u_k))_k$ in $H^1_0(\Omega )\times \mathbb {R}$ of solutions of (1.3)(eq) such that $\lambda _k\to 0$ and $|u_k|_2\to \infty$, while there exists no sequence $((\lambda _k,u_k))_k$ in $H^1_0(\Omega )\times \mathbb {R}$ of solutions of (1.3)(eq) such that $\lambda _k\to 0$ and $|u_k|_2\to 0$. Moreover, if (1.3)(eq) has at most one positive radial solution in $H^1_0(\Omega )$ for every $\lambda >0$, then (1.3) has a positive radial solution for large $\rho$.

Here, we are concerned with the mass-subcritical case when $V\ge 0$ so no minimum solution exists, up to the autonomous case $V\equiv 0$ and $\Omega =\mathbb {R}^N$ (see proposition 1.4). Moreover, we focus on domains that can be not only $\mathbb {R}^N$ but also general exterior domains, answering the question raised in [Reference Molle, Riey and Verzini34] for mass-supercritical problems, whether the existence of bound state solutions can be treated in exterior domains as in the whole space. To the best of our knowledge, these issues are only partially addressed in previous papers. About exterior domains in the mass-subcritical case, it is worth mentioning [Reference Zhang and Zhang41], where the authors consider the autonomous problem $V\equiv 0$ in dimension $N\ge 3$ and they found the existence of a bound state solution if the size of ‘hole’ $\mathbb {R}^N\setminus \Omega$ is small.

The main results are the following:

Theorem 1.1 Let $N\ge 2,$ $\Omega =\mathbb {R}^N$ or $\mathbb {R}^N\setminus \Omega$ compact, $\rho >0$. If $V$ satisfies (1.1) and

(D _ρ)\[ \int_{\mathbb{R}^N} V(x)\,|x|^{N-1} e^{d_\rho|x|}\,{\rm d}\,x<\infty,\]

where

(1.4)\begin{equation} d_\rho=\left[2^{\left(1-\frac{p-2}{4-N(p-2)}\right)}\sqrt\lambda_1\right]\, \rho^\frac{p-2}{4-N(p-2)}, \end{equation}

(see (2.5) for the constant $\lambda _1$), then there exists a solution $(\lambda,\bar u)$ of (P) such that $\lambda >0$ and $\bar u\ge 0$.

Theorem 1.2 Let $N\ge 2$, $\Omega =\mathbb {R}^N$ or $\mathbb {R}^N\setminus \Omega$ compact, $\rho >0$. There exists $L =L(q,\Omega,\rho )>0$ such that if $V$ satisfies (1.1), with

(1.5)\begin{equation} V(x)\mathop{\longrightarrow} 0\ \mbox{ as }|x|\to\infty\quad \mbox{if }V\in L^\infty(\Omega), \end{equation}

and $\|V\|_{q}< L$, then problem (P) has a solution $(\lambda,\bar u)$, verifying $\lambda >0$ and $\bar u\ge 0$.

The case $N = 1$ has its own specificity and will be dealt with in § 6.

A priori, a nonnegative solution $\bar u$ of (P) belongs to $H^1(\Omega )$, so we cannot say that $\bar u>0$, $\forall x\in \Omega$. Anyway, under mild assumptions, $\bar u$ turns out to be sufficiently regular to apply the Harnack inequality and therefore to be pointwise positive. In the following proposition, we collect some regularity properties. Its proof is almost standard, so we only outline it in the appendix.

By proposition 1.3, the solutions given by theorems 1.1 and 1.2 are positive if assumption (1.1) holds with $q>\frac N2$.

The solution we find is a bound state solution. Indeed, in § 2 we verify that no ground state solution can exist:

Actually, if $V$ satisfies both the assumptions of theorems 1.1 and 1.2, then the solutions provided by the theorems are found exploiting the same topological configuration so it is reasonable to expect that they are the same solution. Moreover, the topological characterization suggests that they have Morse index $N$.

In (1.1), the assumption that $V$ vanishes at infinity is necessary to get the compactness condition and cannot be dropped, by the following nonexistence result (see [Reference Esteban and Lions19], [Reference Molle, Riey and Verzini34, Proposition 1.10], [Reference Cerami and Molle12, Theorem 1.1]).

Proposition 1.5 Let $p\in (2,2^*)$, $V\in L^\infty (\mathbb {R}^N)$ and assume that there exists $\frac {\partial V}{\partial \nu }\in L^{\ell }(\mathbb {R}^N)$ for some $\nu \in \mathbb {R}^N\setminus \{0\}$ and $\ell \in [\max (1,\frac {N}{2}) ,+\infty ]$, $\ell \neq 1$ if $N=2$. If $\frac {\partial V}{\partial \nu }\ge 0$ and $\frac {\partial V}{\partial \nu }\not \equiv 0$, then problem

(1.6)\begin{equation} -\Delta u+\lambda u-V(x)u=|u|^{p-2}u\qquad u\in S_\rho, \quad \lambda\in\mathbb{R} \end{equation}

has no solutions in ${\mathcal{C}}^1(\mathbb {R}^N)\cap W^{2,2}(\mathbb {R}^N)$.

See remark 6.2 and proposition 6.3 for nonexistence results in the 1-dimensional case.

Some remarks are in order, concerning the decay assumption (D _ρ) in theorem 1.1. It does not require any smallness assumption on $V$ and moreover does not depend on the domain, hence if it is verified then problem (P) has a solution for every exterior domain $\Omega$.

On the other hand, (D _ρ) depends on $\rho$ by (1.4), where $d_\rho \to \infty$ as $\rho \to \infty$ because $p<2+\frac 4N$. As a consequence, a potential that verifies (D _ρ) for every $\rho >0$ has to decay faster than any exponential.

Remark 1.6 By the proof of theorem 1.2 we verify the existence of a constant $\bar L=\bar L(N)>0$ such that

(1.7)\begin{equation} L(N/2,\mathbb{R}^N,\rho)=\bar L \qquad\forall \rho>0 . \end{equation}

Instead, for $q>\frac N2$,

(1.8)\begin{equation} \lim_{\rho\to 0}L(q,\mathbb{R}^N,\rho)=0, \qquad \lim_{\rho\to\infty}L(q,\mathbb{R}^N,\rho)=\infty. \end{equation}

As a consequence, if $\|V\|_{N/2}$ is suitably small, then problem (P) in $\mathbb {R}^N$ has a solution for every $\rho >0$, while if $V\in L^q(\mathbb {R}^N)$, for $q>N/2$, then there exists $\bar \rho =\bar \rho ({\|V\|_q})\ge 0$ such that problem (P) has a solution for every $\rho >\bar \rho$ (see (5.14)).

In Theorems 1.1 and 1.2 of [Reference Molle, Riey and Verzini34] a mountain pass solution is found in the mass-supercritical case with negative potential, under smallness assumptions on $V$ similar to the ones considered here. In that case, if $q=N/2$ there is a uniform bound as in (1.7) while for $q>N/2$ the limits in (1.8) are reversed.

If $\rho$ is fixed, we will observe that

\[ \lim_{r(\Omega)\to\infty} L(q,\Omega,\rho)=0, \]

where

(1.9)\begin{equation} r(\Omega)=\sup\{r\in\mathbb{R}^+\ :\ B_r(y)\subset \mathbb{R}^N\setminus\Omega\mbox{ for some } y\in\mathbb{R}^N\} \end{equation}

(see remark 5.1). Hence, there is no potential $V\not \equiv 0$ such that theorem 1.2 applies for every exterior domain $\Omega$, for $\rho >0$ fixed.

If we consider $V\equiv 0$, then both theorems 1.1 and 1.2 apply and provide the following result.

Corollary 1.7 Let $N\ge 2,$ $\mathbb {R}^N\setminus \Omega$ compact and $\rho >0$, then there exist $\lambda >0$ and $\bar u\in {\mathcal{C}}^2(\Omega )$, $\bar u(x)>0$, $\forall x\in \Omega$, such that

\[ \left\{ \begin{array}{@{}ll@{}} -\Delta \bar u+\lambda \bar u =\bar u^{p-1} & \mbox{in }\Omega, \\ \lambda\in\mathbb{R}, \quad \bar u\in H^1_0(\Omega),\quad \displaystyle{\int_{\Omega} \bar u^2\,{\rm d}\,x=\rho^2}. \end{array} \right. \]

Corollary 1.7 extends the result of [Reference Zhang and Zhang41] to the dimension 2 and to every exterior domains. The proof in [Reference Zhang and Zhang41] cannot be extended to this more general framework because it does not work in dimension 2 and when the size of $\mathbb {R}^N\setminus \Omega$ is large.

We prove theorems 1.1 and 1.2 by variational methods, looking for bound state solutions. The analysis of the compactness presents a lot of difficulties related to the unboundedness of the domain, that is not assumed to be symmetric. In order to recover a local compactness condition, we first see that the Lagrange multiplier $\lambda$ related to a Palais-Smale sequence in a negative range is positive, and then we employ a splitting Lemma from [Reference Benci and Cerami7] for PS-sequences of $E_\lambda$. With this decomposition in hands, we perform in proposition 3.1 a sharp fine estimate of the first energy interval $I$ above the infimum where the compactness condition holds (see remark 3.4).

The topological argument relies on min-max techniques that make use of a barycentric map and the Brouwer degree. Since we have no smallness assumption on $\mathbb {R}^N\setminus \Omega$, and in theorem 1.1 we have no bound on any Lebesgue norm of the potential, a major difficulty is to work in the compactness interval $I$, in the min-max procedure. To overcome this problem, we will proceed by analysing the energy interaction of positive solutions $w_1$ and $w_2$ of some suitable ‘problems at infinity’, such that $\|w_1\|_{L^2}^2+\|w_2\|_{L^2}^2=\rho ^2$. This idea is inspired by the unconstrained case (see [Reference Cerami and Passaseo14, Reference Lancelotti and Molle28]), where the problem at infinity is univocally determined by the choice of $\lambda$. Here, the need to choose different functions $w_1,w_2$ prevents the use of the arguments developed in [Reference Cerami and Passaseo14, Reference Lancelotti and Molle28]) and requires the introduction of different and more refined estimates.

To verify that the solutions we find do not change sign, we prove in proposition 2.2 that the energy of every solution that changes sign is not in the energy interval we are working in. In particular, proposition 2.2 and corollary 2.3 give information also on changing sign solutions of the autonomous problem in $\mathbb {R}^N$. We point out that to get nonnegative solutions here we could exploit the symmetry of the functional and work near the cone of the positive functions, by using [Reference Ghoussoub21, Theorem 4.5] and proceeding as in [Reference Bartsch, Molle, Rizzi and Verzini6]. The advantage of this other approach is a simplification of the proof of the compactness condition, because the lack of compactness in such a case comes only from the positive solution of the problem at infinity. On the other hand, the approach we employ here allows us both to bound from below the energy of the changing sign solutions and to gain a more general analysis of the Palais-Smale sequences.

The paper is organized as follows: in § 2 we introduce some preliminary results, in § 3 we prove the local compactness condition and § 4 is devoted to the sharp energy estimates that are necessary in § 5 to prove theorems 1.1 and 1.2, § 6 concerns the case $N=1$ and in the appendix we give a sketch of the proof of the regularity proposition, with some references for detailed proofs.

2. Notations, variational framework and preliminary results

Throughout the paper we make use of the following notation:

• • $2_c:=2+\frac 4 N$, ${ s}=\frac 2N\frac {p-2}{2_c-p}$.

• • $L^q(\mathcal {O})$, $1\leq q \leq \infty$, $\mathcal {O}\subseteq \mathbb {R}^N$ a measurable set, denotes the Lebesgue space, the norm in $L^q(\mathcal {O})$ is denoted by $|\cdot |_q$ if there is no ambiguity.

• • For $u\in H^1_0(\Omega )$ we denote by $u$ also the function in $H^1(\mathbb {R}^N)$ obtained setting $u\equiv 0$ in $\mathbb {R}^N\setminus \Omega$.

• • For any $R>0$ and for any $z\in \mathbb {R}^N$, $B_R(z)$ denotes the closed ball of radius $R$ centred at $z,$ and for any measurable set $\mathcal {O} \subset \mathbb {R}^N, \ |\mathcal {O}|$ denotes its Lebesgue measure.

• • $H^{1}(\mathbb {R}^{N})$ is the usual Sobolev space endowed with the standard norm

  \[ \|u\|:=\left[\int_{\mathbb{R}^N}\left(|\nabla u|^{2}+ u^{2}\right){\rm d}\,x\right]^{\frac 12}. \]

• • $c,c', C, C', C_i,\ldots$ denote various positive constants that can also vary from one line to another.

• • $o(f)$ and $O(f)$ denote the usual Landau notations: $\frac {o(f)}{f}\to 0$ as $f\to 0$ and $|O(f)|\le C |f|$ for some positive constant $C$.

We will find solutions $\bar u$ of problem $(P)$ as critical points of the functional $E$ constrained on $S_{\rho }$. If $\lambda$ is the Lagrange multiplier related to $\bar u$, then $\bar u$ is also a free critical point of the related free functional $E_\lambda$.

Let us assume $\lambda >0$ and recall some well-known properties of the limit problem, for $V\equiv 0$ and $\Omega =\mathbb {R}^N$,

(P _∞)\[ \left\{ \begin{array}{@{}ll@{}} -\Delta u+\lambda_{\infty} u=|u|^{p-2}u & \text{in }\mathbb{R}^N, \\ \lambda_{\infty} \in\mathbb{R}, \quad u\in S_\rho. \end{array} \right.\]

(P _∞) has a unique positive solution $w\in H^1(\mathbb {R}^N)$, up to translations, which is radial, radially decreasing, and belongs to $C^2(\mathbb {R}^N)$. The function $w$ verifies the minimality property

(2.1)\begin{equation} m:=E_\infty(w)=\min_{u\in S_\rho} E_\infty(u), \end{equation}

where

\[ E_\infty(u)=\frac{1}{2}\int_{\mathbb{R}^N} |\nabla u|^2\,{\rm d}\,x -\frac{1}{p}\int_{\mathbb{R}^N} |u|^p\,{\rm d}\,x. \]

Correspondingly, the solutions of (P _∞) are also free critical points of the limit functional

\[ E_{\lambda,\infty}(u)=E_\infty (u)+\frac{\lambda}{2}\int_{\mathbb{R}^N} u^2{\rm d}\,x\qquad u\in H^1(\mathbb{R}^N). \]

Moreover,

(2.2)\begin{equation} m<0\quad\mbox{ and }\quad \lambda_\infty>0 \end{equation}

and there exists $c_1>0$ such that

(2.3)\begin{align} & w(|x|)\, e^{\sqrt{\lambda_{\infty}}|x|}\,|x|^{\frac{N-1}{2}} \to c_1 \quad \mbox{as }|x|\to \infty, \end{align}

(2.4)\begin{align} & w'(|x|)\, e^{\sqrt{\lambda_{\infty}}|x|}\,|x|^{\frac{N-1}{2}} \to -c_1\sqrt{\lambda_{\infty}} \quad \mbox{as }|x|\to \infty. \end{align}

Inequality $m<0$ in (2.2) follows choosing $u_1\in S_\rho$ and testing (2.1) on $u_t:=t^{N/2}u_1(t\cdot )\in S_\rho$, $t>0$, taking into account $p<2_c$. The positivity of $\lambda _\infty$ comes from Pohozaev and Nehari identities (see also proposition 2.1). For the properties of $w$ we refer the reader to [Reference Bahri and Lions4, Reference Berestycki and Lions8, Reference Gidas, Ni and Nirenberg22, Reference Kwong27, Reference Strauss38].

For any $k>0$, let us denote by $w_{k\rho ^2}$ the positive solution of

(2.5)\begin{equation} \left\{ \begin{array}{@{}ll@{}} -\Delta u+\lambda_{k\rho^2}\, u=|u|^{p-2}u & \mbox{in }\mathbb{R}^N, \\ \lambda_{k\rho^2}\in\mathbb{R},\quad u\in S_{ \sqrt k\, \rho}, \\ \end{array} \right. \end{equation}

where $w_{k\rho ^2}$ verifies

(2.6)\begin{equation} m_{k\rho^2}:=E_{\infty}(w_{k\rho^2})=\inf_{u\in S_{\sqrt k\, \rho}} E_{\infty}(u). \end{equation}

It turns out that

(2.7)\begin{equation} w_{k\rho^2}(x)=k^{\frac{{ s}}{p-2}}w\left(k^{\frac{{ s}}{2}}x\right), \quad m_{k\rho^2}=k^{1+{ s}}\,m<0, \quad \lambda_{k\rho^2}=k^{{ s}}\,\lambda_{\infty}>0 \end{equation}

where

\[ { s}:=\frac2N\frac{p-2}{2_c-p} \]

(see notations). From $p<2_c$ it follows $s>0$. By (2.7), in particular we have:

(2.8)\begin{equation} \lambda_{\rho^2}=\lambda_\infty =\rho^{s}\lambda_1. \end{equation}

For $k=0$ we set $w_0=0$ and for $k=1$ we simply write $w_{\rho ^2}=w$.

Moreover, for any $k>0$, by (2.3), (2.4) and (2.7) we have

(2.9)\begin{align} & w_{k\rho^2}(|x|)\, e^{\sqrt{k^{{ s}}\lambda_{\infty}}|x|}\,|x|^{\frac{N-1}{2}} \to c_k \quad \mbox{as }|x|\to +\infty, \end{align}

(2.10)\begin{align} & w_{k\rho^2}'(|x|)\, e^{\sqrt{k^{{ s}}\lambda_{\infty}}|x|}\,|x|^{\frac{N-1}{2}} \to -c_k\,\sqrt{k^{{ s}}\,\lambda_{\infty}} \quad \mbox{as }|x|\to +\infty \end{align}

where

\[ c_k=c_1\, k^{{ s}\left(\frac{1}{p-2}-\frac{N-1}{4}\right)}. \]

From $p<2_c$ there follows $\frac {1}{p-2}-\frac {N-1}{4}>0$, so that

\[ c_k\to 0\quad\mbox{ as } k\to 0, \quad c_k\to c_1\quad\mbox{ as } k\to 1. \]

Proof Proof of proposition 1.4

Let us prove that

(2.11)\begin{equation} \inf_{S_{\rho}} E=m \end{equation}

and the infimum is not achieved.

Obviously we have that ${\inf _{S_{\rho }} E\geq m}$. To show that the equality holds, let us consider the sequence $(u_n)_n$ defined by $u_n=w(x-y_n)$, where $(y_n)_n$ is a sequence in $\mathbb {R}^N$ such that $|y_n|\to +\infty$. By (1.1) and (2.3), and taking into account (D _ρ) or (1.5), we have that

\[ \lim_{n\to +\infty} \int_{\mathbb{R}^N} V(x) u_n^2(x)\,{\rm d}\,x =0, \]

which implies $\displaystyle {\lim _{n\to +\infty } E(u_n)=m}$.

Now, assume by contradiction that the infimum ${\inf _{S_{\rho }} E}$ is achieved by a function $\bar u$. Then, by

(2.12)\begin{equation} m\le E_\infty(\bar u)\le E(\bar u)=m,\qquad \bar u\in S_\rho, \end{equation}

and by the uniqueness of the minimizers of (2.1), we should have $\bar {u}=\pm w(x-\bar {y})$ for a suitable $\bar y\in \mathbb {R}^N$. Since $w(x-\bar {y})>0$ for all $x\in \mathbb {R}^N$, we can deduce that $\Omega$ must be the entire space $\mathbb {R}^N$ and by (2.12) we get:

\[ \int_{\mathbb{R}^N} V(x) \bar{u}^2(x)\,{\rm d}\,x =0, \]

that implies $V \equiv 0$ because $V\ge 0$. So we are in contradiction with $\Omega \neq \mathbb {R}^N$ or $V\not \equiv 0$.

Next proposition states the positivity of the Lagrange multipliers in (P).

Proof We have

\[ \int_\Omega \nabla u\cdot \nabla v\, {\rm d}\,x +\lambda\int_\Omega u v\, {\rm d}\,x +\int_\Omega V(x)u v\, {\rm d}\,x-\int_\Omega |u|^{p-2}uv\, {\rm d}\,x=0\qquad \forall v\in H^1_0(\Omega). \]

Then,

(2.13)\begin{align} \lambda\rho^2 & =|u|_p^p-|\nabla u|_2^2-\int_\Omega V(x)u^2 {\rm d}\,x \notag\\ & ={-}p E(u)+\frac{p-2}{2}|\nabla u|_2^2+\frac{p-2}{2}\int_\Omega V(x)u^2 {\rm d}\,x>0. \end{align}

Before concluding this section, let us find out some features of the changing sign solutions.

Proof Since $m<0$, we can assume $E(u)<0$. According to proposition 2.1, let $\lambda >0$ be the Lagrange multiplier corresponding to the solution $u$ and let $w_\infty \in H^1(\mathbb {R}^N)$ be the positive solution of $-\Delta v+\lambda v=v^{p-1}$. By (2.7),

(2.14)\begin{equation} E_\infty(w_\infty)=\min_{S_{|w_\infty|_2}}E_\infty=\left(\frac{|w_\infty|_2^2}{\rho^2}\right)^{1+s}m. \end{equation}

Let us write $u=u^+-u^-$, where $u^\pm =\max \{\pm u,0\}$, $\rho ^2=|u^+|_2^2+|u^-|_2^2$. Since $u^+$ and $u^-$ are on the Nehari manifold ${\mathcal {N}}$ corresponding to $E_\lambda$, then $(E_\lambda )_{|_{\mathcal {N}}}(u^\pm ) > E_{\lambda,\infty }(w_\infty )$ (see [Reference Benci and Cerami7] and [Reference Molle, Musso and Passaseo31, Proposition 2.1]). Namely, by (2.14),

\[ E(u^\pm)> \left(\frac{|w_\infty|_2^2}{\rho^2}\right)^{1+s}m+\frac\lambda 2(|w_\infty|_2^2-|u^\pm|_2^2). \]

Hence, the claim read as

\[ E(u)=E(u^+)+E(u^-)>2\, \left(\frac{|w_\infty|_2^2}{\rho^2}\right)^{1+s}m+\frac\lambda 2(2|w_\infty|_2^2-\rho^2)\ge 2^{{-}s}m, \]

and, equivalently, as

(2.15)\begin{equation} \left[\left(\frac{2|w_\infty|_2^2}{\rho^2}\right)^{1+s}-1\right]2^{{-}s} m +\frac\lambda 2\rho^2\,\left[\left(\frac{2|w_\infty|_2^2}{\rho^2}\right)-1\right]\ge 0. \end{equation}

Since $(\lambda,w_\infty )$ solves (2.5) for $k=\frac {|w_\infty |^2_2}{\rho ^2}$, the following system is fulfilled

(2.16)\begin{equation} \left\{ \begin{array}{@{}ll@{}}\dfrac 12 |\nabla w_\infty|_2^2-\dfrac 1p|w_\infty|_p^p=m_{|w_\infty|_2^2} & Energy\ of\ w_\infty\\ |\nabla w_\infty|_2^2+\lambda | w_\infty|_2^2-|w_\infty|_p^p=0 & Nehari\\ \dfrac{N-2}{2}|\nabla w_\infty|_2^2+\dfrac N 2\lambda|w_\infty|_2^2-\dfrac{N}{p}|w_\infty|_p^p=0 & Pohozaev. \end{array} \right. \end{equation}

Solving (2.16) and taking into account (2.7) we get

(2.17)\begin{equation} \frac \lambda 2\,\rho^2 ={-}\frac{2p-N(p-2)}{4-N(p-2)}\, \left(\frac{|w_\infty|_2^2}{\rho^2}\right)^{s}m. \end{equation}

Putting (2.17) in (2.15), we have to verify

\[ \left[\left(\frac{2|w_\infty|_2^2}{\rho^2}\right)^{1+s}-1\right] -\frac{2p-N(p-2)}{4-N(p-2)} \,\left(\frac{2|w_\infty|_2^2}{\rho^2}\right)^s\left[\left(\frac{2|w_\infty|_2^2}{\rho^2}\right)-1\right]\le 0. \]

Then the assertion is proved because $\frac {2p-N(p-2)}{4-N(p-2)}=1+s$ and

\[ [t^{1+s}-1]-(1+s) \, t^s[t-1]\le 0\qquad\forall t\ge 0. \]

Corollary 2.3 If $u$ is a changing sign solution of

(2.18)\begin{equation} -\Delta v+\lambda v=|v|^{p-2}v\qquad v\in H^1(\mathbb{R}^N), \end{equation}

then $|u|_2^2> 2|w_\infty |_2^2$, where $w_\infty$ denotes the positive solution of (2.18).

Clearly, here $\lambda >0$ by Pohozaev identity.

Proof Let us call $E(u)=M$. From system (2.16) applied to $u$ and $w_\infty$ it follows

(2.19)\begin{equation} |u|_2^2=2\, \frac{1+s}{\lambda}\, ({-}M),\qquad |w_\infty|_2^2=2\, \frac{1+s}{\lambda}\, \left({-}m_{|w_\infty|_2^2}\right). \end{equation}

By proposition 2.2, $M>2^{-s}m_{|u|_2^2}$, then, by (2.7) and (2.19),

\begin{align*} & |u|_2^2 < 2\, \frac{1+s}{\lambda}\, 2^{{-}s} \left({-}m_{|u|_2^2}\right)= 2\, \frac{1+s}{\lambda}\, 2^{{-}s}\left(\frac{|u|_2^2}{|w_\infty|_2^2}\right)^{1+s} \left({-}m_{|w_\infty|_2^2}\right)\\ & \quad =2^{{-}s} |w_\infty|_2^2 \left(\frac{|u|_2^2}{|w_\infty|_2^2}\right)^{1+s}, \end{align*}

that completes the proof.

3. The compactness condition

To prove proposition 3.1, we will use the well known splitting lemma of Benci and Cerami for the unconstrained problem ([Reference Benci and Cerami7, Lemma 3.1]).

Lemma 3.2 Let $\lambda >0$ and let $(u_n)_n$ in $H^1(\mathbb {R}^N)$ be a Palais-Smale sequence for $E_\lambda$. Then there exist a critical point $u_0$ of $E_\lambda$, an integer $h\ge 0$, $h$ non-trivial solutions $w^1,\dots,w^h\in H^1(\mathbb {R}^N)$ to the limit equation

\[ -\Delta v+\lambda v=|v|^{p-2}v \]

and $h$ sequences $(y_n^j)_n\subset \mathbb {R}^N$, $1\le j\le h$, such that $|y_n^j|\to \infty$ as $n\to \infty$, and

(3.1)\begin{equation} u_n=u_0+\sum_{j=1}^h w^j(\cdotp-y^j_n)+o(1)\qquad\text{strongly in $H^1(\mathbb{R}^N)$,} \end{equation}

up to a subsequence. Moreover, we have:

(3.2)\begin{equation} E_\lambda (u_n)\to E_\lambda (u_0)+\sum_{j=1}^h E_{ \lambda,\infty}(w^j)\qquad\text{as $n\to\infty$,} \end{equation}

and

(3.3)\begin{equation} |u_n|_2^2=|u_0|_2^2+\sum_{j=1}^h |w^j|_2^2+o(1). \end{equation}

Proof Proof of proposition 3.1

We claim that $(u_n)_n$ is bounded in $H^1_0(\Omega )$. Indeed, $u_n\in S_\rho$, $\forall n\in \mathbb {N}$, and by the Gagliardo-Nirenberg inequality

(3.4)\begin{equation} |u|_p^p\leq C_{GN} |u|_2^{p-\frac{N(p-2)}{2}}|\nabla u|_2^{\frac{N(p-2)}{2}} \end{equation}

(see [Reference Leoni29, Theorem 12.83]) we have:

(3.5)\begin{equation} c+o(1)=E(u_n)\ge\frac 12 |\nabla u_n|^2_2-C |\nabla u_n|_2^{\frac{N(p-2)}{2}}, \end{equation}

with $\frac {N(p-2)}{2}<2$ because $p<2_c$.

Since $(u_n)_n$ is a constrained PS-sequence, there exists a sequence $(\lambda _n)_n$ in $\mathbb {R}$ such that

(3.6)\begin{align} \int_\Omega \nabla u_n\cdot \nabla v\, {\rm d}\,x & +\lambda_n\int_\Omega u_n v\, {\rm d}\,x+ \int_\Omega V(x)\, u_n v\, {\rm d}\,x\nonumber\\ & -\int_\Omega |u_n|^{p-2}u_nv\, {\rm d}\,x=o(1)\|v\| \qquad \forall v\in H^1_0(\Omega). \end{align}

Setting $v=u_n$ in (3.6), and taking into account that $(u_n)_n$ is bounded in $H^1$, we can argue as in (2.13) obtaining

(3.7)\begin{equation} \lambda_n\rho^2 ={-}pE(u_n)+\frac{p-2}{2}|\nabla u_n|_2^2 +\frac{p-2}{2}\int_\Omega V(x)\, u_n^2 {\rm d}\,x +o(1) \ge -p\, c+o(1)>0, \end{equation}

for large $n$. Since $(u_n)_n$ is bounded in $H^1_0(\Omega )$, the first relation in (3.7) implies that the sequence $(\lambda _n)_n$ is bounded. Moreover, from (3.7) we infer also that $\lambda _n\ge c>0$, for a suitable constant $c$. Then we can assume that $\lambda _n\to \lambda >0$. Hence, by (3.6) we are in position to apply lemma 3.2 and we can decompose $u_n$ according to (3.1).

$(I)$ If $h=0$, we are done.

So, we assume by contradiction $h\ge 1$ and we are going to show that then

(3.8)\begin{equation} E (u_0)+\sum_{j=1}^h E_{\infty}(w^j)\ge 2^{{-}s}m, \end{equation}

up to the case $u_0=0$, $h=1$ and $w^1>0$, that arises for

(3.9)\begin{equation} \lim_{n\to\infty}E(u_n)=m. \end{equation}

Once (3.8) is proved, the contradiction comes out, because (3.2) and (3.3) provide $c=E (u_0)+\sum _{j=1}^h E_{\infty }(w^j)$, and $c\in (m,2^{-s}m)$ by assumption.

$(II)$ If $u_0\equiv 0$ and $h=1$ occurs, then $|w^1|_2=\rho$, by (3.3). Hence, if $w^1>0$ then $c=m$ by (3.2) and (2.1), so (3.9) is proved. We observe that this is the only case in which (3.9) holds, up to the autonomous case $\Omega =\mathbb {R}^N$ and $V\equiv 0$, when also the case $u_0>0$ and $h=1$ verifies (3.9). On the other hand, if $w^1$ is a changing sign solution, then $c>2^{-s} m$ by proposition 2.2.

$(III)$ If $u_0\equiv 0$ and $h\ge 2$, we proceed by induction. For $h=2$, we get (3.8) arguing exactly as in the proof of proposition 2.2, with $w^1$ in place of $u^+$ and $w^2$ in place of $u^-$. Observe that the case $w^1=w^2>0$ is the only case when the equality holds in (3.8).

Suppose now $h\ge 3$ and (3.8) holds for $h-1$, namely for every $\rho _1>0$

(3.10)\begin{equation} \sum_{j=1}^{h-1} E_{\infty}(w^j)\ge 2^{{-}s}m_{\rho_1^2}, \end{equation}

whenever $w^j\not \equiv 0$, for all $j\in \{1,\ldots,h-1\}$, and $\sum _{j=1}^{h-1}|w^j|_2^2=\rho _1^2$. Then, let us prove (3.8) for $h$. We can assume that $|w^h|_2^2\le \rho ^2/3$. Taking into account (3.10) and (2.7), we have:

\begin{align*} \sum_{j=1}^hE_\infty(w^j)& \ge 2^{{-}s} m_{(\rho^2-|w^h|_2^2)} +E_\infty(w^h)\\ & \ge 2^{{-}s} m_{(\rho^2-|w^h|_2^2)} +m_{|w^h|_2^2} \\ & = 2^{{-}s}\left(\frac{\rho^2-|w^h|_2^2}{\rho^2}\right)^{1+s}m+\left(\frac{|w^h|_2^2}{\rho^2}\right)^{1+s}m. \end{align*}

Hence, it is sufficient to verify that

(3.11)\begin{equation} 2^{{-}s}\left(\frac{\rho^2-|w^h|_2^2}{\rho^2}\right)^{1+s}m+\left(\frac{|w^h|_2^2}{\rho^2}\right)^{1+s}m\ge 2^{{-}s}m. \end{equation}

Inequality (3.11) is equivalent to

(3.12)\begin{equation} 2^s t^{1+s}+(1-t)^{1+s}\le 1, \end{equation}

where $t:=\frac {|w^h|_2^2}{\rho ^2}\in (0,1/3]$. Since inequality (3.12) holds for every $t\in [0,1/3]$, estimate (3.8) is proved for $u_0\equiv 0$.

$(IV)$ If $u_0\not \equiv 0$, we can proceed as in the previous steps: first by considering the case $h=1$ and arguing as in the proof of proposition 2.2, and then finishing the proof by induction.

Corollary 3.3 If $(u_n)_n$ in $S_\rho$ satisfies $\lim \limits _{n\to \infty }E(u_n)=m$, then there exists a sequence $(y_n)_n$ in $\mathbb {R}^N$ such that

(3.13)\begin{equation} u_n(x)=w(x-y_n)+o(1)\qquad\mbox{ in }H^1. \end{equation}

If $\Omega \neq \mathbb {R}^N$ or $V\not \equiv 0$, then $|y_n|\mathop {\longrightarrow }\infty$, as $n\to \infty$.

Proof By the Ekeland variational principle, there exists a PS-sequence $(v_n)_n$ for $E$ constrained on $S_\rho$ such that $\lim \limits _{n\to \infty }E(v_n)=m$ and $u_n=v_n+o(1)$ in $H^1$ (see ([Reference Ekeland18, Proposition 5.1]), then we can assume that $(u_n)_n$ is PS-sequence. Then (3.13) is a direct consequence of (3.9) and part $(II)$ in the proof of proposition 3.1.

If $y_n\to \bar y\in \mathbb {R}^N$, up to a subsequence, then $u_n\to w(\cdot -\bar y)$ in $H^1$ and a.e., so that $\Omega =\mathbb {R}^N$ because $w(x)>0$, $\forall x \in \mathbb {R}^N$. Moreover

\begin{align*} m=\lim_{n\to\infty}E(u_n)& =\frac12\int_{\mathbb{R}^N} |\nabla w(x-\bar y)|^2{\rm d}\,x+\frac12\int_{\mathbb{R}^N} V(x)\, w(x-\bar y)^2{\rm d}\,x\\ & \quad -\frac 1p\int_{\mathbb{R}^N} w^p(x-\bar y)\, {\rm d}\,x\\ & =m+\frac12\int_{\mathbb{R}^N} V(x)\, w(x-\bar y)^2{\rm d}\,x \end{align*}

implies $V\equiv 0$, again because $w(x)>0$, $\forall x\in \mathbb {R}^N$.

So the proof is completed.

Remark 3.4 By $(III)$ in the proof of proposition 3.1 we see that, for every sequence $(y_n)_n$ in $\mathbb {R}^N$ such that $\lim _{n\to \infty }|y_n|=\infty$,

\[ E\left(w_{ {\rho^2}/{2}}({\cdot}{-}y_n)+w_{ {\rho^2}/{2}}({\cdot}{+}y_n)\right)=2^{{-}s}m. \]

Then the sequence $(w_{{\rho ^2}/{2}}(\cdot -y_n)+w_{{\rho ^2}/{2}}(\cdot +y_n))_n$ turns out to be a not relatively compact PS-sequence at the level $2^{-s}m$, showing that the compactness interval $(m,2^{-s}m)$ cannot be extended.

4. Energy estimates

4.1 An upper bound

If $\mathbb {R}^N\setminus \Omega$ is a non empty compact set contained in $B_{R-1}(0)$, let us introduce the cut-off function $\vartheta$, verifying

(4.1)\begin{equation} \vartheta\in {{\mathcal{C}}}^\infty(\mathbb{R}^N,[0,1]),\qquad \left\{\begin{array}{@{}ll@{}} \vartheta(x)=1 & \mbox{ if } |x|\ge R\\ \vartheta(x)=0 & \mbox{ if }x\in\mathbb{R}^N\setminus\Omega. \end{array}\right. \end{equation}

If $\Omega =\mathbb {R}^N$, we agree that $\vartheta \equiv 1$ on $\mathbb {R}^N$. Let us set $\Sigma =\partial B_2(e_1)$, where $e_1$ is the first element of the canonical basis of $\mathbb {R}^N$, and for any $r>0$ define the map $\psi _r: [0,1]\times \Sigma \longrightarrow H^1_0(\Omega )$ by

(4.2)\begin{equation} \psi_{r}[t,z](x)= \rho\,\frac{\vartheta(x)\left[w_{t\rho^2}(x-r z)+w_{(1-t)\rho^2}(x-r e_1)\right]} {\left|\vartheta({\cdot})\left[w_{t\rho^2}({\cdot}{-}r z)+w_{(1-t)\rho^2}({\cdot}{-}r e_1)\right]\right|_2}. \end{equation}

Proposition 4.1 Suppose that $V$ verifies (1.1) and (D _ρ), then

1. (a) there exist $\overline {r}>0$ such that for any $r>\overline {r}$

   (4.3)\begin{equation} {\mathcal{A}}_r=\max\left\{E\left(\psi_{r}[t,z]\right):\quad t\in [0,1],\, z\in\Sigma\right\} <2^{-{ s}}\,m; \end{equation}

2. (b) for every $\varepsilon >0$ there exists $r_\varepsilon >0$ such that for any $r>r_\varepsilon$

   (4.4)\begin{equation} {\mathcal{L}}_r=\max\left\{E\left(\psi_{r}[1,z]\right)\ :\ z\in\Sigma\right\} \leq m+\varepsilon. \end{equation}

Remark 4.2 In proposition 4.1, $V\equiv 0$ is allowed.

Before proving proposition 4.1, let us recall two technical lemmas. For the proof of Lemma 4.3 we refer to [Reference Cerami and Passaseo14] while the proof of Lemma 4.4 is in [Reference Bahri and Li3] (see also Lemma 2.9 in [Reference Cerami and Molle13]).

Lemma 4.3 For all $a,b\ge 0$, for all $p\ge 2$, the following relation holds true

\[ (a+b)^{p}\ge a^{p}+b^{p}+(p-1)(a^{p-1}b+ab^{p-1}). \]

Lemma 4.4 If $g \in L^\infty (\mathbb {R}^N)$ and $h\in L^1(\mathbb {R}^N)$ are such that, for some $\alpha \ge 0$, $b\ge 0$, $\gamma \in \mathbb {R}$

(4.5)\begin{equation} \lim_{|x|\to \infty} g(x)\,e^{\alpha |x|}|x|^b=\gamma \end{equation}

and

(4.6)\begin{equation} \int_{\mathbb{R}^N} |h(x)| \, e ^{\alpha |x|}|x|^b{\rm d}\,x<\infty, \end{equation}

then, for every $z\in \mathbb {R}^N\setminus \{0\}$,

\[ \lim_{r \to \infty} \left(\int_{\mathbb{R}^N}g(x+r z)h(x) {\rm d}\,x\right) e^{\alpha |r z|} |r z|^b =\gamma \int_{\mathbb{R}^N}h(x) e^{-\alpha\, \frac{x\cdot z}{|z|}}\, {\rm d}\,x. \]

Lemma 4.5 Let $z\in \Sigma$. For every $t\in [0,1/2]$ and $r>0$, let us set

(4.7)\begin{align} \delta_t(r)& =\left(r^{\frac{N-1}{2}}\,e^{2\sqrt{t^{{ s}}\lambda_{\infty}}\,r}\right)^{{-}1}, \end{align}

(4.8)\begin{align} \tau_t(r)& =\frac{2}{\rho^2}\int_{\mathbb{R}^N} w_{t\rho^2}(x-r z)\,w_{(1-t)\rho^2}(x-r e_1)\,{\rm d}\,x, \end{align}

(4.9)\begin{align} \sigma_t(r)& =\int_{\mathbb{R}^N} \left[w^{p-1}_{t\rho^2}(x-r z)\,w_{(1-t)\rho^2}(x-r e_1)+ w_{t\rho^2}(x-r z)\,w^{p-1}_{(1-t)\rho^2}(x-r e_1)\right]\!{\rm d}\,x. \end{align}

Then the following facts hold:

1. (1) if $t\in [0,\frac 12 )$, then $\displaystyle \frac {\tau _t(r)}{\delta _t(r)}\to c_{1,t}:= c_t\int _{\mathbb {R}^N} w_{(1-t)\rho ^2}(y)\, e^{-\sqrt {t^s\lambda _{\infty }}\,\frac {y\cdot (e_1-z)}{2}}\,{\rm d}y, \mbox {as }r\to \infty$,

2. (2) if $t\in [0,\frac 12 ]$, then $\displaystyle \frac {\sigma _t(r)}{\delta _t(r)}\to c_{2,t}:=2 c_t\int _{\mathbb {R}^N} w^{p-1}_{(1-t)\rho ^2}(y)\, e^{-\sqrt {t^s\lambda _{\infty }}\,\frac {y\cdot (e_1-z)}{2}}\,{\rm d}y, \mbox {as }r\to \infty$ where $c_t=c_1 t^{{ s}}(\frac {1}{p-2}-\frac {N-1}{4})$ (see (2.3)). Moreover,

3. (3) $c_{1,t}\cdot (\frac {1}{2}-t) \leq C$ for every $t\in [0,\frac 12)$.

Remark 4.6 The definition of $\tau _t(r),\sigma _t(r)$ is independent of $z$, by symmetry. Moreover, $c_{1,t}\to \infty$ as $t\to \frac 12$ and, clearly, $\tau _0(r)=\sigma _0(r)\equiv 0$.

Proof Assertions $(1),(2)$ easily follow using (2.9) and by lemma 4.4. Let us prove assertion $3)$.

Without loss of generality we may assume $z=-e_1$, so that $\frac {z-e_1}{2}=-e_1$, and to simplify the notations we consider $\lambda _\infty =1$. Moreover, since $t\mapsto c_{1,t}$ is a continuous function on $[0,1/2]$, it is sufficient to prove

\[ \lim_{t\to \frac12}\left(\frac{1}{2}-t\right)\, \int_{\mathbb{R}^N} w_{(1-t)\rho^2}(y)\, e^{t^{s/2}\,y_1}\,{\rm d}y <\infty, \]

where $y_1$ is the first component of $y\in \mathbb {R}^N$. Moreover, by (2.9) we are left to prove that

(4.10)\begin{align} & \left(\frac{1}{2}-t\right)\int_{\mathbb{R}^N\setminus B_1(0)} |y|^{-\frac{N-1}{2}}\,e^{-(1-t)^{s/2}|y|}\, e^{t^{s/2}\,y_1}\,{\rm d}y\nonumber\\ & \quad =\left(\frac{1}{2}-t\right)\int_{\mathbb{R}^N\setminus B_1(0)} |y|^{-\frac{N-1}{2}}\,e^{-\left[(1-t)^{s/2}-t^{s/2}\right]|y|}\, e^{{-}t^{s/2}(|y|-y_1)}\,{\rm d}y \le C <\infty\nonumber\\ & \qquad \mbox{as }t\to (1/2)^-. \end{align}

If we set $a=\frac {1}{2}-t$, then $a\to 0^+$ as $t\to (1/2)^-$, and since $[(1-t)^{s/2}-t^{s/2}]\ge ca$ as $a\to 0$, with $c>0$, (4.10) can be estimated by

\begin{align*} & a\,\int_{\mathbb{R}^N\setminus B_1(0)} |y|^{-\frac{N-1}{2}}\,e^{{-}ca|y|}\, e^{-\left(\frac{1}{2}-a\right)^{s/2}(|y|-y_1)}\,{\rm d}y\\ & \quad \le a\,\int_{\mathbb{R}^N\setminus B_1(0)} |y|^{-\frac{N-1}{2}}\,e^{{-}ca|y|}\, e^{-\left(\frac 14\right)^{s/2}(|y|-y_1)}\,{\rm d}y. \end{align*}

Making use of spherical coordinates in the subspace $e_1^{\perp }=\left \{v\in \mathbb {R}^N\ :\ v\cdot e_1=0\right \}$, denoting by $b=(\frac 14)^{s/2}$ and $\mathbb {R}^2_+=\mathbb {R}\times [0,+\infty )$, we have to analyse

\begin{align*} & a\int_{\mathbb{R}^2_+{\setminus} B_1(0)} \frac{r^{N-2}}{\left(r^2+y_1^2\right)^{\frac{N-1}{4}} \,e^{ca\left(r^2+y_1^2\right)^{1/2}}\, e^{b\left[\left(r^2+y_1^2\right)^{1/2}-y_1\right]}}\,dr\,{\rm d}y_1\\ & \quad=a\int_0^{\pi}\left(\int_1^{+\infty} \frac{\rho^{(N-1)/2}}{e^{[ca+b(1-\cos{\vartheta})]\rho}}\,{\rm d}\rho\right)\, (\sin{\vartheta})^{N-2}\,{\rm d}\vartheta. \end{align*}

Setting $k=ca+b(1-\cos {\vartheta })$, we can estimate

\begin{align*} \int_1^{+\infty} \frac{\rho^{(N-1)/2}}{e^{[ca+b(1-\cos{\vartheta})]\rho}}\,{\rm d}\rho & = \frac{1}{k^{(N-1)/2}}\,\int_1^{+\infty} \frac{(k\rho)^{(N-1)/2}}{e^{k\rho}}\,{\rm d}\rho\nonumber\\ & \le \frac{1}{k^{(N+1)/2}}\,\int_0^{+\infty} \frac{\mu^{(N-1)/2}}{e^{\mu}}\,{\rm d}\mu. \end{align*}

Then,

\begin{align*} & a\int_0^{\pi}\left(\int_1^{+\infty} \frac{\rho^{(N-1)/2}}{e^{[ca+b(1-\cos{\vartheta})]\rho}}\,{\rm d}\rho\right)\, (\sin{\vartheta})^{N-2}\,{\rm d}\vartheta\\ & \quad \leq C a\int_0^{\pi} \frac{(\sin{\vartheta})^{N-2}}{[ca+b(1-\cos{\vartheta})]^{(N+1)/2}}\,{\rm d}\vartheta. \end{align*}

Since $1-\cos {\vartheta }\ge \frac {1}{4}\sin ^2{\vartheta }$ for small $\vartheta$, if we set $u=\sin {\vartheta }$ and $\beta =b/(4c)$, then it is sufficient to evaluate

\begin{align*} & a\int_0^{\frac 12} \frac{u^{N-2}}{[a+\beta u^2]^{(N+1)/2}}\cdot \frac{1}{(1-u^2)^{1/2}}\,du \\ & \quad \leq \widehat{C} a^{- {1}/{2}} \int_0^{\frac 12} \frac{\left(\sqrt{\beta/a}\,u\right)^{N-2}} {\left[1+\left(\sqrt{\beta/a}\,u\right)^2\right]^{(N+1)/2}}\,du\\ & \quad =\widetilde{C} \int_0^{\frac 12\,\sqrt{\frac \beta a}} \frac{t^{N-2}}{\left(1+t^2\right)^{(N+1)/2}}\,dt\to C\quad\mbox{ as }a\to 0, \end{align*}

and the assertion follows.

Proof Proof of proposition 4.1

In this proof we shall consider $R>1$ fixed such that $\mathbb {R}^N\setminus \Omega \subset B_{R-1}(0)$.

In order to simplify the notations, we often omit $t,z$ and write $\psi _r=\psi _r[t,z]$, $\delta (r)=\delta _t(r)$, $\sigma (r)=\sigma _t(r)$, $\tau (r)=\tau _t(r)$ (see (4.2), (4.7), (4.8), (4.9)).

We have that

\[ E(\psi_r)=\frac{1}{2}\int_{\mathbb{R}^N} \left[|\nabla\psi_r|^2+V(x)\psi_r^2\right] {\rm d}\,x -\frac{1}{p}\int_{\mathbb{R}^N}|\psi_r|^p{\rm d}\,x . \]

So, to get the statement of the proposition, we need to estimate these two integrals.

Let us consider $0\leq t\leq 1/2$. In an entirely analogous way we may treat also the case $1/2< t\leq 1$.

Let us set:

\[ w_1=w_{t\rho^2}, \quad w_2=w_{(1-t)\rho^2}, \quad \lambda_1=\lambda_{t\rho^2}=t^{{ s}}\lambda_{\infty}, \quad \lambda_2=\lambda_{(1-t)\rho^2}=(1-t)^{{ s}}\lambda_{\infty}, \]

and for any $i=1,2$

\[ \quad A_i=|\nabla w_i|_2^2, \quad \quad B_i=|w_i|_p^p. \]

Recall that

\[ -\Delta w_i+\lambda_i w_i=w_i^{p-1}, \]

namely

(4.11)\begin{align} \int_{\mathbb{R}^N} \nabla w_i(x)\cdot \nabla v(x)\,{\rm d}\,x & + \lambda_i\int_{\mathbb{R}^N} w_i(x)\,v(x)\,{\rm d}\,x\notag\\ & = \int_{\mathbb{R}^N} w_i^{p-1}(x)\,v(x)\,{\rm d}\,x, \quad \forall v\in H^1(\mathbb{R}^N), \end{align}

and so

(4.12)\begin{equation} A_i-B_i={-}\lambda_i|w_i|_2^2. \end{equation}

Evidently for $t=\frac {1}{2}$ we have $w_1=w_2$, $\lambda _1=\lambda _2$, $A_1=A_2$, $B_1=B_2$. Moreover, we recall that

(4.13)\begin{equation} \frac{1}{2}A_1-\frac{1}{p}B_1=m_{t\rho^2}=t^{1+{ s}}m, \quad \frac{1}{2}A_2-\frac{1}{p}B_2=m_{(1-t)\rho^2}=(1-t)^{1+{ s}}m. \end{equation}

With these notations,

\[ \psi_r(x)= \rho\,\frac{\vartheta(x)\left[w_1(x-r z)+w_2(x-r e_1)\right]} {\left|\vartheta({\cdot})\left[w_1({\cdot}{-}r z)+w_2({\cdot}{-}r e_1)\right]\right|_2}. \]

Estimate of $\displaystyle {\left |\vartheta (\cdot )[w_1(\cdot -r z)+w_2(\cdot -r e_1)]\right |_2}$.

From above

(4.14)\begin{equation} \begin{aligned} \left|\vartheta({\cdot})\left[w_1({\cdot}{-}r z)+w_2({\cdot}{-}r e_1)\right]\right|_2^2 & \leq\left|w_1({\cdot}{-}r z)+w_2({\cdot}{-}r e_1)\right|_2^2 \\ & =|w_1|_2^2+|w_2|_2^2 +2\int_{\mathbb{R}^N} w_1(x-r z)\,w_2(x-r e_1)\,{\rm d}\,x \\ & =\rho^2+2\int_{\mathbb{R}^N} w_1(x-r z)\,w_2(x-r e_1)\,{\rm d}\,x \\ & =\rho^2(1+\tau(r)). \end{aligned} \end{equation}

From below,

(4.15)\begin{equation} \begin{aligned} \left|\vartheta({\cdot})\left[w_1({\cdot}{-}r z)+w_2({\cdot}{-}r e_1)\right]\right|_2^2 & =\int_{\mathbb{R}^N} \left|\vartheta(x)\left[w_1(x-r z)+w_2(x-r e_1)\right]\right|^2\,{\rm d}\,x\\ & \geq \int_{\mathbb{R}^N} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^2\,{\rm d}\,x\\ & \phantom{\ge}-\int_{B_{R}(0)} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^2\,{\rm d}\,x. \end{aligned} \end{equation}

By the asymptotic behaviour of $w_1$ and $w_2$ (see (2.9)), for any $q\geq 2$ we have

(4.16)\begin{align} & \int_{B_{R}(0)} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^q\,{\rm d}\,x\nonumber\\ & \quad \leq 2^{q-1} \int_{B_{R}(0)} \left[|w_1(x-r z)|^q+|w_2(x-r e_1|^q\right]\,{\rm d}\,x=o(\delta(r)). \end{align}

Therefore, by (4.14), (4.15) and (4.16) we get

(4.17)\begin{equation} \left|\vartheta({\cdot})\left[w_1({\cdot}{-}r z)+w_2({\cdot}{-}r e_1)\right]\right|_2^2\geq \rho^2(1+\tau(r))+o(\delta(r)). \end{equation}

Estimate of $\displaystyle {\int _{\mathbb {R}^N} [|\nabla \psi _r|^2+V(x)\psi _r^2]\,{\rm d}\,x}$.

Now, let us estimate

(4.18)\begin{align} & \int_{\mathbb{R}^N} \left|\nabla\Big(\vartheta(x)\left[w_1(x-r z)+w_2(x-r e_1)\right]\Big)\right|^2\,{\rm d}\,x\nonumber\\ & \le\int_{\mathbb{R}^N} \left(\left|\nabla\left[w_1(x-r z)+w_2(x-r e_1)\right]\right|^2 \right. \nonumber\\ & \quad+2 \int_{\mathbb{R}^N} \Big[\vartheta(x) \nabla\vartheta(x)\Big]\cdot \Big(\left[w_1(x-r z)+w_2(x-r e_1)\right] \nabla [w_1(x-r z)\nonumber\\ & \quad +w_2(x-r e_1)]\Big)\,{\rm d}\,x \nonumber\\ & \quad+ \int_{\mathbb{R}^N} |\nabla \vartheta(x)|^2\,\left[w_1(x-r z)+w_2(x-r e_1)\right]^2\,{\rm d}\,x. \end{align}

By direct computation, (4.11) and (4.12), we obtain

(4.19)\begin{align} & \int_{\mathbb{R}^N} \left|\nabla\left[w_1(x-r z)+w_2(x-r e_1)\right]\right|^2\,{\rm d}\,x \nonumber\\ & \quad=\left|\nabla w_1\right|_2^2+\left|\nabla w_2\right|_2^2 +2\int_{\mathbb{R}^N} \nabla w_1(x-r z)\cdot \nabla w_2(x-r e_1)\,{\rm d}\,x \nonumber\\ & \quad=\left|\nabla w_1\right|_2^2+\left|\nabla w_2\right|_2^2 -(\lambda_1+\lambda_2)\int_{\mathbb{R}^N} w_1(x-r z)\,w_2(x-r e_1)\,{\rm d}\,x \nonumber\\ & \qquad+\int_{\mathbb{R}^N} \left[w^{p-1}_1(x-r z)\,w_2(x-r e_1)+w_1(x-r z)\,w_2^{p-1}(x-r e_1)\right]\,{\rm d}\,x\nonumber \\ & \quad=A_1+A_2-(\lambda_1+\lambda_2)\frac{\rho^2}{2}\tau(r)+\sigma(r). \end{align}

Since $\nabla \vartheta \equiv 0$ on $\mathbb {R}^N\setminus B_R(0)$, by using (2.9) (2.10) we get

(4.20)\begin{align} & \int_{\mathbb{R}^N} |\nabla \vartheta(x)|^2\,\left[w_1(x-r z)+w_2(x-r e_1)\right]^2\,{\rm d}\,x\nonumber\\ & \quad \le c \int_{B_R(0)} \left[w_1(x-r z)+w_2(x-r e_1)\right]^2\,{\rm d}\,x\nonumber\\ & \quad =o(\delta(r)) \end{align}

and

(4.21)\begin{align} & \left|\int_{\mathbb{R}^N} \Big[\vartheta(x) \nabla\vartheta(x)\Big]\cdot \Big([w_1(x-r z)+w_2(x-r e_1)] \nabla [w_1(x-r z)+\,w_2(x-r e_1)]\Big)\,{\rm d}\,x\,\vphantom{\int_{\mathbb{R}^N}}\right|\notag\\ \nonumber\\ & \qquad\le c \int_{B_R(0)} \Big|\left[w_1(x-r z)+w_2(x-r e_1)\right] \nabla \left[w_1(x-r z)+w_2(x-r e_1)\right]\Big|\, {\rm d}\,x\nonumber\\ & \qquad= o(\delta(r)). \end{align}

According to the contribution of the potential, by (D _ρ), (2.9) and by lemma 4.4 we have

(4.22)\begin{align} & \int_{\mathbb{R}^N} V(x)\left|\,\vartheta(x)\left[w_1(x-r z)+w_2(x-r e_1)\right]\right|^2\,{\rm d}\,x\nonumber\\ & \quad\leq \int_{ \mathbb{R}^N} V(x)\Big[w_1(x-r z)+w_2(x-r e_1)\Big]^2\,{\rm d}\,x =o(\delta(r)). \end{align}

By (4.17)–(4.22) we deduce

(4.23)\begin{align} & \int_{\mathbb{R}^N} \left[|\nabla\psi_r|^2+V(x)\psi_r^2\right]\,{\rm d}\,x\nonumber\\ & \quad \leq \frac{1}{1+\tau(r)+o(\delta(r))} \left[A_1+A_2-(\lambda_1+\lambda_2)\frac{\rho^2}{2}\tau(r)+\sigma(r)+o(\delta(r))\right]. \end{align}

Estimate of $\int _{\mathbb {R}^N}|\psi _r|^p{\rm d}\,x$.

Since $0\le \vartheta (x)\le 1$ in $\mathbb {R}^N$ and $\vartheta \equiv 1$ in $\mathbb {R}^N\setminus B_{R}(0)$, by (4.16) and by lemma 4.3, we get

(4.24)\begin{align} & \left|\vartheta({\cdot})\left[w_1({\cdot}{-}r z)+w_2({\cdot}{-}r e_1)\right]\right|_p^p = \int_{\mathbb{R}^N} \Big|\vartheta(x)\left[w_1(x-r z)+w_2(x-r e_1)\right]\Big|^p\,{\rm d}\,x\nonumber\\ & \begin{aligned} & \quad\geq \int_{\mathbb{R}^N} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^p\,{\rm d}\,x-\int_{B_{R}(0)} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^p\,{\rm d}\,x \\ & \quad\geq\left|w_1\right|_p^p+\left|w_2\right|_p^p\\ & \qquad + (p-1)\int_{\mathbb{R}^N} \left[w^{p-1}_1(x-r z)\,w_2(x-r e_1)+w_1(x-r z)\,w_2^{p-1}(x-r e_1)\right]\,{\rm d}\,x\\ & \qquad-\int_{B_{R}(0)} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^p\,{\rm d}\,x\\ & \quad= B_1+B_2+(p-1)\sigma(r)-\int_{B_{R}(0)} \Big|w_1(x-r z)+w_2(x-r e_1)\Big|^p\,{\rm d}\,x \\ & \quad\geq B_1+B_2+(p-1)\sigma(r)+o(\delta(r)). \end{aligned} \end{align}

Taking into account (4.14) and (4.24) we have that

(4.25)\begin{equation} |\psi_r|_p^p \geq \left(\frac{1}{1+\tau(r)}\right)^{p/2}\left[B_1+B_2+(p-1)\sigma(r)+o(\delta(r))\right]. \end{equation}

Estimate of $E(\psi _r)$.

Therefore

\begin{align*} E(\psi_r)& =\frac{1}{2}\int_{\mathbb{R}^N} \left[|\nabla\psi_r|^2+V(x)\psi_r^2\right]\,{\rm d}\,x -\frac{1}{p}|\psi_r|_p^p\leq \\ & \leq\frac{1}{2}\left(\frac{1}{1+\tau(r)+o(\delta(r))}\right) \left[A_1+A_2-(\lambda_1+\lambda_2)\frac{\rho^2}{2}\tau(r)+\sigma(r)+o(\delta(r))\right] \\ & \quad-\frac{1}{p}\left(\frac{1}{1+\tau(r)}\right)^{p/2}\left[B_1+B_2+(p-1)\sigma(r)+o(\delta(r))\right]. \end{align*}

Observe that for every $\sigma,\tau >0$ and $\delta \to 0$ we have

\begin{align*} & \frac{1}{2}\left(\frac{1}{1+\tau+o(\delta)}\right) \left[A_1+A_2-(\lambda_1+\lambda_2)\frac{\rho^2}{2}\tau+\sigma+o(\delta)\right]\\ & \quad- \frac{1}{p}\left(\frac{1}{1+\tau}\right)^{\frac p 2}\left[B_1+B_2+(p-1)\sigma+o(\delta )\right]\\ & \quad=\varphi(\tau,\sigma)+o(\delta ), \end{align*}

where

\begin{align*} \varphi(\tau,\sigma)& =\frac{1}{2}\left(\frac{1}{1+\tau}\right)\left[A_1+A_2-(\lambda_1+\lambda_2)\frac{\rho^2}{2}\tau+\sigma\right] \\ & \quad -\frac{1}{p}\left(\frac{1}{1+\tau}\right)^{\frac p2}\left[B_1+B_2+(p-1)\sigma\right], \end{align*}

and we have performed a Taylor expansion with respect to $o(\delta )$. We will write $\varphi _t$ in place of $\varphi$ when we want to emphasize the role of $t$.

Now, we are going to consider the Taylor expansion of $\varphi$. Observe that these expansions are consistent by (1) and (2) in lemma 4.5.

By (4.13), and taking into account (4.12), we have that

(4.26)\begin{align} \varphi(0,0)& =\left(\frac{1}{2}A_1-\frac{1}{p}B_1\right)+\left(\frac{1}{2}A_2-\frac{1}{p}B_2\right) =\left[t^{1+{ s}}+(1-t)^{1+{ s}}\right]m, \end{align}

(4.27)\begin{align}\displaystyle{\frac{\partial \varphi}{\partial \tau}(0,0)} & = \displaystyle{-\frac{1}{2}\left[A_1+A_2+(\lambda_1+\lambda_2)\frac{\rho^2}{2}-B_1-B_2\right]} \nonumber\\ & =\displaystyle{-\frac{1}{2}\left[A_1-B_1+\lambda_1\frac{\rho^2}{2}\right] -\frac{1}{2}\left[A_2-B_2+\lambda_2\frac{\rho^2}{2}\right]} \nonumber\\ & =\displaystyle{-\frac{1}{2}\left[-\lambda_1 t\rho^2+\lambda_1\frac{\rho^2}{2}\right] -\frac{1}{2}\left[-\lambda_2 (1-t)\rho^2+\lambda_2\frac{\rho^2}{2}\right]}\nonumber\\ & =\displaystyle{-\frac{1}{2}\lambda_1 \rho^2\left(\frac{1}{2}-t\right) +\frac{1}{2}\lambda_2 \rho^2\left(\frac{1}{2}-t\right)} \nonumber\\ & =\displaystyle{\frac{1}{2}\left(\frac{1}{2}-t\right)\left(\lambda_2-\lambda_1\right) \rho^2}. \end{align}

(4.28)\begin{align} \frac{\partial \varphi}{\partial \sigma}(0,0) & ={-}\left(\frac{1}{2}-\frac{1}{p}\right). \end{align}

Hence, we obtain

\begin{align*} \varphi(\tau,\sigma)&= \varphi(0,0)+\frac{1}{2}\left(\frac{1}{2}-t\right)\left(\lambda_2-\lambda_1\right) \rho^2\tau-\left(\frac{1}{2}-\frac{1}{p}\right)\sigma+o\left(\sqrt{\tau^2+\sigma^2}\right), \\ & \qquad \mbox{as }(\tau,\sigma)\to (0,0). \end{align*}

Now, we want to analyse the asymptotic behaviour of $\varphi (\tau (r),\sigma (r))$ and, as a consequence, of $E(\psi _r)$, as $r\to \infty$.

By lemma 4.5, for every $t\in [0,\frac 12)$ we have

\begin{align*} \varphi(\tau_t(r),\sigma_t(r)) &= \displaystyle{\varphi(0,0)+\left[\frac{1}{2}\left(\frac{1}{2}-t\right)\left(\lambda_2-\lambda_1\right) \rho^2c_{1,t} -\left(\frac{1}{2}-\frac{1}{p}\right)c_{2,t}\right]\delta_t(r)}\notag\\ &\quad +o(\delta_t(r)),\quad \mbox{as $r\to \infty$}. \end{align*}

Taking into account lemma 4.5 and $\lambda _1\to \lambda _2$ as $t\to \frac 12$, we get

\begin{align*}&\left[\frac{1}{2}\left(\frac{1}{2}-t\right)\left(\lambda_2-\lambda_1\right) \rho^2c_{1,t} -\left(\frac{1}{2}-\frac{1}{p}\right)c_{2,t}\right]\mathop{\longrightarrow}-\left(\frac 12 -\frac 1p\right) C_2<0,\\ &\qquad\mbox{ as }t\to\left(\frac 12\right)^-.\end{align*}

Then, for suitable constants $C>0$ and $\mu \in ( 0,\frac 12)$

\[ E(\psi_r[t,z])\le [t^{1+s}+(1-t)^{1+s}]\, m-C\delta_t(r)+o(\delta_t(r))\quad\forall t\in\left[\mu, 1/2\right) \]

(see (4.26). Since $\delta _t(r)\to 0$ as $r\to \infty$, uniformly in $t$ (see (4.7)), we can conclude that there exists $r_1>0$ such that

(4.29)\begin{equation} E(\psi_r[t,z])<\max_{t\in [0,1]}[t^{1+s}+(1-t)^{1+s}]\, m=2^{{-}s}m\qquad\forall t\in\left[\mu, 1/2\right),\quad \forall r>r_1. \end{equation}

If $t=\frac 12$, for suitable $\alpha,\beta,\gamma \in \mathbb {R}$ we infer

(4.30)\begin{align}\varphi(\tau,\sigma)&= \varphi(0,0)-\left(\frac{1}{2}-\frac{1}{p}\right)\sigma+ \alpha\tau^2+\beta\sigma^2+\gamma\sigma\tau+o\left(\tau^2+\sigma^2\right), \notag\\ &\quad\quad\mbox{as }(\tau,\sigma)\to (0,0). \end{align}

Now, consider that by lemma 4.5

(4.31)\begin{equation} \sigma_{1/2}(r)=(c_{2,\frac 12}+o(1))\delta_{1/2}(r) \end{equation}

and that, fixed $\eta \in (0,\sqrt {2^{-{ s}}\lambda _{\infty }}/2]$, by lemma 4.4

\[ \tau_{1/2}(r)=o\left(e^{{-}2\left(\sqrt{2^{-{ s}}\lambda_{\infty}} - \eta\right) r}\,r^{-\frac{N-1}{2}}\right), \quad\mbox{ as } r\to +\infty, \]

so that

(4.32)\begin{equation} \left(\tau_{1/2}(r)\,\right)^2=o\left(\delta_{1/2}(r)\right). \end{equation}

By (4.30), (4.31), (4.32), we can conclude that there exists $r_2>0$ such that

(4.33)\begin{equation} E(\psi_r[1/2,z])=\varphi(0,0)-C\left(\frac 12-\frac 1p\right)\delta_{1/2}(r)+o\left(\delta_{1/2}(r)\right)<2^{{-}s}m\qquad\forall r>r_2. \end{equation}

If $t\in [0,\mu ]$, then, taking into account that $\delta _t(r)\le \delta _0(r)$ for every $t\in [0,1/2]$, we get

\begin{align*} E(\psi_r[t,z]) & \le \varphi_t(0,0)+O\left(\delta_0(r)\right)\\ & \le [t^{1+s}+(1-t)^{1+s}]m+O\left(\delta_0(r)\right)\\ & \le [\mu^{1+s}+(1-\mu)^{1+s}]m+O\left(\delta_0(r)\right)\quad \forall t \in[0,\mu]. \end{align*}

Since $[\mu ^{1+s}+(1-\mu )^{1+s}]m<2^{-s}m$, there exists $r_3>0$ such that

(4.34)\begin{equation} E(\psi_r[t,z])<2^{{-}s}m\qquad\forall t\in[0,\mu],\quad\forall r>r_3. \end{equation}

So, assertion $(a)$ follows from (4.29), (4.33), (4.34), for every $r>\bar r:=\max \{r_1,r_2,r_3\}$.

The estimates developed above also show that

\[ E(\psi_r[0,z])\le\varphi_0(0,0)+o\left(\delta_0(r)\right)=m+o\left(\delta_0(r)\right) \]

so that $E(\psi _r[0,z])\mathop {\longrightarrow } m$, as $r\to \infty$. The same arguments work to evaluate

\[ E(\psi_r[1,z])\le m+o\left(\delta_0(r)\right), \]

uniformly in $z\in \Sigma$. So, also $(b)$ is proved.

4.2 Other estimates

In this subsection we consider the nonautonomous case $\Omega \neq \mathbb {R}^N$ or $V\not \equiv 0$.

The following definition of barycentre of a function $u \in H^1(\mathbb {R}^N)\setminus \left \lbrace 0 \right \rbrace,$ has been introduced in [Reference Cerami and Passaseo15]. We set

(4.35)\begin{equation} \mu(u)(x)=\frac{1}{|B_1(0)|}\int_{B_1(x)}|u(y)|{\rm d}y \end{equation}

and we remark that $\mu (u)$ is bounded and continuous, so we can introduce the function

(4.36)\begin{equation} \hat{u}(x)=\left[\mu(u)(x)-\frac{1}{2}\max \mu(u)\right]^+, \end{equation}

that is continuous and has compact support. Thus, we can set $\beta : H^1(\mathbb {R}^N)\setminus \{0\}\rightarrow \mathbb {R}^N$ as

\[ \beta(u)=\frac{1}{|\hat{u}|_{1}}\int_{\mathbb{R}^N}\hat{u}(x)\, x\, {\rm d}\,x. \]

The map $\beta$ has the following properties:

(4.37)\begin{align} & \beta \mbox{ is continuous in }H^1(\mathbb{R}^N)\setminus \{0\}; \end{align}

(4.38)\begin{align} & \mbox{if } u\mbox{ is a radial function, then }\beta(u)=0; \end{align}

(4.39)\begin{align} & \beta(tu)=\beta(u)\quad \forall t\in\mathbb{R}\setminus\{0\},\quad \forall u\in H^1(\mathbb{R}^N)\setminus \{0\}; \end{align}

(4.40)\begin{align} & \beta(u(x-z))=\beta(u)+z\qquad \forall z\in\mathbb{R}^N\quad \forall u\in H^1(\mathbb{R}^N)\setminus \{0\}. \end{align}

Let us set

\[ C_0=\inf\{E(u):\quad u\in H^1_0(\Omega),\quad |u|_2=\rho,\ \beta(u)=0\}. \]

Lemma 4.7 We have that $C_0>m$.

Proof Of course we have that $C_0\geq m$. Assume by contradiction that $C_0=m$. Then by corollary 3.3 there exists a sequence $(y_n)_n$ in $\mathbb {R}^N$ such that $|y_n|\mathop {\longrightarrow } \infty$, as $n\to \infty$, and

\[ u_n(x)=w(x-y_n)+\phi_n(x),\qquad \phi_n\to 0\ \mbox{ strongly in }H^1(\mathbb{R}^N). \]

By (4.37), (4.38) and (4.40) we have

\[ 0=\beta(u_n)=\beta(w({\cdot}{-}y_n)+\phi_n)=\beta(w+\phi({\cdot}{+}y_n))+y_n=y_n+o(1), \]

contrary to $|y_n|\mathop {\longrightarrow } \infty$, as $n\to \infty$.

So the proof is completed

Lemma 4.8 Let ${\mathcal {A}}_r$ and ${\mathcal {L}}_r$ be as in proposition 4.1. Then $\widehat {r}>0$ exists such that

(4.41)\begin{equation} {\mathcal{L}}_r< C_0\leq {\mathcal{A}}_r,\qquad\mbox{ for all }r\geq \widehat{r}. \end{equation}

Proof Inequality ${\mathcal {L}}_r< C_0$, for large $r$, follows from (4.4) and lemma 4.7.

To get the second inequality in (4.40), we claim that $\beta (\vartheta (\cdot )w(\cdot -rz))\cdot z >0$ for all $z\in \Sigma$, for large $r$. Indeed, by (4.36),(4.37) and (4.39) we have

\[ \Big|\beta\big(\vartheta({\cdot})w({\cdot}{-}rz)\big)-rz\Big|= \Big|\beta\big(\vartheta({\cdot}{+}rz)\, w\big)\Big|\xrightarrow[]{r\rightarrow \infty}0, \]

because $\vartheta (\cdot +rz)w\to w$ in $H^1$ as $r\to \infty$, by (2.3) and (2.4). Hence

\[ \beta(\vartheta({\cdot})w({\cdot}{-}rz))=r z+o(1)\qquad\mbox{ as }r\to\infty, \]

as asserted. So, for $r$ large, the deformation ${\mathcal {G}}:[0,1]\times \Sigma \to \mathbb {R}^N\setminus \{0\}$ given by

(4.42)\begin{equation} {\mathcal{G}}(s,z)=s\beta(\psi_r[1,z])+(1-s)\,z \end{equation}

is well defined. Then we claim that there exists $(t_r,z_r)\in [0,1]\times \Sigma$ such that

(4.43)\begin{equation} \beta(\psi_r[t_r,z_r])=0. \end{equation}

Indeed, by the continuity of the maps $\beta$ and $\psi _r$, by ${\mathcal {G}}(s,z)\neq 0$, $\forall (s,z)\in [0,1]\times \Sigma$, and by the invariance of the topological degree by homotopy, we have

\[ 0\neq d(\mathop{\rm Id}\nolimits,\Sigma\times[0,1),0)=d(\beta\circ\psi_r,\Sigma\times[0,1),0). \]

From (4.43) there follows $C_0\leq E(\psi _r[t_r,z_r])\leq {\mathcal {A}}_r$, that completes the proof.

5. Proof of the main results

The existence of a positive solution for the autonomous case $\Omega =\mathbb {R}^N$ and $V\equiv 0$ is well known, so we prove our results when $\Omega =\mathbb {R}^N$ and $V\equiv 0$ does not occur.

Proof Proof of Theorem 1.1

Let us recall the values

(5.1)\begin{align} {\mathcal{A}}_r& =\max\left\{E\left(\psi_{r}[t,z]\right):\quad t\in [0,1],\, z\in\Sigma\right\}, \end{align}

(5.2)\begin{align} {\mathcal{L}}_r& =\max\{E\left(\psi_r[1,y]\right):\quad z\in \Sigma\}, \end{align}

(5.3)\begin{align} C_0& =\inf\{E(u):\quad u\in H^1_0(\Omega),\, |u|_2=\rho,\, \beta(u)=0\}. \end{align}

By propositions 4.1 and 1.4 (see (2.11)), and by lemma 4.8, we have that for all $r>\max \{\bar {r},\widehat {r}\}$

(5.4)\begin{equation} m<{\mathcal{L}}_r< C_0\leq {\mathcal{A}}_r <2^{{-}s}m. \end{equation}

We claim that the functional $E$ has a (PS)-sequence in $[C_0,{\mathcal {A}}_r]$. This done, the existence of a critical point $\bar u$ of $E$ on $S_{\rho }$ with $E(\bar u)\le {\mathcal {A}}_r$ follows from proposition 3.1.

Assume, by contradiction, that no (PS)-sequence exists in $[C_0,{\mathcal {A}}_r]$. Then, usual deformation arguments imply the existence of $\eta >0$ such that the sublevel $E^{C_0-\eta }:=\{u\in H^1_0(\Omega ):\quad |u|_2^2=\rho ^2,\, E(u)\leq C_0-\eta \}$ is a deformation retract of the sublevel $E^{{\mathcal {A}}_r}:=\{u\in H^1_0(\Omega ):\quad |u|_2^2=\rho ^2,\, E(u)\leq {\mathcal {A}}_r\}$, namely there exists a continuous function $\varphi :E^{{\mathcal {A}}_r}\to E^{C_0-\eta }$ such that

(5.5)\begin{equation} \varphi(u)=u\qquad\mbox{ for any }u\in E^{C_0-\eta}. \end{equation}

Furthermore, by (5.4) we can also assume $\eta$ so small that

(5.6)\begin{equation} C_0-\eta>{\mathcal{L}}_r. \end{equation}

Let us define the map ${\mathcal {H}}:[0,1]\times \Sigma \to \mathbb {R}^N$ by

\[ {\mathcal{H}}(s,y)=\beta\left(\varphi\big(\psi_r[t,z]\big)\right). \]

By (5.6), (5.5), and by using the map ${\mathcal {G}}$ introduced in (4.42), we deduce that ${\mathcal {H}}$ maps $\{1\}\times \Sigma$ in a set homotopically equivalent to $\Sigma$ in $\mathbb {R}^N\setminus \{0\}$. Since ${\mathcal {H}}$ is a continuous map, and arguing exactly as for (4.43), we get the existence of a point $(\tilde t,\tilde z)\in [0,1]\times \Sigma$ such that

\[ 0={\mathcal{H}}(\tilde t,\tilde z)=\beta(\varphi(\psi_r[\tilde t,\tilde z])). \]

Then by the definition of $C_0$ we see $E(\varphi (\psi _r[\tilde t,\tilde z]))\ge C_0$, contrary to $\varphi (\psi _r[t,z])\in E^{C_0-\eta }$ for every $(t,z)\in [0,1]\times \Sigma$, so the claim must be true.

Finally, since $E(\bar u)\in (m,2^{-s}m)$ then $\bar u$ has constant sign by proposition 2.2. Observe that since $\bar u$ solves (P) if and only if $-\bar u$ solves (P), then we have a nonnegative solution.

Proof Proof of Theorem 1.2

This proof proceed in two different ways, according to $\Omega \neq \mathbb {R}^N$ or $\Omega =\mathbb {R}^N$. In both cases, we identify a topological configuration analogous to the one employed in the proof of theorem 1.1 to get the solution. We only outline the procedure, because the argument is the same already developed in details.

Case $\Omega \neq \mathbb {R}^N$.

Let us apply proposition 4.1, lemma 4.7 and lemma 4.8 with $V\equiv 0$. Then for a fixed $r>0$, large enough, we get

(5.7)\begin{equation} m<{\mathcal{L}}_{r,0}< C_{0,0}\leq {\mathcal{A}}_{r,0} <2^{{-}s}m, \end{equation}

where ${\mathcal {L}}_{r,0}, C_{0,0},\ {\mathcal {A}}_{r,0}$ are defined as in (5.1), (5.2), (5.3), with the functional $E$ replaced by

\[ E_0(u):=\frac 12 \int_\Omega|\nabla u|^2{\rm d}\,x-\frac 1p \int_\Omega|u|^p{\rm d}\,x,\qquad u\in H^1_0(\Omega). \]

The configuration in (5.7) depends on $\Omega$ and $\rho$.

Now, observe that ${\mathcal {L}}_{r,0}\le {\mathcal {L}}_{r}$, $C_{0,0}\le C_{0}$, ${\mathcal {A}}_{r,0}\le {\mathcal {A}}_{r}$, by (1.1). Moreover, by the Hölder inequality and (2.9), it follows that

\[ \lim_{|V|_q\to 0}\int_{\mathbb{R}^N}V(x)\left(\psi_{r}[t,z]\right)^2 {\rm d}\,x\le \lim_{|V|_q\to 0}|V|_q\max_{t\in[0,1],\ z\in\Sigma}|(\psi_r[t,z])^2|_{q'}=0, \]

uniformly in $t\in [0,1]$ and $z\in \Sigma$, that implies

\[ \lim_{|V|_q\to0}{\mathcal{L}}_{r}= {\mathcal{L}}_{r,0},\quad \lim_{|V|_q\to0}{\mathcal{A}}_{r}= {\mathcal{A}}_{r,0}. \]

Hence, taking also into account lemma 4.8, we see that there exists $L=L(\Omega,\rho )$ such that if $|V|_q< L$ then the configuration (5.4) is restored. In particular,

(5.8)\begin{equation} L<\frac{1}{\max\limits_{t\in[0,1],\ z\in\Sigma}|(\psi_r[t,z])^2|_{q'}}\, (2^{{-}s}m-{\mathcal{A}}_{r,0}). \end{equation}

As a consequence, if $|V|_q< L$ we get a critical value for $E$ constrained on $S_\rho$, in the energy range $(m,2^{-s}m)$.

Again, since the solution $\bar u$ we have found verifies $E(\bar u)\in (m,2^{-s}m)$, it is a constant sign solution by proposition 2.2.

Case $\Omega =\mathbb {R}^N$.

Let $r>0$ and let us introduce the values

(5.9)\begin{align} \widetilde {\mathcal{A}}_r& =\max\left\{E\left(w({\cdot}{-}y)\right):\quad y\in B_r(0)\right\} , \end{align}

(5.10)\begin{align} \widetilde {\mathcal{L}}_r& =\max\{E\left(w({\cdot}{-}y)\right):\quad y\in \partial B_r(0)\}. \end{align}

Then it turns out that for every fixed $V$ there exists $r_V>0$ such that for every $r> r_V$

(5.11)\begin{equation} m<\widetilde {\mathcal{L}}_r< C_0\le \widetilde {\mathcal{A}}_r. \end{equation}

By Hölder inequality, for every $y\in \mathbb {R}^N$

\[ E\big(w({\cdot}{-}y)\big)=m+\frac 12 \int_{\mathbb{R}^N} V(x)\, [w({\cdot}{-}y)]^2{\rm d}\,x\le m+\frac 12 |V|_q\, |w^2|_{q'}. \]

Hence, $\widetilde{\mathcal{A}}_r<2^{-s}m$ provided

(5.12)\begin{equation} \frac 12 |V|_q\, |w^2|_{q'}<\left(1-\frac{1}{2^s}\right)({-}m). \end{equation}

After some computation, by (2.7) with 1 in place of $\rho$ and $\rho ^2$ in place of $k$, we see that (5.12) is equivalent to

(5.13)\begin{equation} |V|_q\, \rho^{-\frac{2s}{q}\left( q-\frac N2\right)} < c , \end{equation}

for a suitable constant $c>0$ depending on $N,p$ and $q$. Hence, setting for example

(5.14)\begin{equation} L=\frac12 c \, \rho^{\frac{2s}{q}\left( q-\frac N2\right)} , \end{equation}

if $|V|_q< L$ then the inequalities (5.11) can be completed as

(5.15)\begin{equation} m<\widetilde {\mathcal{L}}_r< C_0\le \widetilde {\mathcal{A}}_r<2^{{-}s}m,\qquad \forall r>r_V. \end{equation}

Moreover, by (5.13) we obtain (1.7) and (1.8). Finally, by (5.15) we can argue as in the proof of theorem 1.1 to get the solution we are looking for.

Remark 5.1 Let $\rho >0$ be fixed. Arguing as in [Reference Molle and Passaseo32, Theorem 3.1], it is possible to verify that

\[ \lim_{r(\Omega)\to\infty}C_{0,0}= 2^{{-}s}m \]

(see(1.9)). Then by $C_{0,0}\le {\mathcal {A}}_{r,0}<2^{-s}m$ and (5.8) we obtain

\[ \lim_{r(\Omega)\to\infty} L=0. \]

6. The case ${\rm N}=1$

In this section we consider the 1-dimensional case

(P ₁)\[ \left\{ \begin{array}{@{}ll@{}} -u''+\lambda u+V(x)\, u=|u|^{p-2}u & \mbox{in }I, \\ \lambda\in\mathbb{R}, \quad u\in H^1_0(I) , \quad \displaystyle{\int_{I} u^2\,{\rm d}\,x=\rho^2}, \end{array} \right.\]

where we can consider $I=\mathbb {R}$ or $I=(0,\infty )$, $V\in L^q(I)$ for some $q\in [1,\infty ]$, $V\ge 0$ and $2< p<6$.

First, let us consider the entire case. For $N=1$, in the asymptotic behaviour of the limit function $w$ (see (2.3) and (2.4)) we cannot take advantage of the polinomial contribution provided by $|x|^{\frac {N-1}{2}}$. As a consequence, the key estimate (4.3) in proposition 4.1 does not hold and theorem 1.1 fails.

On the other hand, theorem 1.2 does not need proposition 4.1 and it can be again stated:

Sketch of the proof. This theorem can be proved exactly as theorem 1.2-case $\Omega =\mathbb {R}^N$: we introduce $\widetilde {\mathcal {A}}_r$, $C_0$ and $\widetilde {\mathcal {L}}_r$ (see (5.9), (5.3) and (5.10)) and then observe that for $|V|_q$ small $m <\widetilde {\mathcal {L}}_r< C_0\le \widetilde {\mathcal {A}}_r<2^{-s}m$. So, by proposition 3.1, we can argue by deformation as in the proof of theorem 1.1, getting the existence of a nonnegative solution $\bar u$. By proposition 1.3, the solution $\bar u$ is positive.

According to the exterior case, that is $I=(0,\infty )$, first let us state some nonexistence results.

Indeed, if $u$ is a solution of (P ₁), then it is a regular free solution of an equation of the form $-u''=f(u)$, $u(0)=0$, where $f(u)=-\lambda u+ |u|^{p-2}u$. Then $u\equiv 0$ by [Reference Esteban and Lions20, Remark I.3], contrary to $u\in S_\rho$.

Proposition 6.3 is a simplified version of proposition 1.5 in the 1-dimensional case, adapted also to half lines.

Proof We are assuming $V$ non increasing and $I=(0,\infty )$.

Suppose by contradiction that there exists a solution $u$ of (P ₁). Since the solutions of (P ₁) are in $H^2(I)$, we can consider a sequence $(u_n)_n$ in ${\mathcal {C}}^\infty _0((1/n,\infty ))$ such that $u_n\to u$ in $H^2(I)$. For every $n\in \mathbb {N}$, the map $t\mapsto u_n(x+t)$, $t\in (-1/n,\infty )$, turns out to be a smooth curve in $H^1(I)$ and we can consider the regular map $t\mapsto f_n(t):=E(u_n(x+t))$. Since $u$ is a solution of (P ₁), we have

(6.1)\begin{equation} f_n'(0)=E'(u_n)[u_n']=O(1). \end{equation}

From the monotonicity of $V$ and Fatou's lemma we infer

(6.2)\begin{align} f_n'(0) & =\lim_{t\to 0}\frac 1t\, \left[E\big(u_n({\cdot}{+}t)\big)-E\big(u_n\big)\right]\nonumber\\ & =\lim_{t\to 0^+}\frac{1}{2t}\int_{\frac 1n}^\infty V(x)[u_n^2(x+t)-u_n^2(x)]\, {\rm d}\,x\nonumber\\ & =\lim_{t\to 0^+}\frac 12\int_{\frac 1n}^\infty\frac{V(x-t)-V(x)}{t}\, u_n^2(x)\, {\rm d}\,x\nonumber\\ & \ge \frac 12\int_0^\infty ({-}V'(x))\, u_n^2(x)\, {\rm d}\,x \nonumber\\ & \ge 0. \end{align}

Letting $n\to \infty$ in (6.1) and (6.2), we obtain

\[ \int_0^\infty V'(x)u^2(x)\, {\rm d}\,x =0, \]

that is a contradiction. Indeed, by assumption $V'\le 0$ a.e. in $I$ and $V'<0$ on a set of positive measure, while $|\{x\in I\, :\, u(x)=0\}|=0$ because $u(x)=0$ implies $u'(x)\neq 0$ otherwise $u\equiv 0$ by the Cauchy-Lipschitz theorem.

If $I=\mathbb {R}$ we can proceed in a similar way.

Finally, let us state an existence result on half lines, that inherits the topological structure of the entire case.

Proof In order to get a solution, we are going to solve (P ₁) with $V$ fixed, on $H^1_0((-R,\infty ))$.

Let us consider (P ₁) on $H^1 (\mathbb {R})$, with $V$ fixed, and let us define $\widetilde {\mathcal {A}}_r$, $C_0$ and $\widetilde {\mathcal {L}}_r$ as in the proof of theorem 6.1. Moreover, let us fix a cut-off function $\widetilde \vartheta \in C^\infty (\mathbb {R},[0,1])$ such that $\widetilde \vartheta (x)=0$ $\forall x\in (-\infty,0)$, $\widetilde \vartheta (x)=1$ $\forall x\in (1,\infty )$ and introduce

\begin{align*} \widetilde {\mathcal{A}}_{r,R}& =\max\left\{E\left(\widetilde\vartheta({\cdot}{+}R)w({\cdot}{-}y)\right):\quad y\in [{-}r,r]\right\} ,\\ C_{0,R}& =\inf\{E(u)\ :\ u\in H^1({-}R,\infty),\ |u|_2=\rho,\ \beta (u)=0\},\\ \widetilde {\mathcal{L}}_{r,R}& =\max\big\{E\left(\widetilde\vartheta({\cdot}{+}R)w({\cdot}{-}y)\right):\quad y\in\{{-}r,r\}\big\}. \end{align*}

Then,

\[ \lim_{R\to\infty}\widetilde {\mathcal{A}}_{r,R}=\widetilde {\mathcal{A}}_{r},\qquad \lim_{R\to\infty}C_{0,R}=C_{0},\quad \lim_{R\to\infty}\widetilde {\mathcal{L}}_{r,R}=\widetilde {\mathcal{L}}_{r}. \]

Hence, for large $R$, we get

\[ m <\widetilde {\mathcal{L}}_{r,R}< C_{0,R}\le \widetilde {\mathcal{A}}_{r,R}<2^{{-}s}m \]

and we can argue as in the proof of theorem 1.1, by proposition 3.1.

Data

Data sharing not applicable to this article as no datasets were generated or analysed during the current study.