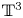Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-23T19:45:11.742Z Has data issue: false hasContentIssue false
  • English
  • Français

Global existence and large time behaviour for the pressureless Euler–Naver–Stokes system in ℝ3

Part of: Kinematics Equations of mathematical physics and other areas of application

Published online by Cambridge University Press:  22 February 2023

Shanshan Guo ,
Guochun Wu  and
Yinghui Zhang
Shanshan Guo
Affiliation:
School of Mathematical Sciences, Chongqing Normal University, Chongqing 400047, China ([email protected])
Guochun Wu
Affiliation:
Fujian Province University Key Laboratory of Computational Science, School of Mathematical Sciences, Huaqiao University, Quanzhou 362021, People's Republic of China ([email protected])
Yinghui Zhang*
Affiliation:
School of Mathematics and Statistics, Guangxi Normal University, Guilin, Guangxi 541004, People's Republic of China ([email protected])
*
*Corresponding author.
Rights & Permissions [Opens in a new window]

Abstract

We investigate the global Cauchy problem for a two–phase flow model consisting of the pressureless Euler equations coupled with the isentropic compressible Navier–Stokes equations through a drag forcing term. This model was first derived by Choi–Kwon [J. Differential Equations, 261(1) (2016), pp. 654–711] by taking the hydrodynamic limit of the Vlasov/compressible Navier–Stokes equations. Under the assumption that the initial perturbation is sufficiently small, Choi–Kwon [J. Differential Equations, 261(1) (2016), pp. 654–711] established the global well–posedness and large time behaviour for the three dimensional periodic domain $\mathbb {T}^3$. However, up to now, the global well–posedness and large time behaviour for the three dimensional Cauchy problem still remain unsolved. In this paper, we resolve this problem by proving the global existence and optimal decay rates of classic solutions for the three dimensional Cauchy problem when the initial data is near its equilibrium. One of key observations here is that to overcome the difficulties arising from the absence of pressure in the Euler equations, we make full use of the drag forcing term and the dissipative structure of the Navier–Stokes equations to closure the energy estimates of the variables for the pressureless Euler equations.

Keywords

Pressureless Euler equationscompressible Navier–Stokes equationsCauchy problemglobal existencelarge time behaviour

MSC classification

Primary: 35Q30: Navier-Stokes equations
Secondary: 35Q70: PDEs in connection with mechanics of particles and systems 70B05: Kinematics of a particle 35Q83: Vlasov-like equations
Type
Research Article
Information
Proceedings of the Royal Society of Edinburgh Section A: Mathematics , Volume 154 , Issue 1 , February 2024 , pp. 328 - 352
Check for updates
Copyright
Copyright © The Author(s), 2023. Published by Cambridge University Press on behalf of The Royal Society of Edinburgh

1. Introduction

In this paper, we are interested in a two–phase flow model consisting of the pressureless Euler equations coupled with the isentropic compressible Navier–Stokes equations through a drag forcing term in the whole space $\mathbb {R}^3$. The coupled hydrodynamic system takes the following form (see [Reference Choi and Kwon12]):

(1.1)\begin{equation} \left\{\begin{array}{@{}l} \partial_t\rho+\mathop{\mathrm{div}}\nolimits \mathbf(\rho u)=0,\\ \partial_t(\rho u)+\mathop{\mathrm{div}}\nolimits \mathbf(\rho u\otimes u)={-}\rho(u-v), \\ \partial_t n+\mathop{\mathrm{div}}\nolimits \mathbf(n v)=0, \\ \partial_t(n v)+\mathop{\mathrm{div}}\nolimits \mathbf(n v \otimes v)+\nabla P(n)-\mu \Delta v-(\mu+\lambda)\nabla\mathop{\mathrm{div}}\nolimits v=\rho(u-v). \end{array}\right. \end{equation}

Here $\rho =\rho (x,\,t)$ and $u=u(x,\,t)$ represent the particle density and velocity for the pressureless flow at a domain $(x,\,t)\in \mathbb {R}^3\times \mathbb {R}_{+}$, and $n=n(x,\,t)$ and $v=v(x,\,t)$ represent the fluid density and velocity for the compressible flow. $P(n)=an^{\gamma }\,(a>0,\, \gamma \geq 1)$ represents the pressure. The symbol $\otimes$ is the Kronecker tensor product. $\mu$ and $\lambda$ stand for the shear and the bulk viscosity coefficients of the fluid satisfying the following physical conditions:

\[ \mu>0, \quad \text{and}\quad \frac{2}{3}\mu+\lambda\geq 0. \]

We consider the initial value problem of (1.1) in the whole space with the initial data

(1.2)\begin{equation} (\rho, u, n, v)|_{t=0}=(\rho_0(x), u_0(x), n_0(x), v_0(x)), \quad x\in \mathbb{R}^3, \end{equation}

satisfying

\[ (\rho_0(x), u_0(x), n_0(x), v_0(x))\longrightarrow (\bar{\rho}, \overrightarrow{0}, \bar{n},\overrightarrow{0}),\quad \text{as}\,\, |x|\longrightarrow \infty, \]

where the positive constants $\bar {\rho }$ and $\bar {n}$ are the reference densities.

The coupled hydrodynamic system (1.1) is closely related to the kinetic–fluid models, which are used to describe the interactions between particles and fluid. Recently, these types of the kinetic–fluid models have received growing attention due to a very large range of applications, for example, sedimentation, sprays, aerosols, biotechnology, and atmospheric pollution, etc. [Reference Baranger, Boudin, Jabin and Mancini1–Reference Carrillo and Goudon6, Reference Choi11–Reference Choi13, Reference Ertzbischoff16–Reference Han-Kwan, Moussa and Moyano22, Reference Mellet and Vasseur27, Reference ORourke29–Reference Williams31, Reference Yu33]. More specifically, Choi–Kwon [Reference Choi and Kwon12] first addressed the formal derivation of the coupled hydrodynamic system (1.1) from the Vlasov/compressible Navier–Stokes equations, under the assumption that the particle distribution is mono–kinetic. For the sake of completeness, we recall the details in the process. To begin with, let us denote the distribution of particles at the position–velocity $(x,\,\omega )\in \mathbb {R}^3\times \mathbb {R}^3$ and at time $t\in \mathbb {R}_+$ by $f(x,\,\omega,\,t)$, and the isentropic compressible fluid density and velocity by $n(x,\,t)$ and $v(x,\,t)$, respectively. Then the motion of the particles and fluid is governed by the following kinetic–fluid equations:

(1.3)\begin{align} \left\{ \begin{array}{@{}ll} f_t+\omega\cdot\nabla_x f+\nabla_\omega\cdot((v-\omega)f)=0,\\ n_t+\nabla_x\cdot(n v)=0,\\ (n v)_t+\nabla_x\cdot(n v\otimes v)+\nabla_x P(n)-\mu\Delta_x v-(\mu+\lambda)\nabla_x\nabla_x\cdot v=\displaystyle\int_{\mathbb{R}^3} (\omega-v)f\mathrm{d}\omega, \end{array} \right. \end{align}

for $(x,\,\omega,\,t)\in \mathbb {R}^3\times \mathbb {R}^3\times \mathbb {R}_+$. Next, we define the macroscopic variables of the local mass $\rho$ and momentum $\rho u$ for the distribution function $f$ as follows:

\begin{align*} \rho(x, t)& :=\int_{\mathbb{R}^{3}} f(x, \omega, t) {\rm d}\omega \quad\hbox{and}\quad\\ (\rho u) (x, t) & :=\int_{\mathbb{R}^{3}} \omega f(x, \omega, t) {\rm d}\omega \quad \hbox{for} \quad(x, t) \in \mathbb{R}^{3} \times \mathbb{R}_{+},\end{align*}

and denote the energy–flux $\hat {q}$, the pressure tensor $\hat {\sigma }$, and the temperature $\theta$ by the fluctuation terms:

\begin{align*} & \hat{q}(x, t):=\frac{1}{2} \int_{\mathbb{R}^{3}}|\omega-u(x, t)|^{2}(\omega-u(x, t)) f(x, \omega, t) {\rm d}\omega \\ & \hat{\sigma}(x, t):=\int_{\mathbb{R}^{3}}(\omega-u(x, t)) \otimes(\omega-u(x, t)) f(x, \omega, t) {\rm d}\omega \end{align*}

and

\[ (\rho \theta)(x, t):=\frac{1}{2} \int_{\mathbb{R}^{3}}|\omega-u(x, t)|^{2} f(x, \omega, t) {\rm d}\omega \]

First, integrating the equation (1.3)1 with respect to $\omega$ over $\mathbb {R}^3$, one can easily get the continuity equation:

\[ \frac{d \rho}{d t}+\nabla_{x} \cdot(\rho u)=0. \]

Second, multiplying (1.3)1 by $\omega$, and then integrating the resultant equation with respect to $\omega$ over $\mathbb {R}^3$, we can deduce the momentum equation:

\[ \frac{d(\rho u)}{d t}+\nabla_{x} \cdot(\rho u \otimes u)+\nabla_{x} \cdot \hat{\sigma}={-}\rho(u-v). \]

Third, multiplying (1.3)1 by $\frac {|\omega |^{2}}{2}$, and then integrating the resultant equation with respect to $\omega$ over $\mathbb {R}^3$, we have from the definitions of the energy–flux $\hat {q}$, the pressure tensor $\hat {\sigma }$, and the temperature $\theta$ that

\[ \frac{d}{d t}\left(\rho\left(\frac{|u|^{2}}{2}+\theta\right)\right)+\nabla_{x} \cdot\left(\left(\rho\left(|u|^{2}+\theta\right)+\hat{\sigma}\right) u+\hat{q}\right)=2 \rho \theta-\rho u \cdot(u-v). \]

Finally, by combining all the equations of macroscopic variables with ones of the compressible fluid variables $(n,\, v)$, we deduce that

(1.4)\begin{equation} \left\{ \begin{array}{@{}ll} \partial_{t} \rho+\nabla_{x} \cdot(\rho u)=0, \\ \partial_{t}\left(\rho\left(\dfrac{|u|^{2}}{2}+\theta\right)\right)+\nabla_{x} \cdot\left(\left(\rho\left(|u|^{2}+\theta\right)+\hat{\sigma}\right) u+\hat{q}\right)=2 \rho \theta-\rho u \cdot(u-v), \\ \partial_{t} n+\nabla_{x} \cdot(n v)=0, \\ \partial_{t}(n v)+\nabla_{x} \cdot(n v \otimes v)+\nabla_{x} p(n)-\mu \Delta_x v-(\mu+\lambda)\nabla_x(\nabla_{x} \cdot v)=\displaystyle\int_{\mathbb{R}^{3}}(\omega-v) f {\rm d}\omega,\end{array} \right. \end{equation}

for $(x,\, t)\in \mathbb {R}^3\times \mathbb {R}_+$. Noticing that the energy–flux $\hat {q}$ is involved in (1.4) 2, the system (1.4) is not closed. In order to close the system (1.4), we make the assumptions that the fluctuations are negligible and the velocity distribution is mono–kinetic, i.e., $f(x,\, \omega,\, t)=\rho (x,\, t) \delta (\omega -u(x,\, t))$, where $\delta$ is the standard Dirac delta function. Then, it is clear that the system (1.4) 2 reduces to the model (1.1). It should be mentioned that the drag forcing term in the Navier–Stokes equations doesn't involve the Navier–Stokes density $n$. We remark that this phenomenon is natural. Indeed, if the density $n$ of Navier–Stokes fluid disappears, then it is obvious that there is no particle, i.e., the distribution of particles $f(x,\, \omega,\, t)=0$. Therefore, the density $\rho$ of the Euler equations is zero since $\rho =\int _{R^3}f(x,\,\omega,\, t)dw=0$. Consequently, the drag forcing term $\rho (u-v)$ in the Navier–Stokes equations disappears.

The global existence and large time behaviour of classical solutions to the pressureless Euler equations coupled with the incompressible/compressible Navier–Stokes equations in the periodic domain $\mathbb {T}^3$ were established by [Reference Choi and Kwon12, Reference Ha, Kang and Kwon20]. Recently, Choi–Jung [Reference Choi and Jung14] proved the global well–posedness and large time behaviour for the pressureless Euler equations coupled with the incompressible Navier–Stokes equations in the whole space $\mathbb {R}^3$.

However, up to now, the global well–posedness and large time behaviour for the three dimensional Cauchy problem of the pressureless Euler equations coupled with the compressible Navier–Stokes equations (1.1) still remain unsolved. Due to absence of the pressure term in the Euler equations, the main difficulty lies in the closure of the energy estimates of the particle density $\rho$. In fact, it is well–known that the pressureless Euler equations may develop the $\delta -$shock in finite-time even with smooth initial data [Reference Carrillo, Choi, Tadmor and Tan7–Reference Chen and Wang9, Reference Engelberg15, Reference Ha, Kang and Kwon20, Reference Liu and Tadmor24]. The main purpose of this paper is to develop a global well–posedness theory for the Cauchy problem of the pressureless Euler system coupled with the compressible Navier–Stokes system (1.1). We first deduce the uniform bound of $(u,\, n-\bar {n},\, v)$ by properly combining the drag forcing effect with the viscous effect in the compressible Navier–Stokes equations under a priori assumption that $\|\varrho (t)\|_{ H^{2}}+\|(u,\,n-\bar {n},\, v)(t)\|_{H^{3}}$ is sufficiently small. Then, the uniform bound of particle density $\rho$ can be obtained by making a priori decay–in–time estimates on $(u,\, n-\bar {n},\, v)$, which is based on linear decay estimates together with high–order energy estimates. Our methods mainly involve Hodge decomposition, low–frequency and high–frequency decomposition, delicate spectral analysis, and energy methods.

Before stating the main result, let us introduce several notations and conventions used throughout this paper. For $m\geq 0$ and $q\geq 1$, we use $\|\cdot \|_m$ and $\|\cdot \|_{m,q}$ to denote the norms in the Sobolev spaces $H^m(\mathbb {R}^3)$ and $W^{m,q}(\mathbb {R}^3)$ respectively. For the sake of conciseness, we do not distinguish in functional space names when they are concerned with scalar–valued or vector–valued functions; $\|(f,\,g)\|_X$ denotes $\|f\|_X+\|g\|_X$. We use $\left \langle \cdot,\, \cdot \right \rangle$ to denote the inner product in $L^2(\mathbb {R}^2)$. We employ the notation $a\lesssim b$ to mean that $a\leq Cb$ for a universal constant $C>0$ which only depends on the parameters coming from the problem. We denote $\nabla =\partial _x=(\partial _1,\, \partial _2,\,\partial _3)$, where $\partial _i=\partial _{x_i}$, $\nabla _i=\partial _i$, and put $\partial _x^lf=\nabla ^l f=\nabla (\nabla ^{l-1}f)$. For $r\in \mathbb {R}$, let $\Lambda ^r$ be the pseudo–differential operator defined by

\[ \Lambda^r f=\mathcal{F}^{{-}1}(|\xi|^r\widehat{f}(\xi)), \]

where $\widehat {f}$ are the Fourier transform of $f$. Let $\eta$ be positive constant defined in § 3. For a radial function $\phi \in C_0^{\infty }(\mathbb {R}^3)$ such that $\phi (\xi )=1$ where $|\xi |\leq \frac {\eta }{2}$ and $\phi (\xi )=0$ where $|\xi | \geq \eta$, we define the low–frequency part of $f$ by

\[ f^l=\mathfrak{F}^{{-}1}[\phi(\xi)\hat{f}] \]

and the high–frequency part of $f$ by

\[ f^h=\mathfrak{F}^{{-}1}[(1-\phi(\xi))\hat{f}]. \]

It is direct to check that $f=f^l+f^h$, if the Fourier transform of $f$ exists.

The main novelty of this paper is to establish the global existence and large time behaviour of classical solutions to the Cauchy problem (1.1)–(1.2), and our main results are stated in the following theorem.

Theorem 1.1 Assume that $\rho _0-\bar \rho \in H^{2}(\mathbb {R}^3)$ and $( u_0,\, n_0-\bar {n},\, v_0)\in H^{3}(\mathbb {R}^3)\cap L^1(\mathbb {R}^3)$. Then there exists a small constant $\delta _0>0$ such that if

(1.5)\begin{equation} \|\rho_0-\bar\rho\|_{ H^{2}}+\|(u_0,n_0-\bar{n}, v_0)\|_{H^{3}\cap L^1}\leq \delta_0, \end{equation}

the Cauchy problem (1.1)–(1.2) admits a unique solution $(\rho,\, u,\, n ,\, v)(x,\,t)$ such that for any $t\in [0,\, \infty )$,

(1.6)\begin{align} & \begin{aligned} & \|(u,n-\bar{n},v)(t)\|_{H^{3}}^2+\int_0^t(\|\nabla (n-\bar{n})(\tau)\|_{ H^{2}}^2+\|(u-v, \nabla v, \nabla u)(\tau)\|_{H^{3}}^2)\,{\rm d}\tau\\ & \quad\leq C \|(u_0,n_0-\bar{n},v_0)\|_{H^{3}}^2, \end{aligned} \end{align}
(1.7)\begin{align} & \|\rho(t)-\bar\rho\|_{ H^{2}}\leq C\left( \|\rho_0-\bar\rho\|_{ H^{2}}+\|(u_0,n_0-\bar{n},v_0)\|_{H^{3}\cap L^1}\right). \end{align}

Moreover, the solution $(\rho -\bar \rho,\,u,\,n-\bar {n},\,v)$ has the following decay estimates:

(1.8)\begin{align} & \|\nabla(u,n-\bar{n}, v)(t)\|_{H^{2}}+\|(u-v)\|_{L^{2}}\leq C (1+t)^{-{5}/{4}}, \end{align}
(1.9)\begin{align} & \|(u, n-\bar{n}, v)(t)\|_{L^2}\leq C (1+t)^{-{3}/{4}}, \end{align}
(1.10)\begin{align} & \|\partial_t (\rho-\bar\rho, u, n-\bar{n}, v)(t)\|_{L^2}\leq C (1+t)^{-{5}/{4}}. \end{align}

Remark 1.2 Compared to Wu–Zhang-Zou [Reference Wu, Zhang and Zou32] where a two–phase model consisting of the isothermal Euler equations coupled with the compressible Navier–Stokes equations through a drag forcing term was investigated, we can not obtain the decay–in–time estimate of the particle density $\rho$ due to the absence of the pressure in the Euler equations. However, all time derivatives $\partial _t (\rho -\bar \rho,\, u,\, n-\bar {n},\, v)$ in $L^2$–norm decay in time.

Remark 1.3 It is interesting to make a comparison between Theorem 1.1 and that of Choi–Jung [Reference Choi and Jung14], where the authors studied the global well–posedness and large time behaviour for the pressureless Euler equations coupled with the incompressible Navier–Stokes equations ($n\equiv 1$ in (1.1)) by combining energy estimates with the standard bootstrapping arguments. The main differences can be outlined as follows: Assume that $\rho _0\in H^{3}(\mathbb {R}^3)\cap L^1(\mathbb {R}^3)$, $u_0\in H^{5}(\mathbb {R}^3)$, $v_0\in H^{4}(\mathbb {R}^3)\cap L^1(\mathbb {R}^3)$, and $\|\rho _0\|_{ H^{3}}+\|u_0\|_{H^{5}}+\|v_0\|_{H^{4}\cap L^1}$ is sufficiently small, the authors in [Reference Choi and Jung14] showed that the pressureless Euler equations coupled with the incompressible Navier–Stokes equations has a small smooth solutions satisfying the following decay estimate:

(1.11)\begin{equation} \|u(t)\|_{H^4}+\|v(t)\|_{H^3}\lesssim (1+t)^{-\vartheta},\text{ for }0<\vartheta<\frac{3}{4}. \end{equation}

In this paper, we only need the smallness assumption on $\|\rho _0-\bar \rho \|_{ H^{2}}+\|(u_0,\,n_0-\bar {n},\, v_0)\|_{H^{3}}$, but $\|\rho _0-\bar \rho \|_{ H^{3}}+\|u_0\|_{H^5}+\|v_0\|_{H^{4}}$ may be arbitrarily large. It should be mentioned that our methods rely on $\bar \rho >0$ heavily, and particularly can not deal with the case $\bar \rho =0$ as in [Reference Choi and Jung14]. Notice that the dissipation term $-\alpha _2(u-v)$ in the fourth equation of (2.1) will disappear if $\bar \rho =0$. Therefore, it seems impossible for us to make full use of the drag forcing term and the dissipative structure of the Navier–Stokes equations to closure the energy estimates of the variables for the pressureless Euler equations. On the other hand, the decay rates in (1.8)–(1.9) imply that $L^2$ decay rates of $(u,\,v)$ and its all–order spatial derivatives are $(1+t)^{-{3}/{4}}$ and $(1+t)^{-{5}/{4}}$ respectively, which are faster that the $L^2$ decay rate $(1+t)^{-\vartheta }$ with $0<\vartheta <\frac {3}{4}$ in (1.11). In addition, the decay rate in (1.8) shows that the optimal $L^2$ decay rate of the difference $u-v$ between the velocities $u$ and $v$ is $(1+t)^{-{5}/{4}}$, which is particularly faster than ones of two velocities themselves, and is totally new as compared to [Reference Choi and Jung14].

The rest of the paper is organized as follows. In § 2, we reformulate the Cauchy problem (1.1)–(1.2). Then, we derive the linear decay estimates by employing Hodge decomposition technique and making careful spectral analysis. In § 3, by properly combining the drag forcing effect with the smooth effect of the viscosity in the compressible Navier–Stokes equations, we deduce the nonlinear energy estimates to get a key Lyapunov–type energy inequality. Then, this crucial Lyapunov–type energy inequality together with linear decay estimates obtained in § 2 gives the proof of Theorem 1.1.

2. Reformulated system

Setting

\[ \varrho=\ln\rho-\ln\bar\rho, \sigma=n-\bar{n},\quad \alpha_1=\frac{P'(\bar{n})}{\bar{n}},\quad \alpha_2=\frac{\bar{\rho}}{\bar{n}}, \quad \bar\mu=\frac{\mu}{\bar{n}},\quad \text{and}\quad \bar{\lambda}=\frac{\lambda}{\bar{n}}, \]

then the Cauchy problem (1.1)–(1.2) can be reformulated as

(2.1)\begin{equation} \begin{cases} \partial_t\varrho={-}\text{div}u-u\nabla\varrho,\\ \partial_t u+(u-v)=F_1,\\ \partial_t \sigma+\bar{n}\mathop{\mathrm{div}}\nolimits v=F_2,\\ \partial_t v+\alpha_1\nabla \sigma-\bar{\mu}\Delta v-(\bar{\mu}+\bar{\lambda})\nabla\mathop{\mathrm{div}}\nolimits v- \alpha_2(u-v)=F_3,\\ (\varrho,u, \sigma, v)|_{t=0}:=(\varrho(x),u_0(x), \sigma_0(x), v_0(x))\rightarrow(0,\overrightarrow{0}, 0, \overrightarrow{0}),\text{ as } |x|\rightarrow \infty, \end{cases} \end{equation}

where

\begin{align*} F_1& ={-}u \cdot \nabla u,\\ F_2& ={-}v\cdot\nabla \sigma-\sigma\mathop{\mathrm{div}}\nolimits v,\\ F_3& ={-}v\cdot \nabla v+\left(\alpha_1-\dfrac{P'(n)}{n}\right)\nabla \sigma+\left(\dfrac{\mu}{n}-\bar{\mu}\right)\Delta v+\left(\dfrac{\mu+\lambda}{n}-(\bar{\mu}+\bar{\lambda})\right)\nabla\mathop{\mathrm{div}}\nolimits v\\& \quad +\left(\dfrac{\rho}{n}-\alpha_2\right)(u-v). \end{align*}

The local existence and uniqueness of the classical solution for the Cauchy problem (2.1) can be established by the methods of Kato [Reference Kato23] or Majda [Reference Majda25].

Proposition 2.1 Local existence

Assume that the initial data $(\varrho _0,\, u_0,\, \sigma _0,\, v_0)\in H^{2}(\mathbb {R}^3)\times H^{3}(\mathbb {R}^3)\times H^{3}(\mathbb {R}^3)\times H^{3}(\mathbb {R}^3),$ and satisfies

(2.2)\begin{equation} \min_{x\in \mathbb{R}^3} \text{e}^{\varrho_0(x)}>0,\quad \min_{x\in \mathbb{R}^3}\{\sigma_0(x)+\bar{n}\}>0.\end{equation}

Then there exists a positive constant $T_0$ depending only on $\|\varrho _0\|_{ H^{2}}+\|u_0\|_{H^{3}}+\|\sigma _0\|_{H^{3}}+\|v_0\|_{H^{3}}$ such that the Cauchy problem (2.1) has a unique solution $(\varrho,\, u,\, \sigma,\, v)$ satisfying

\begin{align*} & \varrho\in C^0(0, T_0; H^{2}(\mathbb{R}^3))\cap C^1(0, T_0; H^{1}(\mathbb{R}^3)),\\ & u\in C^0(0, T_0; H^{3}(\mathbb{R}^3))\cap C^1(0, T_0; H^{2}(\mathbb{R}^3)),\\ & \sigma\in C^0(0, T_0; H^{3}(\mathbb{R}^3))\cap C^1(0, T_0; H^{2}(\mathbb{R}^3))\ \text{and}\\ & v\in C^0(0, T_0; H^{3}(\mathbb{R}^3))\cap C^1(0, T_0; H^{1}(\mathbb{R}^3)). \end{align*}

Moreover, the following estimates hold,

(2.3)\begin{align} & \|\varrho(t)\|_{ H^{2}}^2+\|(u,\sigma,v)(t)\|_{H^{3}}^2+\int_0^{T_0}(\|\nabla (u,\sigma)(\tau)\|_{ H^{2}}^2+\|(u-v, \nabla v)(\tau)\|_{H^{3}}^2)\,{\rm d}\tau\nonumber\\ & \quad\leq C (\|\rho_0\|_{ H^{2}}^2+\|(u_0,\sigma_0,v_0)\|_{H^{3}}^2) , \end{align}

and

(2.4)\begin{equation} \min_{x\in \mathbb{R}^3,0\leq t \leq T_0} \text{e}^{\varrho(x)}>0, \min_{x\in \mathbb{R}^3, 0\leq t \leq T_0}\{\sigma(x)+\bar{n}\}>0.\end{equation}

To prove global existence of smooth solutions, it suffices to establish the following a priori estimates.

Proposition 2.2 A priori estimate

Let $\varrho _0\in H^{2}(\mathbb {R}^3)$, $( u_0,\, \sigma _0,\, v_0)\in H^{3}(\mathbb {R}^3)\cap L^1(\mathbb {R}^3)$. Assume the Cauchy problem (2.1) admits a solution $(\varrho,\, u,\, \sigma,\, v)(x,\,t)$ on $\mathbb {R}^3\times [0,\, T]$ for some $T>0$ in the same function class as in Proposition 2.1. Then there exist a small constant $\epsilon >0$ and a constant $C,$ which are independent of $T,$ such that if

(2.5)\begin{equation} \sup_{0\leq t\leq T}\{\|\varrho(t)\|_{ H^{2}}+\|(u,\sigma, v)(t)\|_{H^{3}}\}\leq \epsilon, \end{equation}

then for any $t\in [0,\, T]$, it holds that

(2.6)\begin{align} & \begin{aligned} & \|(u,\sigma, v)(t)\|_{H^{3}}^2+\int_0^t\|\nabla (u, \sigma)(\tau)\|_{ H^{2}}^2+\|(u-v,\nabla v)(\tau)\|_{H^{3}}^2\,{\rm d}\tau\\ & \quad\leq C\|(u_0,\sigma_0, v_0)\|_{H^{3}}^2, \end{aligned} \end{align}
(2.7)\begin{align} & \|\varrho(t)\|_{ H^{2}}\leq C\left( \|\varrho_0\|_{ H^{2}}+\|(u_0,\sigma_0,v_0)\|_{H^{3}\cap L^1}\right). \end{align}

Moreover, $(\varrho,\,u,\,\sigma,\,v)$ has the following decay proposities

(2.8)\begin{align} & \|\nabla(u, \sigma, v)\|_{H^{2}}+\|(u-v)\|_{L^2}\leq C (1+t)^{-{5}/{4}}, \end{align}
(2.9)\begin{align} & \|(u, \sigma, v)\|_{L^2}\leq C(1+t)^{-{3}/{4}}, \end{align}
(2.10)\begin{align} & \|\partial_t (\varrho, u, \sigma, v)\|_{L^2}\leq C (1+t)^{-{5}/{4}}. \end{align}

Theorem 1.1 follows from proposition 2.1 and proposition 2.2 by standard continuity argument.

3. Spectral analysis and linear ${\rm L}^2$ estimates

Define $U=(u,\, \sigma,\, v)^t$, by semigroup theory for evolutionary equation, we focus on the following linearized dissipative system for Eq. (2.1) $_2$ to Eq. (2.1) $_4$:

(3.1)\begin{equation} \left\{\begin{array}{@{}l} U_t=\mathcal {B} U,\\ U|_{t=0}=U_0, \end{array} \right. \end{equation}

where the operator $\mathcal {B}$ has the form as

\[ \mathcal {B}=\left( \begin{array}{@{}ccc@{}} -I_{3\times 3} & 0 & I_{3\times 3}\\ 0 & 0 & -\bar{n}\mathop{\mathrm{div}}\\ \alpha_2 I_{3\times 3} & -\alpha_1\nabla & (\bar{\mu}\Delta-\alpha_2) I_{3\times 3}+(\bar{\mu}+\bar{\lambda})\nabla\otimes\nabla \end{array}\right). \]

Applying the Fourier transform to the system (3.1), we have

(3.2)\begin{equation} \left\{\begin{array}{@{}l} \widehat{U}_t=\mathcal {A}(\xi) \widehat{U},\\ \widehat{U}|_{t=0}=\widehat{U}_0, \end{array} \right. \end{equation}

where $\widehat {U}(\xi,\,t)=\mathfrak {F}(U(x,\,t))$, $\xi =(\xi ^1,\, \xi ^2,\, \xi ^3)^t$, and $\mathcal {A}(\xi )$ can be written as

\[ \mathcal {A}=\left( \begin{array}{@{}ccc@{}} -I_{3\times 3} & 0 & I_{3\times 3}\\ 0 & 0 & -i\bar{n}\xi^t\\ \alpha_2 I_{3\times 3} & -\alpha_1i\xi & -(\bar{\mu}|\xi|^2+\alpha_2) I_{3\times 3}-(\bar{\mu}+\bar{\lambda})\xi\otimes\xi \end{array}\right). \]

In order to obtain the linear time-decay estimates for the Cauchy problem (3.1), we need to analysis the properties of the semigroup, as in [Reference Matsumura and Nishida26]. Unfortunately, it seems untractable, since the system (3.1) has seven equations. To overcome this difficulty, we employ the Hodge decomposition of the linear system as in [Reference Wu, Zhang and Zou32], and then the system (3.1) can be decoupled into two systems, which enables us to obtain the optimal linear time–decay estimates.

Set

(3.3)\begin{equation} \left\{\begin{array}{@{}l} \varphi=\Lambda^{{-}1}\mathop{\mathrm{div}} u,\\ \psi=\Lambda^{{-}1}\mathop{\mathrm{div}} v,\\ \Phi=\Lambda^{{-}1}\mathop{\mathrm{curl}} u,\\ \Psi=\Lambda^{{-}1}\mathop{\mathrm{curl}} v. \end{array} \right. \end{equation}

Then, we can rewrite the system (3.1) as follows:

(3.4)\begin{equation} \left\{\begin{array}{@{}l} \partial_t \varphi+\varphi-\psi=0,\\ \partial_t \sigma+\bar{n}\Lambda\psi=0,\\ \partial_t \psi-\alpha_1\Lambda\sigma+(2\bar{\mu}+\bar{\lambda})\Lambda^2\psi-\alpha_2(\varphi-\psi)=0,\\ (\varphi, \sigma, \psi)|_{t=0}=(\Lambda^{{-}1}\mathop{\mathrm{div}} u_0(x), \sigma_0(x), \Lambda^{{-}1}\mathop{\mathrm{div}} v_0(x)), \end{array} \right. \end{equation}

and

(3.5)\begin{equation} \left\{\begin{array}{@{}l} \partial_t\Phi+\Phi-\Psi=0,\\ \partial_t \Psi+\bar{\mu}\Lambda^2\Psi-\alpha_2(\Phi-\Psi)=0,\\ (\Phi, \Psi)|_{t=0}=(\Lambda^{{-}1}\mathop{\mathrm{curl}} u_0(x), \Lambda^{{-}1}\mathop{\mathrm{curl}} v_0(x)). \end{array} \right. \end{equation}

3.1 Spectral analysis for IVP (3.4)

By virtue of the semigroup theory for evolutionary equations, we may express the IVP (3.4) for $\mathcal {U}=(\varphi,\, \sigma,\, \psi )^t$ as

(3.6)\begin{equation} \left\{\begin{array}{@{}l} \mathcal {U}_t=\mathcal {B}_1 \mathcal {U},\\ \mathcal {U}|_{t=0}=\mathcal {U}_0, \end{array} \right. \end{equation}

where the operator $\mathcal {B}_1$ is given by

\[ \mathcal {B}_1=\left( \begin{array}{@{}ccc@{}} -1 & 0 & 1\\ 0 & 0 & -\bar{n}\Lambda\\ \alpha_2 & \alpha_1\Lambda & -(2\bar{\mu}+\bar{\lambda})\Lambda^2-\alpha_2 \end{array}\right). \]

Taking the Fourier transform to the system (3.6), we have

(3.7)\begin{equation} \left\{\begin{array}{@{}l} \widehat{\mathcal {U}}_t=\mathcal {A}_1 \widehat{\mathcal {U}},\\ \widehat{\mathcal {U}}|_{t=0}=\widehat{\mathcal {U}}_0, \end{array} \right. \end{equation}

where $\mathcal {A}_1(\xi )$ is defined by

\[ \mathcal {A}_1(\xi)=\left( \begin{array}{@{}ccc@{}} -1 & 0 & 1\\ 0 & 0 & -\bar{n}|\xi|\\ \alpha_2 & \alpha_1|\xi| & -(2\bar{\mu}+\bar{\lambda})|\xi|^2-\alpha_2 \end{array}\right). \]

To get the eigenvalues of the matrix $\mathcal {A}_1(\xi )$, we need to compute the determinant

(3.8)\begin{align} & \mathop{\mathrm{det}}\nolimits (r I-\mathcal{A}_1(\xi))\nonumber\\ & \quad =\left| \begin{array}{@{}ccc@{}} r+1 & 0 & -1\nonumber\\ 0 & r & \bar{n}|\xi|\\ - \alpha_2 & -\alpha_1|\xi| & r+(2\bar{\mu}+\bar{\lambda})|\xi|^2+\alpha_2 \end{array}\right|\nonumber\\ & \quad =(r+1)[r(r+(2\bar{\mu}+\bar{\lambda})|\xi|^2+\alpha_2)+\bar{n}\alpha_1|\xi|^2]-\alpha_2r\nonumber\\ & \quad=r^3+[(2\bar{\mu}+\bar{\lambda})|\xi|^2+1+\alpha_2]r^2+[(2\bar{\mu}+\bar{\lambda})+\bar{n}\alpha_1]|\xi|^2r+\bar{n}\alpha_1|\xi|^2\nonumber\\ & \quad=0, \end{align}

which implies that the matrix $\mathcal {A}(\xi )$ has three different eigenvalues which can be expressed as

\[ r_1=r_1(|\xi|), \quad r_2=r_2(|\xi|),\quad r_3=r_3(|\xi|). \]

By careful computation, we get the following Lemma.

Lemma 3.1 There exists a positive constant $\eta _1\ll 1$ such that, for $|\xi |\leq \eta _1,$ the spectral has the following Taylor series expansion:

(3.9)\begin{equation} \left\{\begin{array}{@{}l} r_1={-}1-\alpha_2+\dfrac{-\alpha_2(\alpha_2+1)(2\bar{\mu}+\bar{\lambda})+\alpha_1\alpha_2\bar{n}}{(1+\alpha_2)^2}|\xi|^2+\mathcal {O}(|\xi|^3),\\ r_2={-}\dfrac{(2\bar{\mu}+\bar{\lambda})(1+\alpha_2)+\alpha_1\alpha_2\bar{n}}{2(1+\alpha_2)^2}|\xi|^2+\mathcal {O}(|\xi|^3)+\left[\sqrt{\dfrac{\bar{n}\alpha_1}{1+\alpha_2}}|\xi|+\mathcal {O}(|\xi|^2)\right]i,\\ r_3={-}\dfrac{(2\bar{\mu}+\bar{\lambda})(1+\alpha_2)+\alpha_1\alpha_2\bar{n}}{2(1+\alpha_2)^2}|\xi|^2+\mathcal {O}(|\xi|^3)-\left[\sqrt{\dfrac{\bar{n}\alpha_1}{1+\alpha_2}}|\xi|+\mathcal {O}(|\xi|^2)\right]i.\\ \end{array} \right. \end{equation}

Lemma 3.2 Let

\[ \nu_1=\frac{(2\bar{\mu}+\bar{\lambda})(1+\alpha_2)+\alpha_1\alpha_2\bar{n}}{2(1+\alpha_2)^2}, \]

for any $|\xi |\leq \eta _1$, we have

(3.10)\begin{equation} |\hat{\varphi}|,|\hat{\sigma}|,|\hat{\psi}|\lesssim e^{-\nu_1|\xi|^2t}(|\hat{\varphi}_0|+|\hat{\sigma}_0|+|\hat{\psi}_0|). \end{equation}

Proof. The semigroup $e^{t\mathcal {A}}$ is expressed as

\[ e^{t\mathcal{A}_1(\xi)}=\sum_{i=1}^3 e^{r_it}P_i(\xi), \]

where the project operators $P_i(\xi )$ can be computed as

\[ P_i(\xi)=\prod_{j\neq i}\frac{\mathcal{A}_1(\xi)-r_jI}{r_i-r_j},\quad i, j=1, 2, 3. \]

thus, we have

(3.11)\begin{align} P_1(|\xi|)& =\frac{1}{1+\alpha_2}\left( \begin{array}{@{}ccc@{}} 1 & 0 & -1\\ 0 & 0 & 0\\ - \alpha_2 & 0 & \alpha_2 \end{array}\right)+\mathcal {O}(|\xi|), \end{align}
(3.12)\begin{align} P_2(|\xi|)& =\frac{1}{2(1+\alpha_2)}\left( \begin{array}{@{}ccc@{}} \alpha_2 & -i\alpha_1\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}} & 1\\ i\alpha_2\bar{n}\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}} & 1+\alpha_2 & i\bar{n}\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}}\\ \alpha_2 & -i\alpha_1\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}} & 1 \end{array}\right)+\mathcal {O}(|\xi|), \end{align}
(3.13)\begin{align} P_3(|\xi|)& =\frac{1}{2(1+\alpha_2)}\left( \begin{array}{@{}ccc@{}} \alpha_2 & i\alpha_1\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}} & 1\\ - i\alpha_2\bar{n}\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}} & 1+\alpha_2 & -i\bar{n}\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}}\\ \alpha_2 & i\alpha_1\sqrt{\dfrac{1+\alpha_2}{\bar{n}\alpha_1}} & 1 \end{array}\right)+\mathcal {O}(|\xi|), \end{align}

for any $|\xi |\leq \eta _1$. The solution of IVP (3.4) can be expressed as

(3.14)\begin{equation} \widehat{\mathcal {U}}(\xi,t)=e^{\mathcal {A}_1(\xi)t}\widehat{\mathcal {U}}_0(\xi)=\left(\sum_{i=1}^3 e^{r_it}P_i(\xi)\right)\widehat{\mathcal {U}}_0(\xi). \end{equation}

Therefore, by combining lemma 3.1 with (3.11)–(3.14), one has (3.10) immediately.

With the key estimate (3.10) in hand, we are able to establish the $L^2$–convergence rate on the low–frequency part of the solution, which is stated in the following proposition.

Proposition 3.3 $L^2$–theory

For any $k>-\frac 3 2,$ there exists a positive constant $C$ which is independent of $t$ such that

\[ \|\nabla^k \mathcal {U}^l\|_{L^2}\leq C(1+t)^{-\frac{k}{2}-\frac{3}{ 4}}\|\widehat{\mathcal {U}^l}(0)\|_{L^{\infty}}. \]

Proof. Using the Plancherel theorem, together with (3.10), we have

(3.15)\begin{equation} \begin{aligned} \|\nabla^k \mathcal {U}^l\|_{L^2}^2 & =\|\widehat{\nabla^k \mathcal {U}^l}\|_{L^2}^2=\||\xi|^k\widehat{\mathcal {U}^l}\|_{L^2}^2\\ & =\||\xi|^k e^{\mathcal {A}_1(\xi)t}\widehat{\mathcal {U}^l}(0)\|_{L^2}^2\\ & \leq C(1+t)^{{-}k-\frac{3}{2}}\|\widehat{\mathcal {U}^l}(0)\|_{L^{\infty}}^2. \end{aligned} \end{equation}

3.2 Spectral analysis for IVP (3.5)

Set $\mathcal {V}=(\Phi,\,\Psi )^t$, the IVP (3.5) can be expressed as

(3.16)\begin{equation} \left\{\begin{array}{@{}l} \mathcal {V}_t=\mathcal {B}_2 \mathcal {V},\\ \mathcal {V}|_{t=0}=\mathcal {V}_0, \end{array} \right. \end{equation}

where

\[ \mathcal {B}_2=\left( \begin{array}{@{}cc@{}} -1 & 1\\ \alpha_2 & \alpha_2-\bar{\mu}\Lambda^2 \end{array}\right). \]

Similar to the derivation of Lemma (3.1), the spectral of (3.16) has the following Taylor series expansion:

\[ \left\{\begin{array}{@{}l} s_1={-}\alpha_2-1-\dfrac{\alpha_2\bar{\mu}}{\alpha_2+1}|\xi|^2+\mathcal {O}(|\xi|^4),\\ s_2={-}\dfrac{\bar{\mu}}{\alpha_2+1}|\xi|^2+\mathcal {O}(|\xi|^4),\\ \end{array} \right. \]

for $|\xi |\leq \eta _2$, where $\eta _2\ll 1$ is a given positive constant.

From the results of Wu–Zhang–Zou [Reference Wu, Zhang and Zou32], the $L^2$–converge rate on the low–frequency part of the solution of $\mathcal {V}$ can be given by following proposition.

Proposition 3.4 For any $k>-\frac 3 2,$ there exists a positive constant C which is independent of $t$ such that

\[ \|\nabla^k \mathcal {V}^l\|_{L^2}\leq C(1+t)^{-\frac{k}{2}-\frac{3}{ 4}}\|\widehat{\mathcal {V}}^l(0)\|_{L^{\infty}}. \]

Combining the definitions of $\phi,\,\psi,\, \Phi$ and $\Psi$, with the relations

\[ u={-}\Lambda^{{-}1}\nabla \varphi-\Lambda^{{-}1}\mathop{\mathrm{div}}\nolimits \Phi, \text{ and } v={-}\Lambda^{{-}1}\nabla \psi-\Lambda^{{-}1} \mathop{\mathrm{div}}\nolimits \Psi, \]

the estimates in space $H^k(\mathbb {R}^3)$ for $(u,\,v)$ are the same as $(\phi,\,\psi,\, \Phi,\, \Psi )$.

Proposition 3.5 For any $k>-\frac 3 2,$ $2\leq r< \infty,$ and any $t\geq 0,$ assume the initial data $U_0\in L^1(\mathbb {R}^3),$ then the global solution $U=(u,\, \sigma,\, v)^t$ of the IVP (3.1) satisfies

\[ \|\nabla^k {U}^l\|_{L^2}\leq C(1+t)^{-\frac{k}{2}-\frac{3}{ 4}}\|\widehat{ U}^l(0)\|_{L^{\infty}}\leq C(1+t)^{-\frac{k}{2}-\frac{3}{ 4}}\| U(0)\|_{L^1}. \]

In the following two lemmas, we recall Sobolev's inequality and the Galiardo–Nirenberg inequality.

Lemma 3.6 Let $f\in H^2(\mathbb {R}^3)$. Then it holds that

\begin{align*} & (i)\quad\|f\|_{L^{\infty}}\leq C \|\nabla f\|_{L^2}^{{1}/{2}}\|\nabla f\|_{H^1}^{{1}/{2}}\leq C \|\nabla f\|_{H^1};\\ & (ii)\quad \|f\|_{L^6} \leq C \|\nabla f\|_{L^2};\\ & (iii)\quad \|f\|_{L^p}\leq C\|f\|_{H^1}, 2\leq p\leq 6. \end{align*}

Lemma 3.7 For $0\leq i,\, j\leq k,$ if

\[ a\in[\frac{i}{k},1] \text{ and } \frac{i}{3}-\frac{1}{p}=\left(\frac{j}{3}-\frac{1}{q}\right)(1-a)+\left(\frac{k}{3}-\frac{1}{r}\right)a \]

hold, then we have

\[ \|\nabla^i f\|_{L^p}\leq C \|\nabla^i f\|_{L^q}^{1-a}\|\nabla^k f\|_{L^r}^a. \]

Especially, when $p=q=r=2$, it holds that

\[ \|\nabla^i f\|_{L^2}\leq C \|\nabla^i f\|_{L^2}^{\frac{k-j}{k-i}}\|\nabla^k f\|_{L^2}^{\frac{i-j}{k-j}}. \]

Proof. This is a special case of [Reference Nirenberg28].

We also record the following lemma, which is used to deal with the $L^2-$norm of the spatial derivatives of the product of two functions.

Lemma 3.8 If $f,\, g \in H^k(\mathbb {R}^3)\subset L^{\infty }(\mathbb {R}^3)$ for any integer $k\geq 1,$ then we have

\[ \|\nabla^k(fg)\|_{L^2}\leq C(\|f\|_{L^{\infty}}\|\nabla^k g\|_{L^2}+\|g\|_{L^{\infty}}\|\nabla^k f\|_{L^2}) \]

and

\[ \|\nabla^k(fg)\|_{L^1}\leq C(\|f\|_{L^2}\|\nabla^k g\|_{L^2}+\|g\|_{L^2}\|\nabla^k f\|_{L^2}). \]

4. A priori estimates

We suppose that the inequality (2.5) in proposition 2.2 holds throughout this section and the next section. We will deduce a series of lemmas about the energy estimates in what follows. The first lemma is concerned with the lower order energy estimate of $(u,\, \sigma,\, v)$.

Lemma 4.1 There exists a suitably large constant $D_1>0$ which is independent of $\epsilon$ such that

(4.1)\begin{align} & \frac{{\rm d}}{{\rm d}t}\left\{D_1\|(u, \sigma, v)(t)\|_{L^2}^2+\left\langle \nabla \sigma, v\right\rangle(t) \right\}+C\big(\|\nabla (\sigma, v)\|_{L^2}^2+\|u-v\|_{L^2}^2\big)\nonumber\\& \quad \lesssim \epsilon(\|\nabla u\|_{L^2}^2+\|\nabla^2 v\|_{L^2}^2), \end{align}

for any $0\leq t\leq T$.

Proof. Multiplying (2.1) 2 – (2.1) 4 by $u,\, \sigma,\, v$ respectively, and then integrating the resultant equations over $\mathbb {R}^3$, we have

(4.2)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t}\|u(t)\|_{L^2}^2+\|u-v\|_{L^2}^2+\left\langle u-v, v \right\rangle =\left\langle F_1, u\right\rangle, \end{align}
(4.3)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t}\|\sigma(t)\|_{L^2}^2+\bar{n}\left\langle \mathop{\mathrm{div}}\nolimits v,\sigma \right\rangle =\left\langle F_2, \sigma\right\rangle, \end{align}

and

(4.4)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t}\|v(t)\|_{L^2}^2-\alpha_1\left\langle \mathop{\mathrm{div}}\nolimits v,\sigma \right\rangle+\bar{\mu}\|\nabla v\|_{L^2}^2\nonumber\\& \quad +(\bar{\mu} +\bar{\lambda})\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}^2-\alpha_2\left\langle u-v, v \right\rangle=\left\langle F_3, v\right\rangle.\end{align}

Multiplying (4.2) by $\alpha _2\bar {n}$, (4.3) by $\alpha _1$, (4.4) by $\bar {n}$, and adding them together, it follows that

(4.5)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t}\left(\alpha_2\bar{n}\|u(t)\|_{L^2}^2+\alpha_1\|\sigma(t)\|_{L^2}^2+\bar{n}\|v(t)\|_{L^2}^2\right) +\alpha_2\bar{n}\|u-v\|_{L^2}^2+\bar{\mu}\bar{n}\|\nabla v\|_{L^2}^2\notag\\ & \qquad+(\bar{\mu}+\bar{\lambda})\bar{n}\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}^2\nonumber\\ & \quad\lesssim ~\big|\left\langle F_1, u\right\rangle \big|+\big|\left\langle F_2, \sigma\right\rangle \big|+\big|\left\langle F_3, v\right\rangle\big|. \end{align}

The three terms on the right hand side of the above inequality can be estimated as follows.

Firstly, for the first term, by virtue of (2.5), lemma 3.6 and H${\rm \ddot o}$lder inequality, we obtain

(4.6)\begin{equation} |\left\langle F_1, u\right\rangle| =|\left\langle -u\cdot\nabla u, u\right\rangle |\lesssim \|u\|_{L^3}\|u\|_{L^6}\|\nabla u\|_{L^2}\lesssim \|u\|_{H^1}\|\nabla u\|_{L^2}^2\lesssim \epsilon\|\nabla u\|_{L^2}^2. \end{equation}

For the second term, by using integration by parts and performing the similar way to the proof of (4.6), one has

(4.7)\begin{equation} \begin{aligned} | \left\langle F_2, \sigma\right\rangle | & =|\left\langle -v\cdot\nabla \sigma, \sigma\right\rangle +\left\langle -\sigma \mathop{\mathrm{div}}\nolimits v, \sigma\right\rangle|\\ & \lesssim|\left\langle \sigma \mathop{\mathrm{div}}\nolimits v, \sigma\right\rangle| \\ & \lesssim\|\sigma\|_{L^3}\|\sigma\|_{L^6}\|\nabla v\|_{L^2}\\ & \lesssim \epsilon( \|\nabla \sigma\|_{L^2}^2+\|\nabla v\|_{L^2}^2). \end{aligned} \end{equation}

Using the fact that

(4.8)\begin{equation} \alpha_1-\frac{P'(n)}{n}\sim \sigma,\quad \frac{\mu}{n}-\bar{\mu}\sim \sigma,\quad \text{and}\quad \frac{\mu+\lambda}{n}-(\bar{\mu}+\bar{\lambda})\sim \sigma, \end{equation}

the third term can be estimated as

(4.9)\begin{align} |\left\langle F_3, v\right\rangle | & \lesssim|\left\langle -v\cdot\nabla v, v\right\rangle| +\left|\left\langle \left(\alpha_1-\frac{P'(n)}{n}\right)\nabla \sigma, v\right\rangle\right|+\left|\left\langle \left(\frac{\mu}{n}-\bar{\mu}\right)\Delta v ,v\right\rangle\right|\nonumber\\ & \quad+\left|\left\langle \left(\frac{\mu+\lambda}{n}-(\bar{\mu}+\bar{\lambda})\right)\nabla\mathop{\mathrm{div}}\nolimits v,v\right\rangle\right| +\left|\left\langle \left(\frac{\rho}{n}-\alpha_2\right)(u-v),v\right\rangle\right| \nonumber\\ & \lesssim \|v\|_{L^3}\|v\|_{L^6}\|\nabla v\|_{L^2}+\|\sigma\|_{L^3}\|\nabla \sigma\|_{L^2}\|v\|_{L^6}\nonumber\\ & \quad +\|(\sigma, v)\|_{H^1}\|\nabla v\|_{L^2}\|\nabla(\sigma, v)\|_{L^2}\nonumber\\ & \quad+\|(\rho, \sigma)\|_{H^1}\|u-v\|_{L^2}\|\nabla v\|_{L^2}\nonumber\\ & \lesssim \epsilon( \|\nabla v\|_{L^2}^2+\|\nabla \sigma\|_{L^2}^2+\|u-v\|_{L^2}^2). \end{align}

Substituting (4.6), (4.7), and (4.9) into (4.5) yields

(4.10)\begin{align} \frac{{\rm d}}{{\rm d}t}\|(u, \sigma, v)(t)\|_{L^2}^2+C\left(\|u-v\|_{L^2}^2+\|\nabla v\|_{L^2}^2+\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}^2\right)\lesssim \epsilon(\|\nabla \sigma\|_{L^2}^2+\|\nabla u\|_{L^2}^2). \end{align}

Next, we shall derive the energy dissipation for $\|\nabla \sigma \|_{L^2}^2$. Multiplying (2.1) 4 by $\nabla \sigma$, integrating them over $\mathbb {R}^3$, we obtain

(4.11)\begin{align} \alpha_1\|\nabla \sigma\|_{L^2}^2& =\left\langle -v_t, \nabla \sigma\right\rangle +\left\langle \bar{\mu}\Delta v+(\bar{\mu}+\bar{\lambda})\nabla\mathop{\mathrm{div}}\nolimits v, \nabla \sigma\right\rangle\nonumber\\ & \quad +\alpha_2\left\langle u-v, \nabla \sigma\right\rangle +\left\langle F_3, \nabla \sigma\right\rangle. \end{align}

From (2.1) 3, the first term on the right hand side can be written as

(4.12)\begin{equation} \begin{aligned} \left\langle -v_t, \nabla \sigma\right\rangle & ={-}\frac{{\rm d}}{{\rm d}t}\left\langle v, \nabla \sigma\right\rangle +\left\langle \nabla\sigma_t, u\right\rangle \\ & ={-}\frac{{\rm d}}{{\rm d}t}\left\langle v, \nabla \sigma\right\rangle +\bar{n}\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}^2+\left\langle -F_2, \mathop{\mathrm{div}}\nolimits v\right\rangle. \end{aligned} \end{equation}

By the definition of $F_2$, we obtain

(4.13)\begin{equation} \begin{aligned} |\left\langle -F_2, \mathop{\mathrm{div}}\nolimits v\right\rangle | & \lesssim |\left\langle v\cdot \nabla \sigma, \mathop{\mathrm{div}}\nolimits v\right\rangle |+|\left\langle \sigma \mathop{\mathrm{div}}\nolimits v, \mathop{\mathrm{div}}\nolimits v\right\rangle |\\ & \lesssim \|\nabla \sigma\|_{L^3}\|v\|_{L^6}\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}+\|\sigma \|_{L^{\infty}}\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}^2\\ & \lesssim \|\nabla \sigma\|_{H^1}\|\nabla v\|_{L^2}^2+\|\nabla \sigma\|_{H^1}\|\mathop{\mathrm{div}}\nolimits v\|_{L^2}^2\lesssim K_0 \|\nabla v\|_{L^2}^2. \end{aligned} \end{equation}

Taking the same argument to the term $\left \langle F_3,\, \nabla \sigma \right \rangle$, it is easy to get

(4.14)\begin{equation} \begin{aligned} |\left\langle F_3, \nabla \sigma\right\rangle | & \leq \left|\left\langle -v\cdot\nabla v, \nabla \sigma\right\rangle \right|+\left|\left\langle \left(\alpha_1-\frac{P'(n)}{n}\right)\nabla \sigma, \nabla \sigma\right\rangle\right|\\ & \quad+\left|\left\langle \left(\frac{\mu}{n}-\bar{\mu}\right)K_0 v ,\nabla \sigma\right\rangle\right|\\ & \quad+\left|\left\langle \left(\frac{\mu+\lambda}{n}-(\bar{\mu}+\bar{\lambda})\right)\nabla\mathop{\mathrm{div}}\nolimits v,\nabla \sigma\right\rangle\right| +\left|\left\langle \left(\frac{\rho}{n}-\alpha_2\right)(u-v),\nabla \sigma\right\rangle\right| \\ & \lesssim \|\nabla v\|_{L^3}\|v\|_{L^6}\|\nabla \sigma\|_{L^2}+\|\sigma\|_{L^{\infty}}\|\nabla \sigma\|^2+\|\sigma\|_{L^{\infty}}\|\nabla^2 v\|_{L^2}\|\nabla \sigma\|_{L^2}\\ & \quad+\|(\rho, \sigma)\|_{H^1}\|u-v\|_{L^2}\|\nabla \sigma\|_{L^2}\\ & \lesssim \|\nabla v\|_{H^1}^2+\|\nabla \sigma\|_{L^2}^2+\|u-v\|_{L^2}^2. \end{aligned} \end{equation}

Substituting (4.12)–(4.14) into (4.11) gives

(4.15)\begin{equation} \frac{{\rm d}}{{\rm d}t}\left\langle v, \nabla \sigma\right\rangle +C\|\nabla\sigma\|_{L^2}^2 \lesssim \|\nabla v\|_{H^1}^2+\|u-v\|_{L^2}^2. \end{equation}

Multiplying (4.10) by $D_1$ suitably large and adding it to (4.15), one has (4.1) since $\epsilon >0$ is sufficiently small. This completes the proof of lemma 4.1

For the higher order energy estimate for $(u,\,\sigma,\,v)$, we have following lemma.

Lemma 4.2 For any $0\leq t\leq T,$ there exists a suitably large constant $D_2>0$ which is independent of $\epsilon$ such that

(4.16)\begin{equation} \begin{aligned} & \frac{{\rm d}}{{\rm d}t}\left\{D_2H_1(u(t), \sigma(t), v(t))+\sum_{1\leq |k| \leq 2}\left\langle\nabla^{k}v, \nabla\nabla^{k}\sigma \right\rangle(t)\right\} +C\big(\|\nabla^2\sigma\|_{H^{1}}^2\\ & \quad+\|(\nabla(u-v),\nabla^2v)\|_{ H^{2}}^2 +\|\nabla^2u\|_{ H^{1}}^2\big)\lesssim\epsilon\|\nabla (u,\sigma, v)\|_{L^2}^2, \end{aligned} \end{equation}

where $H_1(u,\, \sigma,\, v)$ is equivalent to $\|\nabla (u,\, \sigma,\, v)\|_{ H^{2}}^2$.

Proof. For each multi–index $k$ with $1\leq |k| \leq 3$, by applying the operator $\nabla ^k$ to (2.1) 2–(2.1) 4 and multiplying them by $\nabla ^k u$, $\nabla ^k \sigma$, $\nabla ^k v$ respectively, and then integrating them over $\mathbb {R}^3$, we obtain

(4.17)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t} \|\nabla^k u(t)\|_{L^2}^2+ \|\nabla^k(u-v)\|_{L^2}^2+\left\langle \nabla^k(u-v), \nabla^k u \right\rangle=\left\langle \nabla^k F_1, \nabla^k u\right\rangle, \end{align}
(4.18)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t} \|\nabla^k \sigma(t)\|_{L^2}^2+\bar{n}\left\langle \nabla^k \mathop{\mathrm{div}}\nolimits v, \nabla^k\sigma\right\rangle =\left\langle \nabla^k F_2, \nabla^k \sigma\right\rangle, \end{align}

and

(4.19)\begin{align} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t} \|\nabla^k v(t)\|_{L^2}^2 -\alpha_1\left\langle\nabla^k \mathop{\mathrm{div}}\nolimits v,\nabla^k\sigma\right\rangle+\bar{\mu}\|\nabla^{k+1} v\|_{L^2}^2 +(\bar{\mu}+\bar{\lambda})\|\nabla^k \mathop{\mathrm{div}}\nolimits v\|_{L^2}^2\nonumber\\ & \qquad-\alpha_2\left\langle\nabla^k(u-v), \nabla^k u\right\rangle=\left\langle\nabla^k F_3, \nabla^k v\right\rangle. \end{align}

Computing

\[ \sum_{1\leq k \leq 3}(\alpha_2\bar{n}\times \text{(4.17)}+\alpha_1\times \text{(4.18)}+\bar{n} \text{4.19)}, \]

we have

(4.20)\begin{equation} \begin{aligned} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t}\sum_{1\leq k \leq 3}(\alpha_2\bar{n}\|\nabla^k u(t)\|_{L^2}^2+\alpha_1\|\nabla^k \sigma(t)\|_{L^2}^2+\bar{n}\|\nabla^k v(t)\|_{L^2}^2)\\ & \qquad+C\left(\sum_{1\leq k \leq 3}\|\nabla^k(u-v)\|_{L^2}^2+\sum_{1\leq k \leq 3}\|\nabla^{k+1}v\|_{L^2}^2\right)\\ & \quad\lesssim\sum_{1\leq k \leq 3}\Big|\left\langle \nabla^k F_1, \nabla^k u\right\rangle+\left\langle \nabla^k F_2, \nabla^k \sigma\right\rangle+ \left\langle\nabla^k F_3, \nabla^k v\right\rangle\Big|. \end{aligned} \end{equation}

In what follows, we shall give the estimates of the three terms on the right hand side of the above equation one by one.

Firstly, for the term $\left \langle \nabla ^k F_1,\, \nabla ^k u\right \rangle$, making use of integration by parts, (2.5), lemma 3.6, we obtain

(4.21)\begin{equation} \begin{aligned} & \left|\left\langle \nabla^k F_1, \nabla^k u\right\rangle\right|\\ & \quad\lesssim \left|\int_{\mathbb{R}^3}\mathop{\mathrm{div}}\nolimits u |\nabla^k u|^2\,{\rm d}x\right|\\ & \qquad+\left|\int_{\mathbb{R}^3}\nabla u |\nabla^k u|^2\, {\rm d}x\right|+\mathcal{H}(k-2)\sum_{m=2}^{k-1}\left|\int_{\mathbb{R}^3}\nabla^{k-m+1}u\nabla^m u\nabla^k u\,{\rm d}x\right|\\ & \quad\lesssim \|\nabla u\|_{L^{\infty}}\|\nabla^k u\|_{L^2}^2+\mathcal{H}(k-2)\sum_{m=2}^{k-1}\|\nabla^m u\|_{L^4}\|\nabla^{k-m+1}u\|_{L^4}\|\nabla^k u\|_{L^2}\\ & \quad\lesssim \epsilon \|\nabla^k u\|_{L^2}^2, \end{aligned} \end{equation}

where $\mathcal {H}=\mathcal {X}(0,\, \infty )$ is the Heaviside function, and in the last inequality, we have used lemma 3.7 to get

\begin{align*} & \|\nabla^m u\|_{L^4}\leq C \|\nabla^{5/2 }u\|_{L^2}^{\frac{4(k-m)-3}{4k-10}}\|\nabla^k u\|_{L^2}^{\frac{4m-7}{4k-10}},\\ & \|\nabla^{k-m+1} u\|_{L^4}\leq C \|\nabla^{5/2}u\|_{L^2}^{\frac{4m-7}{4k-10}}\|\nabla^k u\|_{L^2}^{\frac{4(k-m)-3}{4k-10}}. \end{align*}

Using the similar argument as (4.21), $\left \langle \nabla ^k F_2,\, \nabla ^k \sigma \right \rangle$ can be estimated as

(4.22)\begin{equation} \begin{aligned} \left|\left\langle \nabla^k F_2, \nabla^k \sigma\right\rangle\right| & \lesssim \left|\left\langle\nabla^k(v\cdot \nabla \sigma),\nabla^k \sigma\right\rangle\right|+\left|\left\langle\nabla^k( \sigma\mathop{\mathrm{div}}\nolimits v),\nabla^k \sigma\right\rangle\right|\\ & \lesssim \epsilon (\|\nabla^k \sigma\|_{L^2}^2+\|\nabla^k v\|_{L^2}^2+\|\nabla^{ k+1 }v\|_{L^2}^2). \end{aligned} \end{equation}

From the definition of $F_3$, we have from a direct computation that

(4.23)\begin{align} & \left|\left\langle \nabla^k F_3, \nabla^k v\right\rangle\right|\nonumber\\ & \quad\lesssim \left|\left\langle \nabla^k(v\cdot \nabla v),\nabla^k v\right\rangle\right|\nonumber\\ & \qquad+ \left|\left\langle \nabla^k[(\alpha_1-\frac{P'(n)}{n})\nabla \sigma],\nabla^k v\right\rangle\right|+\left|\left\langle \nabla^k[(\frac{\mu}{n}-\bar{\mu})\Delta v],\nabla^k v\right\rangle\right|\nonumber\\ & \qquad+\left|\left\langle \nabla^k[(\frac{\mu+\lambda}{n}-(\bar{\mu}+\bar{\lambda}))\nabla\mathop{\mathrm{div}}\nolimits v],\nabla^k v\right\rangle\right|+\left|\left\langle \nabla^k((\frac{\rho}{n}-\alpha_2)(u-v)),\nabla^k v\right\rangle\right|\nonumber\\ & \quad=I_{11}+I_{12}+I_{13}+I_{14}+I_{15}. \end{align}

Similar to the proof of (4.21), we have from (4.8) that

(4.24)\begin{equation} I_{11}+I_{12}+I_{13}+I_{14}\lesssim \epsilon (\|\nabla^k \sigma\|_{L^2}^2+\|\nabla^k v\|_{L^2}^2+\|\nabla^{ k+1 }v\|_{L^2}^2). \end{equation}

For the term $I_{15}$, it follows from (2.5) and lemma 3.7 that

(4.25)\begin{equation} \begin{aligned} I_{15} & =\left| \left\langle \nabla^{k-1}\left(\left(\frac{\rho}{n}-\alpha_2\right)(u-v)\right),\nabla^{k+1} v\right\rangle \right|\\ & \lesssim \|(\rho, \sigma)\|_{L^{\infty}}\|\nabla^{k}(u-v)\|_{L^2}\|\nabla^{k}v\|_{L^2}\\ & \quad+\mathcal{H}(k-1)\sum_{m=1}^{k-1} \|\nabla^m (u-v)\|_{L^4}\|\nabla^{k-1-m} (\rho, \sigma)\|_{L^4}\|\nabla^{k+1}v\|_{L^2}\\ & \lesssim \epsilon(\|\nabla^{k}(u-v)\|_{L^2}^2+\|\nabla^{k-1} \sigma\|_{L^2}^2+\|\nabla^{k+1}v\|_{L^2}^2+\|\nabla^{k}v\|_{L^2}^2), \end{aligned} \end{equation}

where in the last inequality, we have used the fact that

\[ \|\nabla^m (u-v)\|_{L^4}\leq C\|\nabla^{{3}/{2}}(u-v)\|_{L^2}^{\frac{4(k-m)-3}{4k-6}}\|\nabla^{k} (u-v)\|_{L^2}^{\frac{4m-3}{4k-6}}. \]

Putting (4.24) and (4.25) into (4.23), one has

(4.26)\begin{equation} \left|\left\langle \nabla^k F_3, \nabla^k v\right\rangle\right|\lesssim \epsilon (\|\nabla^{k}(u-v)\|_{L^2}^2+\|\nabla^k \sigma\|_{L^2}^2+\|\nabla^k v\|_{L^2}^2+\|\nabla^{ k+1 }v\|_{L^2}^2). \end{equation}

Substituting (4.21), (4.22) and (4.26) into (4.20) gives

(4.27)\begin{equation} \begin{aligned} & \frac{1}{2}\frac{{\rm d}}{{\rm d}t}\sum_{1\leq k \leq 3}\big(\alpha_2\bar{n}\|\nabla^k u(t)\|_{L^2}^2+\alpha_1\|\nabla^k \sigma(t)\|_{L^2}^2\\ & \quad+\bar{n}\|\nabla^k v(t)\|_{L^2}^2\big)+C\sum_{1\leq k \leq 3}\big(\|\nabla^k(u-v)\|_{L^2}^2\\ & \quad+\sum_{1\leq k \leq 3}\|\nabla^{k+1}v\|_{L^2}^2\big)\lesssim \epsilon\sum_{1\leq k \leq 3}\|\nabla^k ( u, v, \sigma)\|_{L^2}^2. \end{aligned} \end{equation}

On the other hand, for the estimates of $\nabla ^k u\, (1\leq k\leq 3)$, we also have the form as

(4.28)\begin{equation} \|\nabla^k u\|_{L^2}^2\le \|\nabla^k (u-v)\|_{L^2}^2+\|\nabla^k v\|_{L^2}^2. \end{equation}

Combining (4.27) with (4.28), we find that there exists a function $H_1(u,\, \sigma,\, v)$ which is equivalent to $\|\nabla (u,\, \sigma,\, v)\|_{ H^{2}}^2$ and satisfies

(4.29)\begin{align} & \frac{{\rm d}}{{\rm d}t}H_1(u(t), \sigma(t), v(t))+C\left(\|(\nabla (u-v),\nabla^2 v)\|_{ H^{2}}^2+ \|\nabla^2 u\|_{ H^{1}}^2\right)\nonumber\\& \quad \lesssim \epsilon(\|\nabla \sigma\|_{ H^{2}}^2+\|\nabla (u,v)\|_{L^2}^2). \end{align}

Next, we shall derive the energy dissipation for $\|\nabla ^k\nabla \sigma \|_{L^2}^2$ for $1\leq |k|\leq 2$. Applying the operator $\nabla ^k$ to (2.1) 4, multiplying the resulting equation by $\nabla \nabla ^k \sigma$, summing up and integrating it over $\mathbb {R}^3$, we get

(4.30)\begin{equation} \begin{aligned} \alpha_1\|\nabla^2\sigma\|_{H^1}^2 & =\sum_{1\leq |k| \leq 2}\Big(-\left\langle \nabla^k v_t, \nabla\nabla^k\sigma \right\rangle+\bar{\mu}\left\langle\nabla^k(\Delta v), \nabla\nabla^k \sigma \right\rangle\\& \quad +(\bar{\mu} +\bar{\lambda})\left\langle\nabla^k \nabla \mathop{\mathrm{div}}\nolimits v, \nabla\nabla^k\sigma\right\rangle\\ & \quad+\alpha_2\left\langle\nabla^k(u-v), \nabla\nabla^k \sigma\right\rangle+\left\langle\nabla^k F_3, \nabla\nabla^k\sigma\right\rangle\Big)\\ & =I_{21}+I_{22}+I_{23}+I_{24}+I_{25}. \end{aligned} \end{equation}

For the term $I_{21}$, by virtue of (2.1) 3, we can apply integration by parts, H${\rm \ddot o}$lder inequality, Lemma (3.7) and lemma 3.2 to deduce that

(4.31)\begin{align} I_{21} & ={-}\frac{{\rm d}}{{\rm d}t}\sum_{1\leq |k| \leq 2}\left\langle\nabla^{k}v, \nabla\nabla^{k}\sigma \right\rangle(t)-\left\langle \nabla^k \mathop{\mathrm{div}}\nolimits v,\nabla^k\sigma_t \right\rangle \nonumber\\ & ={-}\frac{{\rm d}}{{\rm d}t}\sum_{1\leq |k| \leq 2}\left\langle\nabla^{k}v, \nabla\nabla^{k}\sigma \right\rangle(t)+\bar{n}\|\nabla^k \mathop{\mathrm{div}}\nolimits v\|_{L^2}^2+\left\langle\nabla^k(v\cdot \nabla \sigma), \nabla^k \mathop{\mathrm{div}}\nolimits v\right\rangle \nonumber\\ & \quad+\left\langle\nabla^k(\sigma\cdot \mathop{\mathrm{div}}\nolimits v), \nabla^k \mathop{\mathrm{div}}\nolimits v\right\rangle \nonumber\\ & \le -\frac{{\rm d}}{{\rm d}t}\sum_{1\leq |k| \leq 2}\left\langle\nabla^{k}v, \nabla\nabla^{k}\sigma \right\rangle(t)+C\left(\epsilon\|\nabla^{k+1}\sigma\|_{L^2}^2+\|\nabla^{k+1}v\|_{L^2}^2\right). \end{align}

For the terms $I_{22}$, $I_{23}$ and $I_{24}$, by the similar argument, we have

(4.32)\begin{equation} |I_{22}|+|I_{23}|+|I_{24}|\lesssim \epsilon(\|\nabla^{k+1}\sigma\|_{L^2}^2+\|\nabla^{k+2} v\|_{L^2}^2+\|\nabla^k(u-v)\|_{L^2}^2). \end{equation}

For the term $I_{25}$, we write it as

(4.33)\begin{align} \quad I_{25} & ={-}\left\langle\nabla^k(v\cdot \nabla v), \nabla\nabla^k\sigma\right\rangle+\left\langle \nabla^k[(\alpha_1-\frac{P'(n)}{n})\nabla \sigma], \nabla\nabla^k\sigma\right\rangle\nonumber\\ & \quad+\left\langle\nabla^k[(\frac{\mu}{n}-\bar{\mu})K_0 v],\nabla\nabla^k\sigma\right\rangle+\left\langle \nabla^k[\frac{\mu+\lambda}{n}-(\bar{\mu}+\bar{\lambda})\nabla\mathop{\mathrm{div}}\nolimits v], \nabla\nabla^k\sigma\right\rangle\nonumber\\ & \quad+\left\langle\nabla^k[(\frac{\rho}{n}-\alpha_2)(u-v)], \nabla\nabla^k\sigma\right\rangle\nonumber\\ & =I_{251}+I_{252}+I_{253}+I_{254}+I_{255}. \end{align}

For the terms $I_{251}$–$I_{254}$, using (4.8) and making a direct computation, we have

(4.34)\begin{align} |I_{251}|+|I_{252}|+|I_{253}|+|I_{254}|\lesssim \epsilon(\|\nabla^{k+1}\sigma\|_{L^2}^2+\|\nabla^{k+1}v\|_{L^2}^2+\|\nabla^{k+2}v\|_{L^2}^2). \end{align}

For the term $I_{255}$, employing the similar argument used in the proof of (4.25), we get that

(4.35)\begin{equation} \begin{aligned} |I_{255}| & \lesssim \| \frac{\rho}{n}-\alpha_2\|_{L^{\infty}}\|\nabla^k(u-v)\|_{L^2}\|\nabla\nabla^k\sigma\|_{L^2}\\ & \quad+\mathcal{H}(k-1)\sum_{m=1}^{k-1}\|\nabla^m(u-v)\|_{L^4}\|\nabla^{k-1-m}(\rho, \sigma)\|_{L^4}\|\nabla\nabla^k \sigma\|_{L^2}\\ & \lesssim \epsilon(\|\nabla^k(u-v)\|_{L^2}^2+\|\nabla^{k+1}\sigma\|_{L^2}^2+\|\nabla^{k-1}\sigma\|_{L^2}^2). \end{aligned} \end{equation}

Substituting (4.35) and (4.34) into (4.33) yields

(4.36)\begin{align} |I_{25}|\lesssim K_0(\|\nabla^{k}(u-v)\|_{L^2}^2+\|\nabla^{k+1}\sigma\|_{L^2}^2+\|\nabla^{k+1}v\|_{L^2}^2+\|\nabla^{k+2}v\|_{L^2}^2+\|\nabla^k\sigma\|_{L^2}^2). \end{align}

Adding (4.31), (4.32) and (4.36) into (4.30), we obtain

(4.37)\begin{equation} \begin{aligned} & \frac{{\rm d}}{{\rm d}t}\sum_{1\leq |k| \leq 2}\left\langle\nabla^{k}v, \nabla\nabla^{k}\sigma \right\rangle(t)+C\sum_{1\leq k \leq 2}\|\nabla^k\nabla\sigma\|_{L^2}^2\\ & \quad\lesssim \epsilon(\|\nabla\sigma\|_{L^2}^2+\|\nabla(u-v)\|_{H^{1}}^2+\|\nabla^2 v\|_{ H^{2}}^2). \end{aligned} \end{equation}

Since $K_0$ is sufficiently small, multiplying (4.29) by $D_2$ suitably large and adding it to (4.37), we have (4.16). Thus, we complete the proof of the lemma.

5. The proof of global well–posedness

In this section, we are devoted to proving proposition 2.2. We will do it by three steps.

Step 1: Combining lemma 4.1 with lemma 4.2, there exists a function $H_2(u,\, \sigma,\, v)$ which is equivalent to $\|(u,\,\sigma,\, v)\|_{H^{3}}$ and satisfies

(5.1)\begin{equation} \frac{{\rm d}}{{\rm d}t}H_2(u,\sigma,v)+\|\nabla(u, \sigma)\|_{H^2}^2+\|(u-v, \nabla v)\|_{H^{3}}^2\lesssim 0,\end{equation}

for any $0\leq t\leq T$, which implies (2.6).

Step 2: From (2.1) $_2$ and (2.1) $_4$, we see that

\[ \nonumber\partial_t(u-v)+(1+\alpha_2)(u-v)=F_1+F_3-\alpha_1\nabla\sigma+\bar\mu\Delta v-(\bar\mu+\bar\lambda)\nabla\text{div}v.\]

Performing the similar procedure as in lemma 4.1, we have

(5.2)\begin{equation} \frac{{\rm d}}{{\rm d}t}\|(u-v)(t)\|_{L^2}^2+(1+\alpha_2)\|(u-v)\|_{L^2}^2\lesssim \|\nabla(u,\sigma,v)\|_{H^1}^2.\end{equation}

Now we define the temporal energy functional

\[ H_3(t)=D_3H_1(u(t), \sigma(t), v(t))+\sum_{1\leq k \leq2}\left\langle\nabla^{k}v, \nabla\nabla^{k}\sigma\right\rangle+\|(u-v)(t)\|_{L^2}^2, \]

for any $0\leq t\leq T$, where it is noticed that $H_3(t)$ is equivalent to $\|\nabla (u,\,\sigma,\, v)\|_{ H^{2}}^2$ since $D_3$ is large enough.

Using lemma 4.2, we obtain

(5.3)\begin{align} & \frac{{\rm d}}{{\rm d}t}H_3(t)+C(\|\nabla^2\sigma\|_{H^{1}}^2+\|\nabla^2 v\|_{ H^{2}}^2+\|\nabla^2 u\|_{H^{1}}^2)\notag\\& \quad \lesssim \epsilon\|\nabla( u,\sigma, v)\|_{L^2}^2+\|\nabla( u^l,\sigma^l, v^l)\|_{L^2}^2. \end{align}

Adding $\|\nabla (u^l,\,\sigma ^l,\, v^l)\|^2$ to both side of (5.3), we deduce that there exists a suitably large constant $D_4>0$ which is independent of $\epsilon$, such that

(5.4)\begin{equation} \frac{{\rm d}}{{\rm d}t}H_3(t)+\frac{1}{D_4}H_3(t) \lesssim \|\nabla(u^l,\sigma^l, v^l)\|_{L^2}^2, \end{equation}

where we have used the fact that $\|\nabla (u^h,\,\sigma ^h,\, v^h)\|_{L^2}\leq \|\nabla ^2 (u,\, \sigma,\, v)\|_{L^2}$. If we define

(5.5)\begin{equation} M(t)=\sup_{0\leq \tau\leq t}(1+\tau)^{\frac{5}{2}}H_3 (\tau), \end{equation}

then

(5.6)\begin{equation} \|\nabla(u,\sigma, v)\|_{H^{2}}\leq C\sqrt{H_3(t)}\leq C (1+\tau)^{-{5}/{4}}\sqrt{M(t)}, \quad 0\leq \tau\leq t\leq T. \end{equation}

To close the estimate (5.4), we will derive the time–decay estimate of $\|\nabla (u,\,\sigma,\, v)\|_{L^2}^2$.

From Duhamel's principle, the solutions of system (2.1) have the form as

(5.7)\begin{equation} U=e^{{-}t\mathcal {B}}U(0)+\int_0^t e^{-(t-\tau)B}\mathcal {F}(\tau)\,{\rm d}\tau.\end{equation}

By virtue of proposition 3.5, Plancherel theorem, H${\rm \ddot o}$lder inequality, and the Hausdorff-Young inequality, we have

(5.8)\begin{align} & \|\nabla (u^l(t), \sigma^l(t), v^l(t))\|_{L^2}\nonumber\\ & \quad\leq C(1+t)^{-\frac{5}{4}}\| (u,\sigma, v)(0)\|_{L^1}+\int_0^t (1+t-\tau)^{-\frac{5}{ 4}}\|(F_1, F_2, F_3)(\tau)\|_{L^1\cap H^1}\,{\rm d}\tau\nonumber\\ & \quad\leq C\left(\delta_0(1+t)^{-{5}/{4}}+\epsilon\int_0^t(1+t-\tau)^{-{5}/{4}}(1+\tau)^{-{5}/{4}}\sqrt{M(t)}{\rm d}\tau\right)\nonumber\\ & \quad\leq C(1+t)^{-{5}/{4}}(\delta_0+\epsilon\sqrt{M(t)} ), \end{align}

where we have used the fact that

(5.9)\begin{align} & \|(F_1, F_2, F_3)\|_{L^1}\leq C\epsilon\|\nabla(u,\sigma, v)\|_{H^1}\leq C\epsilon\|\nabla(u, \sigma,v)\|_{ H^{2}}. \end{align}
(5.10)\begin{align} & \|(F_1,F_2, F_3)\|_{H^1}\leq C\epsilon\|\nabla(u,\sigma,v)\|_{ H^{2}}. \end{align}

Hence, by using Gronwall's inequality and putting (5.8) into (5.4), we have

(5.11)\begin{equation} \begin{aligned} H_3(t) & \leq e^{-\frac{1}{D_4}t}H_3(0)+C\int_0^te^{-\frac{1}{D_4}(t-\tau)}\|\nabla (u(\tau),\sigma(\tau), v(\tau))\|_{L^2}^2\,{\rm d}\tau\\ & \leq e^{-\frac{1}{D_4}t}H_3(0)+C\int_0^te^{-\frac{1}{D_4}(t-\tau)}(1+\tau)^{-\frac{5}{2}}(\delta_0^2+\epsilon^2 M(t))\,{\rm d}\tau\\ & \leq C(1+t)^{-\frac{5}{2}}(\delta_0^2+\epsilon^2 M(t)). \end{aligned} \end{equation}

Since $M(t)$ is non–decreasing, we have from (5.5) and (5.11) that

\[ M(t)\leq C(N_0^2+K_0^2 M(t)), \]

for any $0\leq t\leq T$, which implies that

\[ M(t)\leq C N_0^2, \]

since $K_0>0$ is small enough. Thus we obtain (2.8).

Next, by making use of proposition 3.5, (5.9) and (5.10), from Duhamel's principle, we obtain

(5.12)\begin{align} & \| (u^l, \sigma^l, v^l)(t)\|_{L^2}\nonumber\\ & \quad\leq C(1+t)^{-\frac{3}{4}}\| (u, \sigma, v)(0)\|_{L^1}+\int_0^t (1+t-\tau)^{-\frac{3}{ 4}}\|(F_1, F_2, F_3)(\tau)\|_{L^1\cap L^2}\,{\rm d}\tau\nonumber\\ & \quad\leq C\delta_0\left((1+t)^{-{3}/{4}}+\int_0^t(1+t-\tau)^{-{3}/{4}}(1+\tau)^{-{5}/{4}}{\rm d}\tau\right)\nonumber\\ & \quad\leq C\delta_0(1+t)^{-{3}/{4}}, \end{align}

for any $0\leq t\leq T$. Thus, this together with the fact that $\|(u^h,\,\sigma ^h,\, v^h)\|_{L^2}\leq \|\nabla (u,\, \sigma,\, v)\|_{L^2}$, we get

(5.13)\begin{equation} \|(u, \sigma, v)(t)\|_{L^2} \lesssim \| (u^l, \sigma^l, v^l)(t)\|_{L^2}+\|\nabla(u, \sigma, v)(t)\|_{L^2}\leq C\delta_0(1+t)^{-{3}/{4}} \end{equation}

which implies (2.9).

Step 3: Multiplying (2.1) 1 by $\varrho$, integrating over $\mathbb {R}^3$ and using Cauchy-Schwarz inequality, we have

(5.14)\begin{equation} \frac{{\rm d}}{{\rm d}t}\|\varrho(t)\|_{L^2}^2={-}\left\langle\mathop{\mathrm{div}}u,\varrho \right\rangle-\left\langle u\cdot\nabla\varrho,\varrho\right\rangle\lesssim\|\nabla u\|_{H^{2}}\|\varrho\|_{L^2}. \end{equation}

Next, applying the operator $\nabla ^k$ to (2.1) 1, Multiplying it by $\nabla ^k\varrho$, and integrating over $\mathbb {R}^3$, for $1\leq |k|\leq 2$, we get

(5.15)\begin{equation} \frac 1 2 \frac{{\rm d}}{{\rm d}t}\|\nabla^k \varrho(t)\|_{L^2}^2={-}\left\langle\nabla^k\mathop{\mathrm{div}} u,\nabla^k \varrho \right\rangle-\left\langle\nabla^k(u\cdot\nabla\varrho),\nabla^k \varrho\right\rangle.\end{equation}

It is easy to obtain

(5.16)\begin{equation} \left|\left\langle\nabla^k \mathop{\mathrm{div}} u, \nabla^k \varrho \right\rangle\right|\lesssim \|\nabla^{k+1} u\|_{L^2}\|\nabla^k\varrho\|_{L^2}, \end{equation}

and

\begin{align*} \left|\left\langle\nabla^k(u\cdot\nabla\rho),\nabla^k \rho \right\rangle\right|& \lesssim \left|\left\langle(u\cdot\nabla\nabla^k\rho),\nabla^k \rho \right\rangle\right|+\sum\limits_{|m|=0}^{|k|-1}\left|\left\langle(\nabla^{k-m}u)\cdot\nabla\nabla^m\rho),\nabla^k \rho \right\rangle\right| \\ & \lesssim\|\nabla u\|_{H^{2}}\|\nabla\varrho\|_{H^1}. \end{align*}

Thus, for $1\leq |k| \leq 2$, we have

(5.17)\begin{equation} \frac{{\rm d}}{{\rm d}t}\|\nabla^k \rho(t)\|_{L^2}^2\lesssim \|\nabla u\|_{H^{2}}\|\nabla\varrho\|_{H^1}. \end{equation}

Combining (5.14) with (5.17), we arrive at

\[ \frac{{\rm d}}{{\rm d}t}\|\rho(t)\|_{ H^{2}}\lesssim \|\nabla u\|_{ H^{2}} \lesssim(1+t)^{-{5}/{4}}\delta_0. \]

Integrating the above inequality from $0$ to $t$, we obtain (2.7). For (2.10), making use of the above estimates and (2.1), we have

\begin{align*} \|\partial_t (\rho, u, \sigma, v)\|_{L^2}& \leq C(\|\nabla u\|_{L^2}+\|\nabla \sigma\|_{L^2}+\|\nabla v\|_{H^1})\\ & \leq C N_0(1+t)^{-{5}/{4}}. \end{align*}

for any $0\leq t\leq T$. Thus, we get (2.10).

Therefore, we have complete the proof of proposition 2.2.

Acknowledgements

Shanshan Guo's research was partially supported by National Natural Science Foundation of China $\#$12001074, and Natural Science Foundation of Chongqing $\#$cstc2020jcyj–msxmX0606, and the Science and Technology Research Program of Chongqing Municipal Education Commission $\#$ KJQN202000536 and the Open Project of Key Laboratory $\#$CSSXKFKTQ202008, Mathematical College, Chongqing Normal University. Guochun Wu's research was partially supported by National Natural Science Foundation of China $\#$12271114, and Natural Science Foundation of Fujian Province $\#$2022J01304. Yinghui Zhang’ research is partially supported by National Natural Science Foundation of China $\#$12271114, and Guangxi Natural Science Foundation $\#$2019JJG110003, $\#$2019AC20214.

References

Baranger, C., Boudin, L., Jabin, P.-E. and Mancini, S.. A modeling of biospray for the upper airways. CEMRACS 2004-mathematics and applications to biology and medicine. ESAIM Proc. 14 (2005), 41–47.CrossRefGoogle Scholar
Boudin, L., Desvillettes, L. and Motte, R.. A modelling of compressible droplets in a fluid. Commun. Math. Sci. 1 (2003), 657–669.CrossRefGoogle Scholar
Boudin, L., Desvillettes, L., Grandmont, C. and Moussa, A.. Global existence of solutions for the coupled Vlasov and Navier–Stokes equations. Differ. Int. Equ. 22 (2009), 1247–1271.Google Scholar
Boudin, L., Grandmont, C. and Moussa, A.. Global existence of solutions to the incompressible Navier–Stokes–Vlasov equations in a time-dependent domain. J. Differ. Equ. 262 (2017), 1317–1340.CrossRefGoogle Scholar
Carrillo, J. A., Choi, Y.-P. and Karper, T. K.. On the analysis of a coupled kinetic–fluid model with local alignment forces. Ann. Inst. H. Poincar Anal. Non Linaire 33 (2016), 273–307.CrossRefGoogle Scholar
Carrillo, J. A. and Goudon, T.. Stability and asymptotic analysis of a fluid–particle interaction model. Comm. Partial Differ. Equ. 31 (2006), 1349–1379.CrossRefGoogle Scholar
Carrillo, J. A., Choi, Y.-P., Tadmor, E. and Tan, C.. Critical thresholds in 1D Euler equations with nonlocal forces. Math. Models Methods Appl. Sci. 26 (2016), 185–206.CrossRefGoogle Scholar
Carrillo, J. A., Choi, Y.-P. and Zatorska, E.. On the pressureless damped Euler–Poisson equations with quadratic confinement: critical thresholds and large–time behavior. Math. Models Methods Appl. Sci. 26 (2016), 2311–2340.CrossRefGoogle Scholar
Chen, G.-Q. and Wang, D.. Convergence of shock capturing schemes for the compressible Euler–Poisson equations. Commun. Math. Phys. 179 (1996), 333–364.CrossRefGoogle Scholar
Chen, Q. and Tan, Z.. Time decay of solutions to the compressible Euler equations with damping. Kinetic Related Models 7 (2014), 605–619.CrossRefGoogle Scholar
Choi, Y.-P.. Compressible Euler equations interacting with incompressible flow. Kinet. Relat. Models 8 (2015), 335–358.CrossRefGoogle Scholar
Choi, Y.-P. and Kwon, B.. The Cauchy problem for the pressureless Euler/isentropic Navier–Stokes equations. J. Differ. Equ. 261 (2016), 654–711.CrossRefGoogle Scholar
Choi, Y.-P.. Global classical solutions and large–time behavior of the two–phase fluid model. SIAM J. Math. Anal. 48 (2016), 3090–3122.CrossRefGoogle Scholar
Choi, Y.-P. and Jung, J.. On the Cauchy problem for the pressureless Euler–Navier–Stokes system in the whole space. J. Math. Fluid Mech. 23 (2021), 16.CrossRefGoogle Scholar
Engelberg, S.. Formation of singularities in the Euler and Euler–Poisson equations. Physica D 98 (1996), 67–74.CrossRefGoogle Scholar
Ertzbischoff, L.. Decay and absorption for the Vlasov–Navier–Stokes system with gravity in a half–space, arXiv:2107.02200 (2021).Google Scholar
Ertzbischoff, L., Han-Kwan, D. and Moussa, A.. Concentration versus absorption for the Vlasov–Navier–Stokes system on bounded domains, arXiv:2101.05157v1 (2021).CrossRefGoogle Scholar
Goudon, T., Jabin, P.-E. and Vasseur, A.. Hydrodynamic limit for the Vlasov–Navier–Stokes equations: I. Light particles regime. Indiana Univ. Math. J. 53 (2004), 1495–1515.CrossRefGoogle Scholar
Goudon, T., Jabin, P.-E. and Vasseur, A.. Hydrodynamic limit for the Vlasov–Navier–Stokes equations: II. Fine particles regime. Indiana Univ. Math. J. 53 (2004), 1517–1536.CrossRefGoogle Scholar
Ha, S.-Y., Kang, M.-J. and Kwon, B.. A hydrodynamic model for the interaction of Cucker–Smale particles and incompressible fluids. Math. Models Methods Appl. Sci. 24 (2014), 2311–2359.CrossRefGoogle Scholar
Han-Kwan, D.. Large time behavior of small data solutions to the Vlasov–Navier–Stokes system on the whole space, arXiv:2006.09848v1 (2020).Google Scholar
Han-Kwan, D., Moussa, A. and Moyano, I.. Large time behavior of the Vlasov–Navier–Stokes system on the torus. Arch. Rational Mech. Anal. 236 (2020), 1273–1323.CrossRefGoogle Scholar
Kato, T.. The Cauchy problem for quasi–linear symmetric hyperbolic systems. Arch. Rational Mech. Anal. 58 (1975), 181–205.CrossRefGoogle Scholar
Liu, H. and Tadmor, E.. Spectral dynamics of the velocity gradient field in restricted fluid flows. Commun. Math. Phys. 228 (2002), 435–466.CrossRefGoogle Scholar
Majda, A.. Compressible fluid flow and systems of conservation laws in several space variables (Berlin/New York: Springer-Verlag, 1984).CrossRefGoogle Scholar
Matsumura, A. and Nishida, T.. The initial value problem for the equation of motion of compressible viscous and heat–conductive fluids. Proc. Japan Acad. Ser. A Math. Sci. 55 (1979), 337–342.CrossRefGoogle Scholar
Mellet, A. and Vasseur, A.. Asymptotic analysis for a Vlasov-Fokker-Planck/compressible Navier-Stokes equations. Comm. Math. Phys. 281 (2008), 573–596.CrossRefGoogle Scholar
Nirenberg, L.. On elliptic partial differential equations. Annali della Scuola Normale Superiore di Pisa–Classe di Scienze 13 (1959), 115–162.Google Scholar
ORourke, P. J.. Collective drop effects on vaporizing liquid sprays, PhD thesis, Los Alamos National Laboratory, 1981.Google Scholar
Vinkovic, I., Aguirre, C. and Simoens, S.. Large eddy simulation of droplet dispersion for inhomogeneous turbulent wall flow. Int. J. Multiph. Flow 32 (2006), 344–364.CrossRefGoogle Scholar
Williams, F. A.. Spray combustion and atomization. Phys. Fluids 1 (1958), 541–555.CrossRefGoogle Scholar
Wu, G. C., Zhang, Y. H. and Zou, L.. Optimal large–time behavior of the two–phase fluid model in the whole space. SIAM J. Math. Anal. 52 (2020), 5748–5774.CrossRefGoogle Scholar
Yu, C.. Global weak solutions to the incompressible Navier–Stokes–Vlasov equations. J. Math.Pures Appl. 100 (2013), 275–293.CrossRefGoogle Scholar