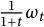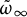1 Introduction
Oeljeklaus–Toma manifolds are a very interesting class of complex manifolds introduced and first studied in [Reference Oeljeklaus and Toma17]. These manifolds are defined as compact quotients of the type
$$ \begin{align*}M=\frac{\mathbb H^r\times\mathbb C^s}{U \ltimes \mathcal O_{\mathbb{K}}}, \end{align*} $$
where
$\mathbb {H}\subseteq {\mathbb C}$
is the upper half-plane,
$\mathcal O_{\mathbb {K}}$
is the ring of algebraic integers of an algebraic extension
$\mathbb K$
of
$\mathbb Q$
satisfying
$[\mathbb K : \mathbb Q]=r+2s$
, and U is a free subgroup of rank r of
$\mathcal O^{*,+}_{\mathbb {K}}$
satisfying some compatible conditions. The action of
$U \ltimes \mathcal O_{\mathbb {K}}$
on
$\mathbb H^r\times \mathbb C^s$
is defined via some embeddings of
$\mathbb K$
in
$\mathbb R$
and
$\mathbb C$
. Oeljeklaus–Toma manifolds have a rich geometric structure. For instance, they have a natural structure of
$\mathbb T^{r+2s}$
-torus bundle over a
$\mathbb T^r$
and a structure of solvmanifold [Reference Kasuya13], i.e., they are always compact quotients of a solvable Lie group by a lattice. The Poincaré metricFootnote
1
$\omega _{\mathbb H^r}=\sqrt {-1}\sum _{a=1}^r\frac {dz_a\wedge d\bar z_a}{4(\Im \mathfrak m z_a)^2}$
induces a degenerate metric
$\omega _\infty $
on M which has a central role in the study of geometric flows on these manifolds. The pair
$(r,s)$
is called the type of the manifold. The case of type
$(r,s)=(1,1)$
corresponds to the Inoue–Bombieri surfaces [Reference Inoue11].
In [Reference Angella and Tosatti2, Reference Fang, Tosatti, Weinkove and Zheng7, Reference Tosatti and Weinkove28, Reference Zheng32], the Chern–Ricci flow [Reference Gill10, Reference Tosatti and Weinkove29] on Oeljeklaus–Toma manifolds M of type
$(r,1)$
is studied. According to the results in [Reference Angella and Tosatti2, Reference Fang, Tosatti, Weinkove and Zheng7, Reference Tosatti and Weinkove28, Reference Zheng32], under some assumptions on the initial Hermitian metric, the flow has a long-time solution
$\omega _t$
such that
$(M,\frac {\omega _t}{1+t})$
converges in the Gromov–Hausdorff sense to an r-dimensional torus
$\mathbb T^r$
as
$t\to \infty $
. The result can be adapted to Oeljeklaus–Toma manifolds of arbitrary type by assuming the initial metric to be left-invariant with respect to the structure of solvmanifold. Moreover, a result of Lauret in [Reference Lauret15, Reference Lauret and Rodríguez Valencia16] allows us to give a characterization of left-invariant Hermitian metrics on an Oeljeklaus–Toma manifold which lift to an algebraic soliton of the Chern–Ricci flow on the universal covering of the manifold (see Proposition 4.1).
Following the same approach, we focus on the pluriclosed flow on Oeljeklaus–Toma manifolds when the initial pluriclosed Hermitian metric is left-invariant. The pluriclosed flow is a geometric flow of pluriclosed metrics, i.e. of Hermitian metrics having the fundamental form
$\partial \bar \partial $
-closed, introduced by Streets and Tian in [Reference Streets and Tian25]. The flow belongs to the family of the Hermitian curvature flows [Reference Streets and Tian26] and evolves an initial pluriclosed metric along the
$(1,1)$
-component of the Bismut–Ricci form. Namely, on a Hermitian manifold
$(M,\omega )$
, there always exists a unique metric connection
$\nabla ^B$
, called the Bismut connection [Reference Bismut4], preserving the complex structure and such that
$$ \begin{align*}\omega(T^B(\cdot,\cdot),J\cdot)\quad \mbox{is a }3\mbox{-form}, \end{align*} $$
where
$T^B$
is the torsion of
$\nabla ^B$
. The Bismut–Ricci form of
$\omega $
is then defined as
$$ \begin{align*}\rho_B(X,Y):=\sqrt{-1}\sum_{i=1}^n R_B(X,Y,X_i,\bar X_i), \end{align*} $$
where
$R_B$
is the curvature tensor of
$\nabla ^B$
and
$\{X_i\}$
is a unitary frame with respect to
$\omega $
.
$\rho _B$
is always a closed real form. Given a pluriclosed Hermitian metric
$\omega $
on M, the pluriclosed flow is then defined as the geometric flow of pluriclosed metrics governed by the equation
$$ \begin{align*}\partial_t\omega_t=-\rho_B^{1,1}(\omega_t),\quad \omega_{|t=0}=\omega. \end{align*} $$
The pluriclosed flow was deeply studied in literature (see, for instance, [Reference Arroyo and Lafuente3, Reference Boling5, Reference Enrietti, Fino and Vezzoni6, Reference Garcia-Fernandez, Jordan and Streets9, Reference Jordan and Streets12, Reference Pujia and Vezzoni19–Reference Streets24, Reference Streets and Tian27] and the references therein).
Our main result is the following theorem.
Theorem 1.1 Let
$\omega $
be a left-invariant pluriclosed Hermitian metric on an Oeljeklaus–Toma manifold M. Then the pluriclosed flow starting from
$\omega $
has a long-time solution
$\omega _t$
such that
$(M, \frac {\omega _t}{1+t})$
converges in the Gromov–Hausdorff sense to
$(\mathbb T^s,d)$
. Moreover,
$\omega $
lifts to an expanding algebraic soliton on the universal covering of M if and only if it is diagonal and the first s diagonal components coincide. Finally,
$(\mathbb H^s\times \mathbb C^s, \frac {\omega _t}{1+t})$
converges in the Cheeger–Gromov sense to
$(\mathbb H^s\times \mathbb C^s, \tilde {\omega }_{\infty })$
, where
$\tilde {\omega }_{\infty }$
is an algebraic soliton.
Here, we recall that a left-invariant Hermitian metric
$\omega $
on a Lie group G with a left-invariant complex structure is an algebraic soliton for a geometric flow of left-invariant Hermitian metrics if
$\omega _t=c_t\varphi _t^*(\omega )$
solves the flow, where
$\{c_t\}$
is a positive scaling and
$\{\varphi _t\}$
is a family of automorphims of G preserving the complex structure. Moreover, the distance d in the statement is the distance induced by
$3\omega _{\infty }$
on the torus base of M. Now, we describe the condition diagonal appearing in the statement of Theorem 1.1. The existence of a pluriclosed metric on an Oeljeklaus–Toma manifold imposes some restrictions (see [Reference Angella, Dubickas, Otiman and Stelzig1, Corollary 3]). In particular, the manifold has type
$(s,s)$
and admits a left-invariant
$(1,0)$
-coframe
$\{\omega ^1,\dots ,\omega ^s,\gamma ^1,\dots ,\gamma ^s\}$
satisfying
$$ \begin{align*} \begin{cases} d\omega^k=\frac{\sqrt{-1}}{2}\omega^k\wedge\bar \omega^k,\quad & k=1,\dots,s,\\ d\gamma^i=\sum_{k=1}^s\lambda_{ki}\,\omega^k\wedge \gamma^{i}-\sum_{k=1}^s\,\lambda_{ki}\bar{\omega}^{k}\wedge\gamma^i,\quad& i=1,\dots, s, \end{cases} \end{align*} $$
with
$$ \begin{align*}\Im\mathfrak m\, \lambda_{ki}=-\frac14\,\delta_{ik} . \end{align*} $$
By
$\omega $
diagonal, we mean that it takes a diagonal form with respect to such a coframe. The first part of Theorem 1.1 in the case of the Inoue–Bombieri surfaces is proved in [Reference Boling5, Corollary 3.18].
Theorem 1.1 provides a description of the long-time behavior of the solution
$\omega _t$
to the pluriclosed flow as
$t\to \infty $
. For the definition of the convergence in the Gromov–Hausdorff sense, we refer to Section 3, whereas here we briefly recall the definition of convergence in the Cheeger–Gromov sense: a sequence of pointed Riemannian manifolds
$(M_k, g_k, p_k)$
converges in the Cheeger–Gromov sense to a pointed Riemannian manifold
$(M,g,p)$
if there exists a sequence of open subsets
$A_k$
of M so that every compact subset of M eventually lies in some
$A_k$
, and a sequence of smooth maps
$\phi _k\colon A_k\to M_k $
which are diffeomorphisms onto some open set of
$M_k$
which satisfy
$\phi _k(p_k)=p$
, such that
$$ \begin{align*}\phi_k^*(g_k)\to g\quad \mbox{smoothly on every compact subset, as } k\to \infty. \end{align*} $$
See [Reference Lauret14, Section 6] for a deep analysis of Cheeger–Gromov convergence both in the general case and in the homogeneous one and [Reference Lauret15, Section 5.1] for the case of Hermitian Lie groups.
2 Definition of Oeljeklaus–Toma manifolds
We briefly recall the construction of Oeljeklaus–Toma manifolds [Reference Oeljeklaus and Toma17].
Let
$\mathbb {Q}\subseteq \mathbb {K}$
be an algebraic number field with
$[\mathbb {K}:\mathbb {Q}]=r+2s$
and
$r,s\ge 1$
. Let
$\sigma _1,\ldots , \sigma _r\colon \mathbb {K}\to \mathbb {R}$
be the real embeddings of
$\mathbb {K}$
and
$\sigma _{r+1},\ldots ,\sigma _{r+2s}\colon \mathbb {K}\to \mathbb {C}$
be the complex embeddings of
$\mathbb {K}$
satisfying
$\sigma _{r+s+i}=\bar \sigma _{r+i},$
for every
$ i=1,\ldots , s.$
We denote by
$\mathcal {O}_{\mathbb {K}}$
the ring of algebraic integers of
$\mathbb {K}$
and by
$\mathcal {O}_{\mathbb {K}}^*$
the group of units of
$\mathcal {O}_{\mathbb {K}}$
. Let
$$ \begin{align*}\mathcal{O}_{\mathbb{K}}^{*,+}=\{u\in\mathcal{O}_{\mathbb{K}}^* \quad | \quad \sigma_i(u)>0\, , \quad \mbox{for every } i=1,\ldots, r\} \end{align*} $$
be the group of totally positive units of
$\mathcal {O}_{\mathbb {K}}$
. The groups
$\mathcal {O}_{\mathbb {K}}$
and
$\mathcal {O}_{\mathbb {K}}^{*,+}$
act on
$\mathbb H^r\times \mathbb C^s$
as
$$ \begin{align*}&a\cdot(z_1,\ldots, z_r,w_1,\ldots, w_s)\\\quad&=(z_1+\sigma_1(a),\ldots, z_r+\sigma_r(a), w_1+\sigma_{r+1}(a),\ldots, w_s+\sigma_{r+s}(a)), \quad \mbox{for all } a\in \mathcal{O}_{\mathbb{K}} \end{align*} $$
and
$$ \begin{align*}&u\cdot(z_1,\ldots, z_r,w_1,\ldots, w_s)\\\quad&=(\sigma_1(u)z_1,\ldots, \sigma_r(u)z_r, \sigma_{r+1}(u)w_1,\ldots, \sigma_{r+s}(u) w_s)\, , \quad \mbox{for every } u\in \mathcal{O}_{\mathbb{K}}^{*,+}. \end{align*} $$
There always exists a free subgroup U of rank r of
$\mathcal {O}_{\mathbb {K}}^{*,+}$
such that
$\mathrm { pr}_{\mathbb {R}^r}\circ l(U)$
is a lattice of rank r in
$\mathbb {R}^r$
, where
$l\colon \mathcal {O}_{\mathbb {K}}^{*,+}\to \mathbb {R}^{r+s}$
is the logarithmic representation of units
$$ \begin{align*}l(u)=(\log \sigma_1(u),\ldots, \log\sigma_r(u),2\log\lvert\sigma_{r+1}(u)\rvert,\ldots, 2\log\lvert\sigma_{r+s}(u)\rvert) \end{align*} $$
and
$\mathrm {pr}_{\mathbb {R}^r}\colon \mathbb {R}^{r+s}\to \mathbb {R}^r$
is the projection on the first r coordinates. The action of
$U\ltimes \mathcal {O}_{\mathbb {K}}$
on
$\mathbb H^r\times \mathbb C^s$
is free, properly discontinuous, and co-compact. An Oeljeklaus–Toma manifold is then defined as the quotient
$$ \begin{align*}M:=\frac{\mathbb H^r\times \mathbb C^s}{U\ltimes\mathcal{O}_{\mathbb{K}}}, \end{align*} $$
and it is a compact complex manifold having complex dimension
$r+s$
.
The structure of torus bundle of an Oeljeklaus–Toma manifold can be seen as follows: we have
$$ \begin{align*}\frac{\mathbb H^r\times \mathbb C^s}{ \mathcal O_{\mathbb{K}}}=\mathbb R_+^{r}\times \mathbb T^{r+2s}, \end{align*} $$
and that the action of U on
$\mathbb H^r\times \mathbb C^s$
induces an action on
$\mathbb R_+^{r}\times \mathbb T^{r+2s}$
such that, for every
$x\in \mathbb {\mathbb R}^r_+$
and
$u\in U$
, the induced map
$$ \begin{align*}u\colon (x,\mathbb T^{r+2s})\mapsto (\sigma_1(u)x_1,\ldots, \sigma_r(u)x_r,\mathbb T^{r+2s}) \end{align*} $$
is a diffeomorphism. Hence,
$$ \begin{align*}M=\frac{\mathbb R_+^{r}\times \mathbb T^{r+2s}}{U} \end{align*} $$
inherits the structure of a
$\mathbb T^{r+2s}$
-bundle over
$\mathbb T^r$
. We denote by
$\pi $
and F the projections
$$ \begin{align*}\pi\colon \mathbb H^r\times \mathbb C^s\to M,\quad F\colon M\to\mathbb T^r. \end{align*} $$
From the viewpoint of Lie groups, the universal covering of an Oeljeklaus–Toma manifold M has a natural structure of solvable Lie group G and the complex structure on M lifts to a left-invariant complex structure [Reference Kasuya13]. Therefore, Oeljeklaus–Toma manifolds can be seen as compact solvmanifolds with a left-invariant complex structure. The solvable structure on the universal covering of M can be described in terms of the existence of a left-invariant
$(1,0)$
-coframe
$\{\omega ^1,\dots ,\omega ^r,\gamma ^1,\dots ,\gamma ^s\}$
such that
$$ \begin{align} \begin{cases} d\omega^k=\frac{\sqrt{-1}}{2}\omega^k\wedge\bar \omega^k,\quad & k=1,\dots,r,\\ d\gamma^i=\sum_{k=1}^r\lambda_{ki}\,\omega^k\wedge \gamma^{i}-\sum_{k=1}^r\,\lambda_{ki}\bar{\omega}^{k}\wedge\gamma^i,\quad & i=1,\dots, s, \end{cases} \end{align} $$
where
$$ \begin{align*}\lambda_{ki}=\frac{\sqrt{-1}}{4}b_{ki}-\frac{1}{2}c_{ki} \end{align*} $$
and
$b_{ki},c_{ki}\in {\mathbb R}$
depend on the embeddings
$\sigma _j$
as
$$ \begin{align} \sigma_{r+i}(u)=\left(\prod_{k=1}^r(\sigma_k(u))^{\frac{b_{ki}}{2}}\right)e^{\sqrt{-1}\sum_{k=1}^r c_{ki} \log\sigma_k(u) }, \end{align} $$
for any
$u\in U$
,
$k=1,\dots , r$
and
$i=1,\dots , s$
. Since
$U\subseteq \mathcal {O}_{\mathbb {K}}^*$
, it is easy to see that
$$ \begin{align*}l(U)\subseteq\left\{x\in {\mathbb R}^{r+s}\quad \middle|\quad \sum_{i=1}^{r+s}x_i=0\right\}. \end{align*} $$
This fact together with (2) implies that, for every
$u\in U$
,
$$ \begin{align*}\sum_{i=1}^r\log\sigma_i(u)\left(1+\sum_{k=1}^sb_{ik}\right)=0\, , \end{align*} $$
which, since
$\mathrm {pr}_{\mathbb {R}^r}\circ l(U)$
is a lattice of rank r in
$\mathbb {R}^r$
, is equivalent to
$$ \begin{align} \sum_{k=1}^sb_{ik}=-1,\quad \mbox{for all } i=1,\ldots, r. \end{align} $$
The dual frame
$\{Z_1,\dots , Z_r,W_1,\dots ,W_s\}$
to
$\{\omega ^1,\dots ,\omega ^r,\gamma ^1,\dots ,\gamma ^s\}$
satisfies the following structure equations:
$$ \begin{align*}[Z_k,\bar Z_k]=\,-\frac{\sqrt{-1}}{2}(Z_k+\bar Z_k),\quad [Z_k,W_i]=-\lambda_{ki}W_i,\quad [ Z_k,\bar W_i]=\bar \lambda_{ki} \bar W_i, \end{align*} $$
for
$k=1,\dots , r$
and
$i=1,\dots , s$
. Consequently, the Lie algebra
$\mathfrak {g}$
of the universal covering of M splits as vector space as
$$ \begin{align*}\mathfrak{g}=\mathfrak{h}\oplus \mathfrak{I}, \end{align*} $$
where
$\mathfrak {I}$
is an abelian ideal and
$\mathfrak {h}$
is a subalgebra isomorphic to
$\underbrace {\mathfrak {f}\oplus \dots \oplus \mathfrak {f}}_{r\mbox {-times}}$
, where
$\mathfrak f$
is the filiform Lie algebra
$\mathfrak {f}=\langle e_1,e_2\rangle $
,
$[e_1,e_2]=-\tfrac 12 e_1$
. The complex structure J induced on
$\mathfrak g$
preserves both
$\mathfrak h$
and
$\mathfrak I$
, and its restriction
$J_{\mathfrak h}$
on
$\mathfrak h$
satisfies
$$ \begin{align*}J_{\mathfrak{h}}=\underbrace{J_{\mathfrak f}\oplus \dots \oplus J_{\mathfrak f}}_{r\mbox{-times}}\, , \end{align*} $$
where
$J_{\mathfrak f}$
is the complex structure on
$\mathfrak f$
defined by
$J_{\mathfrak f}(e_1)= e_2$
. Moreover,
$$ \begin{align*}[\mathfrak h^{1,0},\mathfrak I^{0,1}]\subseteq \mathfrak I^{0,1}. \end{align*} $$
3 Convergence in the Gromov–Hausdorff sense
We briefly recall Gromov–Hausdorff convergence of metric spaces. The Gromov–Hausdorff distance between two metric spaces
$(X,d_X)$
,
$(Y,d_Y)$
is the infimum of all positive
$\epsilon $
for which there exist two functions
$F\colon X\to Y$
,
$G\colon Y\to X$
, not necessarily continuous, satisfying the following four properties:
$$ \begin{align*}\begin{array}{cc} |d_X(x_1,x_2)-d_Y(F(x_1),F(x_2))|\leq \epsilon, & d_X(x,G(F(x)))\leq \epsilon,\\ |d_Y(y_1,y_2)-d_X(G(y_1),G(y_2))|\leq \epsilon, & d_Y(y,F(G(y)))\leq \epsilon, \end{array} \end{align*} $$
for all
$x,x_1,x_2\in X$
and
$y,y_1,y_2\in Y$
. If
$\{d_t\}_{t\in [0,\infty )}$
is a one-parameter family of distances on X,
$(X,d_t)$
converges to
$(Y,d_Y)$
in the Gromov–Hausdorff sense if the Gromov–Hausdorff distance between
$(X,d_t)$
and
$(Y,d)$
tends to
$0$
as
$t\to \infty $
.
Let
$\{\omega _t\}_{t\in [0,\infty )}$
be a smooth curve of Hermitian metrics on an Oeljeklaus–Toma manifold, and let
$d_t$
be the induced distance on M. For a smooth curve
$\gamma $
on M, let
$L_t(\gamma )$
be the length of
$\gamma $
with respect to
$\omega _t$
. We further denote by
$\mathcal H$
the foliation induced by
$\mathfrak h$
on M.
Proposition 3.1 Let
$\{\omega _t\}_{t\in [0,\infty )}$
be a smooth curve of Hermitian metrics on an Oeljeklaus–Toma manifold such that
$$ \begin{align*}\lim_{t\to \infty}\omega_t=\omega_{\infty} \end{align*} $$
pointwise. Assume that there exist
$T\in (0,\infty )$
and
$C>0$
such that:
-
1.
$L_t(\gamma )\leq C L_0(\gamma ),$
for every smooth curve
$\gamma $
in M. -
2.
$L_t(\gamma )\leq (C/\sqrt {t})L_0(\gamma )$
, for every smooth curve
$\gamma $
in M such that
$\dot \gamma \in \ker \omega _\infty $
.
Assume further that:
-
3. For every
$\epsilon ,\ell>0$
, there exists
$T>0$
such that
$|L_t(\gamma )-L_{\infty }(\gamma )|<\epsilon $
, for every
$t>T$
and every curve
$\gamma $
in M tangent to
$\mathcal H$
and such that
$L_\infty (\gamma )<\ell $
.
Then
$(M,d_t)$
converges in the Gromov–Hausdorff sense to
$(\mathbb T^{r},d)$
, where d is the distance induced by
$\omega _{\infty }$
onto
$\mathbb T^{r}$
.
Proof We follow the approach in [Reference Tosatti and Weinkove28, Section 5] and in [Reference Zheng32, Proof of Theorem 1.1]. Let M be an Oeljeklaus–Toma manifold. Consider the structure of M as
$\mathbb T^{r+2s}$
-bundle over a
$\mathbb T^{r}$
. Let
$F\colon M\to \mathbb T^{r}$
be the projection onto the base, and let
$G\colon \mathbb T^{r}\to M$
be an arbitrary map such that
$F\circ G=\mathrm {Id}_{\mathbb T^{r}}$
. We show that, for every
$\epsilon>0$
, there exists
$T>0$
such that
$$ \begin{align} && |d_t(p,q)-d(F(p),F(q))|\leq \epsilon, \end{align} $$
$$ \begin{align} && |d(a,b)-d_t(G(a),G(b))|\leq \epsilon, \end{align} $$
$$ \begin{align} && d_t(p,G(F(p)))\leq \epsilon, \end{align} $$
$$ \begin{align} && d(a,F(G(a)))\leq \epsilon, \end{align} $$
for every
$t\geq T$
,
$p,q\in M$
,
$a,b\in \mathbb T^r$
, which implies the statement.
Note that (7) is trivial since
$$ \begin{align*}d(a,F(G(a)))=0, \end{align*} $$
for every
$a\in \mathbb T^r$
.
Then we show that (6) is satisfied. Let
$p,q\in M$
be two points in the same fiber over
$\mathbb T^r$
. Assume that
$p=\pi (z,w)$
. We denote with
$\mathcal {L}_{(z,w)}$
the leaf of the foliation
$\ker \omega _{\infty }$
on the universal covering of M passing through
$(z,w)$
. We easily see that, for all
$(z,w)\in \mathbb H^r\times \mathbb C^s$
,
$\mathcal {L}_{(z,w)}=\{z\}\times \mathbb C^s$
. In view of [Reference Verbitsky30, Section 2], for every
$z\in \mathbb H^r$
,
$\pi (\{z\}\times \mathbb C^s)$
is the leaf of the foliation
$\ker \omega _{\infty }$
on M passing through p and it is dense in the fiber
$F^{-1}(F(p))$
. Let
$B_{R}$
be the standard ball in
$\mathbb C^s$
about the origin having radius R. We can choose R so that every point in
$F^{-1}(F(p))$
has distance with respect to
$d_0$
less than
$\epsilon /2C$
to
$\pi (\{z\}\times \bar B_{R})$
. On the other hand, given two points in
$\pi (\{z\}\times \bar B_{R})$
, they can be joined with a curve
$\gamma $
in
$F^{-1}(F(p))$
which is tangent to
$\ker \omega _\infty $
. Hence, for any such curve, condition 2 implies
$$ \begin{align*}L_t(\gamma)\leq \frac{C'}{\sqrt t}, \end{align*} $$
for a uniform constant
$C'$
depending only on R. Let
$p_0=\pi (z,0)$
, let
$\gamma _1$
be a curve in
$F^{-1}(F(p))$
connecting p with
$p_0$
tangent to
$\ker \omega _\infty $
, and let
$\gamma _2$
be a curve connecting
$p_0$
with q having minimal length with respect to
$d_0$
. Hence, by using condition 1, for t sufficiently large, we have
$$ \begin{align*}d_t(p,q)\leq L_{t}(\gamma_1)+L_t(\gamma_2)\leq \frac{C'}{\sqrt{t}}+CL_{0}(\gamma_2)\leq \frac{C'}{\sqrt{t}}+\frac{\epsilon}{2}\leq \epsilon, \end{align*} $$
i.e.,
$$ \begin{align*}d_t(p,q)\leq \epsilon, \end{align*} $$
and (6) follows.
Next, we show (4) and (5). First of all, we denote with g the Riemannian metric on
$\mathbb {T}^r$
induced by
$\omega _{\infty }$
, for an explicit expression of g (see [Reference Zheng32, Section 2]), and we observe that
$$ \begin{align} L_{g}(F(\gamma))\leq L_{\infty}(\gamma),\mbox{ for every curve} \gamma\mbox{ in }M, \end{align} $$
and the equality holds if and only if
$$ \begin{align*}\dot \gamma\in\mathcal{Y}=\mathrm{span}_{{\mathbb C}}\left\{ \frac{1}{2\sqrt{-1}}\left(Z_i-\bar Z_i\right)\quad \middle | \quad i=1,\ldots, r \right\}. \end{align*} $$
Let
$p,q\in M$
. We can find a curve
$\gamma $
in M connecting p with a point
$\tilde {q}$
in the
$\mathbb T^{r+2s}$
-fiber containing q which is tangent to
$\mathcal Y$
and such that
$F(\gamma )$
is a minimal geodesic on
$(\mathbb T^r,g)$
(see, for instance, [Reference Tosatti and Weinkove28, Proof of Theorem 5.1] or [Reference Zheng32, Proof of Theorem 1.1]). By applying condition 3, we have
$$ \begin{align*}&d_t(p,q)\leq d_t(p,\tilde q)+d_t(\tilde q,q)\leq d_t(p,\tilde q)+\epsilon\leq L_{t}( \gamma)+\epsilon\leq L_{\infty}(\gamma) +2\epsilon\\&\quad= L_g(F(\gamma))+ 2\epsilon=d(F(p),F(q))+2\epsilon, \end{align*} $$
for t big enough, i.e.,
$$ \begin{align} d_t(p,q)-d(F(p),F(q))\leq \,2\epsilon, \end{align} $$
for t sufficiently large.
Next, using again (8), we obtain, for
$p,q\in M$
,
$$ \begin{align*}d(F(p),F(q))\leq L_g(F(\gamma))\le L_{\infty}(\gamma)\leq L_{t}(\gamma)+\epsilon=d_t(p,q)+\epsilon, \end{align*} $$
for t big enough, where
$\gamma $
is a curve which realizes the distance
$d_t(p, q)$
. Hence, we obtain
$$ \begin{align} d(F(p),F(q))-d_t(p,q)\le \epsilon. \end{align} $$
By substituting
$p=G(a)$
and
$q=G(b)$
in (9) and (10), we infer
$$ \begin{align*}-\epsilon\leq d_t(G(a),G(b))-d(a,b)\leq 2\epsilon, \end{align*} $$
4 The left-invariant Chern–Ricci flow on Oeljeklaus–Toma manifolds
Given a Hermitian manifold
$(M,\omega )$
, the Chern connection of
$\omega $
is the unique connection
$\nabla $
on
$(M,\omega )$
preserving both
$\omega $
and the complex structure such that the
$(1,1)$
-component of its torsion tensor is vanishing. The Chern–Ricci form of
$\omega $
is the real closed
$(1,1)$
-form
$$ \begin{align*}\rho_C(X,Y):=\sqrt{-1}\sum_{i=1}^n R_C(X,Y,X_i,\bar X_i), \end{align*} $$
where
$R_C$
is the curvature tensor of
$\nabla $
and
$\{X_i\}$
is a unitary frame with respect to
$\omega $
. The Chern–Ricci flow is then defined as the geometric flow
$$ \begin{align*}\partial_t\omega_t=-\rho_C(\omega_t),\quad \omega_{|t=0}=\omega. \end{align*} $$
In this section, we prove the following Proposition.
Proposition 4.1 Let
$\omega $
be a left-invariant Hermitian metric on an Oeljeklaus–Toma manifold M. Then
$\omega $
lifts to an expanding algebraic soliton for the Chern–Ricci flow on the universal covering of M if and only if it takes the following expression with respect to the coframe
$\{\omega ^1,\dots ,\omega ^r,\gamma ^1,\dots ,\gamma ^s\}$
satisfying (
1
):
$$ \begin{align} \omega=\sqrt{-1}\left(A\sum_{i=1}^r\omega^i\wedge\bar \omega^i+\sum_{i,j=1}^sg_{r+i\overline{r+j}}\gamma^i\wedge\bar\gamma^j\right). \end{align} $$
Moreover, the Chern–Ricci flow starting from
$\omega $
has a long-time solution
$\{\omega _t\}$
such that
$(M, \frac {\omega _t}{1+t})$
converges as
$t\to \infty $
in the Gromov–Hausdorff sense to
$(\mathbb T^r,d)$
, where d is the distance induced by
$\omega _{\infty }$
onto
$\mathbb T^{r}$
. Finally,
$(\mathbb H^r\times {\mathbb C}^s, \frac {\omega _t}{1+t})$
converges in the Cheeger–Gromov sense to
$(\mathbb H^r\times {\mathbb C}^s, \tilde {\omega }_{\infty })$
, where
$\tilde {\omega }_{\infty }$
is an algebraic soliton.
The proof of Proposition 4.1 is based on the following theorem of Lauret.
Theorem 4.2 (Lauret [Reference Lauret15])
Let
$(G,J)$
be a Lie group with a left-invariant complex structure. Then the Chern–Ricci form of a left-invariant Hermitian metric
$\omega $
on
$(G,J)$
does not depend on the Hermitian metric. Moreover, if
$P\ne 0 $
is the endomorphism associated with
$\rho _C$
with respect to
$\omega $
, then the following are equivalent:
-
(1)
$\omega $
is an algebraic soliton of the Chern–Ricci flow. -
(2)
$P=cI + D$
, for some
$D\in \mathrm {Der}(\mathfrak {g}).$
-
(3) The eigenvalues of P are either
$0$
or c, for some
$c\in {\mathbb R}$
with
$c\neq 0$
,
$\ker P$
is an abelian ideal of the Lie algebra of G, and
$(\ker P)^\perp $
is a subalgebra.
Proof of Proposition 4.1
Let M be an Oeljeklaus–Toma manifold. Since the Chern–Ricci form does not depend on the choice of the left-invariant Hermitian metric, it is enough to compute
$\rho _C$
for the “canonical metric”
$$ \begin{align} \omega=\sqrt{-1}\left(\sum_{i=1}^r\omega^i\wedge\bar\omega^i+\sum_{j=1}^s\gamma^j\wedge\bar \gamma^j\right). \end{align} $$
We recall that the Chern–Ricci form of a left-invariant Hermitian metric
$\omega =\sqrt {-1}\sum _{a=1}^n \alpha ^a\wedge \bar \alpha ^a $
on a Lie group
$G^{2n}$
with a left-invariant complex structure takes the following algebraic expression:
$$ \begin{align} \rho_C(X,Y) = -\sum_{a=1}^n (\omega([[X,Y]^{0,1},X_a],\bar X_a) + \omega ([[X,Y]^{1,0},\bar X_a],X_a ) ) \, , \end{align} $$
for every left-invariant vector fields
$X,Y$
on G, where
$\{\alpha ^i\}$
is a left-invariant unitary
$(1,0)$
-coframe with dual frame
$\{X_a\}$
(see, e.g., [Reference Vezzoni31]). By applying (13) to the canonical metric (12), we have
$$ \begin{align*} \rho_C(X,Y) &=- \sum_{a=1}^r \{ \omega([[X,Y]^{0,1},Z_a],\bar Z_a) + \omega([[X,Y]^{1,0},\bar Z_a],Z_a ) \}\\ &\quad- \sum_{b=1}^s \{ \omega([[X,Y]^{0,1},W_b],\bar W_b) +\omega([[X,Y]^{1,0},\bar W_b],W_b ) \}. \end{align*} $$
Clearly,
$$ \begin{align*}\rho_C(Z_i,\bar Z_j)=0\, , \quad \mbox{ for all } i\ne j, \quad \rho_{C}(W_i,\bar W_j)=0, \quad \mbox{for every }i,j=1,\ldots, s. \end{align*} $$
Moreover, since
$\mathfrak {J}$
is an abelian ideal and
$\omega $
makes
$\mathfrak {J}$
and
$\mathfrak {h}$
orthogonal, we have
$$ \begin{align*}\rho_C(Z_i,\bar W_j)=0, \quad \mbox{for all } i=1,\ldots, r,\quad j=1,\ldots, s. \end{align*} $$
Moreover, we have
$$ \begin{align*}\omega([[Z_i,\bar Z_i]^{0,1},Z_a],\bar Z_a)= \frac{\sqrt{-1}}{4}\delta_{ia}\, ,\quad \omega([[Z_i,\bar Z_i]^{1,0},\bar Z_a], Z_a)=\frac{\sqrt{-1}}{4}\delta_{ia} \end{align*} $$
and
$$ \begin{align*}\omega([[Z_i,\bar Z_i]^{0,1},W_b],\bar W_b)= \frac12\lambda_{ib}, \quad \omega([[Z_i,\bar Z_i]^{1,0},\bar W_b],W_b )= -\frac12\bar\lambda_{ib}, \end{align*} $$
which imply
$$ \begin{align*}\rho_C(Z_i,\bar Z_i)=-\sqrt{-1}\left(\frac12+\sum_{b=1}^s\Im\mathfrak{m}(\lambda_{ib})\right)=-\frac{\sqrt{-1}}{4}, \end{align*} $$
and, consequently,
$$ \begin{align*}\rho_C=-\omega_\infty, \end{align*} $$
where
$\omega _{\infty }$
is the degenerate metric induced on M by the Poincaré metric on
$\mathbb {H}^r$
, namely,
$$ \begin{align*}\omega_{\infty}=\frac{\sqrt{-1}}{4}\sum_{i=1}^r\omega^i\wedge\bar\omega^i. \end{align*} $$
In general, we have that
$$ \begin{align*}P_i^j=(\rho_C)_{i\bar k }g^{\bar k j }=\begin{cases}-\frac14g^{\bar i j }, &\quad \mbox{if }i\in\{1,\ldots, r\},\\ 0, &\quad \mbox{otherwise}. \end{cases} \end{align*} $$
Then part (3) of Theorem 4.2 readily implies that any left-invariant Hermitian metrics of the form (11) lifts to an expanding algebraic soliton on the universal covering of M with cosmological constant
$c=\frac {1}{4A}$
. Conversely, let
$\omega $
be an algebraic soliton for the Chern–Ricci flow. Then, thanks to part (2) of Theorem 4.2, we have that
$$ \begin{align*}P-cI\in\mathrm{Der}(\mathfrak{g}). \end{align*} $$
On the other hand, we can easily see that, if
$ D\in \mathrm {Der}(\mathfrak {g})$
, then
$\mathfrak h \subseteq \ker D$
(see the proof of Corollary 5.4 for the details). This readily implies that
$$ \begin{align*}-\frac14g^{i\bar i }=-\frac14g^{\bar j j}=c, \quad \mbox{ for all }i,j=1,\ldots, r, \quad g^{\bar i j }=0, \quad \mbox{for all }i \in\{1,\ldots, r\},\, j\ne i , \end{align*} $$
from which the claim follows.
Moreover, the Chern–Ricci flow evolves an arbitrary left-invariant Hermitian metric
$\omega $
as
$\omega _t=\omega +t\omega _\infty $
and
$\frac {\omega _t}{1+t}\to \omega _\infty $
as
$t\to \infty $
. In order to obtain the claim regarding the Gromov–Hausdorff convergence, we show that
$\frac {\omega _t}{1+t}$
satisfies conditions 1–3 in Proposition 3.1. Here, we denote by
$|\cdot |_t$
the norm induced by
$\omega _t$
.
Condition 2 is trivially satisfied since
$\omega _{t|\mathfrak I\oplus \mathfrak I}=\omega _0$
, for every
$t\geq 0$
, and
$$ \begin{align*}L_t(\gamma)=\frac{1}{\sqrt{1+t}}L_{0}(\gamma), \end{align*} $$
for every curve
$\gamma $
in M tangent to
$\ker \omega _\infty $
.
On the other hand, for a vector
$v\in \mathfrak h$
, we have
$$ \begin{align*}\frac{1}{\sqrt{1+t}}|v|_t\leq C|v|_{0}, \end{align*} $$
for a constant
$C>0$
independent on v. This, together with condition 2, guarantees condition 1.
In order to prove condition 3, let
$\epsilon , \ell>0$
and
$T>0$
be such that
$$ \begin{align*}\left\vert \frac{|v|_t}{\sqrt{1+t}}-|v|_\infty\right\vert\leq \frac{\epsilon}{\ell}, \end{align*} $$
for every
$v\in \mathfrak h$
and
$t\geq T$
. Let
$\gamma $
be a curve in M tangent to
$\mathcal H$
which is parametrized by arclength with respect to
$\omega _\infty $
and such that
$L_{\infty }(\gamma )<\ell $
. Then
$$ \begin{align*}|L_{t}(\gamma)-L_{\infty}(\gamma)|\leq \int_{0}^{b}\left\vert\frac{1}{\sqrt{1+t}}|\dot \gamma |_t-|\dot \gamma|_\infty\right\vert da\leq \frac{\epsilon}{\ell}b\leq \epsilon, \end{align*} $$
since
$b\leq \ell $
.
For the last statement, we identify
$\omega _t$
with its pullback onto
$\mathbb H^r\times \mathbb C^s$
and we fix as base point the identity element of
$\mathbb H^r\times \mathbb C^s$
. First, we observe that the endomorphism D represented with respect to the frame
$\{Z_1,\ldots , Z_r, W_1,\ldots , W_s\}$
by the following matrix
$$ \begin{align*}\begin{pmatrix} 0&0\\0& \mathrm{I}_{\mathfrak J} \end{pmatrix} \end{align*} $$
is a derivation of
$\mathfrak {g}$
. Moreover, we can construct
$$ \begin{align*}\exp(s(t)D)=\begin{pmatrix}\mathrm{I}_{\mathfrak{h}}& 0 \\ 0 & e^{s(t)}\mathrm{ I}_{\mathfrak{J}}\end{pmatrix}\in \mathrm{Aut}(\mathfrak{g}, J), \quad \mbox{for every } t\ge 0, \end{align*} $$
where
$s(t)=\log (\sqrt {1+t})$
and define the one-parameter family
$\{\varphi _t\}\subseteq \mathrm {Aut}(\mathbb H^r\times {\mathbb C} ^s, J )$
such that
$$ \begin{align*}d\varphi_t=\exp(s(t)D), \quad \mbox{for every } t\ge 0. \end{align*} $$
Trivially, we see that
$$ \begin{align*}\begin{aligned} \varphi_t^*\frac{\omega_t}{1+t}(Z_i,\bar Z_j)=&\, \sqrt{-1}\frac{1}{1+t}\left(g_{i\bar j }+\frac t4\delta_{ij}\right )\to \frac{\sqrt{-1}}{4}\delta_{ij} \quad \mbox{as }t\to\infty,\\ \varphi_t^*\frac{\omega_t}{1+t}(Z_i,\bar W_j)=&\,\sqrt{-1}\frac{e^{s(t)}}{1+t}g_{i\overline{r+j}}\to 0 \quad \mbox{as }t\to\infty,\\ \varphi_t^*\frac{\omega_t}{1+t}(W_i,\bar W_j)=&\,\sqrt{-1}\frac{e^{2s(t)}}{1+t}g_{r+i\overline{r+j}} \to \sqrt{-1}g_{r+i\overline{r+j}} \quad \mbox{as }t\to\infty.\\ \end{aligned} \end{align*} $$
These facts guarantee that
$$ \begin{align*}\varphi_t^*\frac{\omega_t}{1+t}\to \omega_{\infty}+\omega_{\mathfrak{J}\oplus \mathfrak{J}} \quad \mbox{as }t\to\infty\,; \end{align*} $$
hence, the assertion follows.
5 Proof of the main result
In this section, we prove Theorem 1.1.
The existence of pluriclosed metrics on Oeljeklaus–Toma manifolds was studied in [Reference Angella, Dubickas, Otiman and Stelzig1, Reference Fino, Kasuya and Vezzoni8, Reference Otiman18]. In particular, from [Reference Angella, Dubickas, Otiman and Stelzig1] it follows the following result.
Theorem 5.1 ([Reference Angella, Dubickas, Otiman and Stelzig1, Corollary 3])
An Oeljeklaus–Toma manifold of type
$(r,s)$
admits a pluriclosed metric if and only if
$r=s$
and
$$ \begin{align} \sigma_j(u)|\sigma_{r+j}(u)|^2=1,\quad \mbox{ for every } j=1,\dots,s \mbox{ and }u\in U. \end{align} $$
Condition (14) in the previous theorem can be rewritten in terms of the structure constants appearing in (1). Indeed, (1) together with (14) forces
$b_{ki}\in \{0,-1\}$
and
$b_{ki}b_{li}=0$
, for every
$i,k,l=1,\dots , s$
with
$k\neq l$
. In particular, using (3), for every fixed index
$k\in \{1,\ldots , s\}$
, there exists a unique
$i_k\in \{1,\ldots , s\}$
such that
$$ \begin{align*}b_{ki_k}=-1,\quad b_{ki}=0, \end{align*} $$
for all
$i\ne i_k$
and, if
$k\ne l ,$
then
$i_k\ne i_l$
. Hence, up to a reorder of the
$\gamma _j$
’s, we may and do assume, without loss of generality,
$i_k=k$
, for every
$k\in \{1,\dots ,s\}$
, i.e.
$$ \begin{align} \lambda_{ki}=\begin{cases}-\frac12 c_{ki}, \quad &\mbox {if } i\ne k, \\ -\frac12 c_{kk}-\frac{\sqrt{-1}}{4},\quad & \mbox{if } i=k.\end{cases} \end{align} $$
Proposition 5.2 (Characterization of left-invariant pluriclosed metrics on Oeljeklaus–Toma manifolds).
A left-invariant metric
$\omega $
on an Oeljeklaus–Toma manifold admitting pluriclosed metrics is pluriclosed if and only if it takes the following expression with respect to a coframe
$\{\omega ^1,\dots ,\omega ^s,\gamma ^1,\dots ,\gamma ^s\}$
satisfying (
1
) and (
15
):
$$ \begin{align} \omega=\sqrt{-1}\sum_{i=1}^sA_i\omega^i\wedge \bar \omega^i+ B_i\gamma^i\wedge \bar \gamma^i+ \sqrt{-1}\sum_{r=1}^{k}\left( C_{r}\omega^{p_r}\wedge \bar \gamma^{p_r}+\bar C_{r}\gamma^{p_r}\wedge\bar \omega^{p_r} \right) \end{align} $$
for some
$A_1,\dots ,A_s,B_1,\dots ,B_s\in \mathbb R_+$
,
$C_1,\dots ,C_k\in \mathbb C$
, where
$\{p_1,\dots ,p_k\}\subseteq \{1,\dots ,s\}$
are such that
$$ \begin{align*}\lambda_{jp_i}=0\, ,\mbox{ for all }j\neq p_i\, , \mbox{ for all } i=1,\dots,k. \end{align*} $$
Proof We assume
$s>1$
since the case
$s=1$
is trivial. Let
$$\begin{align*}\omega=\sqrt{-1}\sum_{p,q=1}^sA_{p\bar q}\omega^p\wedge\bar{\omega}^q+B_{p\bar q}\gamma^p\wedge\bar{\gamma}^q+C_{p\bar q}\omega^p\wedge\bar{\gamma}^q+\bar C_{ p\bar q} \gamma^q\wedge \bar{\omega}^p\end{align*}$$
be an arbitrary real left-invariant
$(1,1)$
-form on M, with
$A_{p\bar p}, B_{p\bar p}\in {\mathbb R}$
, for every
${p=1,\ldots , s}$
,
$A_{p\bar q}, B_{p\bar q}\in {\mathbb C}$
, for all
$p,q=1,\ldots , s$
with
$ p\ne q$
, and
$ C_{p\bar q}\in {\mathbb C}$
, for every
${p,q=1,\ldots , s}$
.
From the structure equations (1), it easily follows
$$ \begin{align} \begin{cases} & \partial\bar\partial(\omega^p\wedge\bar\omega^q)\in \langle \omega^{p}\wedge \omega^q\wedge \bar \omega^p\wedge \bar\omega ^q \rangle, \\ & \partial\bar\partial(\omega^p\wedge\bar\gamma^q)\in \langle \omega^{i}\wedge \omega^j\wedge \bar \omega^l\wedge \bar\gamma^m\rangle, \\ & \partial\bar\partial(\gamma^p\wedge\bar\gamma^q)\in \langle \omega^{i}\wedge\bar \omega^j\wedge\gamma^l\wedge \bar\gamma^m\rangle, \\ \end{cases} \end{align} $$
and that
$\omega $
is pluriclosed if and only if the following three conditions are satisfied:
$$ \begin{align} && \sum_{p,q=1}^sA_{p\bar q}\partial\bar{\partial}(\omega^p\wedge\bar{\omega}^q)=0 , \end{align} $$
$$ \begin{align} && \sum_{p,q=1}^sB_{p\bar q}\partial\bar{\partial}(\gamma^p\wedge\bar{\gamma}^q)=0 , \end{align} $$
$$ \begin{align} && \sum_{p,q=1}^sC_{p\bar q}\partial\bar{\partial}(\omega^p\wedge\bar{\gamma}^q)=0 . \end{align} $$
The first relation in (17) yields that (18) is satisfied if and only if
$$ \begin{align*}A_{p\bar q}=0, \mbox{ for all }p\neq q. \end{align*} $$
Next, we focus on (19). We have
$$ \begin{align*}\begin{aligned} \partial\bar\partial(\gamma^p\wedge\bar{\gamma}^q)=& \partial\left(-\sum_{\delta=1}^s\lambda_{\delta p}\bar{\omega}^{\delta}\wedge\gamma^p\wedge\bar{\gamma}^q-\gamma^p\wedge\sum_{\delta=1}^s\bar\lambda_{\delta q}\bar{\omega}^{\delta}\wedge\bar{\gamma}^q\right)\, \end{aligned} \end{align*} $$
and
$$ \begin{align*}\begin{aligned} \partial\bar\partial(\gamma^p\wedge\bar{\gamma}^q)=&\, \sum_{\delta=1}^s(\bar\lambda_{\delta q}-\lambda_{\delta p})\left(\partial\bar{\omega}^{\delta}\wedge\gamma^p\wedge\bar{\gamma}^q-\bar{\omega}^{\delta}\wedge\partial\gamma^p\wedge\bar{\gamma}^q+\bar{\omega}^{\delta}\wedge \gamma^p\wedge \partial\bar{\gamma}^q\right), \end{aligned} \end{align*} $$
which implies that
$$ \begin{align*}\begin{aligned} \partial\bar\partial(\gamma^p\wedge\bar{\gamma}^q)=&\, \sum_{\delta=1}^s\frac{\sqrt{-1}}{2}(\bar\lambda_{\delta q}-\lambda_{\delta p})\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge\gamma^p\wedge\bar{\gamma}^q-\sum_{\delta=1}^s(\bar\lambda_{\delta q}-\lambda_{\delta p})\bar{\omega}^{\delta}\wedge\left(\sum_{a=1}^s\lambda_{ap}\omega^a\wedge\gamma^p\right)\wedge\bar{\gamma}^q\\ &\,+\sum_{\delta=1}^s(\bar\lambda_{\delta q}-\lambda_{\delta p})\bar{\omega}^{\delta}\wedge\gamma^{p}\wedge\left(-\sum_{a=1}^s\bar\lambda_{aq}\omega^a\wedge\bar{\gamma}^q\right)\\ =&\sum_{\delta=1}^s\frac{\sqrt{-1}}{2}(\bar\lambda_{\delta q}-\lambda_{\delta p})\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge{\gamma^p}\wedge\bar{\gamma}^q+\sum_{\delta, a}(\lambda_{ap}-\bar\lambda_{aq})(\bar\lambda_{\delta q}-\lambda_{\delta p})\omega^a\wedge\bar{\omega}^{\delta}\kern1.2pt{\wedge}\kern1.2pt\gamma^p\wedge\bar{\gamma}^q. \end{aligned} \end{align*} $$
Finally, we get
$$ \begin{align*} \partial\bar\partial(\gamma^p\wedge\bar{\gamma}^q)=\sum_{\delta=1}^s(\bar\lambda_{\delta q}-\lambda_{\delta p})\left(\frac{\sqrt{-1}}{2}+\lambda_{\delta p}-\bar\lambda_{\delta q}\right)\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge{\gamma^p}\wedge\bar{\gamma}^q\\+\sum_{\delta\ne a}(\lambda_{ap} -\bar\lambda_{aq})(\bar\lambda_{\delta q}-\lambda_{\delta p})\omega^a\wedge\bar{\omega}^{\delta}\wedge\gamma^p\wedge\bar{\gamma}^q \end{align*} $$
and that condition (19) is equivalent to
$$ \begin{align*} B_{p\bar q}\left(\sum_{\delta=1}^s(\bar\lambda_{\delta q}-\lambda_{\delta p})\left(\frac{\sqrt{-1}}{2} +\lambda_{\delta p}-\bar\lambda_{\delta q}\right)\omega^{\delta}\wedge\bar{\omega}^{\delta} +\sum_{\delta\ne a}(\lambda_{ap}-\bar\lambda_{aq})(\bar\lambda_{\delta q}-\lambda_{\delta p})\omega^a\wedge\bar{\omega}^{\delta}\right)=0, \end{align*} $$
for every
$p,q=1,\dots , s.$
By using our conditions on the
$b_{ki}$
’s, it is easy to show that the quantity
$$ \begin{align*}\sum_{\delta=1}^s(\bar\lambda_{\delta q}-\lambda_{\delta p})\left(\frac{\sqrt{-1}}{2} +\lambda_{\delta p}-\bar\lambda_{\delta q}\right)\omega^{\delta}\wedge\bar{\omega}^{\delta} +\sum_{\delta\ne a}(\lambda_{ap}-\bar\lambda_{aq})(\bar\lambda_{\delta q}-\lambda_{\delta p})\omega^a\wedge\bar{\omega}^{\delta} \end{align*} $$
is vanishing for
$p=q$
and, consequently, there are no restrictions on the
$B_{q\bar q}$
’s. Now, we observe that the real part of
$$ \begin{align*}(\bar\lambda_{p q}-\lambda_{p p})\left(\frac{\sqrt{-1}}{2} +\lambda_{p p}-\bar\lambda_{p q}\right) \end{align*} $$
is different from
$0$
, for every
$p,q$
with
$p\neq q$
, which forces
$B_{p\bar q}=0$
, for
$p\neq q$
. Indeed, we have
$$ \begin{align*} &\bar \lambda_{\delta q}-\lambda_{\delta p}=\frac12 (c_{\delta p}-c_{\delta q})-\frac{\sqrt{-1}}{4}(b_{\delta p}+b_{\delta q}), \\ & \frac{\sqrt{-1}}{2}+\lambda_{\delta p}-\bar\lambda_{\delta q}=-\frac12(c_{\delta p}-c_{\delta q})+\frac{\sqrt{-1}}{2}\left(1+\frac{b_{\delta p}+b_{\delta q}}{2}\right), \end{align*} $$
which implies that
$$ \begin{align} &\Re\mathfrak e\,\left((\bar \lambda_{\delta q}-\lambda_{\delta p})\left(\frac{\sqrt{-1}}{2}+\lambda_{\delta q}-\bar \lambda_{\delta p}\right)\right)\\&\quad=-\frac{(c_{\delta p}-c_{\delta q})^2}{4}+\frac14\left(\frac{b_{\delta p}+b_{\delta q}}{2}\right)\left(1+\frac{b_{\delta p}+b_{\delta q}}{2}\right).\nonumber \end{align} $$
Since
$p\ne q$
, we have
$$ \begin{align*}b_{p p}=-1,\quad b_{p q}=0\, , \end{align*} $$
and so (21) computed for
$\delta =q$
gives
$$ \begin{align*}\Re\mathfrak e\left(\left(\bar \lambda_{p q}-\lambda_{p p}\right)\left(\frac{\sqrt{-1}}{2}+\lambda_{p q}-\bar \lambda_{p p})\right)\right)=\frac14\left( -(c_{p p}-c_{p q})^2-\frac14\right)\ne 0, \end{align*} $$
as required. Therefore, equation (19) is satisfied if and only if
$$ \begin{align*}B_{p\bar q}=0, \mbox{ for all }p\neq q. \end{align*} $$
Next, we focus on (20). We have
$$ \begin{align*}\begin{aligned} \partial\bar{\partial}(\omega^p\wedge\bar{\gamma}^q)=&\, \partial\left(\frac{\sqrt{-1}}{2}\omega^p\wedge\bar{\omega}^p\wedge\bar{\gamma}^q-\omega^p\wedge\left(\sum_{\delta=1}^s\bar \lambda_{\delta q}\bar{\omega}^{\delta}\wedge \bar{\gamma}^q\right)\right)\\ \end{aligned} \end{align*} $$
and
$$ \begin{align*}\begin{aligned} \partial\bar{\partial}(\omega^p\wedge\bar{\gamma}^q)=&\, \frac{\sqrt{-1}}{2}\left(-\frac{\sqrt{-1}}{2}\omega^p\wedge\omega^p\wedge\bar{\omega}^p\wedge\bar{\gamma}^q+\omega^p\wedge\bar{\omega}^p\wedge\left(-\sum_{\delta=1}^s\bar {\lambda}_{\delta q}\omega^{\delta}\wedge\bar{\gamma}^q\right)\right)\\ &\,+\sum_{\delta=1}^s\frac{\sqrt{-1}}{2}\bar \lambda_{\delta q}\omega^p\wedge\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge\bar{\gamma}^q+\sum_{\delta=1}^s\bar \lambda_{\delta q}\omega^p\wedge\bar{\omega}^{\delta}\kern1pt{\wedge}\kern1pt\left(\sum_{a=1}^s\bar \lambda_{aq}\omega^a\kern1pt{\wedge}\kern1pt\bar{\gamma}^q\right). \end{aligned} \end{align*} $$
Hence, we get
$$ \begin{align*} \partial\bar{\partial}(\omega^p\wedge\bar{\gamma}^q)&= \sum_{\substack{\delta=1\\ \delta\ne p}}^s\frac{\sqrt{-1}}{2}\bar\lambda_{\delta q}\omega^p\wedge\bar{\omega}^p\wedge\omega^{\delta}\wedge\bar\gamma^q+\sum_{\substack{\delta=1\\ \delta\ne p}}^s\frac{\sqrt{-1}}{2}\bar \lambda_{\delta q}\omega^p\wedge\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge\bar{\gamma}^q\\ &\quad+\sum_{\substack{\delta, a \\ a\ne p}}\bar \lambda_{\delta q}\bar \lambda_{aq}\omega^p\wedge\bar{\omega}^{\delta}\wedge\omega^a\wedge\bar{\gamma}^q \end{align*} $$
and
$$ \begin{align*} \partial\bar{\partial}(\omega^p\wedge\bar{\gamma}^q)&=\sum_{\substack{\delta=1\\ \delta\ne p}}^s\frac{\sqrt{-1}}{2}\bar\lambda_{\delta q}\omega^p\wedge\bar{\omega}^p\wedge\omega^{\delta}\wedge\bar\gamma^q+\sum_{\substack{a=1\\ a\ne p }}^s\bar\lambda_{pq}\bar\lambda_{aq}\omega^p\wedge\bar{\omega}^{p}\wedge\omega^a\wedge\bar{\gamma}^q\\&\quad+\sum_{\substack{\delta=1\\ \delta\ne p}}^s\frac{\sqrt{-1}}{2}\bar\lambda_{\delta q}\omega^p\wedge\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge\bar{\gamma}^q+\sum_{\substack{\delta, a \\ \delta\ne p \\ a\ne p}}\bar\lambda_{\delta q}\bar\lambda_{aq}\omega^p\wedge\bar{\omega}^{\delta}\wedge\omega^a\wedge\bar{\gamma}^q. \end{align*} $$
Therefore,
$$ \begin{align*} &\partial\bar{\partial}(\omega^p\wedge\bar{\gamma}^q)=\sum_{\substack{\delta=1\\ \delta\ne p}}^s\bar\lambda_{\delta q}\left(\frac{\sqrt{-1}}{2}+\bar\lambda_{pq}\right)\omega^p\wedge\bar{\omega}^p\wedge\omega^{\delta}\wedge\bar\gamma^q\\ &\quad+\sum_{\substack{\delta=1\\ \delta\ne p}}^s\bar\lambda_{\delta q}\left(\frac{\sqrt{-1}}{2}-\bar\lambda_{\delta q}\right)\omega^p\wedge\omega^{\delta}\wedge\bar{\omega}^{\delta}\wedge\bar{\gamma}^q+\sum_{\substack{\delta\ne a \\ \delta\ne p \\ a\ne p}}\bar\lambda_{\delta q}\bar\lambda_{aq}\omega^p\wedge\bar{\omega}^{\delta}\wedge\omega^a\wedge\bar{\gamma}^q \end{align*} $$
and (20) is equivalent to
$$ \begin{align*}C_{p\bar{q}}\left(\sum_{\substack{\delta=1\\ \delta\ne p}}^s\bar\lambda_{\delta q}\left(\frac{\sqrt{-1}}{2}+\bar\lambda_{pq}\right)\bar{\omega}^p\wedge\omega^{\delta} +\sum_{\substack{\delta=1\\ \delta\ne p}}^s\bar\lambda_{\delta q}\left(\frac{\sqrt{-1}}{2}-\bar\lambda_{\delta q}\right)\omega^{\delta}\wedge\bar{\omega}^{\delta} +\sum_{\substack{\delta\ne a \\ \delta\ne p \\ a\ne p}}\bar\lambda_{\delta q}\bar\lambda_{aq}\bar{\omega}^{\delta}\wedge\omega^a\right)=0, \end{align*} $$
for every
$p,q=1,\dots ,s$
. Since
$$ \begin{align*}\lambda_{pq}\neq \pm \frac{\sqrt{-1}}{2}\, ,\quad \mbox {for all }\,\,p,q=1,\dots,s\, , \end{align*} $$
the quantity
$$ \begin{align*}E_{p\bar q}:=\sum_{\substack{\delta=1\\ \delta\ne p}}^s\bar\lambda_{\delta q}\left(\frac{\sqrt{-1}}{2}+\bar\lambda_{pq}\right)\bar{\omega}^p\wedge\omega^{\delta} +\sum_{\substack{\delta=1\\ \delta\ne p}}^s\bar\lambda_{\delta q}\left(\frac{\sqrt{-1}}{2}-\bar\lambda_{\delta q}\right)\omega^{\delta}\wedge\bar{\omega}^{\delta} +\sum_{\substack{\delta\ne a \\ \delta\ne p \\ a\ne p}}\bar\lambda_{\delta q}\bar\lambda_{aq}\bar{\omega}^{\delta}\wedge\omega^a \end{align*} $$
is vanishing if and only if
$$ \begin{align*}\lambda_{\delta q}=0\, , \quad \mbox{ for all } \delta\ne p. \end{align*} $$
Since
$\lambda _{qq}\neq 0$
, it follows
$$ \begin{align*}E_{p\bar q}\neq 0,\quad \mbox{ for every }p,q\mbox{ with }p\neq q \end{align*} $$
and
$$ \begin{align*}E_{p\bar p}=0\mbox{ if and only if }c_{\delta p}=0, \mbox{for all }\delta\neq p. \end{align*} $$
Hence, the claim follows.
Proposition 5.3 Let
$$ \begin{align} \omega=\sqrt{-1}\sum_{i=1}^sA_i\omega^i\wedge \bar \omega^i+ B_i\gamma^i\wedge \bar \gamma^i+ \sqrt{-1}\sum_{r=1}^{k}\left( C_{r}\omega^{p_r}\wedge \bar \gamma^{p_r}+\bar C_{r}\gamma^{p_r}\wedge \bar \omega^{p_r} \right) \end{align} $$
be a left-invariant pluriclosed Hermitian metric on an Oeljeklaus–Toma manifold, where the components are with respect to a coframe
$\{\omega ^1,\dots ,\omega ^s,\gamma ^1,\dots ,\gamma ^s\}$
satisfying (1) and (15) and
$\{p_1,\dots ,p_k\}\subseteq \{1,\dots ,s\}$
are such that
$$ \begin{align*}\lambda_{jp_i}=0\, ,\mbox{ for all }j\neq p_i\, , \mbox{ for all } i=1,\dots,k. \end{align*} $$
Then the
$(1,1)$
-part of the Bismut–Ricci form of
$\omega $
takes the following expression:
$$ \begin{align*}\rho^{1,1}_B=-\sqrt{-1}\sum_{r=1}^k\frac{3}{4}\left(1+\frac{\lvert C_{r}\rvert^2}{A_{p_r}B_{p_r}-\lvert C_{r}\rvert^2}\right)\omega^{p_r}\wedge \bar \omega^{p_r}-\sqrt{-1}\sum_{i\not\in\{p_1,\ldots, p_k\}} \frac{3}{4}\omega^i\wedge\bar{\omega}^i \end{align*} $$
$$ \begin{align*}-\sqrt{-1}\sum_{r=1}^k\left(-\frac{3}{16}-\frac{c_{p_rp_r}^2}{4}-\frac{\sqrt{-1}c_{p_rp_r}}{4}\right)\frac{B_{p_r}C_r}{A_{p_r}B_{p_r}-|C_r|^2}\omega^{p_r}\wedge \bar \gamma^{p_r} + \quad {\textit{conjugates}}. \end{align*} $$
Proof We recall that the Bismut–Ricci form of a left-invariant Hermitian metric
$\omega =\sqrt {-1}\sum _{a,b=1}^n g_{a\bar b}\alpha ^a\wedge \bar \alpha ^b $
on a Lie group
$G^{2n}$
with a left-invariant complex structure takes the following algebraic expression:
$$ \begin{align} \rho_B(X, Y)=&-\sum_{a,b=1}^{n}g^{a\bar b}\omega([[X,Y]^{1,0}, X_a], \bar{X_b})+ g^{\bar a b }\omega([[X,Y]^{0,1},\bar{X_a}], X_b)\\&+\sqrt{-1} \sum_{a,b=1}^ng^{a\bar b}\omega([X,Y],J[X_a,\bar{X_b}])\, , \nonumber\end{align} $$
for every left-invariant vector fields
$X,Y$
on G, where
$\{\alpha ^i\}$
is a left-invariant
$(1,0)$
-coframe with dual frame
$\{X_a\}$
and
$(g^{\bar ba})$
is the inverse matrix of
$(g_{i\bar j})$
(see, e.g., [Reference Vezzoni31]). We apply (23) to a left-invariant Hermitian metric on an Oeljeklaus–Toma manifold of the form (22).
We have
$$ \begin{align*} g^{\bar i s+i}=\begin{cases} 0, \quad & \mbox{if }i\not\in\{p_1,\ldots, p_k\}\, , \\ -\frac{C_i}{A_iB_i-\lvert C_i\rvert^2},\quad & \mbox{otherwise}\, , \end{cases}\quad g^{\bar i i}=\frac{B_i}{A_iB_i-\lvert C_i\rvert^2}, \quad g^{\overline{ s+i}s+i }=\frac{A_i}{A_iB_i-\lvert C_i\rvert^2}, \end{align*} $$
and taking into account that the ideal
$\mathfrak {I}$
is abelian, we have
$$ \begin{align*}\rho_B(X,Y)=-\sum_{i=1}^4\rho_i(X,Y), \end{align*} $$
where
$$ \begin{align*} \rho_1(X,Y)&=\sum_{a=1}^sg^{a\bar a}(\omega([[X,Y]^{1,0}, Z_a], \bar Z_a)-\frac{ \sqrt{-1}}{2}\omega([X,Y],Z_a-\bar Z_a)\\&\quad+\omega([[X,Y]^{0,1},\bar Z_a],Z_a)),\\ \rho_2(X,Y)&=\sum_{a=1}^sg^{s+a\overline {s+a}}(\omega([[X,Y]^{1,0}, W_a], \bar W_a)+\omega([[X,Y]^{0,1},\bar W_a], W_a)),\\ \rho_3(X,Y)&=\sum_{r=1}^kg^{p_r\overline{s+p_r}}\left(\omega([[X,Y]^{1,0}, Z_{p_r}], \bar W_{p_r})-\omega([X,Y],[Z_{p_r},\bar W_{p_r}])\right)\\&\quad+g^{\overline {p_r} s+p_r }\omega([[X,Y]^{0,1},\bar Z_{p_r}], W_{p_r})\, ,\\ \rho_4(X,Y)&=\sum_{r=1}^kg^{s+p_r\bar p_r}\left(\omega([[X,Y]^{1,0}, W_{p_r}], \bar Z_{p_r})+\omega([X,Y],[W_{p_r},\bar Z_{p_r}]))\right)\\&\quad+ g^{\overline{s+p_r} p_r }\omega([[X,Y]^{0,1},\bar W_{p_r}], Z_{p_r}). \end{align*} $$
Next, we focus on the computation of
$\rho _B(Z_i,\bar Z_j).$
Thanks to (1), we easily obtain that
$$ \begin{align*}\rho_B(Z_i,\bar Z_j)=0\, , \quad \mbox{for every } i,j=1,\ldots, s\, ,\, \, i\ne j. \end{align*} $$
On the other hand,
$$ \begin{align*} \rho_1(Z_i,\bar Z_i)&= -\frac{\sqrt{-1}}{2}\sum_{a=1}^sg^{a\bar a}\left(-\frac{\sqrt{-1}}{2}\omega(Z_i+\bar Z_i, Z_a-\bar Z_a)\right) &\\&= \,\frac{\sqrt{-1}}{2}g^{i\bar i}A_i=\frac{\sqrt{-1}}{2}\left(\frac{A_iB_i}{A_iB_i-\lvert C_i\rvert^2}\right). \end{align*} $$
Moreover, we have
$$ \begin{align*}\begin{aligned} \rho_2(Z_i,\bar Z_i)=& -\frac{\sqrt{-1}}{2}\sum_{a=1}^sg^{s+a\overline {s+a}} (\omega([Z_i, W_a], \bar W_a)+\omega([\bar Z_i,\bar W_a],W_a)\\ =&-\sqrt{-1}\sum_{a=1}^sg^{s+a\overline {s+a}}\Re\mathfrak e\, \omega([Z_i,W_a], \bar W_a). \end{aligned} \end{align*} $$
Using (1), we have
$$ \begin{align*}\omega([Z_i, W_a], \bar W_a)= -\sqrt{-1}\lambda_{ia}B_a\, , \end{align*} $$
$$ \begin{align*}\Re\mathfrak e\, \omega([Z_i,W_a], \bar W_a)= \frac{B_ab_{ia}}{4}=-\frac{B_a}{4}\delta_{ia}. \end{align*} $$
Then
$$ \begin{align*}\rho_2(Z_i,\bar Z_i)=\sqrt{-1}\frac{g^{s+i\overline{s+i}}B_i}{4}=\frac{\sqrt{-1}}{4}\frac{A_iB_i}{A_i B_i-\lvert C_i\rvert^2}. \end{align*} $$
Next, we observe that
$$ \begin{align*}\rho_3(Z_i,\bar Z_i)+\rho_4(Z_i,\bar Z_i)=0, \end{align*} $$
which implies that
$$ \begin{align} \rho_B(Z_i,\bar Z_i)=\!\begin{cases}-\sqrt{-1}\frac{3}{4}\left(1+\frac{\lvert C_{r}\rvert^2}{A_{p_r}B_{p_r}-\lvert C_{r}\rvert^2}\right),\quad & \!\!\!\!\!\mbox{if there exists } r=1,\ldots, k \,\, \mbox{such that } i=p_r, \\ -\sqrt{-1}\frac34, \quad & \!\!\!\!\!\mbox{if } i\not\in \{p_1,\ldots, p_k\}. \end{cases} \end{align} $$
We have
$$ \begin{align*}\begin{aligned} \rho_3(Z_i,\bar Z_i)=&\sum_{j=1}^kg^{p_j\overline{s+p_j}}\omega([Z_i,\bar Z_i],[Z_{p_j},\bar W_{p_j}]) =-\frac{\sqrt{-1}}{2}\sum_{j=1}^kg^{p_j\overline{s+p_j}}\bar \lambda_{p_jp_j}\omega(Z_i+\bar Z_i, \bar W_{p_j}) \\ =& \begin{cases} 0,\quad & \mbox{if } i\not\in\{p_1,\ldots, p_k \}, \\ \frac{1}{2} g^{i\overline{s+i}}\bar \lambda_{ii}C_{i}, \quad &\mbox{otherwise}. \end{cases} \end{aligned} \end{align*} $$
We compute the three addends in the expression of
$\rho _4$
separately:
$$ \begin{align*} &\begin{aligned} \omega([[Z_i,\bar Z_i]^{1,0}, W_{p_j}], \bar Z_{p_j})=& -\frac{1}{2}\lambda_{ip_j}\bar C_{p_j} =&\!\!\!\!\begin{cases} 0, \quad & \mbox{if } i\not\in\{p_1,\ldots, p_k\}\quad\!\!\! \mbox{or } i\ne p_j, \\ -\frac{1}{2}\lambda_{ii} \bar C_i,\quad & \mbox{otherwise}, \end{cases} \end{aligned}\\ &\begin{aligned} \omega([Z_i,\bar Z_i],[W_{p_j},\bar Z_{p_j}])=& \frac{1}{2}\lambda_{p_jp_j}g_{\overline{i}s+p_j} =&\!\!\!\!\begin{cases}0, \quad& \mbox{if } i\not\in\{p_1,\ldots, p_k\}\quad\!\!\! \mbox{or } \,\, i\ne p_j, \\ \frac{1}{2}\lambda_{ii}\bar C_i,\quad & \mbox{otherwise}, \end{cases} \end{aligned}\\ &\begin{aligned} \omega([[Z_i,\bar Z_i]^{0,1},\bar W_{p_j}], Z_{p_j})=& \frac{1}{2}\bar \lambda_{ip_j}g_{\overline{s+p_j}p_j} =&\!\!\!\!\begin{cases}0, \quad & \mbox{if } i\ne p_j, \\ \frac{1}{2}\bar \lambda_{ii}C_{i}, \quad & \mbox{otherwise}. \end{cases} \end{aligned} \end{align*} $$
It follows
$$ \begin{align*}\rho_3(Z_i,\bar Z_i)=\rho_4(Z_i,\bar Z_i)=0\quad \mbox{ if } i\not\in\{p_1,\ldots,p_k\}, \end{align*} $$
and, for
$i\in \{p_1,\ldots , p_k\}$
,
$$ \begin{align*}&\rho_3(Z_{i},\bar Z_{i})+\rho_4(Z_{i},\bar Z_{i})\\&\quad=-\frac{1}{2} g^{i\overline{s+i}}\bar \lambda_{ii} C_i -g^{s+i\overline{i}}\frac{1}{2}\lambda_{ii}\bar C_i +g^{s+i\overline{i}}\frac{1}{2}\lambda_{ii}\bar C_i+g^{\overline{s+i}i}\frac{1}{2}\bar \lambda_{ii}C_i=0. \end{align*} $$
Now, we focus on the calculation of
$\rho _B(Z_i,\bar W_j)$
. We have
$$ \begin{align*}\begin{aligned} \rho_1(Z_i,\bar W_j)=&\, \sum_{a=1}^sg^{a\bar a}\bar \lambda_{ij}\left(-\frac{\sqrt{-1}}{2}\omega(\bar W_j, Z_a-\bar Z_a)+\omega([\bar W_j, \bar Z_a], Z_a)\right)\\ =&\, \begin{cases}0, \quad &\mbox{if } i=j\in\{p_1,\ldots, p_k\}\,, \\ \sqrt{-1}g^{i\bar i}C_i\bar \lambda_{ii}\left(\frac{\sqrt{-1}}{2}-\bar \lambda_{ii}\right), \quad & \mbox{otherwise} , \end{cases} \end{aligned} \end{align*} $$
and since
$\mathfrak {I}$
is abelian
$$ \begin{align*}\rho_2(Z_i,\bar W_j)=0. \end{align*} $$
Furthermore,
$$ \begin{align*}\begin{aligned} \rho_3(Z_i,\bar W_j)=&\, \sum_{j=1}^kg^{\overline {p_j} s+p_j }\omega([[Z_i,\bar W_j]^{0,1},\bar Z_{p_j}], W_{p_j})=-\sqrt{-1}\sum_{j=1}^kg^{\overline {p_j} s+p_j }\bar \lambda_{ij}\bar \lambda_{p_j p_j}g_{\overline{s+j}s+p_j}\\ =&\, \begin{cases}0, \quad & \mbox{if } i=j\in \{p_1,\ldots, p_k\}, \\ -\sqrt{-1}\bar \lambda_{jj}^2g^{\overline{j}s+j}B_j,\quad & \mbox{otherwise},\end{cases} \end{aligned} \end{align*} $$
and
$$ \begin{align*}\begin{aligned} \rho_4(Z_i,\bar W_j)=&\sum_{j=1}^kg^{s+p_j\bar p_j}\omega([Z_i,\bar W_j],[W_{p_j},\bar Z_{p_j}])=\sqrt{-1}\sum_{j=1}^kg^{s+p_j\bar p_j}\bar \lambda_{ij}\lambda_{p_jp_j}g_{\overline{s+j}s+p_j} \\ =&\, \begin{cases}0, \quad &\mbox{if } i=j\in \{p_1,\ldots, p_k\},\\ \sqrt{-1}g^{s+j\bar j}\bar \lambda_{jj}\lambda_{jj}B_j,\quad & \mbox{otherwise}. \end{cases} \end{aligned} \end{align*} $$
It follows that
$\rho _B(Z_i,\bar W_j)\ne 0 $
if and only if
$i=j\in \{p_1,\ldots , p_k\}.$
In such a case, we have
$$ \begin{align*}\begin{aligned} \rho_B(Z_j,\bar W_j)=& -\sqrt{-1}\left(g^{s+j\overline{j}}B_j\left(\lvert\lambda_{jj}\rvert^2-\bar \lambda_{jj}^2\right)+g^{j\bar j}C_j\bar \lambda_{jj}\left(\frac{\sqrt{-1}}{2}-\bar \lambda_{jj}\right)\right). \end{aligned} \end{align*} $$
Since
$$ \begin{align*}g^{s+j\bar j }B_j=-\frac{B_jC_j}{A_jB_j-\lvert C_j\rvert^2}\quad \mbox{and }\quad g^{j\bar j}C_j=\frac{B_jC_j}{A_jB_j-\lvert C_j\rvert^2}, \end{align*} $$
we infer
$$ \begin{align*}\rho_B(Z_j,\bar W_j)=-\sqrt{-1}\left(\bar\lambda_{jj}\left(\frac{\sqrt{-1}}{2}-\bar\lambda_{jj}\right)-\left(\lvert\lambda_{jj}\rvert^2-\bar\lambda_{jj}^2\right)\right)\frac{B_jC_j}{A_jB_j-\lvert C_j\rvert^2}. \end{align*} $$
Taking into account that
$\lambda _{jj}=-\frac {\sqrt {-1}}{4}-\frac {c_{jj}}{2}$
, we obtain
$$ \begin{align*}\rho_B(Z_j,\bar W_j)=-\sqrt{-1}\left(-\frac{3}{16}-\frac{c_{jj}^2}{4}-\frac{\sqrt{-1}c_{jj}}{4}\right)\frac{B_jC_j}{A_jB_j-\lvert C_j\rvert^2}, \end{align*} $$
and the claim follows.
Corollary 5.4 Let
$\omega $
be a left-invariant pluriclosed Hermitian metric on an Oeljeklaus–Toma manifold M. Then
$\omega $
lifts to an algebraic expanding soliton of the pluriclosed flow on the universal covering of M if and only if it takes the following diagonal expression with respect to a coframe
$\{\omega ^1,\dots ,\omega ^s,\gamma ^1,\dots ,\gamma ^s\}$
satisfying (
1
) and (
15
):
$$ \begin{align} \omega=\sqrt{-1}\sum_{i=1}^sA\omega^i\wedge \bar \omega^i+ B_i\gamma^i\wedge \bar \gamma^i. \end{align} $$
Proof Let
$\omega $
be a pluriclosed left-invariant metric on an Oeljeklaus–Toma manifold M. In view of [Reference Lauret15, Section 7],
$\omega $
lifts to an algebraic expanding soliton of the pluriclosed flow on the universal covering of M if and only if
$$ \begin{align*}\rho^{1,1}_B(\cdot,\cdot)=c \omega(\cdot,\cdot)+\frac12\left(\omega(D\cdot,\cdot)+\omega(\cdot,D\cdot)\right), \end{align*} $$
for some
$c\in {\mathbb R}_{-}$
and some derivation D of
$\mathfrak {g}$
such that
$DJ=JD$
.
Assume that
$\omega $
takes the expression in formula (25). Proposition 5.3 implies that
$\rho _B$
is represented with respect to the basis
$\{Z_1,\ldots , Z_s,W_1,\ldots , W_s\}$
by the matrix
$$ \begin{align*} P=-\frac{3}{4A} \begin{pmatrix} \mathrm{I}_{\mathfrak{h}} & 0\\ 0 & 0 \end{pmatrix}. \end{align*} $$
Since
$$ \begin{align*} \frac{3}{4A} \begin{pmatrix} 0 & 0\\ 0 & \mathrm{I}_{\mathfrak{I}} \end{pmatrix} \end{align*} $$
induces a symmetric derivation on
$\mathfrak {g}$
,
$\omega $
lifts to an algebraic expanding soliton of the pluriclosed flow on the universal covering of M and the first part of the claim follows.
In order to prove the second part of the statement, we need some preliminary observations on derivations D of
$\mathfrak {g}$
that commute with J, i.e., such that
$$ \begin{align*}D(\mathfrak{g}^{1,0})\subseteq \mathfrak{g}^{1,0}\, ,\quad D(\mathfrak{g}^{0,1})\subseteq \mathfrak{g}^{0,1}. \end{align*} $$
We can write
$$ \begin{align*}DZ_i= \sum_{j=1}^s k^i_jZ_j+m^i_jW_j\quad \mbox{ and }\quad D\bar Z_i= \sum_{j=1}^sl^i_j\bar Z_j+ r^i_j\bar W_j. \end{align*} $$
Since D is a derivation, we have, for all
$i=1,\ldots , s$
,
$$ \begin{align*}D[Z_i,\bar Z_i]=[DZ_i,\bar Z_i]+[Z_i,D\bar Z_i]. \end{align*} $$
On the other hand,
$$ \begin{align*}\begin{aligned} D[Z_i,\bar Z_i]=&\, -\frac{\sqrt{-1}}{2}\left(\sum_{j=1}^sk^i_jZ_j+l^i_j\bar Z_j+m^i_jW_j+r^i_j\bar W_j \right)\, ,\\ [DZ_i,\bar Z_i]=&\, -\frac{\sqrt{-1}}{2}k^i_i(Z_i+\bar Z_i)-\sum_{j=1}^sm^i_j\lambda_{ij}W_j,\\ [Z_i,D\bar Z_i]=&\, -\frac{\sqrt{-1}}{2}l^i_j(Z_i+\bar Z_i)+\sum_{j=1}^sr^i_j\bar \lambda_{ij}\bar W_j \end{aligned} \end{align*} $$
and
$$ \begin{align*}\begin{aligned} 0=&\, D[Z_i,\bar Z_i]-[DZ_i,\bar Z_i]-[Z_i,D\bar Z_i]\\ =&\, -\frac{\sqrt{-1}}{2}\sum_{j\ne i}k^i_jZ_j+l^i_j\bar Z_j+\frac{\sqrt{-1}}{2}l^i_iZ_i+\frac{\sqrt{-1}}{2}k^i_i\bar Z_i\\&\,+\sum_{j=1}^sm^i_j\left(\lambda_{ij}-\frac{\sqrt{-1}}{2}\right)W_j-r^i_j\left(\frac{\sqrt{-1}}{2}+\bar \lambda_{ij}\right)\bar W_j, \end{aligned} \end{align*} $$
which forces
$DZ_i, D\bar Z_i=0$
, for all
$i=1,\ldots , s$
. It follows that
$D_{|\mathfrak {h}}=0$
.
Moreover, for all
$I,I'\in \mathfrak {J}$
, we have
$$ \begin{align*}0=D[I,I']=[DI,I']+[I,DI']\, , \end{align*} $$
which implies that
$$ \begin{align*}[DI,I']=-[I,DI']. \end{align*} $$
Assume that
$$ \begin{align*}DW_i= \sum_{j=1}^s k^{s+i}_jZ_j+m^{s+i}_jW_j \quad \mbox{ and }\quad D\bar W_i= \sum_{j=1}^sl^{s+i}_j\bar Z_j+ r^{s+i}_j\bar W_j, \end{align*} $$
then
$$ \begin{align*}[DW_i,\bar W_i]=\sum_{j=1}^sk^{s+i}_j[Z_j,\bar W_i]\in\mathfrak{J}^{0,1} \quad \mbox{ and }\quad [W_i,D\bar W_i]=\sum_{j=1}^sl^{s+i}_j[W_i,\bar Z_j]\in \mathfrak{J}^{1,0}. \end{align*} $$
This implies that
$$ \begin{align*}DW_i= \sum_{j=1}^sm^{s+i}_jW_j\, ,\quad D\bar W_i= \sum_{j=1}^s r^{s+i}_j\bar W_j, \end{align*} $$
i.e.,
$D(\mathfrak {J})\subseteq \mathfrak {J}$
. Moreover, for all
$i=1,\ldots , s$
, we have that
$$ \begin{align*}D[Z_i, W_i]=-\lambda_{ii}DW_i=-\sum_{j=1}^s\lambda_{ii}m^{s+i}_jW_j, \end{align*} $$
whereas
$[DZ_i,W_i]=0$
and
$$ \begin{align*}[Z_i, DW_i]=-\sum_{j=1}^sm^{s+i}_j\lambda_{ij}W_j. \end{align*} $$
Using again the fact that D is a derivation, we have
$$ \begin{align*}DW_i=\sum_{j\in J_i}m_jW_j, \end{align*} $$
where
$$ \begin{align*}J_i=\{j\in \{1,\ldots, s\}\quad | \quad \lambda_{ii}=\lambda_{ij}\}. \end{align*} $$
With analogous computations, we infer
$$ \begin{align*}D\bar W_i=\sum_{j\in J_i}r^{s+i}_j\bar W_j. \end{align*} $$
Clearly,
$i\in J_i$
. On the other hand, for all
$i=1,\ldots , s$
, we know that
$\Im \mathfrak {m}(\lambda _{ii})\ne 0 $
, whereas, for all
$i\ne j$
,
$\lambda _{ij}\in {\mathbb R}.$
This guarantees that, for all
$i=1,\ldots , s$
,
$$ \begin{align*}J_{i}=\{i\}. \end{align*} $$
This allows us to write
$$ \begin{align*}DW_i= m^{s+i}_iW_i,\quad D\bar W_i= r^{s+i}_i\bar W_i. \end{align*} $$
From the relations above, we obtain that
$$ \begin{align*}\mathrm{Der}(\mathfrak{g})^{1,0}=\{E\in \mathrm{End}(\mathfrak{g})^{1,0}\ |\ \mathfrak{h}\subseteq\ker(E), \,\, E(\langle W_i\rangle)\subseteq \langle W_i\rangle, \quad \mbox{for all } i =1,\ldots, s\}. \end{align*} $$
First of all, we suppose that
$\omega $
is a pluriclosed Hermitian metric which takes the following diagonal expression with respect to a coframe
$\{\omega ^1,\dots ,\omega ^s,\gamma ^1,\dots ,\gamma ^s\}$
satisfying (1) and (15):
$$ \begin{align*}\omega=\sqrt{-1}\sum_{i=1}^sA_i\omega^i\wedge \bar \omega^i+ B_i\gamma^i\wedge \bar \gamma^i, \end{align*} $$
such that there exist
$i, j\in \{1,\ldots , s\}$
such that
$A_i\ne A_j$
and we suppose that
$\omega $
is an algebraic soliton. Thanks to the facts regarding derivations proved before, we have that
$$ \begin{align*}\begin{aligned} -\sqrt{-1}\frac34=\rho_B(Z_i,\bar Z_i)=&\, c\omega(Z_i,\bar Z_i)+ \frac12\left(\omega(DZ_i,\bar Z_i)+\omega(Z_i, D\bar Z_i)\right)=\sqrt{-1}cA_i,\\ -\sqrt{-1}\frac34=\rho_B(Z_j,\bar Z_j)=&\, c\omega(Z_j,\bar Z_j)+ \frac12\left(\omega(DZ_j,\bar Z_j)+\omega(Z_j, D\bar Z_j)\right)=\sqrt{-1}cA_j, \end{aligned} \end{align*} $$
which is impossible, since
$A_i\ne A_j$
.
Now, suppose that
$\omega $
is a pluriclosed metric on M which is not diagonal. So, we suppose that there exists
$\tilde {j}=1,\ldots , s$
such that
$C_{\tilde {j}}\ne 0.$
Then assume that there exist a constant
$c\in \mathbb {R}$
and
$D\in \mathrm {Der(}\mathfrak {g})$
such that
$$ \begin{align*}(\rho_B)^{1,1}(\cdot, \cdot)=c\omega(\cdot, \cdot)+ \frac12\left(\omega(D\cdot,\cdot)+\omega(\cdot,D\cdot)\right),\quad DJ=JD. \end{align*} $$
On the other hand,
$$ \begin{align*}\begin{aligned} 0\kern1pt{=}\kern1pt\rho_B(W_{\tilde{j}},\bar W_{\tilde{j}})=&\, c\omega(W_{\tilde{j}},\bar W_{\tilde{j}})+ \frac12\left(\omega(DW_{\tilde{j}},\bar W_{\tilde{j}})+\omega(W_{\tilde{j}}, D\bar W_{\tilde{j}})\right)=\sqrt{-1}cB_{\tilde{j}}+\frac{\sqrt{-1}}{2}(r_{\tilde{j}}^{s+\tilde{j}}+m_{\tilde{j}}^{s+\tilde{j}})B_{\tilde{j}}, \\ \rho_B(Z_{\tilde{j}},\bar W_{\tilde{j}})= & c\omega(Z_{\tilde{j}},\bar W_{\tilde{j}})+ \frac{1}{2}\left(\omega(DZ_{\tilde{j}},\bar W_{\tilde{j}})+\omega(Z_{\tilde{j}}, D\bar W_{\tilde{j}})\right)=\sqrt{-1}cC_{\tilde{j}}+\frac{\sqrt{-1}}{2}r^{s+\tilde{j}}_{\tilde{j}}C_{\tilde{j}}\, ,\\ \rho_B(\bar Z_{\tilde{j}}, W_{\tilde{j}})=& c\omega(\bar Z_{\tilde{j}}, W_{\tilde{j}})+ \frac12\left(\omega(D\bar Z_{\tilde{j}}, W_{\tilde{j}})+\omega(\bar Z_{\tilde{j}}, D W_{\tilde{j}})\right)=-\sqrt{-1}c\bar C_{\tilde{j}}-\frac{\sqrt{-1}}{2}m^{s+\tilde{j}}_{\tilde{j}}\bar C_{\tilde{j}}, \end{aligned} \end{align*} $$
which implies that
$$ \begin{align*}c=-\frac12(r_{\tilde{j}}^{s+{\tilde{j}}}+m_{\tilde{j}}^{s+\tilde{j}}). \end{align*} $$
On the other hand,
$$ \begin{align*}\rho_B(Z_{\tilde{j}},\bar W_{\tilde{j}})=\sqrt{-1}KC_{\tilde{j}}\, , \end{align*} $$
where
$$ \begin{align*}K=\left(\frac{3}{16}+\frac{c_{\tilde{j}\tilde{j}}^2}{4}+\frac{\sqrt{-1}c_{\tilde{j}\tilde{j}}}{4}\right)\frac{B_{\tilde{j}}}{A_{\tilde{j}}B_{\tilde{j}}-\lvert C_{\tilde{j}}\rvert^2}. \end{align*} $$
Then
$$ \begin{align*}K=c+\frac12r_{\tilde{j}}^{s+\tilde{j}}=-\frac12m_{\tilde{j}}^{s+\tilde{j}} \end{align*} $$
and
$$ \begin{align*}\bar K=c+\frac12m_{\tilde{j}}^{s+\tilde{j}}=-\frac12r_{\tilde{j}}^{s+\tilde{j}}. \end{align*} $$
From this, we obtain that
$$ \begin{align*}c=K+\bar K=2\Re\mathfrak{e}(K)>0. \end{align*} $$
On the other hand, we have
$$ \begin{align*}&-\sqrt{-1}\frac34\left(1+\frac{\lvert C_{\tilde{j}}\rvert^2}{A_{\tilde{j}}B_{\tilde{j}}-\lvert C_{\tilde{j}}\rvert^2}\right)= \rho_B(Z_{\tilde{j}},\bar Z_{\tilde{j}})\\&\quad= c\omega(Z_{\tilde{j}},\bar Z_{\tilde{j}})+ \frac12\left(\omega(DZ_{\tilde{j}},\bar Z_{\tilde{j}})+\omega(Z_{\tilde{j}},D\bar Z_{\tilde{j}})\right)=\sqrt{-1}cA_{\tilde{j}}, \end{align*} $$
which implies that c must be negative. From this, the claim follows.
Corollary 5.5 Let
$\omega $
be a pluriclosed Hermitian metric on an Oeljeklaus–Toma manifold which takes the form (16). Then the pluriclosed flow starting from
$\omega $
is equivalent to the following system of ODEs:
$$ \begin{align} \begin{cases} A_i'=\frac{3}{4},\quad &\mbox{if } i\not\in\{p_1,\ldots, p_k\}, \\ A_{p_r}'=\frac{3}{4}\left(1+\frac{\lvert C_{r}\rvert^2}{A_{p_r}B_{p_r}-\lvert C_r\rvert^2}\right),\quad& \mbox{for all } r=1,\ldots, k\, , \\ B_j'=0,\quad &\mbox{for all }j=1,\ldots, s\, , \\ C_r'= -\left(\frac{3}{16}+\frac{c_{p_rp_r}^2}{4}+\frac{\sqrt{-1}c_{p_rp_r}}{4}\right)\frac{B_{p_r}C_r}{A_{p_r}B_{p_r}-|C_r|^2},\quad &\mbox{for all } r=1,\ldots, k\, .\end{cases} \end{align} $$
Moreover,
$\lvert C_r\rvert $
is bounded, for all
$r =1,\ldots , k$
, and the solution exists for all
$ t\in [0,+\infty )$
and
$A_i\sim \frac {3}{4}t$
, as
$t\to +\infty ,$
for all
$ i=1,\ldots , s.$
In particular,
$$ \begin{align*}\frac{\omega_t}{1+t}\to 3\omega_{\infty}, \end{align*} $$
as
$t\to \infty $
.
Proof Observe that, for every
$ r\in \{1,\ldots , k\}$
,
$$ \begin{align*}\begin{aligned} (\lvert C_r\rvert^2)'=&\, - \left(\frac{3}{8}+\frac{c_{p_rp_r}^2}{2}\right)\frac{B_{p_r}\lvert C_r\rvert^2}{A_{p_r}B_{p_r}-\lvert C_r\rvert^2}\le 0\, , \end{aligned} \end{align*} $$
which guarantees that
$\lvert C_r\rvert ^2$
is bounded. On the other hand, denote, for all
$ r=1,\ldots , k$
,
$$ \begin{align*}u_r=A_{p_r}B_{p_r}- \lvert C_r\rvert^2. \end{align*} $$
We have that
$$ \begin{align*}u_r'=A^{\prime}_{p_r}B_{p_r}-(\lvert C_r\rvert^2)'=\frac{3}{4}B_{p_r}+\left(\frac{9}{8}+\frac{c^2_{p_rp_r}}{2}\right)\frac{B_{p_r}\lvert C_r\rvert^2}{A_{p_r}B_{p_r}-\lvert C_r\rvert^2}\ge 0. \end{align*} $$
This guarantees
$$ \begin{align*}A_{p_r}'=\frac{3}{4}\left(1+\frac{\lvert C_{r}\rvert^2}{A_{p_r}B_{p_r}-\lvert C_r\rvert^2}\right)\le \frac{3}{4}\left(1+\frac{K}{u_r(0)}\right), \end{align*} $$
where
$K>0$
such that
$\lvert C_r\rvert ^2\le K$
, for all
$ t\ge 0$
. This implies the long-time existence. As regards the last part of the statement, it is sufficient to prove that
$$ \begin{align*}\lim_{t\to+\infty} \frac{\lvert C_r\rvert^2}{u_r}=0. \end{align*} $$
However,
$$ \begin{align*}u_r'\ge \frac{3}{4}B_{p_r}. \end{align*} $$
Therefore,
$$ \begin{align*}u_r\ge \frac{3}{4}B_{p_r}t+u_r(0)\to +\infty\, ,\,\,\, t\to+\infty. \end{align*} $$
Then
$$ \begin{align*}\lim_{t\to+\infty}u_r(t)=+\infty\, , \end{align*} $$
and, since
$\lvert C_r\rvert ^2$
is bounded, the assertion follows.
Proof of Theorem 1.1 Let
$\omega $
be a left-invariant pluriclosed metric on an Oeljeklaus–Toma manifold. Corollary 5.5 implies that pluriclosed flow starting from
$\omega $
has a long-time solution
$\omega _t$
such that
$$ \begin{align*}\frac{\omega_t}{1+t}\to3\omega_\infty \quad \mbox{ as }\quad t\to \infty. \end{align*} $$
We show that
$\frac {\omega _t}{1+t}$
satisfies conditions 1–3 in Proposition 3.1. Here, we denote by
$|\cdot |_t$
the norm induced by
$\omega _t$
.
Taking into account that
$$ \begin{align*}\omega_{t|\mathfrak I\oplus \mathfrak I}=\omega_{0|\mathfrak I\oplus \mathfrak I}\, , \end{align*} $$
condition 2 follows.
Thanks to the fact that condition 2 holds,
$$ \begin{align*}\omega_{t|\mathfrak h\oplus \mathfrak h}= \sum_{i=1}^sA_i(t)\omega^i\wedge \bar \omega^i \end{align*} $$
with
$\frac {A_i(t)}{1+t}\to \frac 34$
as
$t\to \infty $
, and there exist
$C,T>0$
such that, for every vector
$v\in \mathfrak h$
,
$$ \begin{align*}\frac{1}{\sqrt{1+t}}|v|_t\leq C|v|_{0}, \end{align*} $$
for every
$t\geq T$
, condition 1 is satisfied.
In order to prove condition 3, let
$\epsilon , \ell>0$
and let
$\gamma $
be a curve in M tangent to
$\mathcal H$
which is parameterized by arclength with respect to
$3\omega _\infty $
and such that
$L_{\infty }(\gamma )<\ell $
. Let
$v=\dot \gamma $
and
$T>0$
such that
$$ \begin{align*}\left\vert \frac{A_i(t)}{1+t}-\frac{3}{4}\right\vert\leq \frac{3\epsilon^2}{4\ell^2}, \end{align*} $$
for
$t\ge T$
. Then
$$ \begin{align*}\left\vert\frac{1}{1+t}|v|^2_t-|v|^2_{\infty}\right\vert\le \sum_{i=1}^{s}\left\vert\frac{A_i(t)}{1+t}-\frac34 \right\vert\lvert v_i\rvert^2\leq \frac{\epsilon^2}{\ell^2} \end{align*} $$
and
$$ \begin{align*}|L_{t}(\gamma)-L_{\infty}(\gamma)|\le \int_{0}^{b}\left\lvert\frac{1}{\sqrt{1+t}}|\dot \gamma |_t-|\dot \gamma|_\infty\right\rvert da\leq \frac{\epsilon}{\ell}b\leq \epsilon , \end{align*} $$
since
$b\leq \ell $
.
Now, we show the last part of the statement, using the same argument as in Proposition 4.1, and we prove that
$(\mathbb H^s\times \mathbb C^s, \frac {\omega _t}{1+t})$
converges in the Cheeger–Gromov sense to
$(\mathbb H^s\times \mathbb C^s, \tilde {\omega }_{\infty })$
, where
$\tilde {\omega }_{\infty }$
is an algebraic soliton. Again, here we are identifying
$\omega _t$
with its pullback onto
$\mathbb H^s\times \mathbb C^s$
and we are fixing as base point the identity element of
$\mathbb H^s\times \mathbb C^s$
. It is enough to construct a one-parameter family of biholomorphisms
$\{\varphi _t\}$
of
$\mathbb H^s\times \mathbb C^s$
such that
$$ \begin{align*}\varphi_t^*\frac{\omega_t}{1+t}\to \tilde{\omega}_{\infty}. \end{align*} $$
As we already observed, since
$\mathfrak {I}$
is abelian, the endomorphism represented by the matrix
$$ \begin{align*}D=\begin{pmatrix} 0 & 0 \\ 0 & I_{\mathfrak{I}}\end{pmatrix} \end{align*} $$
is a derivation of
$\mathfrak {g}$
that commutes with the complex structure J. Then we can consider
$$ \begin{align*}d\varphi_t= \exp(s(t)D)=\begin{pmatrix}I_{\mathfrak{h}}& 0 \\ 0 & e^{s(t)}I_{\mathfrak{I}}\end{pmatrix}\in \mathrm{Aut}(\mathfrak{g}, J), \end{align*} $$
where
$s(t)=\log (\sqrt {1+t})$
. Using
$d\varphi _t$
, we can define
$$ \begin{align*}\varphi_t\in \mathrm{Aut}(\mathbb{H}^s\times\mathbb{C}^s, J). \end{align*} $$
For
$i=1,\ldots , s$
, we have
$$ \begin{align*}\begin{aligned} \frac{1}{1+t}(\varphi_t^*\omega_t)(Z_i,\bar Z_i)=&\, \frac{1}{1+t}\omega_t(Z_i,\bar Z_i)\to \frac34\sqrt{-1}\, , \quad \mbox{as } t\to \infty, \\ \frac{1}{1+t}(\varphi_t^*\omega_t)(Z_i,\bar W_i)=&\, \frac{1}{\sqrt{1+t}}\omega_t(Z_i, \bar W_i )\to 0\, , \quad \mbox{as } t\to \infty, \\ \frac{1}{1+t}(\varphi_t^*\omega_t)(W_i,\bar W_i)=&\,\omega_t(W_i,\bar W_i)=\sqrt{-1}B_i(0). \end{aligned} \end{align*} $$
Then
$$ \begin{align*}\frac{1}{1+t}\varphi_t^*\omega_t\to \tilde{\omega}_{\infty}\, , \quad \mbox{as } t\to \infty, \end{align*} $$
where
$$ \begin{align*}\tilde{\omega}_{\infty}=3\,\omega_{\infty}+\omega_{|\mathfrak{I}\oplus \mathfrak{I}}. \end{align*} $$
Notice that
$\tilde {\omega }_{\infty }$
is an algebraic soliton diagonal since
$\omega _{|\mathfrak {I}\oplus \mathfrak {I}}$
is diagonal in view of Proposition 5.2.
6 A generalization to semidirect product of Lie algebras
From the viewpoint of Lie groups, the algebraic structure of Oeljeklaus–Toma manifolds is quite rigid and some of the results in the previous sections can be generalized to semidirect product of Lie algebras.
In this section, we consider a Lie algebra
$\mathfrak {g}$
which is a semidirect product of Lie algebras
$$ \begin{align*}\mathfrak g=\mathfrak h \ltimes_{\lambda} \mathfrak I, \end{align*} $$
where
$\lambda \colon \mathfrak h\to \mathrm {Der}(\mathfrak I)$
is a representation. We further assume that
$\mathfrak {g}$
has a complex structure of the form
$$ \begin{align*}J=J_{\mathfrak h}\oplus J_{\mathfrak I}, \end{align*} $$
where
$J_{\mathfrak h}$
and
$J_{\mathfrak I}$
are complex structures on
$\mathfrak h$
and
$\mathfrak I$
, respectively.
The following assumptions are all satisfied in the case of an Oeljeklaus–Toma manifold:
-
i.
$\mathfrak h$
has
$(1,0)$
-frame such that
$\{Z_1,\dots ,Z_r\}$
such that
$[Z_k,\bar Z_k]=\,-\frac {\sqrt {-1}}{2}(Z_k+\bar Z_k)$
, for all
$k=1,\dots ,r$
, and the other brackets vanish. -
ii.
$\mathfrak I$
is a
$2s$
-dimensional abelian Lie algebra, and
$J_{\mathfrak I}$
is a complex structure on
$\mathfrak I$
. -
iii.
$\lambda (\mathfrak h^{1,0})\subseteq \mathrm {End}(\mathfrak I)^{1,0}$
. -
iv.
$\mathfrak I$
has a
$(1,0)$
-frame
$\{W_1,\dots W_s\}$
such that
$\lambda (Z)\cdot \bar W_r=\lambda _r(Z)\bar W_r$
, for every
$r=1,\dots ,s$
, where
$\lambda _r\in \Lambda ^{1,0}(\mathfrak {h})$
. -
v.
$\sum _{a=1}^s\Im \mathfrak m(\lambda _a(Z_i))$
is constant on i. -
vi.
$\mathfrak I$
has a
$(1,0)$
-frame
$\{W_1,\dots W_s\}$
such that
$\lambda (Z)\cdot W_r=\lambda ^{\prime }_r(Z) W_r$
, for every
$r=1,\dots ,s$
, where
$\lambda _r'\in \Lambda ^{1,0}(\mathfrak {h})$
and
$\sum _{a=1}^s\Im \mathfrak m(\lambda _a'(Z_i))$
is constant on i.
Note that condition i is equivalent to require that
$\mathfrak h=\underbrace {\mathfrak {f}\oplus \dots \oplus \mathfrak {f}}_{r\mbox {-times}}$
equipped with the complex structure
$J_{\mathfrak {h}}=\underbrace {J_{\mathfrak f}\oplus \dots \oplus J_{\mathfrak f}}_{r\mbox {-times}}$
, whereas in condition iv, the existence of
$\{W_r\}$
and
$\lambda _r$
is equivalent to require that
$$ \begin{align*}\lambda(Z)\circ \lambda(Z')=\lambda(Z') \circ \lambda(Z), \end{align*} $$
for every
$Z,Z'\in \mathfrak h^{1,0}$
.
The computations in Section 5 can be used to study solutions to the flow
$$ \begin{align} \partial_t\omega_t=-\rho_B^{1,1}(\omega_t) \end{align} $$
in semidirect products of Lie algebras (this flow coincides with the pluriclosed flow only when the initial metric is pluriclosed). We have the following proposition.
Proposition 6.1 Let
$\mathfrak g=\mathfrak h \ltimes _{\lambda } \mathfrak I$
be a semidirect product of Lie algebras equipped with a splitting complex structure
$J=J_{\mathfrak h}\oplus J_{\mathfrak I}$
, and let
$\omega $
be a Hermitian metric on
$\mathfrak {g}$
making
$\mathfrak h$
and
$\mathfrak I$
orthogonal. Then the Bismut–Ricci form of
$\omega $
satisfies
$\rho ^{1,1} _{B|\mathfrak h\oplus \mathfrak I}=\rho ^{1,1} _{B|\mathfrak I\oplus \mathfrak I}=0.$
If conditions
$\mathrm {i}$
–
$\mathrm {iv}$
hold and
$\omega _{|\mathfrak h\oplus \mathfrak h}$
is diagonal with respect to the frame
$\{Z_i\}$
, then the
$(1,1)$
-component of the Bismut–Ricci form of
$\omega $
does not depend on
$\omega $
and the solution to the flow (27) starting from
$\omega $
takes the following expression:
$$ \begin{align*}\omega_t=\omega-t\rho^{1,1}_B(\omega). \end{align*} $$
If conditions
$\mathrm {i}$
–
$\mathrm {iv}$
and
$\mathrm {vi}$
hold and
$\omega _{|\mathfrak h\oplus \mathfrak h}$
is a multiple of the canonical metric with respect to the frame
$\{Z_i\}$
, then
$\omega $
is a soliton for flow (27) with cosmological constant
$c=\frac {1}{2}+\sum _{a=1}^s\Im \mathfrak m(\lambda _a'(Z_i))$
.
The previous proposition does not cover the case when properties i–iv are satisfied and the restriction to
$\mathfrak h\oplus \mathfrak h$
of the initial Hermitian inner product
$$ \begin{align*}\omega=\sqrt{-1}\sum _{a,b=1}^r g_{a\bar b}\omega^a\wedge \bar \omega^b+\sqrt{-1}\sum _{a,b=1}^s g_{r+a\overline{r+ b}}\gamma^a\wedge \bar \gamma^b \end{align*} $$
is not diagonal with respect to
$\{Z_i\}$
. In this case flow (27) evolves only the components
$g_{i\bar i}$
of
$\omega $
along
$\omega ^i\wedge \bar \omega ^i$
via the ODE
$$ \begin{align*}\partial_{t}g_{i\bar i}=\frac14 \sum_{a=1}^r g^{\bar a a}\Re \mathfrak{e}\,g_{i\bar a} -\frac12 \sum_{c,d=1}^s g^{\overline{r+d} r+ c} \left\lbrace \omega([Z_i,W_c],\bar W_d) + \omega([\bar Z_i,\bar W_c],W_d) \right\rbrace, \end{align*} $$
where
$g_{i\bar i}$
depends on t. Note that the quantities
$-\frac 12 \sum _{c,d=1}^s g^{\overline {r+d} r+ c} \left \lbrace \omega ([Z_i,W_c], \bar W_d) + \omega ([\bar Z_i,\bar W_c],W_d) \right \rbrace $
appearing in the evolution of
$g_{i\bar i}$
are independent on t.
The same computations as in Section 4 imply the following proposition.
Proposition 6.2 Let
$\mathfrak g=\mathfrak h \ltimes _{\lambda } \mathfrak I$
be a semidirect product of Lie algebras equipped with a splitting complex structure
$J=J_{\mathfrak h}\oplus J_{\mathfrak I}$
. Assume that properties
$\mathrm {i}$
–
$\mathrm {iii}$
are satisfied, and let
$\omega $
be a left-invariant Hermitian metric on
$\mathfrak {g}$
. Then
$$ \begin{align*}\rho_{C | \mathfrak I \oplus \mathfrak I }=\rho_{C|\mathfrak h \oplus \mathfrak I}=0, \end{align*} $$
whereas
$ \rho _{C |\mathfrak h\oplus \mathfrak h}$
is diagonal with respect to
$\{Z_1,\ldots , Z_r\}$
.
If, in addition, property
$\mathrm {iv}$
holds, then
$$ \begin{align*}\rho_C(Z_i,\bar Z_i)=-\sqrt{-1}\left(\frac12-\sum_{a=1}^s\Im\mathfrak m(\lambda_{a}(Z_i))\right), \quad \mbox{for all } i=1,\ldots, r. \end{align*} $$
If, in addition, property
$\mathrm {v}$
holds, then
$\omega $
is a soliton for the Chern–Ricci flow with cosmological constant
$c=\frac {1}{2}-\sum _{a=1}^s\Im \mathfrak m(\lambda _a(Z_i))$
if and only if
$\omega _{\mathfrak {h}\oplus \mathfrak h}$
is a multiple of the canonical metric on
$\mathfrak h $
with respect to the frame
$\{Z_i\}$
and
$\omega _{\mathfrak h\oplus \mathfrak J }=0$
.
Acknowledgment
We are grateful to Daniele Angella, Ramiro Lafuente, Francesco Pediconi, and Alberto Raffero for useful conversations. In particular, Ramiro Lafuente suggested us how to prove the convergence in the Cheeger–Gromov sense in Theorem 1.1.
