Article contents
Attenuation of the unsteady loading on a high-rise building using top-surface open-loop control
Published online by Cambridge University Press: 03 August 2023
Abstract
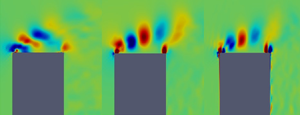
With the severity and frequency of significant weather events increasing, methods for alleviating unsteady wind loading for high-rise buildings are gaining interest. This study numerically investigates the three-dimensional flow structures around a canonical high-rise building immersed in an atmospheric boundary layer at different oncoming wind angles, using wall-resolved large eddy simulations. A synthetic jet located on the top surface is used as open-loop active actuation with the aim of suppressing the building's side-force fluctuations when exposed to oncoming wind variations. Three different frequencies of jet forcing are considered, all half an order of magnitude larger than the vortex shedding frequency. The behaviour of the synthetic jet and its effect on the building's unsteady side force, time-averaged flow fields and unsteady flow structures are investigated numerically. The synthetic jet actuation is found to reduce the side-force fluctuation of the building, enhance the downwash flow and successfully attenuate the antisymmetric vortex shedding. This was achieved to different extents across the range of oncoming wind angles considered and may motivate future attempts to explore experimental active control strategies for attenuation of unsteady wind loading.
JFM classification
- Type
- JFM Papers
- Information
- Copyright
- © The Author(s), 2023. Published by Cambridge University Press
References
- 2
- Cited by



