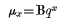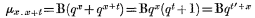The ordinary method of approximating to three-life annuities is as follows:—Let the lives be aged respectively x, y, z, (x being the youngest). Find an age w such that at the given rate of interest aw=ayz, then axyz=axw nearly. Baily diminishes the value thus found by .05, but I cannot say if this correction is usually made. It will generally be found to be too small if the lives are very young, but much too large at the older ages.
It has been shown that the above method (neglecting now the correction referred to) is strictly accurate in any mortality table where the mortality conforms to Mr. Gompertz's hypothesis, and where consequently the force of mortality can be represented throughout by the expression
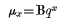
(B and q being constants). The reason for this is, that the force of mortality for two joint lives, or, x, x + t, may be represented by a similar expression
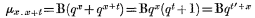
(where qt′ = qt + 1).
It follows from this that if we have

we have also
and, the force of mortality in the joint status being equal to that for the single life, throughout the table, the two annuities are identical, not only as to their total value, but also as to the value of each successive payment.