Published online by Cambridge University Press: 14 February 2012
Two eigenvalue problems associated with steady rotations of a chain are considered. To compare the spectra of these two problems, let σK(n) denote the set of all angular velocities with which a chain of unit length with one end fixed and the other free can rotate in a vertical plane so as to have exactly n nodes on thevertical axis (including the fixed end). In a linearised theory σK(n) is a single point, i.e.
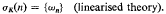
In the full non-linear theory σK(n) is an infinite interval lying to the right of ⍵n. Indeed,
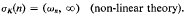
This is established in [1].
Next, let σM(β, n) denote the set of all angular velocities with which a chain, having ends fixed at unit distance apart on the vertical axis, can rotate in a vertical plane so as to have exactly n+l nodes on the vertical axis (including the ends) and so that the tension takes the value β at the lower end. This problem, in which the length of the chain is not prescribed is a model for a spinning process in which the ‘chain’ is continuously created in a rotating configuration. For β>0, we again have in the linearised theory that σM(β, n) is a singleton, i.e.

In the full non-linear theory σM(β, n) lies to the left of λn(β). Although unable to determine exactly σM(β, n) for β>0, we have
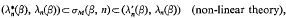
where ⍵n λn(β), 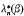 and
and  are all characterised as the nth zeros of known combinations of Bessel functions.
are all characterised as the nth zeros of known combinations of Bessel functions.