Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Chandra, Prem
1972.
Absolute Cesàro summability factors of trigonometric series.
Rendiconti del Circolo Matematico di Palermo,
Vol. 21,
Issue. 1-2,
p.
140.
Krasniqi, Xhevat Z.
2014.
On absolute matrix summability of orthogonal series.
Applied Mathematics and Computation,
Vol. 230,
Issue. ,
p.
711.


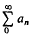 be any given infinite series with
be any given infinite series with