Hostname: page-component-78c5997874-mlc7c Total loading time: 0 Render date: 2024-11-20T04:42:04.410Z Has data issue: false hasContentIssue false
  • English
  • Français

On rooted properties of complex analytic systems*

Published online by Cambridge University Press:  20 January 2009

Xiao-Qiang Zhao
Xiao-Qiang Zhao
Affiliation:
Institute of Applied MathematicsAcademia SinicaP.O. Box 2734Beijing 100080P.R., China
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper is devoted to the study of rooted properties of phase surfaces defined by complex analytic systems. We first obtain the Rooted Theorem of Analytic Systems. Then we prove the Generalized Strong Rooted Theorem of 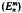(m ≧ 2), which implying the Strong Rooted Theorem of a Class of .

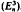Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 37 , Issue 2 , June 1994 , pp. 255 - 270
Copyright
Copyright © Edinburgh Mathematical Society 1994

References

REFERENCES

1.Ahlfors, L. V., Complex Analysis, Third Edition (McGraw-Hill Book Co., 1979).Google Scholar
2.Qin, Yuan-Xun, On Integral Surfaces Defined by Ordinary Differential Equations (in Chinese) (North West University Press, Xian, China, 1985).Google Scholar
3.Qin, Yuan-Xun (Y. S. Chin), On Surfaces Defined by Ordinary Differential Equations, A New Approach to Hilbert's 16th Problem, Ordinary and Partial Differential Equations (Sleeman, B. D. and Jarvis, R. J., eds.), Springer-Verlag, Lecture Notes in Math. 1151 (1985), 115–131.Google Scholar
4.Qin, Yuan-Xun, New Progress in the Theory of Surfaces Defined by Ordinary Differential Equations, Kexue Tongbao 33 (1988), 1361–1363.Google Scholar
5.Zhao, Xiao-Qiang, On Basic Theorems in The Complex Qualitative Theory of Ordinary Differential Equations, Kexue Tongbao 35 (1990).Google Scholar