Published online by Cambridge University Press: 10 February 2022
We consider meromorphic solutions of functional-differential equations
\[ f^{(k)}(z)=a(f^{n}\circ g)(z)+bf(z)+c, \]where $n,\,~k$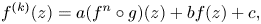
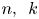 are two positive integers. Firstly, using an elementary method, we describe the forms of $f$
are two positive integers. Firstly, using an elementary method, we describe the forms of $f$ and $g$
and $g$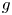 when $f$
when $f$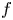 is rational and $a(\neq 0)$
is rational and $a(\neq 0)$ , $b$
, $b$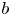 , $c$
, $c$ are constants. In addition, by employing Nevanlinna theory, we show that $g$
are constants. In addition, by employing Nevanlinna theory, we show that $g$ must be linear when $f$
must be linear when $f$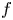 is transcendental and $a(\neq 0)$
is transcendental and $a(\neq 0)$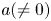 , $b$
, $b$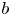 , $c$
, $c$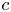 are polynomials in $\mathbb {C}$
are polynomials in $\mathbb {C}$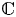 .
.