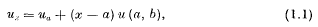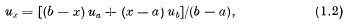Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Aitken, A. C.
1939.
XI.—Studies in Practical Mathematics. III. The Application of Quadratic Extrapolation to the Evaluation of Derivatives, and to Inverse Interpolation.
Proceedings of the Royal Society of Edinburgh,
Vol. 58,
Issue. ,
p.
161.
Womersley, J. R.
1946.
Scientific computing in Great Britain.
Mathematics of Computation,
Vol. 2,
Issue. 15,
p.
110.
Greville, Thomas N. E.
1948.
Recent Developments in Graduation and Interpolation.
Journal of the American Statistical Association,
Vol. 43,
Issue. 243,
p.
428.
Thomas, J. M.
Aroian, L. A.
Ransom, W. R.
Young, F. H.
Ericksen, J. L.
and
Matteson, L. J.
1948.
Classroom Notes.
The American Mathematical Monthly,
Vol. 55,
Issue. 9,
p.
567.
Tweedie, M. C. K.
1954.
A modification of the Aitken-Neville linear iterative procedures for polynomial interpolation.
Mathematics of Computation,
Vol. 8,
Issue. 45,
p.
13.
1958.
Electronic Digital Computers - Their Use in Science and Engineering.
Vol. 4,
Issue. ,
p.
313.
Mariani, J.
Spira, Robert
Farnell, A. B.
Graves, Lawrence M.
Moore, D. H.
and
Butler, R.
1962.
Mathematical Notes.
The American Mathematical Monthly,
Vol. 69,
Issue. 2,
p.
125.
Gershinsky, Morris
and
Levine, David A.
1964.
Aitken-Hermite Interpolation.
Journal of the ACM,
Vol. 11,
Issue. 3,
p.
352.
Jackson, Robert
and
Erdelyi, Edward
1968.
Combination and Separation of Coordinates and Modular Programming for DC Machine Fields.
IEEE Transactions on Power Apparatus and Systems,
Vol. PAS-87,
Issue. 3,
p.
659.
Saveland, Walt
and
Glick, Paul C.
1969.
First-marriage decrement tables by color and sex for the United States in 1958–60.
Demography,
Vol. 6,
Issue. 3,
p.
243.
Krogh, Fred T.
1970.
Efficient algorithms for polynomial interpolation and numerical differentiation.
Mathematics of Computation,
Vol. 24,
Issue. 109,
p.
185.
Joyce, D. C.
1971.
Survey of Extrapolation Processes in Numerical Analysis.
SIAM Review,
Vol. 13,
Issue. 4,
p.
435.
Radzyner, R.
and
Bason, P.
1972.
An error bound for Lagrange interpolation of low-pass functions (Corresp.).
IEEE Transactions on Information Theory,
Vol. 18,
Issue. 5,
p.
669.
Wynn, P.
1973.
Upon some continuous prediction algorithms. II.
Calcolo,
Vol. 9,
Issue. 4,
p.
235.
1976.
The Methods and Materials of Demography.
p.
531.
Wynn, P.
1977.
The calculus of finite differences over certain systems of numbers.
Calcolo,
Vol. 14,
Issue. 4,
p.
303.
Ohba, R.
Ono, G.
Suyama, E.
Seto, N.
and
Fukui, J.
1977.
Microprocessor application to an intelligent terminal for the accurate length measurement of moving materials.
IFAC Proceedings Volumes,
Vol. 10,
Issue. 16,
p.
225.
M�hlbach, G.
1978.
The general Neville-Aitken-algorithm and some applications.
Numerische Mathematik,
Vol. 31,
Issue. 1,
p.
97.
1988.
Numerical Methods for Initial Value Problems in Ordinary Differential Equations.
p.
247.
Hazewinkel, M.
1988.
Encyclopaedia of Mathematics.
p.
1.