Hostname: page-component-cd9895bd7-gxg78 Total loading time: 0 Render date: 2024-12-26T00:24:26.383Z Has data issue: false hasContentIssue false
  • English
  • Français

Nearly directed subspaces of partially ordered linear spaces

Published online by Cambridge University Press:  20 January 2009

G. J. O. Jameson
G. J. O. Jameson
Affiliation:
University of Edinburgh and University of Warwick
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let X be a partially ordered linear space, i.e. a real linear space with a reflexive, transitive relation ≦ such that

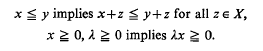
Type
Research Article
Information
Proceedings of the Edinburgh Mathematical Society , Volume 16 , Issue 2 , December 1968 , pp. 135 - 144
Copyright
Copyright © Edinburgh Mathematical Society 1968

References

REFERENCES

(1) Bonsall, F. F., Extreme maximal ideals of a partially ordered vector space, Proc. Amer. Math. Soc. 7 (1956), 831–837.CrossRefGoogle Scholar
(2) Edwards, D. A., On the homeomorphic affine embedding of a locally compact cone into a Banach dual space endowed with the vague topology, Proc. London Math. Soc. (3) 14 (1964), 399–414.CrossRefGoogle Scholar
(3) Ellis, A. J., Perfect order ideals, J. London Math Soc. 40 (1965), 288-294.Google Scholar
(4) Hayes, A., Indecomposable positive additive functionals, J. London Math Soc. 41 (1966), 318–322.CrossRefGoogle Scholar
(5) Jameson, G. J. O., Topological M-spaces, Math. Zeitschrift 103 (1968), 139–150.CrossRefGoogle Scholar
(6) Kist, J., Indecomposable maximal ideals of a partially ordered vector space, London Math. Soc. 36 (1961), 436–438.CrossRefGoogle Scholar
(7) Schaefer, H. H., Topological Vector Spaces (Macmillan, New York 1966).Google Scholar