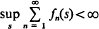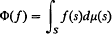Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Kalton, N. J.
1977.
Linear operators whose domain is locally convex.
Proceedings of the Edinburgh Mathematical Society,
Vol. 20,
Issue. 4,
p.
293.
Fischer, Werner
and
Schöler, Ulrich
1979.
Integral Representation of Additive Operators with Respect to a Vector Measure and its Applications.
Mathematische Nachrichten,
Vol. 87,
Issue. 1,
p.
249.
Kalton, N. J.
1983.
Isomorphisms between spaces of vector-valued continuous functions.
Proceedings of the Edinburgh Mathematical Society,
Vol. 26,
Issue. 1,
p.
29.
Kakol, Jerzy
1987.
Basic sequences and non locally convex topological vector spaces.
Rendiconti del Circolo Matematico di Palermo,
Vol. 36,
Issue. 1,
p.
95.
Labuda, Iwo
and
Szeptycki, Pawel
1988.
Extensions of integral operators.
Mathematische Annalen,
Vol. 281,
Issue. 2,
p.
341.
Nawrocki, M.
1991.
Duals of vector-valued Hp-spaces for 0<p<1.
Indagationes Mathematicae,
Vol. 2,
Issue. 2,
p.
233.
Díaz, Santiago
1993.
Unconditional convergence and weakly compact maps.
Proceedings of the American Mathematical Society,
Vol. 117,
Issue. 2,
p.
417.
Bonet, José
and
Lindström, Mikael
1994.
Spaces of operators between Fréchet spaces.
Mathematical Proceedings of the Cambridge Philosophical Society,
Vol. 115,
Issue. 1,
p.
133.
Jefferies, Brian
and
Ricker, Werner J.
1994.
Integration with respect to vector valued Radon polymeasures.
Journal of the Australian Mathematical Society. Series A. Pure Mathematics and Statistics,
Vol. 56,
Issue. 1,
p.
17.
Bonet, J.
Domański, P.
Lindström, M.
and
Ramanujan, M. S.
1995.
Operator Spaces Containing c 0 OR l∞.
Results in Mathematics,
Vol. 28,
Issue. 3-4,
p.
250.
Drewnowski, Lech
and
Labuda, Iwo
1998.
Copies of 𝑐₀ and ℓ_{∞} in topological Riesz spaces.
Transactions of the American Mathematical Society,
Vol. 350,
Issue. 9,
p.
3555.
DEL CAMPO, R.
and
RICKER, W. J.
2010.
THE FATOU COMPLETION OF A FRÉCHET FUNCTION SPACE AND APPLICATIONS.
Journal of the Australian Mathematical Society,
Vol. 88,
Issue. 1,
p.
49.
Labuda, Iwo
2010.
L 1(μ) for vector-valued measure μ.
Positivity,
Vol. 14,
Issue. 4,
p.
801.
Drewnowski, Lech
and
Labuda, Iwo
2013.
Bartle–Dunford–Schwartz integration.
Journal of Mathematical Analysis and Applications,
Vol. 401,
Issue. 2,
p.
620.