Article contents
A model for estimating standing crop in ancient communities
Published online by Cambridge University Press: 08 February 2016
Abstract
The paleoecologist has been hampered in his studies of ancient communities by an inability to obtain reasonable estimates of standing crops and diversities. Finding the diversity of an ancient community is no simple matter, because a fossil assemblage is a mixture of living and recently dead individuals from that community. Considerable numbers of each group may be missing. The relationship of the elements in a fossil population for an individual species can be expressed as follows: F = Fl + Fd, where F is the average number of fossil individuals collected per unit area, Fl is the part of the sample produced by the burial of live individuals, and Fd is the part produced by the individuals already dead at the time of burial. Fl is a product of the standing crop S (number of individuals per unit area), multiplied by the probability of failure P (proportion of the standing crop which can be expected to fail to escape anastrophic burial), or Fl = (S) (P). Fd is the product of the death rate D (number of individuals which die per age-class time unit) multiplied by the residence time R (average length of time expressed in age-class time units that the remains of an individual will persist after death), or Fd = (D) (R). The probability of failure, residence time, and death rate can all be estimated from modern communities; the death rate can also be estimated from the fossils. Thus, the standing crop of an individual species can be expressed as follows:
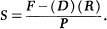
This equation can be modified to eliminate the need to estimate D, as follows
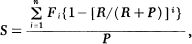 where i is the subscript indicating the number of the specific age class where the youngest is 1 and oldest is n. Once the standing crop for each species in the community has been estimated, a meaningful diversity can be calculated. Study of the model reveals that in populations where high-early mortality is the rule the likelihood of error in estimating population parameters is lower than for constant and low-early mortality populations.
where i is the subscript indicating the number of the specific age class where the youngest is 1 and oldest is n. Once the standing crop for each species in the community has been estimated, a meaningful diversity can be calculated. Study of the model reveals that in populations where high-early mortality is the rule the likelihood of error in estimating population parameters is lower than for constant and low-early mortality populations.
- Type
- Research Article
- Information
- Copyright
- Copyright © The Paleontological Society
References
Literature Cited
- 7
- Cited by


