Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-23T23:36:42.242Z Has data issue: false hasContentIssue false
  • English
  • Français

Sur les variétés canoniques de dimension 3 d’indice positif

Published online by Cambridge University Press:  22 January 2016

X. Benveniste
X. Benveniste*
Affiliation:
Centre de Mathématiques, Ecole Polytechnique, F-91 128 PALAISEAU, France
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Dans tout ce qui suit, les varietés qui interviendront seront définies sur le corps des nombres complexes C. L’objet de cet article est de préciser le résultat suivant obtenu dans [B-2] et [K-1]:

Théorème 0. Soit X une variété protective normale de dimension 3, dont les singularités sont canoniques au sens de [R-1]. Soient e le p.p.c.m. des indices des points singuliers de X. Soit R un diviseur de Cartier sur X, tel que le bidual de 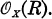soit isomorphe à Supposons que R soit numériquement positif et que R3 > 0. Alors il existe un entier n0 > 0 tel que pour tout le système linéaire |nR| soit sans point base; en particulier l’anneau 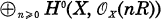est de type fini sur C.

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 97 , March 1985 , pp. 137 - 167
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1985

References

[B-1] Benveniste, X., Sur les variétés de dimension 3 de type général dont le diviseur canonique est numériquement positif, Math. Ann., 266 (1984), 479–497.CrossRefGoogle Scholar
[B-2] Benveniste, X., Sur Panneau canonique de certaines variétés de dimension 3, Invent. Math., 73 (1983), 153–164.Google Scholar
[B-3] Benveniste, X., Sur la décomposition de Zariski en dimension 3, A paraître.Google Scholar
[E] Elkik, R., Rationnalité des singularités canoniques, Invent. Math., 47 (1978), 139–147.CrossRefGoogle Scholar
[F] Fujita, T., Semi-positive line bundles, A paraître.Google Scholar
[Ha] Hartshorne, R., Algebraic Geometry, G.T.M. 52, Springer Verlag (1977).Google Scholar
[Hi] Hironaka, H., Resolution of an algebraic variety over a field of characteristic zero (I, II), Ann. of Math., 79 no. 1 (1964).Google Scholar
[K] Kawamata, Y., A generalisation of Kodaira Ramanujam’s vanishing theorem, Math. Ann., 261 (1983), 43–46.CrossRefGoogle Scholar
[K-1] Kawamata, Y., The cone of curves of algebraic varieties, Ann. of Math., 119 (1984), 603–633.CrossRefGoogle Scholar
[M] Mumford, D., The topology of normal singularities of an algebraic surface and a criterion for simplicity, I.H.E.S. Publ. Math. no. 9 (1971).Google Scholar
[Ra] Ramanujam, C. P., Supplément à l’article “Remarks on Kodaira Vanishing Theorem”, J. Indian, Math. Soc, 38 (1974), 121–124.Google Scholar
[R-1] Reid, M., Canonical 3-folds, Proceedings de “Journees de Géométrie Algébrique” Angers 1979, A. Beauville éditeur, 273–310, Sijthoff and Noordhoff 1980.Google Scholar
[R-2] Reid, M., Minimal models of canonical 3-folds, Adv. Stu. in Pure Math., Algebraic Varieties and Analytic Varieties, ed. Iitaka, S., 1 (1983), 131–180.Google Scholar
[S] Shepherd-Barron, N., Canonical 3-fold singularities are Cohen-Macaulay, Warwick, preprint.Google Scholar
[V] Viehweg, E., Vanishing theorem, J. Reine Angew. Math., 335 (1982).Google Scholar
[Z] Zariski, O., The Theorem of Riemann-Roch for high multiples of an effective divisor on an algebraic surface, Ann. of Math., 76 (1962), 560–615.CrossRefGoogle Scholar