Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-23T23:24:20.711Z Has data issue: false hasContentIssue false
  • English
  • Français

On global cluster sets for functions meromorphic on some Riemann surfaces

Published online by Cambridge University Press:  22 January 2016

Shigeo Segawa
Shigeo Segawa*
Affiliation:
Department of Mathematics Daido, Institute of Technology Daido, Minami, Nagoya 457, Japan
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Consider a single-valued meromorphic function w = f(p) defined on an open Riemann surface R with an ideal boundary β. In [1], Collingwood and Cartwright introduced the global cluster set for a function meromorphic on the unit disk. Generalizing the definition of global cluster sets to our present setting, we define the global cluster set for w = f(p) as follows;

A value w in the extended complex plane is called a cluster value at β if there exists a sequence 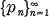in R converging to β such that

Type
Research Article
Information
Nagoya Mathematical Journal , Volume 70 , July 1978 , pp. 1 - 6
Copyright
Copyright © Editorial Board of Nagoya Mathematical Journal 1978

References

[1] Collingwood, E. F. and Cartwright, M. L.: Boundary theorems for a function meromorphic in the unit circle. Acta Math. 87 (1952), 83–146.Google Scholar
[2] Heins, M.: Riemann surfaces of infinite genus. Ann. of Math. 55(2) (1952), 296–317.Google Scholar
[3] Heins, M.: Lindelöfian maps. Ann. of Math. 62 (1955), 418–446.Google Scholar
[4] Kuroda, T.: On analytic functions on some Riemann surfaces. Nagoya Math. J. 10 (1956), 27–50.Google Scholar
[5] Myrberg, P.J.: Über die analytische Fortsetzung von beschränkten Funktionen. Ann. Acad. Sci. Fenn. A.I. 58 (1949).Google Scholar
[6] Noshiro, K.: Cluster Sets. Springer (1960).CrossRefGoogle Scholar
[7] Stoïlow, S.: Lecon sur les principes topologiques de la théorie des fonctions analytiques. Gauthier-Villars (1956).Google Scholar
[8] Stoïlow, S.: Sur la théorie topologique des recouvrements Riemanniens. Ann. Acad. Sci. Fenn. A.I. 250/35 (1958).Google Scholar
[9] Tsuji, M.: On non-prolongable Riemann surfaces. Proc. Imp. Acad. Japan 19 (1943).Google Scholar