Hostname: page-component-78c5997874-g7gxr Total loading time: 0 Render date: 2024-11-17T17:07:08.163Z Has data issue: false hasContentIssue false
  • English
  • Français

INVARIANCE OF CERTAIN PLURIGENERA FOR SURFACES IN MIXED CHARACTERISTICS

Part of: Birational geometry Surfaces and higher-dimensional varieties

Published online by Cambridge University Press:  16 January 2020

ANDREW EGBERT  and
CHRISTOPHER D. HACON
ANDREW EGBERT
Affiliation:
Department of Mathematics, University of Utah, Salt Lake City, UT84112, USA email [email protected]
CHRISTOPHER D. HACON
Affiliation:
Department of Mathematics, University of Utah, Salt Lake City, UT84112, USA email [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

We prove the deformation invariance of Kodaira dimension and of certain plurigenera and the existence of canonical models for log surfaces which are smooth over an integral Noetherian scheme 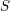$S$.

MSC classification

Primary: 14J10: Families, moduli, classification 14E30: Minimal model program (Mori theory, extremal rays)
Type
Article
Information
Nagoya Mathematical Journal , Volume 243 , September 2021 , pp. 1 - 10
Check for updates
Copyright
© 2020 Foundation Nagoya Mathematical Journal

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The second author was partially supported by NSF research grants no: DMS-1300750, DMS-1265285, by a grant from the Simons Foundation; Award Number: 256202 and by the Research Institute for Mathematical Sciences, a Joint Usage/Research Center located in Kyoto University. The authors are grateful to an anonymous referee for many useful suggestions.

References

Alexeev, V., Boundedness and K 2 for log surfaces, Internat. J. Math. 5(6) (1994), 779–810.CrossRefGoogle Scholar
Birkar, C., Cascini, P., Hacon, C. D. and McKernan, J., Existence of minimal models for varieties of log general type, J. Amer. Math. Soc. 23(2) (2010), 405–468.CrossRefGoogle Scholar
Birkar, C., Existence of flips and minimal models for 3-folds in char p, Ann. Sci. Éc. Norm. Supér. (4) 49(1) (2016), 169–212.CrossRefGoogle Scholar
Egbert, P. A., Log minimal models for arithmetic threefolds. Ph.D. Thesis, The University of Utah, 2016, 65 pp. ISBN: 978-1369-12426-2, ProQuest LLC.Google Scholar
Hacon, C. D. and Kovács, S., On the boundedness of slc surfaces of general type, Ann. Sc. Norm. Super. Pisa Cl. Sci. (5) 19(1) (2019), 191–215.Google Scholar
Hacon, C. D., McKernan, J. and Xu, C., Boundedness of moduli of varieties of general type, J. Eur. Math. Soc. (JEMS) 20(4) (2018), 865–901.CrossRefGoogle Scholar
Hacon, C. D. and Xu, C., Existence of log canonical closures, Invent. Math. 192(1) (2013), 161–195.10.1007/s00222-012-0409-0CrossRefGoogle Scholar
Hacon, C. D. and Xu, C., On the three dimensional minimal model program in positive characteristic, J. Amer. Math. Soc. 28(3) (2015), 711–744.CrossRefGoogle Scholar
Kawamata, Y., Semistable minimal models of threefolds in positive or mixed characteristic, J. Algebraic Geom. 3(3) (1994), 463–491.Google Scholar
Kollár, J. and Mori, S., Birational Geometry of Algebraic Varieties, Cambridge Tracts in Mathematics 134, Cambridge University Press, Cambridge, 1998, With the collaboration of C. H. Clemens and A. Corti, Translated from the 1998 Japanese original.10.1017/CBO9780511662560CrossRefGoogle Scholar
Katsura, T. and Ueno, K., On elliptic surfaces in characteristic p, Math. Ann. 272(3) (1985), 291–330.CrossRefGoogle Scholar
Lang, W., On Enriques surfaces in characteristic p. I, Math. Ann. 265(1) (1983), 45–65.CrossRefGoogle Scholar
Siu, Y.-T., Invariance of plurigenera, Invent. Math. 134(3) (1998), 661–673.10.1007/s002220050276CrossRefGoogle Scholar
Siu, Y.-T., Extension of twisted pluricanonical sections with plurisubharmonic weight and invariance of semipositively twisted plurigenera for manifolds not necessarily of general type, in Complex Geometry (Göttingen, 2000), Springer, Berlin, 2002, 223–277.10.1007/978-3-642-56202-0_15CrossRefGoogle Scholar
Suh, J., Plurigenera of general type surfaces in mixed characteristic, Compos. Math. 144(5) (2008), 1214–1226.10.1112/S0010437X08003527CrossRefGoogle Scholar
The Stacks project, https://stacks.math.columbia.edu, 2019.Google Scholar
Tanaka, H., Minimal models and abundance for positive characteristic log surfaces, Nagoya Math. J. 216 (2014), 1–70.10.1215/00277630-2801646CrossRefGoogle Scholar
Tanaka, H., The X-method for klt surfaces in positive characteristic, J. Algebraic Geom. 24(4) (2015), 605–628.CrossRefGoogle Scholar
Tanaka, H., Minimal model program for excellent surfaces, Ann. Inst. Fourier (Grenoble) 68(1) (2018), 345–376.CrossRefGoogle Scholar