Silicon-based quantum computer proposals

A review on the developments of silicon-based quantum computers was published recently.^[Reference Zwanenburg, Dzurak, Morello, Simmons, Hollenberg, Klimeck, Rogge, Coppersmith and Eriksson^16] This subsection provides a brief summary on the studies on Si-based quantum computers that require isotope engineering. Figure 1 shows two examples of silicon quantum computer proposals that use isotope engineering. The report by Kane [Fig. 1(a)] proposed to use the nuclear spins of phosphorus donors embedded in isotopically enriched ²⁸Si as qubits.^[Reference Kane^3] The phosphorus donors form a one-dimensional array with a separation of ~20 nm. The quantum information stored and processed by each ³¹P nuclear spin (I = 1/2) is read out by the tunneling current induced by the electron bound to ³¹P as it transports to the adjacent phosphorus donor. At the beginning of the calculation, each nuclear spin is initialized, so that they have identical nuclear spin states, m _s = 1/2 or −1/2. This can be achieved by reading out each nuclear spin state using the tunneling current and performing nuclear magnetic resonance (NMR) on the ³¹P qubits to align them into the correct state when their initial state is incorrect. No action is taken if the ³¹P qubit is already in the correct state. NMR is also used for the quantum logic operation of the ³¹P qubits. Qubit selectivity is achieved by applying an electronic bias to a gate that is placed immediately above each qubit (A-gate). This causes the ³¹P to be either in or out of resonance with the frequency employed during the NMR. The two-qubit interactions are switched on and off by electric gates placed between the neighboring qubits (J-gates). The electrons bound to the phosphorus donors mediate the ³¹P–³¹P nuclear spin interactions between the adjacent qubits. The nuclear spin state for each ³¹P qubit is read out at the end of the calculation by detecting the single-electron tunneling current using a single-electron transistor (SET). Kane's vision has triggered many proposals that use the electron spins in donors imbedded in silicon as qubits.^[Reference Vrijen, Yablonovitch, Wang, Jiang, Balandin, Roychowdhury, Mor and DiVincenzo^17–Reference Hollenberg, Greentree, Fowler and Wellard^19]

Figure 1. Two examples of silicon quantum computer schemes using isotope engineering. (a) Phosphorus donor nuclear spin qubits^[3] and (b) ²⁹Si nuclear spin qubits.^[6]

The second class of silicon-based quantum computation studies uses ²⁹Si nuclear spins as qubits.^[Reference Ladd, Goldman, Yamaguchi, Yamamoto, Abe and Itoh^4–Reference Itoh^6]Fig. 1(b) shows the one-dimensional array of the ²⁹Si nuclear spins formed on an isotopically enriched nuclear-spin-free ²⁸Si substrate.^[Reference Itoh^6] Here, the dipolar coupling between adjacent ²⁹Si nuclear spins is used for two-qubit operations and the qubit selectivity is ensured by the large magnetic field gradient induced by a small magnet placed near the array. Readout of each qubit is achieved by shuttling the state to the end of the chain by a sequence of swap operations between the adjacent qubits. The ²⁹Si nuclear spin state at the end of the chain was read out from the electron in the phosphorus atom that is placed next to the ²⁹Si atom.

The third class of silicon-based quantum computing studies uses the electrons in quantum dots as qubits.^[Reference Friesen, Rugheimer, Savage, Lagally, van der Weide, Joynt and Eriksson^20–Reference Shi, Simmons, Prance, Gamble, Koh, Shim, Hu, Savage, Lagally, Eriksson, Friesen and Coppersmith^22] A single-electron spin is confined in each quantum dot and is employed as a qubit. The qubit state is described by either the spin state of electrons in a dot or a single electron within a pair of two quantum dots.^[Reference Zwanenburg, Dzurak, Morello, Simmons, Hollenberg, Klimeck, Rogge, Coppersmith and Eriksson^16]

Isotopically engineered silicon for quantum computation

Naturally available silicon (^natSi) is composed of 92.2% ²⁸Si, 4.7% ²⁹Si, and 3.1% ³⁰Si. These stable isotopes can be separated by centrifuging SiF₄ gas.^[Reference Itoh, Kato, Uemura, Kaliteevski, Godisov, Devyatych, Bulanov, Gusev, Kovalev, Sennikov, Pohl, Abrosimov and Riemann^23, Reference Sennikov, Vodopyanov, Golubev, Mansfeld, Drozdov, Drozdov, Andreev, Gavrilenko, Pryakhin, Shashkin, Godisov, Glasunov, Safonov, Pohl, Thewalt, Becker, Riemann, Abrosimov and Valkiers^24] The first isotopically engineered silicon was produced in 1958 to characterize the electron spin resonance (ESR) of donors in silicon.^[Reference Gordon and Bowers^25] Since then, no results on this topic were reported until a strong interest emerged in the mid-1990s, when isotopically enriched ²⁸Si single crystals were employed as the standard to define the Avogadro constant.^[Reference Becker, Bettin, Debievre, Holm, Kutgens, Spieweck, Stumpel, Valkiers and Zulehner^26–Reference Becker, Pohl, Riemann and Abrosimov^28] The goal was to produce silicon crystals with a well-defined number of Si atoms per unit volume and to establish the definition of the kilogram by the mass of a given number of silicon atoms. Silicon was chosen because there had been extensive industrial efforts in Si electronics. Silicon has unsurpassed crystalline quality and chemical purity in comparison with other crystalline materials. Isotope enrichment with the stable isotopes is needed because the Si–Si bond length depends on the combination of isotopes.^[Reference Sozontov, Cao, Kazimirov, Kohn, Konuma, Cardona and Zegenhagen^29, Reference Yang, Steger, Lian, Thewalt, Uemura, Sagara, Itoh, Haller, Ager, Lyon, Konuma and Cardona^30] Fluctuations in the bond lengths for different isotopes must be removed to obtain a well-defined number of Si atoms per unit volume. The floating-zone growth performed at the Leibniz Institute for Crystal Growth (IKZ) in Berlin resulted in a 5-kg ²⁸Si single crystal of 99.99% isotopically enriched silicon with <10¹⁵ cm⁻³ background carbon content, <10¹³ cm⁻³ electrically active impurities such as boron and phosphorus, and was free of dislocations.^[Reference Becker, Schiel, Pohl, Kaliteevski, Godisov, Churbanov, Devyatykh, Gusev, Bulanov, Adamchik, Gavva, Kovalev, Abrosimov, Hallmann-Seiffert, Riemann, Valkiers, Taylor, De Bievre and Dianov^31, Reference Becker^32] The silicon crystals fabricated for the Avogadro measurement were shaped into precise spheres and the sawn-off pieces generated during the shaping process were used to perform basic characterization on the crystal. Some pieces were also used in some of the silicon quantum information research described below. Herein, these samples will be referred to as the “Avogadro samples”. They had the highest isotopic and chemical purity and the best crystalline quality among all of the isotopically enriched silicon crystals ever produced.

The second group of isotopically enriched, bulk Si crystals was also grown at the IKZ and will be referred to as the “IKZ crystals”. IKZ established various Czochralski^[Reference Itoh, Kato, Uemura, Kaliteevski, Godisov, Devyatych, Bulanov, Gusev, Kovalev, Sennikov, Pohl, Abrosimov and Riemann^23, Reference Bulanov, Devyatych, Gusev, Sennikov, Pohl, Riemann, Schilling and Becker^33] and floating-zone^[Reference Ager, Beeman, Hansen, Haller, Sharp, Liao, Yang, Thewalt and Riemann^34] growth techniques to maintain the isotopic composition of the starting charge. Here various isotopes (²⁸Si, ²⁹Si, and ³⁰Si) of different enrichments were employed as starting charges as described in Ref. Reference Itoh, Kato, Uemura, Kaliteevski, Godisov, Devyatych, Bulanov, Gusev, Kovalev, Sennikov, Pohl, Abrosimov and Riemann23. They also developed a method to dope the crystal with the desired group V donors during its growth. A variety of dislocation-free, isotopically enriched ²⁸Si, ²⁹Si, and ³⁰Si crystals were grown at IKZ to fulfill orders placed by the Simon Fraser University, Canada,^[Reference Thewalt, Steger, Yang, Stavrias, Cardona, Riemann, Abrosimov, Churbanov, Gusev, Bulanov, Kovalev, Kaliteevskii, Godisov, Becker, Pohl, Ager and Haller^35, Reference Steger, Yang, Stavrias, Thewalt, Riemann, Abrosimov, Churbanov, Gusev, Bulanov, Kovalev, Kaliteevskii, Godisov, Becker and Pohl^36] the Keio University, Japan,^[Reference Itoh, Kato, Uemura, Kaliteevski, Godisov, Devyatych, Bulanov, Gusev, Kovalev, Sennikov, Pohl, Abrosimov and Riemann^23, Reference Kato, Itoh, Yamada-Kaneta and Pohl^37] and the University of California (UC), Berkeley, CA, USA.^[Reference Ager, Beeman, Hansen, Haller, Sharp, Liao, Yang, Thewalt and Riemann^34, Reference Morton, Tyryshkin, Brown, Shankar, Lovett, Ardavan, Schenkel, Haller, Ager and Lyon^38]

The third group of isotopically enriched, bulk Si crystals was grown at Keio University, Japan and will be referred to as the “Keio crystals”. The floating-zone growth machine at Keio was designed to grow small crystals (<7 mm diameter) of high crystalline quality and chemical purity.^[Reference Takyu, Itoh, Oka, Saito and Ozhogin^39] The machine is suited to grow isotopically mixed crystals with arbitrary compositions of ²⁸Si, ²⁹Si, and ³⁰Si. Isotopically mixed bulk Si crystals have been produced exclusively at Keio University.^[Reference Abe, Itoh, Isoya and Yamasaki^40]

The isotopically enriched ²⁸Si chemical vapor deposition (CVD) films were grown from isotopically enriched silane (²⁸SiH₄) gas by the Isonics Corporation, USA, from 1998 to 2005. Because centrifuge isotope separation was performed using SiF₄ as described elsewhere ²³, the resulting isotopically enriched ²⁸SiF₄ was transformed to ²⁸SiH₄ (silane) by an electronic materials company named Voltaix. The quality of the Isonics epilayers, especially the residual impurity concentration, fluctuated significantly between the different epilayers because the company outsourced the CVD growth to other companies. Therefore, obtaining quantum-information-research-grade ²⁸Si CVD wafers, including ²⁸Si on insulator (SOI) wafers,^[Reference Lo, Persaud, Dhuey, Olynick, Borondics, Martin, Bechtel, Bokor and Schenkel^41] required in-depth discussions with Isonics to establish appropriate fabrication procedures. High quality, Isonics ²⁸Si ep-wafers employed in single-qubit experiments (the section “Single-spin qubits in silicon”) have been grown at Lawrence Semiconductor Research Laboratory, Inc. The Isonics ²⁸Si CVD epi-layers have the isotopic compositions ²⁸Si (~99.924%), ²⁹Si (~0.073%), and ³⁰Si (~0.003%).

Isotopically enriched, strained ²⁸Si thin layers were grown by solid-source molecular beam epitaxy (MBE) at the Technical University of Munich (TUM), Germany^[Reference Sailer, Lang, Abstreiter, Tsuchiya, Itoh, Ager, Haller, Kupidura, Harbusch, Ludwig and Bougeard^42] and by CVD at Princeton University, USA.^[Reference Li, Huang, Rokhinson and Sturm^43] Some electron double-quantum dots were fabricated using the strained silicon.^[Reference Wild, Kierig, Sailer, Ager, Haller, Abstreiter, Ludwig and Bougeard^44] Other isotopically controlled silicon low-dimensional structures, typically grown by MBE,^[Reference Kojima, Nebashi, Itoh and Shiraki^45–Reference Bracht, Wehmeier, Eon, Plech, Issenmann, Hansen, Larsen, Ager and Haller^48] have been employed, predominantly for diffusion^[Reference Bracht, Haller and Clark-Phelps^49–Reference Shimizu, Uematsu, Itoh, Takano, Sawano and Shiraki^52] and amorphization^[Reference Shimizu, Uematsu, Itoh, Takano, Sawano and Shiraki^53] studies.

Proof-of-concept experiments with spin ensembles in isotopically engineered silicon

A qubit must be made of a single quantum. However, one can prepare an ensemble of identical qubits, initialize them in the same quantum states, manipulate them together quantum mechanically (perform quantum calculations), and read them out all at once to obtain the results expected for single quantum bit operation. To demonstrate this, phosphorus donors in silicon were used. Figure 2(a) shows two phosphorus donors placed in ^natSi. Each phosphorus atom has an electron spin for the bound electron and the ³¹P nuclear spin in the nucleus; it has two qubits. This was used to determine whether the two phosphorus donors in Fig. 2(a) are quantum mechanically identical. They are not because of the coexistence of ²⁸Si, ²⁹Si, and ³⁰Si stable isotopes and their random distribution in ^natSi, and the silicon isotope configuration around each phosphorus donor differed from those of others. Additionally, the mass difference between the stable isotopes changes the bond lengths (the local lattice strain), leading to perturbation of the hyperfine interactions between the electron spin and the ³¹P nuclear spin in each phosphorus atom. This could lead to a shift in the NMR frequencies of the ³¹P between different donors, making each phosphorus atom distinguishable and the ensemble of phosphorus donors would not be able to be manipulated all together. Moreover, there was a random distribution of ²⁹Si that changes the local magnetic field near each phosphorus donor. This leads to even larger changes in the ³¹P NMR and electron paramagnetic resonance (EPR) frequencies than those caused by the mass fluctuations. Therefore, the silicon matrix must be composed of a single type of stable isotopes with no nuclear spins, either ²⁸Si or ³⁰Si. Figure 2(b) shows two phosphorus donors placed in an isotopically enriched, ²⁸Si single crystal. The two phosphorus donors could be considered identical if the separation between them was long enough to have small interactions. This changes their EPR frequencies, where their quantum states could be initialized to be the same such that the electron and nuclear spins were set in the up (or down) state in every donor. By using an ensemble of identical phosphorus donors, one can start from the same quantum states, perform the same quantum calculation using every donor in parallel and read them all out together to obtain the result from the quantum calculation that is expected for a single phosphorus donor qubit. Therefore, isotope enrichment is a powerful and necessary tool to allow for proof-of-concept quantum computer experiments with an ensemble of identical qubits in silicon to be carried out.

Figure 2. Schematic representations of (a) quantum mechanically different phosphorus donors placed in ^natSi and (b) quantum mechanically identical phosphorus donors placed in isotopically enriched ²⁸Si.

One important issue yet to be mentioned thus far is the coherence time (T ₂) of the qubits. If the coherence time (the quantum information storage time) is too short, particularly shorter than the total time required for completing the desired quantum computation routine, the quantum computer will lose information in the middle of the calculation. Because a high crystalline quality and low concentration of residual impurities can be achieved with silicon, the major decoherence source of the electron and nuclear spins in silicon has been assumed to be caused by magnetic field fluctuations arising from the flip-flops of the ²⁹Si nuclear spins in the matrix.^[Reference Chiba and Hirai^54] The mass variations in the isotopes also change the local lattice strain to vary the spin manipulation frequencies. However, they are static and have little effect on the coherence time. Therefore, the first step was to understand the decoherence mechanisms of the electron and nuclear spins in silicon as a function of the host ²⁹Si contents. An example of this is a series of pulsed EPR measurements performed on an ensemble of phosphorus donors using the Keio bulk crystals in which the ²⁹Si fraction was deliberately changed.^[Reference Abe, Itoh, Isoya and Yamasaki^40, Reference Abe, Isoya and Itoh^55–Reference Abe, Tyryshkin, Tojo, Morton, Witzel, Fujimoto, Ager, Haller, Isoya, Lyon, Thewalt and Itoh^57] The spin coherence time of the phosphorus-bound electron and its interaction with the precessing ²⁹Si nuclear spins in the neighboring atoms [electron spin echo envelope modulation (ESEEM)] was determined experimentally and compared with the theory^[Reference Witzel, Hu and Sarma^58–Reference de Sousa and Das Sarma^60] to achieve a solid understanding of the decoherence mechanisms. The most important issue associated with quantum computation is how far the phosphorus coherence time can be extended by eliminating ²⁹Si from the matrix. The first experiment of this type was performed by Gordon and Bowers in 1958 using an isotopically enriched, ²⁸Si bulk single crystal. However, no significant improvement in the coherence was obtained because it was limited by the interactions between the donor electrons, caused by the relatively high concentration of phosphorus.^[Reference Gordon and Bowers^25] More recently, in 2003, an extended coherence time of the electron spins bound to the phosphorus donors in isotopically enriched, Isonics ²⁸Si epilayers was reported by the Princeton University group.^[Reference Tyryshkin, Lyon, Astashkin and Raitsimring^61] The coherence was improved even further in 2011 by the international collaboration headed by the same group using the IKZ ²⁸Si bulk crystals.^[Reference Tyryshkin, Tojo, Morton, Riemann, Abrosimov, Becker, Pohl, Schenkel, Thewalt, Itoh and Lyon^62] The study achieved electron coherence times exceeding seconds. This time is long enough to perform a quantum error correction protocol that, in principle, can store information forever. The dominant decoherence mechanisms were recorded as a function of temperature.^[Reference Tyryshkin, Tojo, Morton, Riemann, Abrosimov, Becker, Pohl, Schenkel, Thewalt, Itoh and Lyon^62] Because the ³¹P nuclear spins couple to the electron spins bound to phosphorus, the nuclear spin coherence can be affected by the presence of ²⁹Si through hyperfine interactions with the electron spins. Therefore, the research team headed by the Simon Fraser University used the isotopically enriched, ²⁸Si Avogadro crystal to probe the coherence time of the ³¹P nuclear spins in neutral^[Reference Steger, Saeedi, Thewalt, Morton, Riemann, Abrosimov, Becker and Pohl^63] and ionized^[Reference Saeedi, Simmons, Salvail, Dluhy, Riemann, Abrosimov, Becker, Pohl, Morton and Thewalt^64] donors. Thanks to the removal of the background ²⁹Si nuclear spins, coherence time of the ³¹P nuclear spin in its neutral state at the cryogenic temperature exceeded 180 s, ^[Reference Steger, Saeedi, Thewalt, Morton, Riemann, Abrosimov, Becker and Pohl^63] while that of ionized donors at room and cryogenic temperatures exceeded 39 min and 3 h, respectively.^[Reference Saeedi, Simmons, Salvail, Dluhy, Riemann, Abrosimov, Becker, Pohl, Morton and Thewalt^64] The important finding here was that the coherence times of not only the electron but also the nuclear spins of the phosphorus donors in silicon were extended by eliminating the ²⁹Si nuclear spins through isotope engineering. These coherence times are more than sufficient for practical application in quantum computing.

Following the success of phosphorus in isotopically enriched ²⁸Si, the group headed by the Simon Fraser University and University College London investigated other shallow donors, focusing on bismuth in isotopically enriched ²⁸Si.^[Reference Wolfowicz, Simmons, Tyryshkin, George, Riemann, Abrosimov, Becker, Pohl, Lyon, Thewalt and Morton^65, Reference Wolfowicz, Tyryshkin, George, Riemann, Abrosimov, Becker, Pohl, Thewalt, Lyon and Morton^66] The coherence times of the bismuth electron spins were as long as those of the phosphorus atoms.^[Reference Wolfowicz, Simmons, Tyryshkin, George, Riemann, Abrosimov, Becker, Pohl, Lyon, Thewalt and Morton^65] The existence of the optimal magnetic field (atomic clock transition), at which the electron decoherence was suppressed by its insensitivity to the external magnetic field perturbation was demonstrated.^[Reference Wolfowicz, Tyryshkin, George, Riemann, Abrosimov, Becker, Pohl, Thewalt, Lyon and Morton^66] The Keio University group investigated the hyperfine clock transition of bismuth using the magnetic field at which the resonant frequency was insensitive to fluctuations in the hyperfine constant in isotopically enriched ²⁸Si^[Reference Mortemousque, Berger, Sekiguchi, Culan, Elliman and Itoh^67] using the spin-dependent-recombination EPR technique developed especially for this purpose.^[Reference Mortemousque, Sekiguchi, Culan, Vlasenko, Elliman, Vlasenko and Itoh^68]

The decoherence and decoupling mechanisms for the ²⁹Si nuclear spins as qubits were investigated with bulk ^natSi and isotopically enriched IKZ ²⁹Si at Stanford University, USA and Keio University, Japan.^[Reference Ladd, Maryenko, Yamamoto, Abe and Itoh^69] The decoupling pulse sequences were used to extend the ²⁹Si coherence time up to 20 s and beyond at room temperature.^[Reference Ladd, Maryenko, Yamamoto, Abe and Itoh^69]

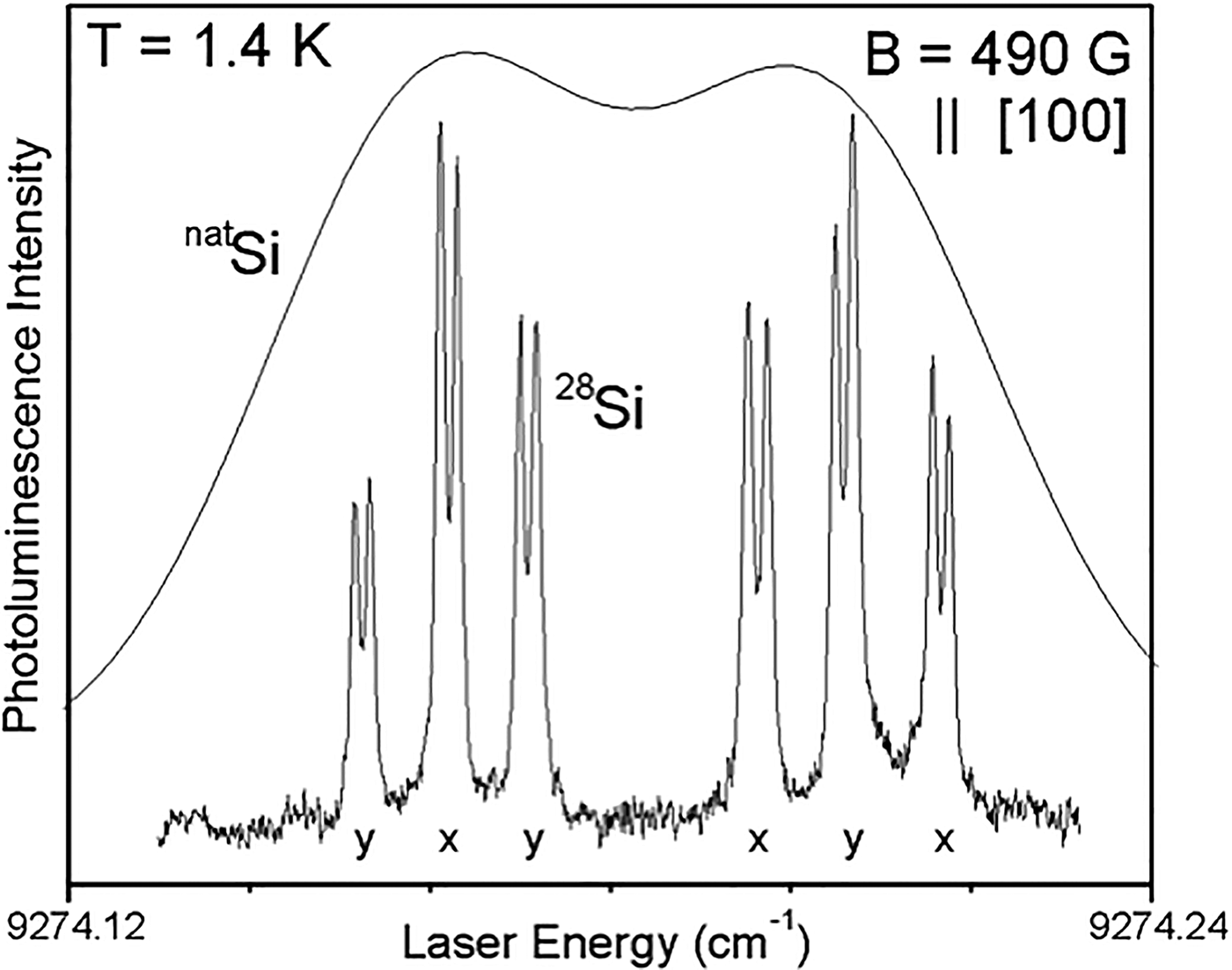
Confirmation of long-enough coherence times in various electron and nuclear spins in silicon encouraged researchers to develop qubit initialization schemes. Although initialization of the electron spins can be achieved by reducing the sample temperature and increasing the externally applied magnetic field to make the thermal energy much smaller than the Zeeman split electronic levels,^[Reference Morton, Tyryshkin, Brown, Shankar, Lovett, Ardavan, Schenkel, Haller, Ager and Lyon^38, Reference Simmons, Brown, Riemann, Abrosimov, Becker, Pohl, Thewalt, Itoh and Morton^70] initialization of the nuclear spins is not straightforward. This is because of the extremely small gyromagnetic ratios of the nuclear spins that do not allow a large-enough Zeeman energy separation with respect to the thermal energy, even with an externally applied magnetic field ~10 T at a temperature of ~1 K. Therefore, it was necessary to develop a method to polarize the nuclear spins in silicon using reasonable experimental conditions. Again, isotope engineering was proven useful for such purposes. The international collaboration led by Simon Fraser University developed an all-optical NMR of ³¹P using the Avogadro bulk ²⁸Si samples.^[Reference Yang, Steger, Karaiskaj, Thewalt, Cardona, Itoh, Riemann, Abrosimov, Churbanov, Gusev, Bulanov, Kaliteevskii, Godisov, Becker, Pohl, Ager and Haller^71] When the inhomogeneous broadening inherent in natural Si was eliminated using enriched ²⁸Si, the photoluminescence excitation (PLE) spectrum of the ensemble of phosphorus-bound excitons revealed a well-resolved hyperfine splitting resulting from the ³¹P nuclear spin (Fig. 3).^[Reference Yang, Steger, Karaiskaj, Thewalt, Cardona, Itoh, Riemann, Abrosimov, Churbanov, Gusev, Bulanov, Kaliteevskii, Godisov, Becker, Pohl, Ager and Haller^71–Reference Thewalt, Yang, Steger, Karaiskaj, Cardona, Riemann, Abrosimov, Gusev, Bulanov, Kovalev, Kaliteevskii, Godisov, Becker, Pohl, Haller, Ager and Itoh^73] Furthermore, introducing one more laser irradiation at one of the phosphorus PLE peak frequencies, corresponding to the ³¹P nuclear spin up or down, led to extremely fast (~0.1 s) electron and nuclear polarizations of 90 and 76%, respectively.^[Reference Yang, Steger, Sekiguchi, Thewalt, Ladd, Itoh, Riemann, Abrosimov, Becker and Pohl^74] This fast initialization and nuclear spin readout technique was employed to demonstrate the long ³¹P nuclear coherence times reported in Refs. Reference Steger, Saeedi, Thewalt, Morton, Riemann, Abrosimov, Becker and Pohl63, Reference Saeedi, Simmons, Salvail, Dluhy, Riemann, Abrosimov, Becker, Pohl, Morton and Thewalt64. Using isotopically enriched ²⁸Si device structures, it was demonstrated that all-electrical ³¹P nuclear polarization was possible.^[Reference Lo, Weis, van Tol, Bokor and Schenkel^75] Inhomogeneous broadening of the bismuth-bound exciton photoluminescence peaks were also dominated by variations in the masses of the silicon isotopes.^[Reference Sekiguchi, Steger, Saeedi, Thewalt, Riemann, Abrosimov and Notzel^76]28Si enrichment allowed for the ²⁰⁹Bi nuclear spin peaks to be clearly observed.^[Reference Saeedi Ilkhchy, Steger, Thewalt, Abrosimov, Riemann, Becker and Pohl^77]

Figure 3. Comparison of the PLE spectra for an ensemble of phosphorus-bound excitons in ^natSi and isotopically enriched ²⁸Si. The spectrum of ^natSi is significantly broader because of the random distribution of the three stable isotopes, while that of ²⁸Si has sharp doublet features, corresponding to the hyperfine splittings due to the ³¹P nuclear spins.^[71]

Achieving a large polarization for the ²⁹Si nuclear spins in silicon was challenging because, unlike donor nuclear spins, there is no electron spin that couples strongly to the ²⁹Si at the substitutional lattice sites. One approach taken by the international collaboration led by Keio University was to enrich the phosphorus doped single silicon crystal with ²⁹Si to reduce the average distance between the phosphorus electrons and the ²⁹Si nuclear spins.^[Reference Verhulst, Maryenko, Yamamoto and Itoh^78–Reference Hayashi, Itahashi, Itoh, Vlasenko and Vlasenko^81] In this phenomenon, referred to as dynamical nuclear polarization, selective excitation of the symmetry prohibited ESR transitions in the phosphorus donor coupling to the nearby ²⁹Si, led to preferential relaxation to a certain ²⁹Si nuclear spin state (solid effect). This nuclear spin polarization around the phosphorus atom diffused through the crystal via nuclear spin diffusion (²⁹Si–²⁹Si flip-flops). However, this process is slow (>1 h) and the highest ²⁹Si polarization achieved was only ~4%.^[Reference Hayashi, Itahashi, Itoh, Vlasenko and Vlasenko^81] An efficient method would be to prepare electron spins that couple more directly to the ²⁹Si nuclear spins, similar to the way that an electron bound to a phosphorus atom couples strongly to the ³¹P nuclear spin. To achieve this condition, the group led by Keio University employed vacancy-oxygen complexes in silicon that had ²⁹Si nearest neighbors.^[Reference Akhtar, Morishita, Sawano, Shiraki, Vlasenko and Itoh^82–Reference Akhtar, Sekiguchi, Itahashi, Filidou, Morton, Vlasenko and Itoh^84] This defect is unique as it can be excited into the spin triplet state to achieve large electron spin polarization that can be transferred to the nearby ²⁹Si nuclear spins. This allowed a very large, most likely >90%, polarization of the ²⁹Si nuclear spins.^[Reference Akhtar, Filidou, Sekiguchi, Kawakami, Itahashi, Vlasenko, Morton and Itoh^83]

Taking the advantages of the long coherence time and the absence of inhomogeneous broadening in the phosphorus ESR and electron nuclear double resonance (ENDOR) lines for the phosphorus donors in isotopically enriched IKZ bulk ²⁸Si, the international collaboration led by John Morton (formally at University of Oxford and presently at University College London) succeeded in a series of proof-of-concept two-qubit experiments. Here, one qubit was the electron spin of the phosphorus atom and the other was the ³¹P nuclear spin. By sharpening the electron ESR and ³¹P ENDOR transitions in ²⁸Si, the quantum manipulation fidelity of the ensemble of phosphorus donor states was improved significantly. Additionally, eliminating the ²⁹Si nuclear spins from the matrix provided enough coherence time to complete the operation and readout procedures, such as the coherent transfer of the quantum states,^[Reference Morton, Tyryshkin, Brown, Shankar, Lovett, Ardavan, Schenkel, Haller, Ager and Lyon^38] and create and detect the two-qubit entanglement^[Reference Simmons, Brown, Riemann, Abrosimov, Becker, Pohl, Thewalt, Itoh and Morton^70] between the phosphorus electron and ³¹P nuclear spins. The same team also succeeded in employing an ensemble of phosphorus electron spins to store and retrieve multiple microwave pulses, demonstrating the basic operation of the so-called quantum holographic memory^[Reference Wu, George, Wesenberg, Molmer, Schuster, Schoelkopf, Itoh, Ardavan, Morton and Briggs^85] and the geometric phase gate operation of the phosphorus electron spins for fault-tolerant quantum computation.^[Reference Wu, Gauger, George, Mottonen, Riemann, Abrosimov, Becker, Pohl, Itoh, Thewalt and Morton^86] They also performed fundamental experiments testing the nature of the quantum superposition states.^[Reference Knee, Simmons, Gauger, Morton, Riemann, Abrosimov, Becker, Pohl, Itoh, Thewalt, Briggs and Benjamin^87] In parallel, the Keio–UCL collaboration demonstrated coherent transfer between the electron spin states and ²⁹Si nuclear spin states for two-qubit operations.^[Reference Akhtar, Filidou, Sekiguchi, Kawakami, Itahashi, Vlasenko, Morton and Itoh^83] These accomplishments, obtained with ensembles of donor electron spins, donor nuclear spins, and ²⁹Si nuclear spins in silicon formed the foundation of single-bit quantum information processing (QIP) in silicon, which will be discussed in the next section.

The properties of ensembles containing boron accepters were studied using IKZ bulk ²⁸Si single crystals. It is well known that the ESR spectra of acceptors are broadened by the existence of the random strains that lift the degeneracy of the light- and heavy-hole bands from one acceptor to another by different amounts. However, the main source of random strain has not been identified. The group led by Keio University and TUM identified that the source of strain was the random distribution of the three stable isotopes in ^natSi. They observed significant narrowing of the boron ESR lines in ²⁸Si.^[Reference Tezuka, Stegner, Tyryshkin, Shankar, Thewalt, Lyon, Itoh and Brandt^88, Reference Stegner, Tezuka, Andlauer, Stutzmann, Thewalt, Brandt and Itoh^89] However, the coherence time of the holes bound to the boron acceptors were limited by the short-hole relaxation time (T ₁), even at 3 K. Further cooling is needed to extend the T ₁ and T ₂ for the application of boron acceptors in quantum information processing. Additionally, the properties of the donors have been studied in isotopically enriched, ²⁸Si device structures. The group led by Berkeley investigated the spin-dependent scattering in ²⁸Si field-effect transistors.^[Reference Lo, Bokor, Schenkel, Tyryshkin and Lyon^90] The formation of ²⁹Si nuclear spin wires was also attempted by templating a silicon substrate,^[Reference Yoshida, Sekiguchi and Itoh^91] followed by forcing the alignment of MBE-deposited Si atoms.^[Reference Sekiguchi, Yoshida and Itoh^92] However, a ²⁹Si device is yet to be realized.

Single-spin qubits in silicon

The most important steps toward realizing a silicon-based quantum computer are to prepare, operate, and readout a single charge (or double-quantum dot) qubit or single electron and/or nuclear spin qubit placed in a silicon matrix. While, truly single qubit operations in silicon have been reported in the past few years,^[Reference Maune, Borselli, Huang, Ladd, Deelman, Holabird, Kiselev, Alvarado-Rodriguez, Ross, Schmitz, Sokolich, Watson, Gyure and Hunter^93–Reference Shi, Simmons, Ward, Prance, Mohr, Koh, Gamble, Wu, Savage, Lagally, Friesen, Coppersmith and Eriksson^96] it was only this year that the isotope engineering of silicon was proven essential, even for single qubits in silicon.^[Reference Muhonen, Dehollain, Laucht, Hudson, Karla, Sekiguchi, Itoh, Jamieson, McCallum, Dzurak and Morello^97, Reference Veldhorst, Hwang, Yang, Leenstra, de Ronde, Dehollain, Muhonen, Hudson, Itoh, Morello and Dzurak^98] For a single qubit, such as a single donor, a single quantum dot or a single double-quantum dot, a variety of foreign materials and structures, including gate insulators, metal contacts, and SETs for readouts should be placed nearby. Therefore, unlike the ensemble of isolated donors described in the previous section whose coherence was limited by the presence of ²⁹Si nuclear spins in ^natSi, the common assumption was that purifying the isotope to eliminate ²⁹Si would not be effective in extending the coherence time because the major decoherence sources would come from foreign objects rather than the ²⁹Si nuclear spin fluctuations. Despite such skepticism, the “let us try and see” approach employed by experimentalists has shown that isotope enrichment is essential to successfully fabricate single-donor spin qubits^[Reference Muhonen, Dehollain, Laucht, Hudson, Karla, Sekiguchi, Itoh, Jamieson, McCallum, Dzurak and Morello^97] and single quantum-dot qubits^[Reference Veldhorst, Hwang, Yang, Leenstra, de Ronde, Dehollain, Muhonen, Hudson, Itoh, Morello and Dzurak^98] in silicon.

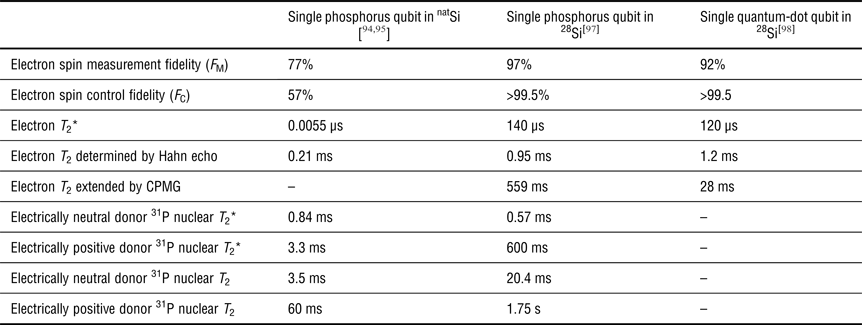
Figure 4 shows a scanning electron microscope (SEM) image of the single phosphorus device developed by the group led by Andera Morello and Andrew Dzurak at the University of New South Wales (UNSW). This device allowed, not only initialization, operation, and readout of the quantum information stored in a single-electron qubit and the single ³¹P nuclear qubit associated with the single phosphorus atom, but also coherent transfer between the electron and nuclear spin qubits. The device structure was essentially the same as the one developed by the same group using a ^natSi substrate, demonstrating single-electron spin^[Reference Pla, Tan, Dehollain, Lim, Morton, Jamieson, Dzurak and Morello^94] and nuclear spin^[Reference Pla, Tan, Dehollain, Lim, Morton, Zwanenburg, Jamieson, Dzurak and Morello^95] qubits. Figures 5(a) and 5(b) compare the Rabi oscillations of the single phosphorus qubits in ^natSi^[Reference Pla, Tan, Dehollain, Lim, Morton, Jamieson, Dzurak and Morello^94] and ²⁸Si, respectively.^[Reference Muhonen, Dehollain, Laucht, Hudson, Karla, Sekiguchi, Itoh, Jamieson, McCallum, Dzurak and Morello^97] The single-electron spin qubit placed near the surface had a much longer coherence (T ₂^*) when isotopically enriched ²⁸Si was employed. In fact, every aspect of the qubit properties were significantly improved in the ²⁸Si wafer (summarized in Table I).

Figure 4. SEM image of the single phosphorus spin qubit device developed at UNSW. The red spin indicates where the single phosphorus qubit is placed.^[97]

Figure 5. Comparison of the Rabi oscillations for the single phosphorus qubit placed in (a) ^natSi^[94] and (b) isotopically enriched ²⁸Si.^[97]

Table I. Comparison of the fidelities and coherence times of phosphorus-donor and quantum-dot qubits in naturally available silicon and isotopically enriched ²⁸Si epilayers.

Isotope engineering was also proven to be highly beneficial for the single-electron spin quantum-dot qubits in the ²⁸Si samples fabricated and measured by the same group at UNSW.^[Reference Veldhorst, Hwang, Yang, Leenstra, de Ronde, Dehollain, Muhonen, Hudson, Itoh, Morello and Dzurak^98]Figure 6 shows the device structure and the Rabi oscillations of the electrons. Table I summarizes the coherence times that are orders of the magnitude longer than other quantum-dot qubits. Moreover, the ESR linewidth of the quantum-dot qubit was only 2.4 kHz and its resonant frequency could easily be shifted by more than 3000 times the linewidth by tuning the gate voltage. This ensured the selectivity to specific qubits integrated in large-scale systems, which could be fabricated using the standard silicon complementary metal-oxide-semiconductor (CMOS) technologies.

Figure 6. (a) SEM image of a gate-defined single-electron quantum-dot qubit in silicon. (b) Rabi oscillations for a single-electron spin confined in a quantum dot.^[98]

Developments in the design of quantum mechanically coupled, multiple phosphorus qubits and quantum dots are required for future large-scale integration. The two experiments described in this section showed that using isotope engineering to eliminate the background nuclear spins is crucial to extend the coherence time to a useful level. It was also shown in Fig. 2 of Ref. Reference Laucht, Hill and Morello99 that the isotope enrichment is needed to improve the gate fidelity. Overall, single qubits in ²⁸Si have greatly surpassed the properties of the other single qubits tested in semiconductor systems.

Diamond quantum sensing

Diamond has been identified as one of the ideal matrixes for use in solid-state QIP^[Reference Shahriar, Hemmer, Lloyd, Bhatia and Craig^100] when ESR of a single NV center was realized.^[Reference Gruber, Drabenstedt, Tietz, Fleury, Wrachtrup and vonBorczyskowski^101] The group from the University of Stuttgart, Germany performed a series of pioneering works on the qubit operations of single NV centers at room temperature.^[Reference Jelezko, Popa, Gruber, Tietz, Wrachtrup, Nizovtsev and Kilin^7, Reference Jelezko, Gaebel, Popa, Domhan, Gruber and Wrachtrup^9, Reference Jelezko, Tietz, Gruber, Popa, Nizovtsev, Kilin and Wrachtrup^102, Reference Jelezko and Wrachtrup^103] Their ground-breaking works were extended to a variety of applications of single NV centers in QIP.^[Reference Childress, Dutt, Taylor, Zibrov, Jelezko, Wrachtrup, Hemmer and Lukin^104–Reference Dolde, Jakobi, Naydenov, Zhao, Pezzagna, Trautmann, Meijer, Neumann, Jelezko and Wrachtrup^109] In parallel, studies on how to use quantum phenomena in metrology and sensing emerged, aiming to reach sensitivities beyond the limit imposed by classical physics.^[Reference Giovannetti, Lloyd and Maccone^110, Reference Giovannetti, Lloyd and Maccone^111] While discussions continue on the ultimate limit of quantum sensing,^[Reference Joo, Munro and Spiller^112–Reference Cappellaro, Goldstein, Hodges, Jiang, Maze, Sorensen and Lukin^116] the use of single-electron spins bound to single NV centers in diamond has been proposed.^[Reference Degen^11, Reference Taylor, Cappellaro, Childress, Jiang, Budker, Hemmer, Yacoby, Walsworth and Lukin^12, Reference Balasubramanian, Chan, Kolesov, Al-Hmoud, Tisler, Shin, Kim, Wojcik, Hemmer, Krueger, Hanke, Leitenstorfer, Bratschitsch, Jelezko and Wrachtrup^14] An example of quantum sensing by the NV centers is shown in Fig. 7, demonstrating the basic configuration used to probe the magnetic field that arose from the single nuclear spin of an atom, which is one of the constituents of a molecule. A single NV (blue arrow) embedded in a diamond substrate was used as a quantum sensor to probe the field coming from a molecule placed on the surface [Fig. 7(a)], while a single NV placed at the tip of a cantilever was used as a quantum sensor [Fig. 7(b)]. To realize such measurements, two criteria must be fulfilled: (i) the electron spin of each NV is tilted by 90° with respect to the direction of the externally applied magnetic field (B), so that it precesses around the B-field axis. The change in the precession rate is measured to probe the magnetic field arising from the nuclear spins of the molecule, thus, longer precession times have better sensitivities. The precession hold time decays exponentially and the coherence time (T ₂*) for the DC measurements and the T ₂ for the AC measurements are the relevant figures-of-merit because the minimum magnetic field required is proportional to $1/\sqrt {{T_2}} $. (ii) Because the magnetic field strength decreases by 1/d ³, where d is the distance, the distance between the target nuclear spins and the NV electron sensor must be reduced, preferably to the order of a few nanometers, to detect single nuclear spins. The NV center has advantages over other quantum system because its single-electron spin, T ₂, is long and can be measured at room temperature. Because an NV electron is spatially confined within 1 nm, it can be placed within a few nanometers from the target. Therefore, research leading to the application of NV centers for magnetic sensing has become very active recently^[Reference Laraoui, Hodges and Meriles^117–Reference Cooper, Magesan, Yum and Cappellaro^128] and the use of such sensors in solid-state physics research is emerging.^[Reference Bouchard, Acosta, Bauch and Budker^129–Reference Tetienne, Hingant, Kim, Herrera Diez, Adam, Garcia, Roch, Rohart, Thiaville, Ravelosona and Jacques^131] Because the electronic levels of NVs are affected by temperature^[Reference Acosta, Bauch, Ledbetter, Waxman, Bouchard and Budker^132] and other external perturbations, NVs can be used to sense temperature,^[Reference Kucsko, Maurer, Yao, Kubo, Noh, Lo, Park and Lukin^133–Reference Neumann, Jakobi, Dolde, Burk, Reuter, Waldherr, Honert, Wolf, Brunner, Shim, Suter, Sumiya, Isoya and Wrachtrup^135] electric fields,^[Reference Dolde, Fedder, Doherty, Nobauer, Rempp, Balasubramanian, Wolf, Reinhard, Hollenberg, Jelezko and Wrachtrup^136] pressure,^[Reference Doherty, Struzhkin, Simpson, McGuinness, Meng, Stacey, Karle, Hemley, Manson, Hollenberg and Prawer^137] and mechanical motions.^[Reference Kolkowitz, Jayich, Unterreithmeier, Bennett, Rabl, Harris and Lukin^138] Moreover, diamond is biocompatible and harmless, allowing for in-vivo quantum sensing in biology and medicine.^[Reference McGuinness, Yan, Stacey, Simpson, Hall, Maclaurin, Prawer, Mulvaney, Wrachtrup, Caruso, Scholten and Hollenberg^139–Reference Hall, Beart, Thomas, Simpson, McGuinness, Cole, Manton, Scholten, Jelezko, Wrachtrup, Petrou and Hollenberg^141]

Figure 7. Sensing the magnetic field that arose from the nuclear spins of a molecule using (a) a single NV center embedded in a diamond substrate and (b) a single NV center embedded in the tip of a diamond cantilever.

Diamond isotope engineering for quantum sensing

Pioneering works on the isotope engineering of bulk diamonds have been performed at the National Institute for Research in Inorganic Materials (currently the National Institute of Materials Science) in Japan^[Reference Collins, Davies, Kanda and Woods^142] and at the General Electric Company, USA.^[Reference Anthony, Banholzer, Fleischer, Wei, Kuo, Thomas and Pryor^143] The isotope effect on the band gap was investigated at Waseda University using ¹³C isotopically enriched CVD diamond.^[Reference Yamaguchi, Yamashita, Tsutsumi and Kawarada^144] The National Institute of Advanced Industrial Science and Technology (AIST) in Japan pioneered an isotope superlattice consisting of alternating thin layers of ¹²C and ¹³C.^[Reference Watanabe, Nebel and Shikata^145–Reference Watanabe, Koretsune, Nakashima, Saito and Shikata^147]12C and ¹³C enriched methane gases used in CVD at AIST were obtained from Cambridge Isotope Laboratories, Inc. Similar to silicon, the coherence time of the NV centers was studied in isotopically controlled diamond as a function of the background ¹³C nuclear spin concentration.^[Reference Mizuochi, Neumann, Rempp, Beck, Jacques, Siyushev, Nakamura, Twitchen, Watanabe, Yamasaki, Jelezko and Wrachtrup^148] It was extended to ~2 ms at room temperature by isotopic enrichment with nuclear-spin-free ¹²C.^[Reference Balasubramanian, Neumann, Twitchen, Markham, Kolesov, Mizuochi, Isoya, Achard, Beck, Tissler, Jacques, Hemmer, Jelezko and Wrachtrup^15] Here, the isotopically enriched diamond grown by Element Six was employed. The long coherence time was maintained in 100 nm thick, isotopically enriched ¹²C CVD single-crystalline films where NV centers were introduced by nitrogen doping during growth.^[Reference Ishikawa, Fu, Santori, Acosta, Beausoleil, Watanabe, Shikata and Itoh^149] In the isotopically enriched, ¹²C CVD polycrystalline films, NV centers were introduced by nitrogen doping during growth.^[Reference Teraji, Taniguchi, Koizumi, Watanabe, Liao, Koide and Isoya^150–Reference Jahnke, Naydenov, Teraji, Koizumi, Umeda, Isoya and Jelezko^152] In the isotopically enriched ¹²C CVD films, NV centers were introduced by nitrogen ion implantation and post-implantation annealing.^[Reference Yamamoto, Umeda, Watanabe, Onoda, Markham, Twitchen, Naydenov, McGuinness, Teraji, Koizumi, Dolde, Fedder, Honert, Wrachtrup, Ohshima, Jelezko and Isoya^153, Reference Yamamoto, Muller, McGuinness, Teraji, Naydenov, Onoda, Ohshima, Wrachtrup, Jelezko and Isoya^154] Thus, the remaining challenge for the application of quantum sensors is placing negatively charged NV⁻ centers as close as possible to the diamond surface. If the NV⁻ center loses its paramagnetic electron spin, it becomes a neutral NV⁰ center and cannot function as a quantum sensor. This happens near the surface of the film (within 5 nm) because of the existence of surface states that can change significantly with the crystalline quality of the surface and variations in surface-terminating species.^[Reference Rondin, Dantelle, Slablab, Grosshans, Treussart, Bergonzo, Perruchas, Gacoin, Chaigneau, Chang, Jacques and Roch^155–Reference Grotz, Hauf, Dankerl, Naydenov, Pezzagna, Meijer, Jelezko, Wrachtrup, Stutzmann, Reinhard and Garrido^157] To improve the crystalline quality, CVD isotopically enriched ¹²C diamond films were used. Shallow NV⁻ centers can be introduced into isotopically enriched CVD ¹²C diamond films either by nitrogen doping during CVD,^[Reference Ishikawa, Fu, Santori, Acosta, Beausoleil, Watanabe, Shikata and Itoh^149, Reference Ohashi, Rosskopf, Watanabe, Loretz, Tao, Hauert, Tomizawa, Ishikawa, Ishi-Hayase, Shikata, Degen and Itoh^158] which is sometimes followed by electron beam irradiation to create vacancies,^[Reference Ohno, Heremans, Bassett, Myers, Toyli, Jayich, Palmstrom and Awschalom^159] or by ion implantation and annealing.^[Reference Orwa, Santori, Fu, Gibson, Simpson, Aharonovich, Stacey, Cimmino, Balog, Markham, Twitchen, Greentree, Beausoleil and Prawer^160]Figure 8 compares the typical NV⁻ electron T ₂ values, measured by the Hahn echo sequence at room temperature. Figure 8(a) shows an isotopically enriched ¹²C bulk crystal.^[Reference Balasubramanian, Neumann, Twitchen, Markham, Kolesov, Mizuochi, Isoya, Achard, Beck, Tissler, Jacques, Hemmer, Jelezko and Wrachtrup^15]Figure 8(b) shows a 100-nm-thick isotopically enriched ¹²C film^[Reference Ishikawa, Fu, Santori, Acosta, Beausoleil, Watanabe, Shikata and Itoh^149] and Fig. 8(c) displays a 5-nm-thick isotopically enriched ¹²C film.^[Reference Ohashi, Rosskopf, Watanabe, Loretz, Tao, Hauert, Tomizawa, Ishikawa, Ishi-Hayase, Shikata, Degen and Itoh^158] For the 100-nm-thick film, T ₂ ~ 1.7 ms [Fig. 8(b)]. This was the same as the bulk value of T ₂ ~ 1.7 ms [Fig 8(a)]. T ₂ ~ 45 µs in the 5-nm-thick film, which was shortened significantly by decoherence, most likely caused by the surface states. Making use of the long NV⁻ electron T ₂, extended by ¹²C enrichment, a single NV⁻ situated in the deep region (away from the surface) has been used to detect a single ¹³C nuclear spin situated either next to the NV^−[Reference Maurer, Kucsko, Latta, Jiang, Yao, Bennett, Pastawski, Hunger, Chisholm, Markham, Twitchen, Cirac and Lukin^161] or far away from it, but still in the same diamond crystal.^[Reference Zhao, Honert, Schmid, Klas, Isoya, Markham, Twitchen, Jelezko, Liu, Fedder and Wrachtrup^162] In a similar manner, a shallow NV⁻ placed near the surface should be able to detect nuclear fields that originate from the nuclear spins on the surface of the diamond. In this regard, the T ₂ ~ 45 µs in the 5-nm-thick film was long enough (at least theoretically) to detect a magnetic field from a single-proton nuclear spin.^[Reference Ohashi, Rosskopf, Watanabe, Loretz, Tao, Hauert, Tomizawa, Ishikawa, Ishi-Hayase, Shikata, Degen and Itoh^158] There have been two types of isotopically enriched, ¹²C diamond CVD films containing shallow NV⁻ centers that have succeeded in detecting the magnetic field from a small ensemble of proton nuclear spins; one was produced by UC Santa Barbara (UCSB)^[Reference Ohno, Heremans, Bassett, Myers, Toyli, Jayich, Palmstrom and Awschalom^159] and the other was fabricated by the Keio University–AIST collaboration in Japan.^[Reference Ohashi, Rosskopf, Watanabe, Loretz, Tao, Hauert, Tomizawa, Ishikawa, Ishi-Hayase, Shikata, Degen and Itoh^158] While the UCSB sample was employed successfully by the IBM Almaden Research Center to detect the NMR of the proton spins confined in 24 nm³ of polymethyl methacrylate (PMMA), placed directly on the diamond surface,^[Reference Mamin, Kim, Sherwood, Rettner, Ohno, Awschalom and Rugar^163] the Keio–AIST sample succeeded in (along with the Keio–AIST–ETH collaboration) detecting ~6000 nuclear spins in an emulsion oil placed on the surface of diamond.^[Reference Ohashi, Rosskopf, Watanabe, Loretz, Tao, Hauert, Tomizawa, Ishikawa, Ishi-Hayase, Shikata, Degen and Itoh^158] The nanoscale NMRs of the protons placed on the diamond surface were also demonstrated using shallow NV⁻ centers created in natural diamond.^[Reference Staudacher, Shi, Pezzagna, Meijer, Du, Meriles, Reinhard and Wrachtrup^164, Reference Loretz, Pezzagna, Meijer and Degen^165] Because the coherence time of the shallow NV⁻ is currently limited by some sorts of defects near the surface rather than by the presence of ¹³C nuclear spins, advantage of isotopically enriched ¹²C is limited to the absence of oscillations in the echo decay (ESEEM) of the NV electron precession arising from the precession of the nearby ¹³C nuclear spins. ESEEM is an additional noise that occurs when the nuclear spins on the surface are the targets for detection. However, the coherence times (T ₂) of the shallow NVs in both natural and ¹²C diamonds are limited by the fluctuations that arise from the surface electronic states. At present, efforts are focused on identifying and understanding the nature of the surface electron spins using single shallow NV⁻ centers as a sensor.^[Reference Grinolds, Maletinsky, Hong, Lukin, Walsworth and Yacoby^166–Reference Myers, Das, Dartiailh, Ohno, Awschalom and Bleszynski Jayich^168] However, for the noise spectra, such as the intensity of the detected AC magnetic fields versus AC frequency, there is a disagreement between publications, indicating that the near-surface spin states are different for samples that have been prepared differently. Advancements in the materials science of diamond thin-film growth and surface preparation are needed to understand why these differences occur. One interesting direction explored recently was investigating the surface orientation rather than the (100) direction, which is usually studied; recently the (111) surface has been extensively studied.^[Reference Michl, Teraji, Zaiser, Jakobi, Waldherr, Dolde, Neumann, Doherty, Manson, Isoya and Wrachtrup^169–Reference Lesik, Tetienne, Tallaire, Achard, Mille, Gicquel, Roch and Jacques^171] Other defects in diamond, such as silicon vacancy complexes, may be of interest for sensing in the future. Such defects have been studied recently using isotopically enriched ¹²C diamond.^[Reference Rogers, Jahnke, Doherty, Dietrich, McGuinness, Muller, Teraji, Sumiya, Isoya, Manson and Jelezko^172]

Figure 8. Comparison of the typical NV⁻ electron T ₂ values measured by the Hahn echo sequence at room temperature in (a) an isotopically enriched ¹²C bulk crystal,^[15] (b) a 100-nm-thick isotopically enriched ¹²C film,^[149] and (c) a 5-nm-thick isotopically enriched ¹²C film.^[158]