Published online by Cambridge University Press: 06 April 2018
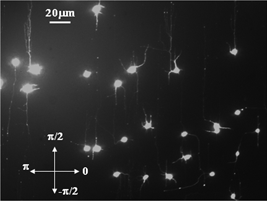
Geometrical features are known to be very important in neuronal growth and the formation of neuronal networks. We present an experimental and theoretical investigation of axonal growth and dynamics for neurons cultured on patterned polydimethylsiloxane surfaces. We utilize fluorescence microscopy to image the axonal dynamics and show that these substrates impart a strong directional bias to neuronal growth. We model axonal dynamics using a general stochastic model and use this framework to extract key dynamical parameters. These results provide novel insight into how geometrical cues influence neuronal growth and represent important advances toward bioengineering neuronal growth platforms.