Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T16:25:49.831Z Has data issue: false hasContentIssue false
  • English
  • Français

QUANTITATIVE ESTIMATES FOR SIMPLE ZEROS OF $L$-FUNCTIONS

Part of: Zeta and $L$-functions: analytic theory Discontinuous groups and automorphic forms

Published online by Cambridge University Press:  07 January 2019

Andrew R. Booker ,
Micah B. Milinovich  and
Nathan Ng
Andrew R. Booker
Affiliation:
School of Mathematics, University of Bristol, Bristol BS8 1TW, U.K. email [email protected]
Micah B. Milinovich
Affiliation:
Department of Mathematics, University of Mississippi, University, MS 38677, U.S.A. email [email protected]
Nathan Ng
Affiliation:
Department of Mathematics and Computer Science, University of Lethbridge, Lethbridge, AB T1K 3M4, Canada email [email protected]
Get access

Abstract

We generalize a method of Conrey and Ghosh [Simple zeros of the Ramanujan $\unicode[STIX]{x1D70F}$-Dirichlet series. Invent. Math. 94(2) (1988), 403–419] to prove quantitative estimates for simple zeros of modular form 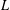$L$-functions of arbitrary conductor.

MSC classification

Primary: 11F66: Langlands $L$-functions; one variable Dirichlet series and functional equations 11F11: Holomorphic modular forms of integral weight 11M41: Other Dirichlet series and zeta functions
Type
Research Article
Information
Mathematika , Volume 65 , Issue 2 , 2019 , pp. 375 - 399
Copyright
Copyright © University College London 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

Research of the first author was supported by EPSRC Grant EP/K034383/1. Research of the second author was supported by the NSA Young Investigator Grants H98230-15-1-0231 and H98230-16-1-0311. Research of the third author was supported by NSERC Discovery Grant (RGPIN-2015-05972). No data were created in the course of this study.

References

Booker, A. R., Simple zeros of degree 2 L-functions. J. Eur. Math. Soc. (JEMS) 18(4) 2016, 813–823; MR 3474457.Google Scholar
Booker, A. R. and Krishnamurthy, M., A strengthening of the GL(2) converse theorem. Compos. Math. 147(3) 2011, 669–715; MR 2801397.Google Scholar
Booker, A. R., Milinovich, M. B. and Ng, N., Subconvexity for modular form L-functions in the t aspect. Adv. Math. 341 2019, 299–335; MR 3872849.Google Scholar
Coleman, M. D., A zero-free region for the Hecke L-functions. Mathematika 37(2) 1990, 287–304; MR 1099777.Google Scholar
Conrey, J. B. and Ghosh, A., Simple zeros of the Ramanujan 𝜏-Dirichlet series. Invent. Math. 94(2) 1988, 403–419; MR 958837 (89k:11078).Google Scholar
Iwaniec, H. and Kowalski, E., Analytic Number Theory (Colloquium Publications 53 ), American Mathematical Society (Providence, RI, 2004); MR 2061214.Google Scholar
Jacquet, H. and Shalika, J. A., A non-vanishing theorem for zeta functions of GL n . Invent. Math. 38(1) 1976/77, 1–16; MR 0432596 (55 #5583).Google Scholar
Kowalski, E., Michel, P. and VanderKam, J., Rankin–Selberg L-functions in the level aspect. Duke Math. J. 114(1) 2002, 123–191; MR 1915038.Google Scholar
Milinovich, M. B. and Ng, N., Simple zeros of modular L-functions. Proc. Lond. Math. Soc. (3) 109(6) 2014, 1465–1506; MR 3293156.Google Scholar
Munshi, R., Sub-Weyl bounds for $\text{GL}(2)\;L$ -functions. Preprint, 2018, arXiv:1806.07352.Google Scholar
Weil, A., Über die Bestimmung Dirichletscher Reihen durch Funktionalgleichungen. Math. Ann. 168 1967, 149–156; MR 0207658 (34 #7473).Google Scholar