Hostname: page-component-78c5997874-s2hrs Total loading time: 0 Render date: 2024-11-05T21:16:22.416Z Has data issue: false hasContentIssue false
  • English
  • Français

On Integer Sets Containing Strings of Consecutive Integers

Published online by Cambridge University Press:  26 February 2010

Adolf Hildebrand
Adolf Hildebrand
Affiliation:
Department of Mathematics, University of Illinois, Urbana, IL 61801, U.S.A.
Get access

Extract

By a well-known theorem of Szemerdi 8 any set of integers that has positive density contains arithmetic progressions of arbitrary length. One might expect that there are conditions of similar generality, under which an integer set contains arbitrarily long strings of consecutive integers, i.e., arithmetic progressions with 1 as common difference. Results of this kind would be of great importance because of potential applications to arithmetically interesting sets such as the set n: (n) = 1, where (n) is the Liouville function, or the sets

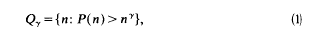
where P(n) denotes the greatest prime factor of n and 0< < 1. One naturally expects that such sets contain arbitrarily long strings of consecutive integers, but no results in this direction are known, and the problem seems to be a very difficult one, perhaps comparable in depth to the prime k-tuple conjecture.

Type
Research Article
Information
Mathematika , Volume 36 , Issue 1 , June 1989 , pp. 60 - 70
Copyright
Copyright University College London 1989

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

1.Balog, A.. Problem in: Tagungsbericht Math. Forschungsinstitut Oberwolfach, 41 (1982), p. 29.Google Scholar
2.de Bruijn, N. G.. On the number of positive integers x and free of prime factors >y. Nederl. Akad. Wetensch. Proc. Ser. A, 54 (1951), 5060.Google Scholar
3.De Koninck, J.-M., Ktai, I. and Mercier, A.. Additive functions and the largest prime factor of integers. Preprint.Google Scholar
4.Elliott, P. D. T. A.. Probabilistic Number Theory I (Springer-Verlag, New York, 1980).CrossRefGoogle Scholar
5.Heath-Brown, D. R.. The divisor function at consecutive integers. Mathematika, 31 (1984), 141149.CrossRefGoogle Scholar
6.Heath-Brown, D. R.. Consecutive almost-primes. J Indian Math. Soc., 52 (1987), 3949.Google Scholar
7.Hildebrand, A.. On a conjecture of Balog. Proc. Amer. Math. Soc., 95 (1985), 517523.CrossRefGoogle Scholar
8.Szemeredi, E.. On sets of integers containing no k elements in arithmetic progression. Acta Aritk, 27 (1975), 199245.CrossRefGoogle Scholar