Hostname: page-component-cd9895bd7-dzt6s Total loading time: 0 Render date: 2024-12-25T13:49:16.715Z Has data issue: false hasContentIssue false
  • English
  • Français

ARITHMETIC ASPECTS OF SYMMETRIC EDGE POLYTOPES

Part of: Combinatorics Polytopes and polyhedra Computational aspects and applications Polynomials, rational functions

Published online by Cambridge University Press:  14 May 2019

Akihiro Higashitani ,
Katharina Jochemko  and
Mateusz Michałek
Akihiro Higashitani
Affiliation:
Department of Mathematics, Graduate School of Science, Kyoto Sangyo University, Kamigamo Motoyama, Kita-ku, Kyoto, 603-8555, Japan email [email protected]
Katharina Jochemko
Affiliation:
Department of Mathematics, Royal Institute of Technology (KTH), SE-100 44 Stockholm, Sweden email [email protected]
Mateusz Michałek
Affiliation:
Max Planck Institute for Mathematics in the Sciences, Inselstr. 22, 04103 Leipzig, Germany email [email protected] Institute of Mathematics of the Polish Academy of Sciences, ul. Śniadeckich 8, 00-656 Warszawa, Poland
Get access

Abstract

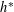We investigate arithmetic, geometric and combinatorial properties of symmetric edge polytopes. We give a complete combinatorial description of their facets. By combining Gröbner basis techniques, half-open decompositions and methods for interlacing polynomials we provide an explicit formula for the $h^{\ast }$-polynomial in case of complete bipartite graphs. In particular, we show that the 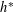$h^{\ast }$-polynomial is $\unicode[STIX]{x1D6FE}$-positive and real-rooted. This proves Gal’s conjecture for arbitrary flag unimodular triangulations in this case, and, beyond that, we prove a strengthening due to Nevo and Petersen [On 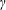$\unicode[STIX]{x1D6FE}$-vectors satisfying the Kruskal–Katona inequalities. Discrete Comput. Geom.45(3) (2011), 503–521].

MSC classification

Primary: 05A15: Exact enumeration problems, generating functions 52B12: Special polytopes (linear programming, centrally symmetric, etc.)
Secondary: 13P10: Gröbner bases; other bases for ideals and modules (e.g., Janet and border bases) 26C10: Polynomials 52B15: Symmetry properties of polytopes 52B20: Lattice polytopes (including relations with commutative algebra and algebraic geometry)
Type
Research Article
Information
Mathematika , Volume 65 , Issue 3 , 2019 , pp. 763 - 784
Copyright
Copyright © University College London 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

References

Batyrev, V. V., Dual polyhedra and mirror symmetry for Calabi–Yau hypersurfaces in toric varieties. J. Algebraic Geom. 3 1994, 493–545.Google Scholar
Beck, M., Jochemko, K. and McCullough, E., h ∗ -polynomials of zonotopes. Trans. Amer. Math. Soc. 371 2019, 2021–2042.Google Scholar
Beck, M. and Robins, S., Computing the Continuous Discretely, 2nd edn. (Undergraduate Texts in Mathematics), Springer (New York, NY, 2015).Google Scholar
Blind, R. and Mani-Levitska, P., Puzzles and polytope isomorphisms. Aequationes Math. 34(2–3) 1987, 287–297.Google Scholar
Brändén, P., Unimodality, log-concavity, real-rootedness and beyond. In Handbook of Enumerative Combinatorics (Discrete Mathematics and Its Applications), CRC Press (Boca Raton, FL, 2015), 437–483.Google Scholar
Bump, D., Choi, K.-K., Kurlberg, P. and Vaaler, J., A local Riemann hypothesis. I. Math. Z. 233(1) 2000, 1–19.Google Scholar
Charney, R. and Davis, M., The Euler characteristic of a nonpositively curved, piecewise Euclidean manifold. Pacific J. Math. 171(1) 1995, 117–137.Google Scholar
Craven, T. and Csordas, G., The Fox-Wright functions and Laguerre multiplier sequences. J. Math. Anal. Appl. 314(1) 2006, 109–125.Google Scholar
Dantzig, G. B., Linear Programming and Extensions, Princeton University Press (Princeton, NJ, 1963).Google Scholar
Davis, M. W., Dymara, J., Januszkiewicz, T. and Okun, B., Weighted L2–cohomology of Coxeter groups. Geom. Topol. 11(1) 2007, 47–138.Google Scholar
Deza, M. M. and Laurent, M., Geometry of Cuts and Metrics, reprint of 1997 original edn. (Algorithms and Combinatorics 15 ), Springer (Heidelberg, 2010); MR 1460488.Google Scholar
Dupont, L. A. and Villarreal, R. H., Symbolic Rees algebras, vertex covers and irreducible representations of Rees cones. Algebra Discrete Math. 10(2) 2011, 64–86, 2010.Google Scholar
Ehrhart, E., Sur les polyèdres rationnels homothétiques a n dimensions. C. R. Acad. Sci. Paris 254 1962, 616–618.Google Scholar
Fisk, S., Polynomials, roots, and interlacing. Preprint, 2006, arXiv:math/0612833.Google Scholar
Frankl, P., Füredi, Z. and Kalai, G., Shadows of colored complexes. Math. Scand. 63(2) 1988, 169–178.Google Scholar
Gal, Ś. R., On normal subgroups of Coxeter groups generated by standard parabolic subgroups. Geom. Dedicata 115 2005, 65–78.Google Scholar
Gal, S. R., Real root conjecture fails for five-and higher-dimensional spheres. Discrete Comput. Geom. 34(2) 2005, 269–284.Google Scholar
Hibi, T., Dual polytopes of rational convex polytopes. Combinatorica 12(2) 1992, 237–240.Google Scholar
Hibi, T., Li, N. and Zhang, Y. X., Separating hyperplanes of edge polytopes. J. Combin. Theory Ser. A 120(1) 2013, 218–231.Google Scholar
Higashitani, A., Smooth Fano polytopes arising from finite directed graphs. Kyoto J. Math. 55(3) 2015, 579–592.Google Scholar
Higashitani, A., Kummer, M. and Michałek, M., Interlacing Ehrhart polynomials of reflexive polytopes. Selecta Math. (N.S.) 23(4) 2017, 2977–2998.Google Scholar
Jochemko, K., On the real-rootedness of the Veronese construction for rational formal power series. Int. Math. Res. Not. IMRN 2018(15) 2018, 4780–4798.Google Scholar
Köppe, M. and Verdoolaege, S., Computing parametric rational generating functions with a primal Barvinok algorithm. Electron. J. Combin. 15(1) 2008, R16.Google Scholar
Lovász, L., Normal hypergraphs and the perfect graph conjecture. Discrete Math. 2(3) 1972, 253–267.Google Scholar
Marcus, A. W., Spielman, D. A. and Srivastava, N., Interlacing families II: mixed characteristic polynomials and the Kadison-Singer problem. Ann. of Math. (2) 182(1) 2015, 327–350.Google Scholar
Matsui, T., Higashitani, A., Nagazawa, Y., Ohsugi, H. and Hibi, T., Roots of Ehrhart polynomials arising from graphs. J. Algebraic Combin. 34(4) 2011, 721–749.Google Scholar
Michałek, M., Selected topics on toric varieties. In The 50th Anniversary of Gröbner Bases (Advanced Studies in Pure Mathematics) (ed. Hibi, T.), Mathematical Society of Japan (Tokyo, 2018), 207–252.Google Scholar
Nevo, E. and Petersen, T. K., On 𝛾-vectors satisfying the Kruskal–Katona inequalities. Discrete Comput. Geom. 45(3) 2011, 503–521.Google Scholar
Obreschkoff, N., Verteilung und Berechnung der Nullstellen reeller Polynome, VEB Deutscher Verlag der Wissenschaften (Berlin, 1963).Google Scholar
Ohsugi, H. and Hibi, T., Normal polytopes arising from finite graphs. J. Algebra 207(2) 1998, 409–426.Google Scholar
Polya, G. and Schur, J., Über zwei Arten von Faktorenfolgen in der Theorie der algebraischen Gleichungen. J. Reine Angew. Math. 144 1914, 89–113.Google Scholar
Postnikov, A., Permutohedra, associahedra, and beyond. Int. Math. Res. Not. IMRN 2009(6) 2009, 1026–1106.Google Scholar
Santos, F., A counterexample to the Hirsch conjecture. Ann. of Math. (2) 176(1) 2012, 383–412.Google Scholar
Savage, C. and Visontai, M., The s-eulerian polynomials have only real roots. Trans. Amer. Math. Soc. 367(2) 2015, 1441–1466.Google Scholar
Solus, L., Simplices for numeral systems. Trans. Amer. Math. Soc. 371(3) 2019, 2089–2107.Google Scholar
Stanley, R. P., Decompositions of rational convex polytopes. Ann. Discrete Math. 6 1980, 333–342.Google Scholar
Stanley, R. P., Log-concave and unimodal sequences in algebra, combinatorics, and geometry. Ann. N. Y. Acad. Sci. 576 1989, 500–535.Google Scholar
Steinitz, E., Polyeder und Raumeinteilungen. In Enzyklopädie der mathematischen Wissenschaften, B.G. Teubner (Leipzig, 1922), III.1.2(9): 1–139.Google Scholar
Sturmfels, B., Gröbner Bases and Convex Polytopes, Vol. 8, American Mathematical Society (Providence, RI, 1996).Google Scholar
Tran, T. and Ziegler, G. M., Extremal edge polytopes. Electron. J. Combin. 21(2) 2014, P2.57.Google Scholar
Ziegler, G. M., Lectures on Polytopes, Springer (New York, NY, 1995).Google Scholar