Hostname: page-component-cd9895bd7-jn8rn Total loading time: 0 Render date: 2024-12-25T14:22:11.788Z Has data issue: false hasContentIssue false
  • English
  • Français

A COUNTEREXAMPLE TO A CONJECTURE OF LARMAN AND ROGERS ON SETS AVOIDING DISTANCE 1

Part of: Discrete geometry Distance geometry

Published online by Cambridge University Press:  14 May 2019

Fernando Mário de Oliveira Filho  and
Frank Vallentin
Fernando Mário de Oliveira Filho
Affiliation:
Delft Institute of Applied Mathematics, Delft University of Technology, Van Mourik Broekmanweg 6, 2628 XE Delft, The Netherlands email [email protected]
Frank Vallentin
Affiliation:
Mathematisches Institut, Universität zu Köln, Weyertal 86–90, 50931 Köln, Germany email [email protected]
Get access

Abstract

For each 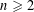$n\geqslant 2$ we construct a measurable subset of the unit ball in 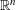$\mathbb{R}^{n}$ that does not contain pairs of points at distance 1 and whose volume is greater than $(1/2)^{n}$ times the volume of the unit ball. This disproves a conjecture of Larman and Rogers from 1972.

MSC classification

Primary: 52C10: Erdős problems and related topics of discrete geometry 51K99: None of the above, but in this section
Type
Research Article
Information
Mathematika , Volume 65 , Issue 3 , 2019 , pp. 785 - 787
Copyright
Copyright © University College London 2019 

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The second author was partially supported by the SFB/TRR 191 “Symplectic Structures in Geometry, Algebra and Dynamics”, funded by the DFG, and the European Union’s Horizon 2020 research and innovation programme under the Marie Skłodowska-Curie agreement number 764759.

References

Ball, K., An elementary introduction to modern convex geometry. In Flavors of Geometry (Mathematical Sciences Research Institute Publications 31 ), Cambridge University Press (Cambridge, 1997), 1–58.Google Scholar
Blum, A., Hopcroft, J. and Kannan, R., Foundations of Data Science, 2018, http://www.cs.cornell.edu/jeh.Google Scholar
Croft, H. T., Falconer, K. J. and Guy, R., Unsolved Problems in Geometry, Springer (New York, 1991).Google Scholar
DeCorte, E., de Oliveira Filho, F. M. and Vallentin, F., Complete positivity and distance-avoiding sets. Preprint, 2018, arXiv:1804:09099.Google Scholar
Kalai, G., Some old and new problems in combinatorial geometry I: around Borsuk’s problem. In Surveys in Combinatorics 2015 (London Mathematical Society Lecture Note Series 424 ), Cambridge University Press (Cambridge, 2015), 147–174.Google Scholar
Larman, D. G. and Rogers, C. A., The realization of distances within sets in Euclidean space. Mathematika 19 1972, 1–24.Google Scholar
Matoušek, J., Lectures on Discrete Geometry (Graduate Texts in Mathematics 212 ), Springer (New York, 2002).Google Scholar