We aim to re-prove a theorem conjectured by Gauss, namely there are exactly nine imaginary quadratic fields 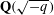$\mathbf{Q}(\sqrt{-q})$ with class number one: specifically the list is 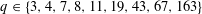$q\in \{3,4,7,8,11,19,43,67,163\}$. Our method initially follows an idea of Goldfeld, but rather than using an elliptic curve of analytic rank three (provided by the Gross–Zagier theorem), we instead use an elliptic curve of analytic rank two, where this 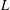$L$-function vanishing can be proven by modular symbols rather than a difficult height formula. It is already clear that Goldfeld’s work yields a constant lower bound for the class number by such means, but unfortunately it seems that even for the best choice of elliptic curve this numerical constant is less than 1, unless one can show non-trivial cancellation in the 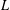$L$-function coefficients restricted to values taken by quadratic forms. To show the latter, we consider a specific analytic rank-two elliptic curve with complex multiplication by 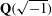$\mathbf{Q}(\sqrt{-1})$, and then by adapting a result of Hooley’s regarding equi-distrbution of roots of a quadratic polynomial to varying moduli, are able to show that there is indeed sufficient coefficient cancellation, giving an effective resolution of class number one. As we use various aspects of the principal form, our proof seems inapplicable for larger class numbers. We also comment on the possibility of using spectral techniques (following Templier and Tsimerman) to show the desired coefficient cancellation, though postpone the details of this to elsewhere.